Skip to main content
SpringerLink
Log in

A low computational cost eight algebraic order hybrid method with vanished phase-lag and its first, second, third and fourth derivatives for the approximate solution of the Schrödinger equation

  • Original Paper
  • Published:
Journal of Mathematical Chemistry Aims and scope Submit manuscript

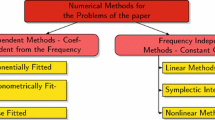
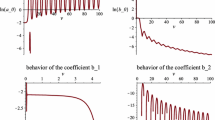
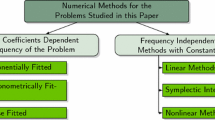
Abstract

A low computational cost eighth algebraic order hybrid two-step method with vanished phase-lag and its first, second, third and fourth derivatives is developed in this paper. We also investigate the local truncation error, the stability and the result of the elimination of the phase-lag and its derivatives on the effectiveness of the produced method.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2

Similar content being viewed by others

Notes

  1. where \(S\) is a set of distinct points

References

  1. Z.A. Anastassi, T.E. Simos, A parametric symmetric linear four-step method for the efficient integration of the Schrödinger equation and related oscillatory problems. J. Comput. Appl. Math. 236, 3880–3889 (2012)

    Article  Google Scholar 

  2. A.D. Raptis, T.E. Simos, A four-step phase-fitted method for the numerical integration of second order initial-value problem. BIT 31, 160–168 (1991)

    Article  Google Scholar 

  3. D.G. Quinlan, S. Tremaine, Symmetric multistep methods for the numerical integration of planetary orbits. Astron. J. 100(5), 1694–1700 (1990)

    Article  Google Scholar 

  4. J.M. Franco, M. Palacios, J. Comput. Appl. Math. 30, 1 (1990)

    Article  Google Scholar 

  5. J.D. Lambert, Numerical Methods for Ordinary Differential Systems, The Initial Value Problem (Wiley, Chichester, 1991)

  6. E. Stiefel, D.G. Bettis, Stabilization of Cowell’s method. Numer. Math. 13, 154–175 (1969)

    Article  Google Scholar 

  7. G.A. Panopoulos, Z.A. Anastassi, T.E. Simos, Two new optimized eight-step symmetric methods for the efficient solution of the Schrödinger equation and related problems. MATCH Commun. Math. Comput. Chem. 60(3), 773–785 (2008)

    CAS  Google Scholar 

  8. G.A. Panopoulos, Z.A. Anastassi, T.E. Simos, Two optimized symmetric eight-step implicit methods for initial-value problems with oscillating solutions. J. Math. Chem. 46(2), 604–620 (2009)

    Article  CAS  Google Scholar 

  9. http://www.burtleburtle.net/bob/math/multistep.html

  10. T.E. Simos, P.S. Williams, Bessel and Neumann fitted methods for the numerical solution of the radial Schrödinger equation. Comput. Chem. 21, 175–179 (1977)

    Article  Google Scholar 

  11. T.E. Simos, J. Vigo-Aguiar, A dissipative exponentially-fitted method for the numerical solution of the Schrödinger equation and related problems. Comput. Phys. Commun. 152, 274–294 (2003)

    Article  CAS  Google Scholar 

  12. T.E. Simos, G. Psihoyios, J. Comput. Appl. Math. 175(1), IX–IX (2005)

  13. T. Lyche, Chebyshevian multistep methods for ordinary differential eqations. Numer. Math. 19, 65–75 (1972)

    Article  Google Scholar 

  14. T.E. Simos, P.S. Williams, A finite-difference method for the numerical solution of the Schrödinger equation. J. Comput. Appl. Math. 79, 189205 (1997)

    Article  Google Scholar 

  15. R.M. Thomas, Phase properties of high order almost P-stable formulae. BIT 24, 225238 (1984)

    Article  Google Scholar 

  16. J.D. Lambert, I.A. Watson, Symmetric multistep methods for periodic initial values problems. J. Inst. Math. Appl. 18, 189202 (1976)

    Article  Google Scholar 

  17. A. Konguetsof, T.E. Simos, A generator of hybrid symmetric four-step methods for the numerical solution of the Schrödinger equation. J. Comput. Appl. Math. 158(1), 93–106 (2003)

    Article  Google Scholar 

  18. Z. Kalogiratou, T. Monovasilis, T.E. Simos, Symplectic integrators for the numerical solution of the Schrödinger equation. J. Comput. Appl. Math. 158(1), 83–92 (2003)

    Article  Google Scholar 

  19. Z. Kalogiratou, T.E. Simos, Newton-Cotes formulae for long-time integration. J. Comput. Appl. Math. 158(1), 75–82 (2003)

    Article  Google Scholar 

  20. G. Psihoyios, T.E. Simos, Trigonometrically fitted predictor-corrector methods for IVPs with oscillating solutions. J. Comput. Appl. Math. 158(1), 135–144 (2003)

    Article  Google Scholar 

  21. T.E. Simos, I.T. Famelis, C. Tsitouras, Zero dissipative, explicit Numerov-type methods for second order IVPs with oscillating solutions. Numer. Algorithms 34(1), 27–40 (2003)

    Article  Google Scholar 

  22. T.E. Simos, Dissipative trigonometrically-fitted methods for linear second-order IVPs with oscillating solution. Appl. Math. Lett. 17(5), 601–607 (2004)

    Article  Google Scholar 

  23. K. Tselios, T.E. Simos, Runge–Kutta methods with minimal dispersion and dissipation for problems arising from computational acoustics. J. Comput. Appl. Math. 175(1), 173–181 (2005)

    Article  Google Scholar 

  24. D.P. Sakas, T.E. Simos, Multiderivative methods of eighth algrebraic order with minimal phase-lag for the numerical solution of the radial Schrödinger equation. J. Comput. Appl. Math. 175(1), 161–172 (2005)

    Article  Google Scholar 

  25. G. Psihoyios, T.E. Simos, A fourth algebraic order trigonometrically fitted predictor-corrector scheme for IVPs with oscillating solutions. J. Comput. Appl. Math. 175(1), 137–147 (2005)

    Article  Google Scholar 

  26. Z.A. Anastassi, T.E. Simos, An optimized Runge–Kutta method for the solution of orbital problems. J. Comput. Appl. Math. 175(1), 1–9 (2005)

    Article  Google Scholar 

  27. T.E. Simos, Closed Newton–Cotes trigonometrically-fitted formulae of high order for long-time integration of orbital problems. Appl. Math. Lett 22(10), 1616–1621 (2009)

    Article  Google Scholar 

  28. S. Stavroyiannis, T.E. Simos, Optimization as a function of the phase-lag order of nonlinear explicit two-step P-stable method for linear periodic IVPs. Appl. Numer. Math. 59(10), 2467–2474 (2009)

    Article  Google Scholar 

  29. T.E. Simos, Exponentially and trigonometrically fitted methods for the solution of the Schrödinger equation. Acta Appl. Math. 110(3), 1331–1352 (2010)

    Article  Google Scholar 

  30. T. E. Simos, New stable closed Newton–Cotes trigonometrically fitted formulae for long-time integration. Abstr. Appl. Anal. 2012, Article ID 182536, 15 (2012). doi:10.1155/2012/182536

  31. T.E. Simos, Optimizing a hybrid two-step method for the numerical solution of the Schrödinger equation and related problems with respect to phase-lag. J. Appl. Math. 2012, Article ID 420387, 17 (2012). doi:10.1155/2012/420387

  32. Z.A. Anastassi, T.E. Simos, A parametric symmetric linear four-step method for the efficient integration of the Schrödinger equation and related oscillatory problems. J. Comput. Appl. Math. 236, 38803889 (2012)

    Article  Google Scholar 

  33. D.F. Papadopoulos, T.E. Simos, A modified Runge–Kutta-Nyström method by using phase lag properties for the numerical solution of orbital problems. Appl. Math. Inf. Sci. 7(2), 433–437 (2013)

    Article  Google Scholar 

  34. T. Monovasilis, Z. Kalogiratou, T.E. Simos, Exponentially fitted symplectic Runge–Kutta–Nyström methods. Appl. Math. Inf. Sci. 7(1), 81–85 (2013)

    Article  Google Scholar 

  35. G.A. Panopoulos, T.E. Simos, An optimized symmetric 8-step semi-embedded predictor–corrector method for IVPs with oscillating solutions. Appl. Math. Inf. Sci. 7(1), 73–80 (2013)

    Article  Google Scholar 

  36. D.F. Papadopoulos, T.E Simos, The use of phase lag and amplification error derivatives for the construction of a modified Runge–Kutta–Nyström method. Abstr. Appl. Anal. Article Number: 910624 (2013)

  37. I. Alolyan, Z.A. Anastassi, T.E. Simos, A new family of symmetric linear four-step methods for the efficient integration of the Schrödinger equation and related oscillatory problems. Appl. Math. Comput. 218(9), 5370–5382 (2012)

    Article  Google Scholar 

  38. I. Alolyan, T.E. Simos, A family of high-order multistep methods with vanished phase-lag and its derivatives for the numerical solution of the Schrödinger equation. Comput. Math. Appl. 62(10), 3756–3774 (2011)

    Article  Google Scholar 

  39. C. Tsitouras, I.T. Famelis, T.E. Simos, On modified Runge–Kutta trees and methods. Comput. Math. Appl. 62(4), 2101–2111 (2011)

    Article  Google Scholar 

  40. A.A. Kosti, Z.A. Anastassi, T.E. Simos, Construction of an optimized explicit Runge–Kutta-Nyström method for the numerical solution of oscillatory initial value problems. Comput. Math. Appl. 61(11), 3381–3390 (2011)

    Article  Google Scholar 

  41. Z. Kalogiratou, T. Monovasilis, T.E. Simos, New modified Runge–Kutta–Nystrom methods for the numerical integration of the Schrödinger equation. Comput. Math. Appl. 60(6), 1639–1647 (2010)

    Article  Google Scholar 

  42. T. Monovasilis, Z. Kalogiratou, T.E. Simos, A family of trigonometrically fitted partitioned Runge–Kutta symplectic methods. Appl. Math. Comput. 209(1), 91–96 (2009)

    Article  Google Scholar 

  43. T.E. Simos, High order closed Newton–Cotes trigonometrically-fitted formulae for the numerical solution of the Schrödinger equation. Appl. Math. Comput. 209(1), 137–151 (2009)

    Article  Google Scholar 

  44. A. Konguetsof, T.E. Simos, An exponentially-fitted and trigonometrically-fitted method for the numerical solution of periodic initial-value problems. Comput. Math. Appl. 45(1–3), 547–554 Article Number: PII S0898–1221(02)00354–1 (2003)

  45. T.E. Simos, On the explicit four-step methods with vanished phase-lag and its first derivative. Appl. Math. Inf. Sci. 8(2), 447–458 (2014)

    Article  Google Scholar 

  46. G.A. Panopoulos, T.E. Simos, A new optimized symmetric embedded predictor–corrector method (EPCM) for initial-value problems with oscillatory solutions. Appl. Math. Inf. Sci. 8(2), 703–713 (2014)

    Article  Google Scholar 

  47. L. Gr. Ixaru, M. Rizea, Comparison of some four-step methods for the numerical solution of the Schrödinger equation. Comput. Phys. Commun. 38(3), 329–337 (1985)

    Article  Google Scholar 

  48. L.G. Ixaru, M. Micu, Topics in Theoretical Physics (Central Institute of Physics, Bucharest, 1978)

    Google Scholar 

  49. L.G. Ixaru, M. Rizea, A Numerov-like scheme for the numerical solution of the Schrödinger equation in the deep continuum spectrum of energies. Comput. Phys. Commun. 19, 23–27 (1980)

    Article  Google Scholar 

  50. J.R. Dormand, M.E.A. El-Mikkawy, P.J. Prince, Families of Runge–Kutta–Nyström formulae. IMA J. Numer. Anal. 7, 235–250 (1987)

    Article  Google Scholar 

  51. J.R. Dormand, P.J. Prince, A family of embedded Runge Kutta formulae. J. Comput. Appl. Math. 6, 19–26 (1980)

    Article  Google Scholar 

  52. G.D. Quinlan, S. Tremaine, Symmetric multistep methods for the numerical integration of planetary orbits. Astron. J. 100, 1694–1700 (1990)

    Article  Google Scholar 

  53. A.D. Raptis, A.C. Allison, Exponential-fitting methods for the numerical solution of the Schrödinger equation. Comput. Phys. Commun. 14, 1–5 (1978)

    Article  Google Scholar 

  54. M.M. Chawla, P.S. Rao, An Noumerov-typ method with minimal phase-lag for the integration of second order periodic initial-value problems II Explicit Method. J. Comput. Appl. Math. 15, 329–337 (1986)

    Article  Google Scholar 

  55. M.M. Chawla, P.S. Rao, An explicit sixth-order method with phase-lag of order eight for \(y^{\prime \prime }=f(t, y)\). J. Comput. Appl. Math. 17, 363–368 (1987)

    Google Scholar 

  56. T.E. Simos, A new Numerov-type method for the numerical solution of the Schrödinger equation. J. Math. Chem. 46, 981–1007 (2009)

    Article  CAS  Google Scholar 

  57. A. Konguetsof, Two-step high order hybrid explicit method for the numerical solution of the Schrödinger equation. J. Math. Chem. 48, 224252 (2010)

    Article  Google Scholar 

  58. A.D. Raptis, J.R. Cash, A variable step method for the numerical integration of the one-dimensional Schrödinger equation. Comput. Phys. Commun. 36, 113–119 (1985)

    Article  CAS  Google Scholar 

  59. A.C. Allison, The numerical solution of coupled differential equations arising from the Schrödinger equation. J. Comput. Phys. 6, 378–391 (1970)

    Article  Google Scholar 

  60. R.B. Bernstein, A. Dalgarno, H. Massey, I.C. Percival, Thermal scattering of atoms by homonuclear diatomic molecules. Proc. R. Soc. Ser. A 274, 427–442 (1963)

    Article  CAS  Google Scholar 

  61. R.B. Bernstein, Quantum mechanical (phase shift) analysis of differential elastic scattering of molecular beams. J. Chem. Phys. 33, 795–804 (1960)

    Article  CAS  Google Scholar 

  62. T.E. Simos, Exponentially fitted Runge–Kutta methods for the numerical solution of the Schrödinger equation and related problems. Comput. Mater. Sci. 18, 315–332 (2000)

    Article  Google Scholar 

  63. J.R. Dormand, P.J. Prince, A family of embedded Runge–Kutta formula. J. Comput. Appl. Math. 6, 19–26 (1980)

    Article  Google Scholar 

  64. Jing Ma, T.E. Simos, Runge–Kutta type eighth algebraic order method with vanished phase-lag and its first, second and third derivatives for the numerical solution of the radial Schrödinger equation and related problems. Appl. Math. Inf. Sci. (in press)

Download references

Conflict of interest

The authors declare that they have no conflict of interest.

Author information

Authors and Affiliations

  1. School of information engineering, Chang’an University, Xi’an, 710064, China

    Hang Ning

  2. Department of Mathematics, College of Sciences, King Saud University, P. O. Box 2455, Riyadh, 11451, Saudi Arabia

    T. E. Simos

  3. Laboratory of Computational Sciences, Department of Informatics and Telecommunications, Faculty of Economy, Management and Informatics, University of Peloponnese, 221 00, Tripolis, Greece

    T. E. Simos

  4. 10 Konitsis Street, Amfithea - Paleon Faliron, 175 64, Athens, Greece

    T. E. Simos

Authors
  1. Hang Ning
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. T. E. Simos
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to T. E. Simos.

Additional information

Authors whose names appear on the submission have contributed sufficiently to the scientific work and therefore share collective responsibility and accountability for the results.

Highly Cited Researcher (http://isihighlycited.com/), Active Member of the European Academy of Sciences and Arts. Active Member of the European Academy of Sciences. Corresponding Member of European Academy of Arts, Sciences and Humanities.

Appendix: Formulae of the derivatives of \(q_{n}\)

Appendix: Formulae of the derivatives of \(q_{n}\)

Formulae of the derivatives which presented in the formulae of the LTEs:

$$\begin{aligned} y_{n}^{(2)} \,= & {} \, \left( V(x)-V_{c} + G\right) \, y(x) \\ y_{n}^{(3)} \,= & {} \, \left( {\frac{d}{dx}}g \left( x \right) \right) y \left( x \right) + \left( g \left( x \right) +G \right) {\frac{d}{dx}}y \left( x \right) \\ y_{n}^{(4)} \,= & {} \, \left( {\frac{d^{2}}{d{x}^{2}}}g \left( x \right) \right) y \left( x \right) +2\, \left( {\frac{d}{dx}}g \left( x \right) \right) {\frac{d}{dx}}y \left( x \right) \\&+ \left( g \left( x \right) +G \right) ^{2}y \left( x \right) \\ y_{n}^{(5)} \,= & {} \, \left( {\frac{d^{3}}{d{x}^{3}}}g \left( x \right) \right) y \left( x \right) +3\, \left( {\frac{d^{2}}{d{x}^{2}}}g \left( x \right) \right) {\frac{d}{dx}}y \left( x \right) \\&+\,4\, \left( g \left( x \right) +G \right) y \left( x \right) {\frac{d}{dx}}g \left( x \right) + \left( g \left( x \right) +G \right) ^{2}{\frac{d}{dx}}y \left( x \right) \\ y_{n}^{(6)} \,= & {} \, \left( {\frac{d^{4}}{d{x}^{4}}}g \left( x \right) \right) y \left( x \right) +4\, \left( {\frac{d^{3}}{d{x}^{3}}}g \left( x \right) \right) {\frac{d}{dx}}y \left( x \right) \\&+\,7\, \left( g \left( x \right) +G \right) y \left( x \right) {\frac{d^{2}}{d{x}^{2}}}g \left( x \right) +4\, \left( {\frac{d}{dx}}g \left( x \right) \right) ^{2}y \left( x \right) \\&+\,6\, \left( g \left( x \right) +G \right) \left( {\frac{d}{dx}}y \left( x \right) \right) {\frac{d}{dx}}g \left( x \right) + \left( g \left( x \right) +G \right) ^{3}y \left( x \right) \\ y_{n}^{(7)} \,= & {} \, \left( {\frac{d^{5}}{d{x}^{5}}}g \left( x \right) \right) y \left( x \right) +\,5\, \left( {\frac{d^{4}}{d{x}^{4}}}g \left( x \right) \right) {\frac{d}{dx}}y \left( x \right) \\&+\,11\, \left( g \left( x \right) +G \right) y \left( x \right) {\frac{d^{3}}{d{x}^{3}}}g \left( x \right) +\,15\, \left( {\frac{d}{dx}}g \left( x \right) \right) y \left( x \right) {\frac{d^{2}}{d{x}^{2}}}g \left( x \right) \\&+\,13\, \left( g \left( x \right) +G \right) \left( {\frac{d}{dx}}y \left( x \right) \right) {\frac{d^{2}}{d{x}^{2}}}g \left( x \right) \\&+\,10\, \left( {\frac{d}{dx}}g \left( x \right) \right) ^{2}{\frac{d}{dx}}y \left( x \right) +9\, \left( g \left( x \right) +G \right) ^{2}y \left( x \right) {\frac{d}{dx}}g \left( x \right) \\&+\, \left( g \left( x \right) +G \right) ^{3}{\frac{d}{dx}}y \left( x \right) \end{aligned}$$
$$\begin{aligned} y_{n}^{(8)} \,= & {} \, \left( {\frac{d^{6}}{d{x}^{6}}}g \left( x \right) \right) y \left( x \right) +6\, \left( {\frac{d^{5}}{d{x}^{5}}}g \left( x \right) \right) {\frac{d}{dx}}y \left( x \right) \\&+\,16\, \left( g \left( x \right) +G \right) y \left( x \right) {\frac{d^{4}}{d{x}^{4}}}g \left( x \right) +\,26\, \left( {\frac{d}{dx}}g \left( x \right) \right) y \left( x \right) {\frac{d^{3}}{d{x}^{3}}}g \left( x \right) \\&+\,24\, \left( g \left( x \right) +G \right) \left( {\frac{d}{dx}}y \left( x \right) \right) {\frac{d^{3}}{d{x}^{3}}}g \left( x \right) \\&+\,15\, \left( {\frac{d^{2}}{d{x}^{2}}}g \left( x \right) \right) ^{2}y \left( x \right) +48\, \left( {\frac{d}{dx}}g \left( x \right) \right) \left( {\frac{d}{dx}}y \left( x \right) \right) {\frac{d^{2}}{d{x}^{2}}}g \left( x \right) \\&+\,22\, \left( g \left( x \right) +G \right) ^{2}y \left( x \right) {\frac{d^{2}}{d{x}^{2}}}g \left( x \right) +28\, \left( g \left( x \right) +G \right) y \left( x \right) \left( {\frac{d}{dx}}g \left( x \right) \right) ^{2}\\&+\,12\, \left( g \left( x \right) +G \right) ^{2} \left( {\frac{d}{dx}}y \left( x \right) \right) {\frac{d}{dx}}g \left( x \right) + \left( g \left( x \right) +G \right) ^{4}y \left( x \right) \ldots \end{aligned}$$

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Ning, H., Simos, T. A low computational cost eight algebraic order hybrid method with vanished phase-lag and its first, second, third and fourth derivatives for the approximate solution of the Schrödinger equation. J Math Chem 53, 1295–1312 (2015). https://doi.org/10.1007/s10910-015-0489-3

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10910-015-0489-3

Keywords

Search

Navigation