Skip to main content
SpringerLink
Log in

Bell Length in the Entanglement Geometry

  • Published:
International Journal of Theoretical Physics Aims and scope Submit manuscript

Abstract

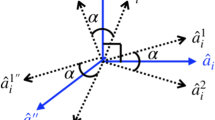
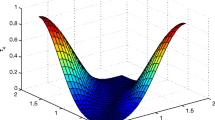
A geometric approach to entangled qubit pairs is outlined via Bohm Theory. An entropic quantum correlation distance is here proposed as a mark of the non-local “handshaking” between two systems under the action of Quantum Potential. The Bell-CHSH inequalities and Berry Phase are analyzed in terms of this new correlation measure we called Bell Length, in honour of J.S. Bell (1928-1990).

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Bohm, D.: A Suggested Interpretation of the Quantum Theory in Terms of “Hidden” Variables. I. Phys. Rev. 85, 166 (1952)

    Article  ADS  MATH  Google Scholar 

  2. Bohm, D.: A Suggested Interpretation of the Quantum Theory in Terms of “Hidden” Variables. II. Phys. Rev. 85, 180 (1952)

    Article  ADS  Google Scholar 

  3. Bohm, D., Hiley, B.: The Undivided Universe: An Ontological Interpretation of Quantum Theory. Routledge, London (1993)

    Google Scholar 

  4. Holland, P.R.: The Quantum Theory of Motion. Cambridge University Press, Cambridge (1995)

    MATH  Google Scholar 

  5. Dürr, D., Goldstein, S., Zanghì, N.: Quantum Physics Without Quantum Philosophy. Springer (2013)

  6. Licata, I., Fiscaletti, D.: Quantum Potential. Physics, Geometry, Algebra. Springer (2014)

  7. Bacciagaluppi, G., Valentini, A.: Quantum theory at the Crossroads: Reconsidering the 1927 Solvay Conference. Cambridge University Press, Cambridge (2009)

    Book  Google Scholar 

  8. Cushing, J.T.: Quantum mechanics: Historical Contingency and the Copenhagen Hegemony. Chicago University Press, Chicago (1994)

    MATH  Google Scholar 

  9. Fiscaletti, D.: The Quantum Entropy as an Ultimate Visiting Card of the de Broglie–Bohm Theory. Uk. J. Phys. 57(9), 946 (2012)

    Google Scholar 

  10. Resconi, G., Licata, I., Fiscaletti, D.: Unification of Quantum and Gravity by Non Classical Information Entropy Space. Entropy 15, 3602 (2013)

    Article  ADS  MathSciNet  Google Scholar 

  11. Licata, I., Fiscaletti, D.: Bohm Trajectories Feynman Paths in Light of Quantum Entropy. Acta. Phys. Pol. B 45(4), 885 (2014)

    Article  ADS  MathSciNet  Google Scholar 

  12. Licata, I., Fiscaletti, D.: Bell Length as Mutual Information in Quantum Interference. Axioms 3, 153 (2014)

    Article  Google Scholar 

  13. Sbitnev, V.: Bohmian split of the Schrodinger equation onto two equations describing evolution of real functions. Kvantovaya Magiya 5(1), 1101–1011 (2008)

    Google Scholar 

  14. Novello, M., Salim, J.M., Falciano, F.T.: On a Geometrical Description of Quantum Mechanics. Int. J. Geom. Meth. Mod. Phys 08, 87 (2011)

    Article  MathSciNet  Google Scholar 

  15. Novello, M., Salim, J.M., Falciano, F.T.: Geometrizing Relativistic Quantum Mechanics Found. Phys 40(12), 1885–1901 (2010)

    MATH  MathSciNet  Google Scholar 

  16. Fiscaletti, D., Licata, I.: Weyl Geometries, Fisher Information and Quantum Entropy in Quantum Mechanics. Int. J. Theor. Phys. 51(11), 3587–3595 (2012)

    Article  MATH  MathSciNet  Google Scholar 

  17. Holland, P.R.: Causal interpretation of a system of two spin-1/2. Phys. Rep. 169(5), 293–327 (1988)

    Article  ADS  MathSciNet  Google Scholar 

  18. Ramsak, A.: Geometrical view of quantum entanglement. EPL 96(4), 40004 (2011)

    Article  ADS  Google Scholar 

  19. Ramsak, A.: Spin–spin correlations of entangled qubit pairs in the Bohm interpretation of quantum mechanics. J. Phys. A: Math. Theor. 45(11), 115310 (2012)

    Article  ADS  MathSciNet  Google Scholar 

  20. Bell, J.S.: On the Einstein Podolsky Rosen Paradox. Physics 1,3, 195–200 (1964)

  21. Clauser, J.F., Horn, M.A., Shimony, A., Holt, R.A.: Proposed Experiment to Test Local Hidden-Variable Theories. Phys. Rev. Lett. 23, 880 (1969)

    Article  ADS  Google Scholar 

  22. Clauser, J.F., Horne, M.A.: Experimental consequences of objective local theories. Phys. Rev. D 10, 526 (1974)

    Article  ADS  Google Scholar 

  23. Werner, R.F.: Quantum states with Einstein-Podolsky-Rosen correlations admitting a hidden-variable model. Phys. Rev. A 40, 4277 (1989)

    Article  ADS  Google Scholar 

  24. Gisin, N.: Bell’s inequality holds for all non-product states. Phys. Lett. A 154, 201–202 (1991)

    Article  ADS  MathSciNet  Google Scholar 

  25. Popescu, S.: Bell’s, Inequalities and Density Matrices: Revealing “Hidden” Nonlocality. Phys. Rev. Lett. 74(14), 2619 (1995)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  26. Collins, D., Gisin, N.A.: relevant two qubit Bell inequality inequivalent to the CHSH inequality. J. Phys. A: Math. Theor. 37(5), 1775 (2004)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  27. Liang, Y.C.: Correlations, Bell Inequality Violation & Quantum Entanglement. arXiv:0810.5400v1[quant-ph] (2008)

  28. Methot, A.A., Scarani, V.: An anomaly of non-locality. Quantum Inf. Comput. 7(1), 157–170 (2007)

    MATH  MathSciNet  Google Scholar 

  29. Horst, B., Bartkiewicz, K., Miranowicz, A.: Two-qubit mixed states more entangled than pure states: Comparison of the relative entropy of entanglement for a given nonlocality. Phys. Rev. A 87, 042108 (2013)

    Article  ADS  Google Scholar 

  30. Hall, M.W.: Correlation distance and bounds for mutual information. Entropy 15, 3698–3713 (2013)

    Article  ADS  MathSciNet  Google Scholar 

  31. Chaves, R., Fritz, T.: Entropic approach to local realism and noncontextuality. Phys. Rev. A 032113, 85 (2012)

    Google Scholar 

  32. Chaves, R., Fritz, T.: Entropic Inequalities and Marginal Problems. IEEE Trans. Inf. Theory 59(2), 803–817 (2013)

    Article  MathSciNet  Google Scholar 

  33. Bruno, A., Capolupo, A., Kak, S., Raimondo, G., Vitiello, G.: Gauge theory and two level systems. Mod. Phys. Lett. B 25(20), 1661 (2011)

    Article  ADS  MATH  MathSciNet  Google Scholar 

  34. Peng, Z.H., Zhang, M.J., Zheng, D.N.: Detection of geometric phases in flux qubits with coherent pulses. Phys. Rev. B 73(2), 020502 (2006)

    Article  ADS  Google Scholar 

  35. Mousolou, V.A., Canali, C.M., Sjoqvist, E.: Unifying Geometric Entanglement and Geometric Phase in a Quantum Phase Transition. Phys. Rev. A 88, 012310 (2013)

    Article  ADS  Google Scholar 

  36. Chen, A.M., Cho, S.Y., Su, H.Y., Choi, T.: Relation between Quantum Entanglement and the Berry Phase in Systems of Two Interacting Qubits. J. Korean Phys. Soc. 57(4), 696–703 (2010)

    Article  ADS  Google Scholar 

  37. Kak, S.: The Problem of Testing a Quantum Gate. Infocommunications Journal 4(4), 18–22 (2012)

    Google Scholar 

  38. Licata, I.: Effective Physical Processes and Active Information in Quantum Computing Quantum Biosystems 1, 51 (2007)

  39. Licata, I.: Beyond Turing: Hypercomputation and Quantum Morphogenesis. Asian Pacific Math News 2(3), 20–24 (2012)

    MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. ISEM, Institute for Scientific Methodology, Palermo, Italy

    Davide Fiscaletti

  2. SpaceLife Institute, San Lorenzo in Campo, PU, Italy

    Ignazio Licata

Authors
  1. Davide Fiscaletti
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Ignazio Licata
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Davide Fiscaletti.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Fiscaletti, D., Licata, I. Bell Length in the Entanglement Geometry. Int J Theor Phys 54, 2362–2381 (2015). https://doi.org/10.1007/s10773-014-2461-6

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10773-014-2461-6

Keywords

Search

Navigation