Skip to main content
SpringerLink
Log in

Escape dynamics and fractal basins boundaries in the three-dimensional Earth-Moon system

  • Original Article
  • Published:
Astrophysics and Space Science Aims and scope Submit manuscript

Abstract

The orbital dynamics of a spacecraft, or a comet, or an asteroid in the Earth-Moon system in a scattering region around the Moon using the three dimensional version of the circular restricted three-body problem is numerically investigated. The test particle can move in bounded orbits around the Moon or escape through the openings around the Lagrange points \(L_{1}\) and \(L_{2}\) or even collide with the surface of the Moon. We explore in detail the first four of the five possible Hill’s regions configurations depending on the value of the Jacobi constant which is of course related with the total orbital energy. We conduct a thorough numerical analysis on the phase space mixing by classifying initial conditions of orbits in several two-dimensional types of planes and distinguishing between four types of motion: (i) ordered bounded, (ii) trapped chaotic, (iii) escaping and (iv) collisional. In particular, we locate the different basins and we relate them with the corresponding spatial distributions of the escape and collision times. Our outcomes reveal the high complexity of this planetary system. Furthermore, the numerical analysis suggests a strong dependence of the properties of the considered basins with both the total orbital energy and the initial value of the \(z\) coordinate, with a remarkable presence of fractal basin boundaries along all the regimes. Our results are compared with earlier ones regarding the planar version of the Earth-Moon system.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19

Similar content being viewed by others

Notes

  1. For the value of the mass ratio \(\mu\) we adopted the same precision (number of significant decimal digits) as in Paper I.

  2. In Paper I the authors classified two dimensional orbits with \(\dot{x_{0}} = 0\) and \(\dot {y_{0}} > 0\). In our work we expand into three dimensions this choice of initial conditions thus considering orbits with \(\dot{x_{0}} = \dot{z_{0}} = 0\) and \(\dot{y_{0}} > 0\). The case with non-zero values in \(x\) and \(z\) components of the velocity of the test particle is another choice which however is not considered here, obviously for saving space.

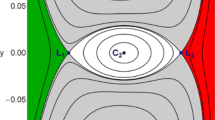
  3. The set of initial conditions of orbits which lead to a certain final state (escape, collision or bounded motion) is defined as a basin.

  4. Obviously, if we numerically integrate initial conditions inside this region we will see that they lead to immediate collision to the Moon.

  5. When we state that an area is fractal we simply mean that it has a fractal-like geometry without conducting any specific calculations as in Aguirre et al. (2009).

References

  • Aguirre, J., Vallego, J.C., Sanjuán, M.A.F.: Wada basins and chaotic invariant sets in the Hénon-Heiles system. Phys. Rev. E 64, 066208 (2001)

    Article  ADS  Google Scholar 

  • Aguirre, J., Viana, R.L., Sanjuán, M.A.F.: Fractal structures in nonlinear dynamics. Rev. Mod. Phys. 81, 333–386 (2009)

    Article  ADS  Google Scholar 

  • Barrio, R., Blesa, F., Serrano, S.: Fractal structures in the Hénon-Heiles Hamiltonian. Europhys. Lett. 82, 10003 (2008)

    Article  Google Scholar 

  • Barrio, R., Blesa, F., Serrano, S.: Bifurcations and safe regions in open Hamiltonians. New J. Phys. 11, 053004 (2009)

    Article  ADS  Google Scholar 

  • Benet, L., Trautman, D., Seligman, T.: Chaotic scattering in the restricted three-body problem. I. The Copenhagen problem. Celest. Mech. Dyn. Astron. 66, 203–228 (1996)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  • Benet, L., Seligman, T., Trautman, D.: Chaotic scattering in the restricted three-body problem II. Small mass parameters. Celest. Mech. Dyn. Astron. 71, 167–189 (1998)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  • Bleher, S., Grebogi, C., Ott, E., Brown, R.: Fractal boundaries for exit in Hamiltonian dynamics. Phys. Rev. A 38, 930–938 (1988)

    Article  ADS  MathSciNet  Google Scholar 

  • Bleher, S., Ott, E., Grebogi, C.: Routes to chaotic scattering. Phys. Rev. Lett. 63, 919–922 (1989)

    Article  ADS  Google Scholar 

  • Contopoulos, G.: Asymptotic curves and escapes in Hamiltonian systems. Astron. Astrophys. 231, 41–55 (1990)

    ADS  MathSciNet  Google Scholar 

  • Contopoulos, G.: Order and Chaos in Dynamical Astronomy. Springer, Berlin (2002)

    Book  MATH  Google Scholar 

  • Contopoulos, G., Kaufmann, D.: Types of escapes in a simple Hamiltonian system. Astron. Astrophys. 253, 379–388 (1992)

    ADS  MathSciNet  MATH  Google Scholar 

  • Contopoulos, G., Kandrup, H.E., Kaufmann, D.: Fractal properties of escape from a two-dimensional potential. Physica D 64, 310–323 (1993)

    Article  ADS  MATH  Google Scholar 

  • de Assis, S.C., Terra, M.O.: Escape dynamics and fractal basin boundaries in the planar Earth-Moon system. Celest. Mech. Dyn. Astron. 120, 105–130 (2014), (Paper I)

    Article  ADS  MathSciNet  Google Scholar 

  • de Moura, A.P.S., Grebogi, C.: Countable and uncountable boundaries in chaotic scattering. Phys. Rev. E 66, 046214 (2002)

    Article  ADS  MathSciNet  Google Scholar 

  • de Moura, A.P.S., Letelier, P.S.: Fractal basins in Hénon-Heiles and other polynomial potentials. Phys. Lett. A 256, 362–368 (1999)

    Article  ADS  MathSciNet  Google Scholar 

  • Ernst, A., Peters, T.: Fractal basins of escape and the formation of spiral arms in a galactic potential with a bar. Mon. Not. R. Astron. Soc. 443, 2579–2589 (2014)

    Article  ADS  Google Scholar 

  • Hénon, M.: Numerical exploration of the restricted problem, V. Astron. Astrophys. 1, 223–238 (1969)

    ADS  MATH  Google Scholar 

  • Jung, C., Tél, T.: Dimension and escape rate of chaotic scattering from classical and semiclassical cross section data. J. Phys. A 24, 2793–2805 (1991)

    Article  ADS  Google Scholar 

  • Jung, C., Mejia-Monasterio, C., Seligman, T.H.: Scattering one step from chaos. Phys. Lett. A 198, 306–314 (1995)

    Article  ADS  Google Scholar 

  • Jung, C., Lipp, C., Seligman, T.H.: The inverse scattering problem for chaotic Hamiltonian systems. Ann. Phys. 275, 151–189 (1999)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  • Kandrup, H.E., Siopis, C., Contopoulos, G., Dvorak, R.: Diffusion and scaling in escapes from two-degrees-of-freedom Hamiltonian systems. Chaos 9, 381–392 (1999)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  • Kennedy, J., Yorke, J.A.: Basins of Wada. Physica D 51, 213–225 (1991)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  • Lai, Y.-C., Tél, T.: Transient Chaos. Springer, New York (2011)

    Book  MATH  Google Scholar 

  • Lyapunov, A.: Probléme General de las Stabilité de Mouvement. Ann. Math. Stud., vol. 17 (1949)

    Google Scholar 

  • Nagler, J.: Crash test for the Copenhagen problem. Phys. Rev. E 69, 066218 (2004)

    Article  ADS  MathSciNet  Google Scholar 

  • Nagler, J.: Crash test for the restricted three-body problem. Phys. Rev. E 71, 026227 (2005)

    Article  ADS  MathSciNet  Google Scholar 

  • Navarro, J.F., Henrard, J.: Spiral windows for escaping stars. Astron. Astrophys. 369, 1112–1121 (2001)

    Article  ADS  Google Scholar 

  • Poon, L., Campos, J., Ott, E., Grebogi, C.: Wada basins boundaries in chaotic scattering. Int. J. Bifurc. Chaos 6, 251–266 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  • Press, H.P., Teukolsky, S.A., Vetterling, W.T., Flannery, B.P.: Numerical Recipes in FORTRAN 77, 2nd edn. Cambridge University Press, Cambridge (1992)

    MATH  Google Scholar 

  • Schneider, J., Tél, T.: Extracting flow structures from tracer data. Ocean Dyn. 53, 64–72 (2003)

    Article  ADS  Google Scholar 

  • Schneider, J., Tél, T., Neufeld, Z.: Dynamics of “leaking” Hamiltonian systems. Phys. Rev. E 66, 066218 (2002)

    Article  ADS  MathSciNet  Google Scholar 

  • Seoane, J.M., Aguirre, J., Sanjuán, M.A.F., Lai, Y.C.: Basin topology in dissipative chaotic scattering. Chaos 16, 023101 (2006)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  • Seoane, J.M., Sanjuán, M.A.F., Lai, Y.C.: Fractal dimension in dissipative chaotic scattering. Phys. Rev. E 76, 016208 (2007)

    Article  ADS  Google Scholar 

  • Simó, C., Stuchi, T.: Central stable/unstable manifolds and the destruction of KAM tori in the planar Hill problem. Physica D 140, 1–32 (2000)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  • Skokos, C.: Alignment indices: a new, simple method for determining the ordered or chaotic nature of orbits. J. Phys. A, Math. Gen. 34, 10029–10043 (2001)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  • Szebehely, V.: Theory of Orbits. Academic Press, New York (1967)

    Google Scholar 

  • Tuval, I., Schneider, J., Piro, O., Tél, T.: Opening up fractal structures of three-dimensional flows via leaking. Europhys. Lett. 65, 633–639 (2004)

    Article  ADS  Google Scholar 

  • Wolfram, S.: The Mathematica Book. Wolfram Media, Champaign (2003)

    MATH  Google Scholar 

  • Zotos, E.E.: A Hamiltonian system of three degrees of freedom with eight channels of escape: the great escape. Nonlinear Dyn. 76, 1301–1326 (2014a)

    Article  MathSciNet  Google Scholar 

  • Zotos, E.E.: Escapes in Hamiltonian systems with multiple exit channels: Part I. Nonlinear Dyn. 78, 1389–1420 (2014b)

    Article  MathSciNet  Google Scholar 

  • Zotos, E.E.: Revealing the escape mechanism of three-dimensional orbits in a tidally limited star cluster. Mon. Not. R. Astron. Soc. 446, 770–792 (2015a)

    Article  ADS  Google Scholar 

  • Zotos, E.E.: Escapes in Hamiltonian systems with multiple exit channels: Part II. Nonlinear Dyn. 82, 357–398 (2015b)

    Article  MathSciNet  Google Scholar 

  • Zotos, E.E.: Orbital dynamics in the planar Saturn-Titan system. Astrophys. Space Sci. 358, 4 (2015c)

    Article  ADS  Google Scholar 

  • Zotos, E.E.: Orbit classification in the planar circular Pluto-Charon system. Astrophys. Space Sci. 360, 7 (2015d)

    Article  ADS  Google Scholar 

  • Zotos, E.E., Caranicolas, N.D.: Order and chaos in a new 3D dynamical model describing motion in non-axially symmetric galaxies. Nonlinear Dyn. 74, 1203–1221 (2013)

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgements

I would like to express my warmest thanks to the anonymous referee for the careful reading of the manuscript and for all the apt suggestions and comments which allowed us to improve both the quality and the clarity of the paper.

Author information

Authors and Affiliations

  1. Department of Physics, School of Science, Aristotle University of Thessaloniki, 541 24, Thessaloniki, Greece

    Euaggelos E. Zotos

Authors
  1. Euaggelos E. Zotos
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Euaggelos E. Zotos.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zotos, E.E. Escape dynamics and fractal basins boundaries in the three-dimensional Earth-Moon system. Astrophys Space Sci 361, 94 (2016). https://doi.org/10.1007/s10509-016-2683-6

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10509-016-2683-6

Keywords

Search

Navigation