Influence of nucleus deformability on cell entry into cylindrical structures

Abstract

The mechanical properties of cell nuclei have been demonstrated to play a fundamental role in cell movement across extracellular networks and micro-channels. In this work, we focus on a mathematical description of a cell entering a cylindrical channel composed of extracellular matrix. An energetic approach is derived in order to obtain a necessary condition for which cells enter cylindrical structures. The nucleus of the cell is treated either (i) as an elastic membrane surrounding a liquid droplet or (ii) as an incompressible elastic material with Neo-Hookean constitutive equation. The results obtained highlight the importance of the interplay between mechanical deformability of the nucleus and the capability of the cell to establish adhesive bonds and generate active forces in the cytoskeleton due to myosin action.

Appendices

Appendix 1: Micropipette models applied to cell migration inside channels

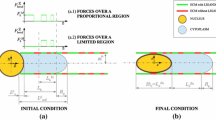
For sake of completeness, we consider here the case in which nucleus entry obeys the classical relations (2.2) or (2.5), deforming the initial spherical nucleus into a cigar-like shape, with the assumption that $L_p$ in (2.2) and (2.5) represents the length of the deformed nucleus, i.e., $L_p=L_{n,\mathrm{cigar}}^{\mathrm{fin}}=2(h+R_p)$ with $h$ given by Eq. (4.3). We define the critical pressure as the value of $\Delta P$ for which $L_p=L_{n,\mathrm{cigar}}^{\mathrm{fin}}$ and we assume that a proper representation for $\Delta P$ in Eqs. (2.2) and (2.5) is $\dfrac{F_{\mathrm{active}}^Z}{\pi R_p^2}$, where $F_{\mathrm{active}}^Z$ is the $Z$-component of the active force given either by Eq. (3.8) or (3.9) or (3.10). Then, assuming that a pressure above the critical one makes the cell move inside the pipette, it is possible to obtain the relation between mechanical and active properties that should hold for the cell to enter the channel, depending on the geometrical properties (i.e., $R_{n},R_{c}$ and $R_{p}$).

The inequalities that should be satisfied in each case are summarized in Table 2, as a function of the diameter ratio $\tilde{R}_p=\dfrac{R_p}{R_n}$. On the left-hand side of each relation, we have characteristic parameters representing the ratio between active properties and mechanical properties of cell nucleus. In particular, we identify

$$\begin{aligned}&G_{\gamma }^{k}= \dfrac{\rho _b \alpha _{\mathrm{ECM}} k_b R_n^2}{\gamma }, \quad G_{\gamma }^{F}= \dfrac{\rho _b \alpha _{\mathrm{ECM}} F_{b}^{M} R_n}{\gamma },\\&G_{E}^{k}= \dfrac{\rho _b \alpha _{\mathrm{ECM}} k_b R_n}{E}, \quad G_{E}^{F}=\dfrac{\rho _b \alpha _{\mathrm{ECM}} F_{b}^{M}}{E} . \end{aligned}$$

Table 2 Entry criteria

On the right-hand side of each relation, we have the critical value of the characteristic number (indicated with $\overline{G}_{i}^{j}$, with $i=\left\{ \gamma , E \right\} ,j= \left\{ k,F \right\}$), which is a function of the diameter ratio, and we have set

$$\begin{aligned}&\tilde{L}_p= \dfrac{L_p}{R_n}=\dfrac{2}{3} \tilde{R}_p \left[ 1+ 2 \left( \dfrac{1}{\tilde{R}_p}\right) ^3 \right] ,\\&\tilde{L}_b= \dfrac{L_b}{R_n}=\tilde{R}_p \left[ \dfrac{4}{3} \left( \dfrac{\tilde{R}_c}{\tilde{R}_p}\right) ^3 - \dfrac{1}{\tilde{R}_p^3} +\dfrac{1}{3}+ \right. \\&\quad \quad \quad \left. + \dfrac{\left( \tilde{L}_n^0\right) ^2}{\tilde{R}_p^3} \left( 1- \dfrac{1}{3} \tilde{L}_n^0 \right) \right] \end{aligned}$$

with $\tilde{L}_n^0=1-\sqrt{1-\tilde{R}_p^2}$ and $\tilde{R}_c=R_c/R_n$.

The critical characteristic numbers are plotted in Fig. 9 as a function of the diameter ratio of the channel. The graphs represent the minimum value that each constant should assume in order to have the cell totally inside the channel, according to Chien’s criterion (Fig. 9a) and Theret’s one (Fig. 9b). Results obtained with the linearized Chien’s equation (2.4) are comparable with the ones obtained with the more complex formula (2.2). In Fig. 9, the dashed line represents results obtained using constant forces over a bounded domain (where we set $\tilde{L}_b^M=5$). It is possible to see that for big $\tilde{R}_p,\overline{G}_{\gamma }^F$ and $\overline{G}_{E}^F$ are obviously not influenced by the assumption on the boundedness of the contact region in which integrins are expressed (i.e., the red-dashed curve and the black-solid one overlap). Indeed, it exists an $\tilde{R}_p^*$ such that $L_b^*=L_b$ for $\tilde{R}_p \ge \tilde{R}_p^*$, whereas $L_b^*=L_b^M$ for $\tilde{R}_p < \tilde{R}_p^*$. Therefore, the active work is influenced by the boundedness assumption only for $\tilde{R}_p < \tilde{R}_p^*$.

Fig. 9

Critical value of the characteristic numbers obtained applying a Chien’s model and b Theret’s model, under either a linear force (blue) or a constant force (black) or a constant force over a bounded region (red dashed) assumption

For instance, Fig. 10 explains how these graphs can be interpreted (for the particular case of Chien model): The bar charts below the graph represent the range of $\tilde{R}_p$ for which a cell characterized by either a given $G_{\gamma }^{k}$ or a given $G_{\gamma }^{F}$ can enter the channel.

Fig. 10

Interpretation of the results: bar charts represents the range for which a cell, with a given $G_{\gamma }^{k}$ or $G_{\gamma }^{F}$ can enter the channel, for the different hypotheses of bond forces

In the figure, ‘cell 1’ (orange) is characterized by higher $G_{\gamma }^{k}$ or $G_{\gamma }^{F}$ than ‘cell 2’ (violet). This means that we are considering either a softer cell (i.e., smaller $\gamma$) or a cell that is able to establish a higher number of adhesive bonds (i.e., higher $\rho _b \alpha _{\mathrm{ECM}}$) or a cell with better contractile capabilities (i.e., bigger $k_b$ or $F_b^M$). In any case, the range for which ‘cell 1’ can enter the pipette is bigger than for ‘cell 2’ (orange bars vs. violet bars), according to what we expect from biological observations. Moreover, using the constant force assumption, it is possible to see that the range for which cells can enter the pipette is bounded both from below and from above. On the other hand, using the linear force assumption, we do not have any inferior limit, in contrast with biological observation. This contradictory result is due to the hypothesis used in the representation of forces. Indeed, in this case, the more the cytoplasm of the cell spreads inside the channel (small $\tilde{R}_p$), the more the traction force (which is related to the adhesive region) can pull the nucleus inside. In particular, even though the force required to deform the nucleus grows as $\tilde{R}_p^{-3}$, as $\tilde{R}_p \rightarrow 0$, the adhesive-dependent active force raises faster, since $\tilde{L}_b^2= \mathcal O \left( \tilde{R}_p^{-4}\right)$. On the other hand, when a constant force assumption is used, for small $\tilde{R}_p$, the length for which bonds are formed augments $\left( \tilde{L}_b= \mathcal O \left( \tilde{R}_p^{-2}\right) \, \text{ for } \, \tilde{R}_p \rightarrow 0 \right)$. Thus, the total integrin-dependent traction force exerted on the nucleus increases, but it is not sufficient to compensate the greater deformation required by the nucleus, which goes like $\tilde{R}_p^{-3} \, \text{ for } \, \tilde{R}_p \rightarrow 0$. Conversely, introducing the boundedness assumption on $L_b$, the active force is limited.

In particular, we have that for $\tilde{R}_p \rightarrow 0, \overline{G}^{\gamma }_{F}$ goes like $\tilde{R}_p^{-\alpha }$ (with $\alpha =1$ for unbounded $L_b$ and $\alpha =3$ when the adhesive region is limited) and $\overline{G}^{\gamma }_{k}$ grows linearly.

On the other hand, when the radius of the pipette is very big, the entry of the cell into the channel is limited due to the decrease in the contact area between the cell and the channel wall, where adhesive bonds are formed. It is likely that, in this case, the force exerted by actomyosin is not equal to the maximum executable force. Thus, a linear force can better describe the physiological behaviour. Therefore, a good choice for the bond force relation could be a ramp force on a bounded adhesive region, which is also the most conservative case.

In Theret’s model, it is possible to see that, for $\tilde{R}_p \rightarrow 0, \overline{G}_{E}^{k}= \mathcal O \left( \tilde{R}_p^2\right)$ and $\overline{G}_{E}^{F}= \mathcal O \left( 1 \right)$ when the constant force assumption with unbounded adhesive region is implemented. Thus, neither the constant force assumption nor the linear force one can account for the inferior limit in pipette calibres. Only enforcing the boundedness of the adhesive region, the capability of cells to enter very small channels is prevented.

Both Chien’s and Theret’s models, with the assumption of active forces over a bounded region, provide evidence for a biphasic cell migratory behaviour that reveals most optimal migration at pore sizes at nuclear and subnuclear diameters and diminishes at gaps greatly bigger or smaller than the cell nucleus diameter.

However, even though the results obtained by applying the classical models above seem promising, especially when adhesion is active on a bounded domain, they cannot account for the finite boundaries of the nucleus. Indeed, Chien’s model refers to an infinite 2D membrane, whereas Theret’s one was derived for a 3D half-space aspired inside a pipette, only for a small portion. Therefore, these criteria cannot be applied to describe the total entry of the cell into a pipette. The consequence of this assumption is evident in Fig. 9, where, for $\tilde{R}_p=1$, the force needed to deform the nucleus does not vanish.

Appendix 2: Influence of bending

In Sect. 4.2, we considered only the contribution to the surface deformation energy due to the stretching of the nuclear membrane, but we disregarded the energy contribution associated with bending. In order to introduce in the model the bending of the nuclear membrane, we refer to Helfrich’s work on lipid bilayers (Helfrich 1973). Helfrich introduced a model (Helfrich 1973) in which the bending energy of a membrane is given by

$$\begin{aligned}&\mathcal W _{\mathrm{bending}}=\dfrac{k_c}{2} \int \limits _S (2 H - c_0)^2 \hbox {d}S + \dfrac{k_g}{2} \int \limits _S K \hbox {d}S, \end{aligned}$$

(6.1)

where $H=\dfrac{1}{2} (\textit{k}_1+ \textit{k}_2)$ is the mean curvature of the membrane surface, $S,\textit{k}_1$ and $\textit{k}_2$ are the principal curvatures, $K= \textit{k}_1 \textit{k}_2$ is the Gaussian curvature, and $c_0$ represents the spontaneous curvature that describes the asymmetric effect of the membrane. We remark that, from the Gauss-Bonnet theorem, the second term in the Helfrich energy is a topological invariant, and thus, in this work, it can be omitted (Laadhari et al. 2010). The total energy of deformation of the nuclear membrane, considering both the contribution due to stretching and the one due to bending, is Landau and Lifschitz (1986)

$$\begin{aligned}&\mathcal W _{\mathrm{tot}}^S= \lambda (\Delta S)^2+ \mathcal W _{\mathrm{bending}}^{\mathrm{deformed}}- \mathcal W _{\mathrm{bending}}^{\mathrm{sphere}}, \end{aligned}$$

(6.2)

where $\mathcal W _{\mathrm{bending}}^{\mathrm{sphere}}= 8 \pi k_c$. For the cigar-shaped deformed configuration, we define

$$\begin{aligned}&\mathcal W _{\mathrm{bending}}^{\mathrm{deformed}}=\mathcal W _{\mathrm{bending}}^{\mathrm{cigar}}= 2 \pi k_c \dfrac{h}{R_p} + 8 \pi k_c, \end{aligned}$$

(6.3)

whereas, for a nucleus deformed into an ellipsoid, we have

$$\begin{aligned} \mathcal W _{\mathrm{bending}}^{\mathrm{deformed}}=\mathcal W _{\mathrm{bending}}^{\mathrm{ellips}}&=\dfrac{k_c}{2} \int \limits _{S_{\mathrm{ellips}}} (2 H )^2 \hbox {d}S=\nonumber \\&= k_c \pi \int \limits _0^{\pi } (2 H )^2 \mathcal A \hbox {d} \Theta , \end{aligned}$$

(6.4)

where we referred to Poelaert et al. (2011), in which the principal curvatures of the general ellipsoid are derived, i.e.,

$$\begin{aligned}&2 H= \dfrac{F^3 (h_e^2 + 2 R_p^2 - R^2)}{h_e^2 R_p^4}, \end{aligned}$$

(6.5)

$$\begin{aligned}&F = h_e R_p \dfrac{\sqrt{R_p^2 \cos ^2 \Theta + h_e^2 \sin ^2 \Theta }}{\sqrt{R_p^4 \cos ^2 \Theta + h_e^4 \sin ^2 \Theta }}, \end{aligned}$$

(6.6)

$$\begin{aligned}&R=\dfrac{h_e R_p}{\sqrt{R_p^2 \cos ^2 \Theta + h_e^2 \sin ^2 \Theta }}, \end{aligned}$$

(6.7)

$$\begin{aligned}&\mathcal A = h_e^2 R_p^2 \dfrac{ \sqrt{R_p^4 \cos ^2 \Theta + h_e^4 \sin ^2 \Theta }}{R_p^2 \cos ^2 \Theta + h_e^2 \sin ^2 \Theta } \sin \Theta \end{aligned}$$

(6.8)

and $h_e=R_n \dfrac{R_n^2}{R_p^2}$ is defined in (4.2). The integral (6.4) is solved numerically.

Once $\mathcal W _{\mathrm{tot}}^S$ is known, it is possible to obtain an expression for the dimensionless parameters $G_{\lambda }^{k}$ and $G_{\lambda }^{F}$. The results are reported in Table 3, where $\tilde{k}_c= \dfrac{k_c}{\lambda R_n^4}, Q(R_p)= \int _0^{\pi } (2 H )^2 \mathcal A \hbox {d} \Theta , G_{\lambda }^{k}$ and $G_{\lambda }^{F}$ have the same definition given in Sect. 5.

Table 3 Energy-based criteria: stretching and bending

The results are reported in Fig. 11 for the cigar-shaped nucleus and in Fig. 12 for the ellipsoidal nucleus. In order to observe the bending contribution, the ratio $k_c/\lambda$ should be larger than $10\mu \hbox {m}^4$. However, from Helfrich (1973), we, know that for lipid bilayer membrane of a nucleus of radius $4\mu$m, we have $k_c/\lambda = 4 \cdot 10^{-5} \mu \hbox {m}^4$. Thus, under the assumption that the surface of the nucleus increases, the major contribution is due to stretching.

Fig. 11

Critical value of $G_{\lambda }^k$ and $G_{\lambda }^F$ for different values of $k_c$, assuming a cigar-shaped deformation

Fig. 12

Critical value of $G_{\lambda }^k$ and $G_{\lambda }^F$ for different values of $k_c$, assuming an ellipsoidal deformation