Abstract
Our purpose is to study the rigidity of complete hypersurfaces immersed into a Riemannian space form. In this setting, first we use a classical characterization of the Euclidean sphere \(\mathbb S ^{n+1}\) due to Obata (J Math Soc Jpn 14:333–340, 1962) in order to prove that a closed orientable hypersurface \(\Sigma ^n\) immersed with null second-order mean curvature in \(\mathbb S ^{n+1}\) must be isometric to a totally geodesic sphere \(\mathbb S ^{n}\), provided that its Gauss mapping is contained in a closed hemisphere. Furthermore, as suitable applications of a maximum principle at the infinity for complete noncompact Riemannian manifolds due to Yau (Indiana Univ Math J 25:659–670, 1976), we establish new characterizations of totally geodesic hypersurfaces in the Euclidean and hyperbolic spaces. We also obtain a lower estimate of the index of minimum relative nullity concerning complete noncompact hypersurfaces immersed in such ambient spaces.
Similar content being viewed by others
Avoid common mistakes on your manuscript.
1 Introduction
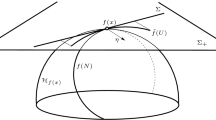
The study of the behavior of the Gauss mapping plays an important role in order to obtain rigidity results concerning complete hypersurfaces immersed into a space form; for instance, it was proved, independently, by De Giorgi in [13] and Simons in [20] that if the image of the Gauss mapping of a compact minimal hypersurface \(M^n\) in the Euclidean sphere \(\mathbb S ^{n+1}\) lies in an open hemisphere, then \(M^n\) must be a great hypersphere of \(\mathbb S ^{n+1}\). A few years late, Nomizu and Smyth [16] have shown a similar result for a closed orientable hypersurface \(x:M^{n}\looparrowright \mathbb S ^{n+1}\) with constant mean curvature. We observe that such results are natural extensions of the classical Bernstein’s theorem established in [6] for a complete minimal surface \(M^2\) into the \(3\)-dimensional Euclidean space \(\mathbb R ^3\). The same question was treated by Alencar et al. [3] for the case of null high-order mean curvature \(H_r\), with the additional hypothesis that \(H_{r-1}\) does not change sign.
On the other hand, on the middle of the last century, many geometers tried to prove that a compact Riemannian manifold with constant scalar curvature is isometric to a standard sphere provided that it carries a nontrivial conformal vector field. But this result is not true according to a counterexample exhibited by Ejiri [11]. Meanwhile, some characterizations of the Euclidean sphere were obtained related to this problem. Among them, we point out a classical one due to Obata [17], which proves that a complete Riemannian manifold \((M^n,g)\) is isometric to a standard sphere \(\mathbb S ^n(r),\) provided that there exists a nontrivial solution for the PDE \(\nabla ^2 \rho =-\rho g,\) where \(\nabla ^2\rho \) stands for the Hessian of \(\rho \).
In this paper, by using such Obata’s theorem jointly with some suitable formulas related to the height and support functions of a hypersurface, we extend the above-mentioned results of De Giorgi and Simons for the context of compact orientable hypersurfaces \(x:M^{n}\looparrowright \mathbb S ^{n+1}\) with null second-order mean curvature. More precisely, we obtain the following theorem:
Theorem 1
Let \(x:\Sigma ^n\looparrowright \mathbb S ^{n+1}\) be a closed orientable hypersurface such that \(H_2=0\). If the image of the Gauss mapping of \(\Sigma ^n\) is contained into a closed hemisphere of the Euclidean sphere \(\mathbb S ^{n+1}\), then \(\Sigma ^n\) is a totally geodesic sphere of \(\mathbb S ^{n+1}\).
Afterward, we deal with hypersurfaces of the hyperbolic space \(\mathbb H ^{n+1}\). First, we recall the following extension of Hopf’s theorem on a complete noncompact Riemannian manifold \(\Sigma ^n\) due to Yau [21]: a subharmonic (or superharmonic) function whose gradient has integrable norm on \(\Sigma ^n\) must actually be harmonic. More recently, Camargo et al. [9] extended Yau’s result concerning a complete noncompact oriented hypersurface \(\Sigma ^n\) immersed in a space form, with bounded second fundamental form. In this setting, they showed that if a smooth function \(f\) defined on \(\Sigma ^n\) is such that \(\nabla f\) has integrable norm and \(L_rf\) does not change sign on \(\Sigma ^n\), then \(L_rf=0\) on \(\Sigma ^n\) (for the details about the \(L_r\) operators, see Sect. 2).
Here, we use such analytical machinery in order to obtain a rigidity theorem for hypersurfaces immersed in the hyperbolic space \(\mathbb H ^{n+1}\). For this, we consider the Lorentz model of \(\mathbb H ^{n+1}\) obtained by furnishing the hyperquadric \(\{p\in \mathbb L ^{n+2};\langle p,p\rangle =-1,\,\,p_{n+2}>0\}\) with the Riemannian metric induced by the Lorentz metric of the Minkowski space \(\mathbb L ^{n+2}\). Moreover, we denote by \(a^\top \) the tangential component of a vector \(a\in \mathbb L ^{n+2}\) with respect to an immersion \(x:\Sigma ^n\looparrowright \mathbb H ^{n+1}\hookrightarrow \mathbb L ^{n+2}\), and along this paper, \(\mathcal L ^1(\Sigma )\) stands for the space of Lebesgue integrable functions on \(\Sigma ^n\). In this setting, we get the following result:
Theorem 2
Let \(x:\Sigma ^n\looparrowright \mathbb H ^{n+1}\) be a complete hypersurface immersed in \(\mathbb H ^{n+1}\) with bounded nonnegative mean curvature \(H\) and such that \(H_2=0\). Suppose that \(\Sigma ^n\) lies between two hyperspheres of \(\mathbb H ^{n+1}\) determined by a spacelike vector \(a\in \mathbb L ^{n+2}\). If \(|a^\top |\in \mathcal L ^1(\Sigma )\), then \(\Sigma ^n\) is a totally geodesic hypersphere of \(\mathbb H ^{n+1}\).
The proofs of Theorems 1 and 2 are presented in Sect. 3. Furthermore, in Sect. 4, we establish rigidity results in the Euclidean space (cf. Theorems 3 and 4; see also Corollaries 1 and 2) and, in Sect. 5, we obtain a lower estimate to the index of minimum relative nullity concerning complete noncompact hypersurfaces \(\Sigma ^n\) immersed either in \(\mathbb R ^{n+1}\) or in \(\mathbb H ^{n+1}\) (cf. Theorems 5 and 6).
2 Preliminaries
In this section, we present some known results that we use in order to prove our theorems. Throughout this paper, we denote by \(Q_{c}^{n+1}\) a Riemannian space form of constant sectional curvature \(c\in \{-1,0,1\}\), and \(x:\Sigma ^n\looparrowright {Q}_{c}^{n+1}\) stands for an immersed hypersurface in \({Q}_{c}^{n+1}\).
If we let A denote the corresponding shape operator, then, at each \(p\in \Sigma ^n, A\) restricts to a self-adjoint linear map \(A_p:T_p\Sigma \rightarrow T_p\Sigma \). For \(0\le r\le n\), let \(S_r(p)\) denotes the \(r\)th elementary symmetric function on the eigenvalues of \(A_p\); in this way, one gets n smooth functions \(S_r:\Sigma ^n\rightarrow \mathbb R \), such that
where \(S_0=1\) by convention. If \(p\in \Sigma ^n\) and \(\{e_k\}\) are a basis of \(T_p\Sigma \) formed by eigenvectors of \(A_p\), with corresponding eigenvalues \(\{\lambda _k\}\), one immediately sees that
where \(\sigma _r\in \mathbb R [X_1,\ldots ,X_n]\) is the \(r\)th elementary symmetric polynomial on the indeterminates \(X_1,\ldots ,X_n\).
Also, we define the \(r\)th mean curvature \(H_r\) of \(\Sigma ^n, 0\le r\le n\), by
We observe that \(H_0=1\), while \(H_1\) is the usual mean curvature \(H\) of \(\Sigma ^n\).
For \(0\le r\le n\), one defines the \(r\)th Newton transformation \(P_r\) on \(\Sigma ^n\) by setting \(P_0=I\) (the identity operator) and, for \(1\le r\le n\), via the recurrence relation
On the other hand, given \(f\in C^{\infty }(\Sigma )\), for each \(0\le r\le n\), the second-order differential operator \(L_r\) is defined as follows
It is important to note that this operator is divergence type provided that we have a hypersurface \(\Sigma ^n\looparrowright Q_{c}^{n+1}\), where \(Q_{c}^{n+1}\) is a space form. This fact was proved by Rosenberg [18] and it reads as follows
Moreover, for a smooth function \(\varphi :\mathbb R \rightarrow \mathbb R \) and \(f\in C^{\infty }(\Sigma )\), it follows from the properties of the Hessian that
When we are dealing with the Euclidean space \(\mathbb R ^{n+1}\) or the Euclidean sphere \(\mathbb S ^{n+1}\), we may fix a Euclidean vector a; whereas for the hyperbolic space \(\mathbb H ^{n+1}\), we may fix a vector a of the Minkowski space \(\mathbb L ^{n+2}\). We recall that the height function \(l_a\) and the support function \(f_a\) of an immersion \(x:\Sigma ^n\looparrowright {Q}_{c}^{n+1}\) are defined, respectively, according to
where N stands for the Gauss mapping of x. In this setting, we have the following split
where \(a^\top \) is the orthogonal projection of a over the tangent bundle \(T\Sigma \).
Moreover, based on the paper due to Reilly [19], Rosenberg [18] showed the following identities related with the action of \(L_r\) on these functions:
and
In particular, letting \(r=0\), we deduce the following well-known relations:
and
To close this section, we point out the next inequality that can be found in [2] or [7], which is valid for an immersion \(x:\Sigma ^n\looparrowright {Q}_{c}^{n+1}\) with \(S_2\) constant:
In particular, if \(S_2\ge 0\), then \(\vert \nabla A\vert ^2-\vert \nabla S_1\vert ^2\ge 0\).
3 Proofs of Theorems 1 and 2
Let us begin with the proof of Theorem 1.
Proof
First, one notices the existence of an unit vector \(a\in \mathbb R ^{n+2}\) such that the associated support function \(f_a=\langle N,a\rangle \) does not change sign, where \(N\) is an unit normal vector field globally defined on \(\Sigma ^n\). On the other hand, since \(S_2=0\), we use Gauss equation
to conclude that the scalar curvature of \(\Sigma ^n\) is identically one. Now, we use formula (2.5) to infer
If \(S_1=0\), we have \(|A|\equiv 0\), which gives the desired result. Otherwise, we deduce from previous equation that
On the other hand, using Proposition 3.1 of [7], we get
If \(f_a\) is identically null, we can apply Theorem 1 of [16], due to Nomizu and Smyth, to deduce that \(\Sigma ^n\) is totally geodesic. Otherwise, we can multiply the last identity by \(f_a\) to obtain
Now, we use the symmetry of \(L_1\) to infer
Therefore, we deduce
From where we conclude
By using (2.8), we have
and
On the other hand, since \(H_2=0\), we have that \(\vert A\vert ^2=n^2H^2\). Consequently, from (3.3), we get
At this point, we recall the next relation involving \(l_a\) and \(f_a\), which can be found in Alías [4]:
where g stands for the Riemannian metric of \(\Sigma ^n\).
Hence, combining (3.4) and (3.5), one obtains the following:
Now, one observes that if \(l_a\) is constant, then \(\Sigma ^n\) is totally umbilical. But, \(S_2=0\) gives that it must be, in fact, totally geodesic. Otherwise, we may apply Obata’s theorem [17] in order to conclude that \(\Sigma ^n\) is isometric to a standard sphere. Once more, \(S_2=0\) gives that \(\Sigma ^n\) is totally geodesic, which completes the proof of Theorem 1. \(\square \)
Before to start the proof of Theorem 2, we recall that hyperspheres of the hyperbolic space \(\mathbb H ^{n+1}\) can be realized in Minkowski’s model as the following level sets:
for some spacelike vector \(a\in \mathbb L ^{n+2}\), where \(\tau ^2>\langle a,a\rangle \) (see e.g. [5], Sect. 3).
Proof
Initially, we observe that, since we are supposing that \(\Sigma ^n\) lies between two hyperspheres \(L_{\underline{\tau }}\) and \(L_{\overline{\tau }}\) of \(\mathbb H ^{n+1}\) determined by a spacelike vector \(a\in \mathbb L ^{n+2}\), the height function \(l_a=\langle x,a\rangle \) is bounded on \(\Sigma ^n\). Moreover, the hypothesis under the second-order mean curvature of \(\Sigma ^n\) assures us that \(S_2=0\) on \(\Sigma ^n\). Consequently, since \(H\ge 0\) on \(\Sigma ^n\), from Lemma 1.1 and equation (1.3) in [14] we have that \(P_1\) is a positive semi-definite operator. Thus, using (2.2) and (2.4), we deduce
On the other hand, we note that the second fundamental form A of \(\Sigma ^n\) is bounded and
Thus, we are in position to apply Corollary 1 of [9], to get that \(L_{1}l_a^2=0\) on \(\Sigma ^n\). Consequently, from equation (3.7), \(S_1l_a=0\) on \(\Sigma ^n\). Now, from (2.6), we obtain
Reasoning as before, we see that \(l_a\) vanishes identically on \(\Sigma ^n\), and therefore, this allows us to conclude that \(\Sigma ^n\) is a totally geodesic hypersphere of \(\mathbb H ^{n+1}\). \(\square \)
4 Rigidity theorems in the Euclidean space
Initially, we observe that a paraboloid has positive Gaussian curvature, it is contained in a semispace, and its Gauss mapping covers only an open hemisphere of \(\mathbb S ^2\) determined by such semispace. We also observe that a cylinder over a plane curve inside of a slice, has null Gaussian curvature, lies between two parallel planes orthogonal to a fixed vector \(a\in \mathbb R ^{3}\), but its Gauss mapping is not contained in a closed hemisphere determined by a. However, by applying a classical result due to Huber [15] concerning parabolic surfaces, we obtain the following rigidity result in the three-dimensional Euclidean space \(\mathbb R ^3\) related to complete surfaces with nonnegative Gaussian curvature:
Theorem 3
Let \(x:\Sigma ^2\looparrowright \mathbb R ^3\) be a complete surface with nonnegative Gaussian curvature \(K_{\Sigma }\) and such that its mean curvature H does not change sign. Suppose that \(\Sigma ^2\) lies between two parallel planes of \(\mathbb R ^3\) which are orthogonal to an unit vector \(a\in \mathbb R ^3\) and that the image of its Gauss mapping lies in a closed hemisphere of \(\mathbb S ^2\) determined by a. Then, \(\Sigma ^2\) is a plane of \(\mathbb R ^3\) orthogonal to a.
Proof
Since we are supposing that the mean curvature \(H\) does not change sign, we can choose an orientation for \(\Sigma ^2\) in such away that \(H\ge 0\). We note that the hypothesis under the Gauss mapping of \(\Sigma ^2\) also guarantees that \(f_a\) does not change sign. On the other hand, since \(\Sigma ^2\) lies between two parallel planes determined by \(a\), there exists constants \(\alpha \) and \(\beta \) such that \(\alpha \le l_a\le \beta \). Thus, if \(f_a\le 0\), we have that \(l_a-\alpha \) is a nonnegative function on \(\Sigma ^2\) and, from (2.6), we obtain
However, the quoted result of Huber [15] assures that complete surfaces of nonnegative Gaussian curvature must be parabolic. Therefore, \(l_a\) is constant on \(\Sigma ^2\), and hence, \(\Sigma ^2\) is a plane orthogonal to a.
Now, if \(f_a\ge 0\), we see that \(\beta -l_a\) is a nonnegative function on \(\Sigma ^2\), and taking into account once more formula (2.6), we get
Therefore, reasoning as before, we also conclude that \(\Sigma ^2\) is a plane orthogonal to a. \(\square \)
Corollary 1
Let \(\Sigma ^2(u)=\{(x,y,u(x,y)):(x,y)\in \mathbb R ^2\}\subset \mathbb R ^3\) be a complete graph of a smooth function \(u:\mathbb R ^2 \rightarrow \mathbb R \) with nonnegative Gaussian curvature and such that its mean curvature does not change sign. If u is bounded, then \(\Sigma ^2(u)\) is a plane of \(\mathbb R ^3\).
Proof
Since the support function \(f_{e_3}\) has strict sign on \(\Sigma ^2(u)\), the image of the Gauss mapping of \(\Sigma ^2(u)\) lies in an open hemisphere of \(\mathbb S ^2\) determined by \(e_3\). Therefore, the result follows from Theorem 3. \(\square \)
Now, we observe that the examples presented in the beginning of this section also satisfy the same conditions of the next theorem, up to \(|a^\top |\in \mathcal L ^1(\Sigma )\). But, using Yau’s result [21] mentioned in the introduction, we prove the following rigidity result in the \((n+1)\)-dimensional Euclidean space:
Theorem 4
Let \(x:\Sigma ^n\looparrowright \mathbb R ^{n+1}\) be a complete hypersurface such that its mean curvature H does not change sign. Suppose that there exists an unit vector \(a\in \mathbb R ^{n+1}\) such that one of the following conditions is satisfied:
-
(a)
\(\Sigma ^n\) has scalar curvature R bounded from below and the image of its Gauss mapping lies in an open hemisphere of \(\mathbb S ^n\) determined by a;
-
(b)
\(\Sigma ^n\) lies between two hyperplanes which are orthogonal to a and the image of its Gauss mapping lies in a closed hemisphere of \(\mathbb S ^n\) determined by a.
If \(|a^\top |\in \mathcal L ^1(\Sigma )\), then \(\Sigma ^n\) is a hyperplane of \(\mathbb R ^{n+1}\) orthogonal to a.
Proof
First, let us suppose that \(\Sigma ^n\) has scalar curvature R bounded from below and that the image of the Gauss mapping of \(\Sigma ^n\) lies in an open hemisphere of \(\mathbb S ^n\) determined by a. In this case, the support function \(f_a\) has strict sign on \(\Sigma ^n\). Moreover, from (2.6), we deduce
Consequently, \(\Delta l_a\) does not change sign on \(\Sigma ^n\).
On the other hand, taking into account that \(|\nabla l_a|=|a^\top |\in \mathcal L ^1(\Sigma )\), we may apply the result due to Yau [21], which was described in the introduction, to deduce that \(l_a\) is harmonic. Hence, H vanishes identically on \(\Sigma ^n\). In particular, we have \(|A|^2=-n(n-1)R\) and consequently, \(|A|\) is bounded on \(\Sigma ^n\). This allows us to conclude that \(|\nabla f_a|\in \mathcal L ^1(\Sigma ).\) Indeed,
Furthermore, from (2.7), we get
Then, \(\Delta f_a\) also does not change sign on \(\Sigma ^n\). Thus, by applying again Yau’s result, we conclude that \(f_a\) is harmonic. Hence, A vanishes identically on \(\Sigma ^n\), that is, \(\Sigma ^n\) is totally geodesic. Therefore, \(\Sigma ^n\) is a hyperplane of \(\mathbb R ^{n+1}\) orthogonal to a; otherwise \(|a^\top |\) does not belong to \(\mathcal L ^1(\Sigma )\), which finishes the proof of the first assertion.
Now, let us suppose that \(\Sigma ^n\) lies between two hyperplanes which are orthogonal to a and that the image of the Gauss mapping of \(\Sigma ^n\) lies in a closed hemisphere of \(\mathbb S ^n\) determined by a. In such situation, analogously to the previous one, we also conclude that \(l_a\) is harmonic. From where, since
we verify that \(\Delta l_a^2\ge 0\) on \(\Sigma ^n\).
On the other hand, since \(\Sigma ^n\) lies between two hyperplanes which are orthogonal to a, we have that \(|l_a|\le C_1\). This gives
Hence, we deduce that \(l_a^2\) is also harmonic and consequently, \(\nabla l_a\) vanishes identically on \(\Sigma ^n\). Therefore, \(\Sigma ^n\) is a hyperplane of \(\mathbb R ^{n+1}\) orthogonal to a and we complete the proof of Theorem 4. \(\square \)
Corollary 2
Let \(\Sigma ^n(u)=\{(x,u(x)):x\in \mathbb R ^n\}\subset \mathbb R ^{n+1}\) be a complete graph of a smooth function \(u:\mathbb R ^n \rightarrow \mathbb R \) such that its mean curvature does not change sign. Suppose that either the scalar curvature \(R\) of \(\Sigma ^n(u)\) or \(u\) is bounded. If \(|\nabla u|\in \mathcal L ^1(\mathbb R ^n)\), then \(\Sigma ^n(u)\) is a hyperplane of \(\mathbb R ^{n+1}\).
Proof
It is well known that the unit normal vector field
defines a Gauss mapping for \(\Sigma ^n(u)\). Letting \(a=e_{n+1}\), we have \(|a^\top |^2=1-f_{a}^2\). Hence, we obtain
Therefore, \(|\nabla u|\in \mathcal L ^1(\mathbb R ^n)\) assures that \(|a^\top |\in \mathcal L ^1(\Sigma ^n(u))\), and hence, the result follows from Theorem 4. \(\square \)
5 Lower estimates for the index of relative nullity
Let \(x:\Sigma ^n\looparrowright {Q}_{c}^{n+1}\) be a hypersurface immersed in a space form \({Q}_{c}^{n+1}\), with second fundamental form A. According to [10], for \(p\in \Sigma ^n\), we define the space of relative nullity \(\Delta (p)\) of \(\Sigma ^n\) at p by
where \(\ker (A_p)\) denotes the kernel of \(A_p\). The index of relative nullity \(\nu (p)\) of \(\Sigma ^n\) at p is the dimension of \(\Delta (p)\), that is,
and the index of minimum relative nullity \(\nu _0\) of \(\Sigma ^n\) is defined by
Now, we are in position to prove the following extension of Theorem 3 to the case of the \(r\)th mean curvatures:
Theorem 5
Let \(x:\Sigma ^n\looparrowright \mathbb R ^{n+1}\) be a complete hypersurface with bounded second fundamental form A and such that, for some \(0\le r\le n-2, H_{r+1}\) and \(H_{r+2}\) do not change sign. Suppose that the image of the Gauss mapping of \(\Sigma ^n\) lies in an open hemisphere of \(\mathbb S ^n\) determined by an unit vector \(a\in \mathbb R ^{n+1}\). If \(|a^\top |\in \mathcal L ^1(\Sigma )\), then the index of minimum relative nullity \(\nu _0\) of \(\Sigma ^n\) is at least \(n-r\). Moreover, if \(H_r\) does not vanish on \(\Sigma ^n\), then through every point of \(\Sigma ^n\), there passes an \((n-r)\)-hyperplane of \(\mathbb R ^{n+1}\) totally contained in \(\Sigma ^n\).
Proof
From (2.4) we have
Thus, the hypothesis that \(H_{r+1}\) does not change sign on \(\Sigma ^n\) and that the image of the Gauss mapping of \(\Sigma ^n\) lies in an open hemisphere of \(\mathbb S ^n\) determined by a assure that \(L_rl_a\) also does not change sign on \(\Sigma ^n\). Consequently, since we are supposing that A is bounded and \(|a^\top |\in \mathcal L ^1(\Sigma )\), from Corollary 1 of [9], we conclude that \(H_{r+1}=0\) on \(\Sigma ^n\). With analogous arguments, from the calculus of \(L_{r+1}l_a\), we also get that \(H_{r+2}=0\) on \(\Sigma ^n\). Thus, from Proposition 2.3(c) of [8], we see that \(H_j=0\) for all \(j\ge r+1\), and hence, \(\nu _0\ge n-r\).
Now, suppose that \(H_r\) does not vanish on \(\Sigma ^n\). By Theorem 5.3 of [10] (see also [12]), the distribution \(p\mapsto \Delta (p)\) of minimal relative nullity of \(\Sigma ^n\) is smooth and integrable with complete leaves, totally geodesic in \(\Sigma ^n\) and in \(\mathbb R ^{n+1}\). Therefore, the result follows from the characterization of complete totally geodesic submanifolds of \(\mathbb R ^{n+1}\) as hyperplanes of suitable dimension. \(\square \)
In what follows, let \(a\in \mathbb L ^{n+2}\) be an unit timelike vector. The level set given by
defines a round sphere of radius one which is a totally geodesic hypersurface of the de Sitter space \(\mathbb S _1^{n+1}\). According to the terminology established in [1], we will refer to that sphere as the equator of \(\mathbb S _1^{n+1}\) determined by \(a\). This equator divides \(\mathbb S _1^{n+1}\) into two connected components, the chronological future which is given by
and the chronological past, given by
In order to establish our last result, we also recall that a hypersurface \(\Sigma ^n\) immersed in a space form is said to be r-minimal if \(H_{r+1}\) vanishes identically on \(\Sigma ^n\). In this setting, we can reason as in the proof of Theorem 5 (working with the support function \(f_a\) instead of the height function \(l_a\), and taking into account the characterization of complete totally geodesic submanifolds of \(\mathbb H ^{n+1}\)) to get the following
Theorem 6
Let \(x:\Sigma ^n\looparrowright \mathbb H ^{n+1}\) be a \(r\)-minimal complete noncompact hypersurface with bounded second fundamental form \(A\) and such that \(H_{r+2}\) does not change sign. Suppose that the image of the Gauss mapping of \(\Sigma ^n\) lies in the chronological future (or past) of the equator of \(\mathbb S _1^{n+1}\) determined by an unit timelike vector \(a\in \mathbb L ^{n+2}\). If \(|a^\top |\in \mathcal L ^1(\Sigma )\), then the index of minimum relative nullity \(\nu _0\) of \(\Sigma ^n\) is at least \(n-r\). Moreover, if \(H_r\) does not vanish on \(\Sigma ^n\), then through every point of \(\Sigma ^n\), there passes an \((n-r)\)-dimensional hyperbolic space \(\mathbb H ^{n-r}\hookrightarrow \mathbb H ^{n+1}\) totally contained in \(\Sigma ^n\).
References
Aledo, J.A., Alías, L.J., Romero, A.: Integral formulas for compact space-like hypersurfaces in de Sitter space: applications to the case of constant higher order mean curvature. J. Geom. Phys. 31, 195–208 (1999)
Alencar, H., do Carmo, M., Colares, G.: Stable hypersurfaces with constant scalar curvature. Math. Z. 213, 117–131 (1993)
Alencar, H., Rosenberg, H., Santos, W.: On the Gauß map of hypersurfaces with constant scalar curvature in spheres. Proc. Am. Math. Soc. 132, 3731–3739 (2004)
Alías, L.J.: On the stability index of minimal and constant mean curvature hypersurfaces in spheres. Revista Uni{\’o}n Math. Argent. 47, 39–61 (2006)
Aquino, C.P., de Lima, H.F.: On the Gauss map of complete CMC hypersurfaces in the hyperbolic space. J. Math. Anal. Appl. 386, 862–869 (2012)
Bernstein, S.: Sur les surfaces d’efinies au moyen de leur courboure moyenne ou totale. Ann. Ec. Norm. Sup. 27, 233–256 (1910)
Caminha, A.: On hypersurfaces into Riemannnian spaces of constant sectional curvature. Kodai Math. J. 29, 185–210 (2006)
Caminha, A.: A rigidity theorem for complete CMC hypersurfaces in Lorentz manifolds. Diff. Geom. Appl. 24, 652–659 (2006)
Camargo, F., Caminha, A., Sousa, P.: Complete foliations of space forms by hypersurfaces. Bull. Braz. Math. Soc. 41, 339–353 (2010)
Dajczer, M., et al.: Submanifolds and Isometric Immersions. Publish or Perish, Houston (1990)
Ejiri, N.: A negative answer to a conjecture of conformal transformations of Riemannian manifolds. J. Math. Soc. Jpn. 33, 261–266 (1981)
Ferus, D.: On the completeness of nullity foliations. Mich. Math. J. 18, 61–64 (1971)
De Giorgi, E.: Una estensione del teorema di Bernstein. Ann. Scuola Norm. Sup. Pisa 19(3), 79–85 (1965)
Hounie, J., Leite, M.L.: The maximum principle for hypersurfaces with vanishing curvature functions. J. Diff. Geom. 41, 247–258 (1995)
Huber, A.: On subharmonic functions and differential geometry in the large. Comment. Math. Helv. 32, 13–72 (1957)
Nomizu, K., Smyth, B.: On the Gauss mapping for hypersurfaces of constant mean curvature in the sphere. Comment. Math. Helv. 44, 484–490 (1969)
Obata, M.: Certain conditions for a Riemannian manifold to be isometric to the sphere. J. Math. Soc. Jpn. 14, 333–340 (1962)
Rosenberg, H.: Hypersurfaces of constant curvature in space forms. Bull. Sci. Math. 117, 217–239 (1993)
Reilly, R.: Variational properties of functions of the mean curvature for hypersurfaces in space form. J. Diff. Geom. 8, 447–453 (1973)
Simons, J.: Minimal varieties in Riemannian manifolds. Ann. Math. 88, 62–105 (1968)
Yau, S.T.: Some function-theoretic properties of complete Riemannian manifolds and their applications to geometry. Indiana Univ. Math. J. 25, 659–670 (1976)
Acknowledgments
This work was started when the first and second authors were visiting the Mathematics and Statistics Department of the Universidade Federal de Campina Grande, with financial support from CNPq, Brazil. They would like to thank this institution for its hospitality. The second author is partially supported by CNPq, Brazil. The third author is partially supported by CAPES/CNPq, Brazil, grant Casadinho/Procad 552.464/2011-2. The authors would like to thank the referee for giving some valuable suggestions which improved the paper.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Barros, A., Aquino, C. & de Lima, H. On the rigidity of hypersurfaces into space forms. Annali di Matematica 193, 689–698 (2014). https://doi.org/10.1007/s10231-012-0297-3
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10231-012-0297-3
Keywords
- Space forms
- Complete hypersurfaces
- Totally geodesic hypersurfaces
- Gauss mapping
- Higher order mean curvatures
- Index of minimum relative nullity