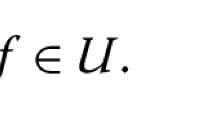Abstract
We study the central limit theorem of the k-th eigenvalue of a random matrix in the log-gas ensemble with an external potential V = q 2m x 2m. More precisely, let P n (dH) = C n e -nTrV(H) dH be the distribution of n × n Hermitian random matrices, ρV (x)dx the equilibrium measure, where C n is a normalization constant, V (x) = q 2m x 2m with \(q2m = \frac{{\Gamma \left( m \right)\Gamma \left( {\frac{1}{2}} \right)}}{{\Gamma \left( {\frac{{2m + 1}}{2}} \right)}}\), and m ≥ 1. Let x 1 ≤... ≤ x n be the eigenvalues of H. Let k:= k(n) be such that \(\frac{{k\left( n \right)}}{n} \in \left[ {a,1 - a} \right]\) for n large enough, where a ∈ (0, 1/2). Define \(G\left( s \right): = \int_{ - 1}^s {\rho v\left( x \right)dx, - 1 \leqslant s \leqslant 1} ,\) and set t:= G −1(k/n). We prove that, as n → ∞, \(\frac{{xk - t}}{{\frac{{\left( {\sqrt {\log n} } \right)}}{{\sqrt {2{\pi ^2}} n\rho v\left( t \right)}}}} \to N\left( {0,1} \right)\) in distribution. Multi-dimensional central limit theorem is also proved. Our results can be viewed as natural extensions of the bulk central limit theorems for GUE ensemble established by J. Gustavsson in 2005.
Similar content being viewed by others
References
Deift, P.: Orthogonal polynomials and random matrices: A Riemann–Hilbert approach, Courant Lecture Notes in Mathematics 3, American Mathematical Society, Providence, RI, 1999
Deift, P., Kriecherbauer, T., McLaughlin, K. T.-R., et al.: Uniform asymptotics for polynomials orthogonal with respect to varying exponential weights and applications to universality questions in random matrix theory. Commun. Pure Appl. Math., 52 1335–1425 (1999)
Deift, P., Kriecherbauer, T., McLaughlin, K. T.-R., et al.: Strong asymptotics of orthogonal polynomials with respect to exponential weights. Comm. Pure Appl. Math., 52 1491–1552 (1999)
Deift, P., Kriecherbauer, T., McLaughlin, K. T.-R., et al.: A Riemann–Hilbert approach to asymptotic questions for orthogonal polynomials. J. Comput. Appl. Math., 133, 47–63 (2001)
Ercolani, N. M., McLaughlin, K. D. T.-R.: Asymptotics of the partition function for random matrices via Riemann–Hilbert techniques and applications to graphical enumeration. Int. Math. Res. Not., 14 755–820 (2003)
Gustavsson, J.: Gaussian fluctuations of eigenvalues in the GUE. Ann. Inst. H. Poincaré–Probabilités et Statistiques, 41 151–178 (2005)
Kriecherbauer, T., McLaughlin, K. T.-R.: Strong asymptotics of polynomials orthogonal with respect to freud weights. Int. Math. Res. Not., 6 299–333 (1999)
Johansson, K.: On fluctuations of eigenvalues of random Hermitian matrices. Duke Math. J., 91 151–204 (1998)
O’Rourke, S.: Gaussian fluctuations of eigenvalues in Wigner random matrices. J. Stat. Phys., 138(6), 1045–1066 (2009)
Soshnikov, A.: Gaussian fluctuations in Airy, Bessel, sine and other determinantal random point fields. J. Statist. Phys., 100 (3/4), 491–522 (2000)
Soshnikov, A.: Gaussian limit for determinantal random point fields. Ann. Probab., 30(1), 171–187 (2002)
Su, Z. G.: Gaussian fluctuations in complex sample covariance matrices. Electr. J. Probab., 11 1284–1320 (2006)
Tao, T., Vu, V.: Random matrices: universality of local eigenvalue statistics. Acta Math., 206 127–204 (2011)
Tracy, C., Widom, H.: Level spacing distributions and the Airy kernel. Commun. Math. Phys., 159 151–174 (1994)
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Zhang, D. Gaussian fluctuations of eigenvalues in log-gas ensemble: Bulk case I. Acta. Math. Sin.-English Ser. 31, 1487–1500 (2015). https://doi.org/10.1007/s10114-015-3685-y
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10114-015-3685-y