Skip to main content
SpringerLink
Log in

Buckling analysis and dynamic response of FGM sandwich cylindrical panels in thermal environments using nonlocal strain gradient theory

  • Original Paper
  • Published:
Acta Mechanica Aims and scope Submit manuscript

Abstract

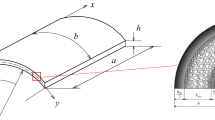
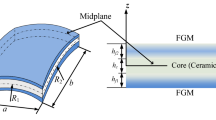
The present paper provides an analysis to obtain the critical buckling load and vibration frequencies of the sandwich cylindrical panel with functionally graded (FG) face sheets and FG porous core resting on an elastic foundation, subjected to mechanical load and in thermal environments. The panel is formulated within the framework of the nonlocal strain gradient theory for shell model and classical shell theory. Based on Hamilton’s principle and Galerkin’s method, the effects of nonlocal and strain gradient parameters, materials and geometrical characteristics, porosity, temperature and elastic foundation on buckling load, fundamental frequencies, and dynamic response of the panel are considered.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

References

  1. Hassan, A.H.A., Kurgan, N.: A review on buckling analysis of functionally graded plates under thermo-mechanical loads. Int. J. Eng. Appl. Sci. 11(1), 345–368 (2019). https://doi.org/10.1016/j.compstruct.2016.05.042

    Article  Google Scholar 

  2. Dai, H.L., Rao, Y.N., Dai, T.: A review of recent researches on FGM cylindrical structures under coupled physical interactions, 2000–2015. Compos. Struct. 152, 199–225 (2016). https://doi.org/10.1016/j.compstruct.2016.05.042

    Article  Google Scholar 

  3. Nejad, M.Z., Jabbari, M., Hadi, A.: A review of functionally graded thick cylindrical and conical shells. J. Comput. Appl. Mech. 48(2), 357–370 (2017)

    Google Scholar 

  4. Mauricio, F.C., Antonio, J.M.F., Volnei, T.: A Review on plate and shell theories for laminated and sandwich Structures highlighting the finite element method. Compos. Struct. 156, 63–77 (2016)

    Article  Google Scholar 

  5. Sun, J., Xu, X., Lim, C.W.: Buckling of functionally graded cylindrical shells under combined thermal and compressive loads. J. Thermal Stress. 37, 340–362 (2014). https://doi.org/10.1080/01495739.2013.869143

    Article  Google Scholar 

  6. Zhou, F., Chen, Z., Fan, H., Huang, S.: An analytical study on the buckling of cylindrical shells with stepwise variable thickness subjected to uniform external pressure. Mech. Adv. Mater. Struct. 23(10), 1207–1215 (2016). https://doi.org/10.1080/15376494.2015.1068401

    Article  Google Scholar 

  7. Nam, V.H., Trung, N.T., Hoa, L.K.: Buckling and postbuckling of porous cylindrical shells with functionally graded composite coating under torsion in thermal environment. Thin-Walled Struct. 144, 106253 (2019). https://doi.org/10.1016/j.tws.2019.106253

    Article  Google Scholar 

  8. Khazaeinejad, P., Najafizadeh, M.M., Jenabi, J., Isvandzibaei, M.R.: On the buckling of functionally graded cylindrical shells under combined external pressure and axial compression. J. Pressure Vessel Technol. 132(6), 064501 (2010). https://doi.org/10.1115/1.4001659

    Article  Google Scholar 

  9. Bagherizadeh, E., Kiani, Y., Eslami, M.R.: Mechanical buckling of functionally graded material cylindrical shells surrounded by Pasternak elastic foundation. Compos. Struct. 93, 3063–3071 (2011). https://doi.org/10.1016/j.compstruct.2011.04.022

    Article  Google Scholar 

  10. Sun, J., Xu, X., Lim, C.W., Qiao, W.: Accurate buckling analysis for shear deformable FGM cylindrical shells under axial compression and thermal loads. Compos. Struct. 123, 246–256 (2015). https://doi.org/10.1016/j.compstruct.2014.12.030

    Article  Google Scholar 

  11. Zenkou, A.M., Radwan, A.F.: Bending and buckling analysis of FGM plates resting on elastic foundations in hygrothermal environment. Arch. Civil. Mech. Enginee. 20, 112 (2020). https://doi.org/10.1007/s43452-020-00116-z

    Article  Google Scholar 

  12. Akbari, M., Azadi, M., Fahham, H.: Free vibration analysis of thick sandwich cylindrical panels with saturated FG-porous core. Mech. Adv. Mater. Struct. (2020). https://doi.org/10.1080/15397734.2020.1748051

    Article  Google Scholar 

  13. Chehreghani, M., Pazhooh, M.D., Shakeri, M.: Vibration analysis of a fluid conveying sandwich cylindrical shell with a soft core. Compos. Struct. 230, 111470 (2019). https://doi.org/10.1016/j.compstruct.2019.111470

    Article  Google Scholar 

  14. Karroubi, R., Irani-rahaghi, M.: Rotating sandwich cylindrical shells with an FGM core and two FGPM layers: free vibration analysis. Appl. Math. Mech. -Engl. Ed. 40(4), 563–578 (2019). https://doi.org/10.1007/s10483-019-2469-8

    Article  MathSciNet  MATH  Google Scholar 

  15. Keleshteri, M.M., Jelovica, J.: Nonlinear vibration behavior of functionally graded porous cylindrical panels. Compos. Struct. 239, 112028 (2020). https://doi.org/10.1016/j.compstruct.2020.112028

    Article  Google Scholar 

  16. Fard, K.M., Gholami, M., Reshadi, F., Livani, M.: Free vibration and buckling analyses of cylindrical sandwich panel with magneto rheological fluid laye. J. Sand. Struct. Mat. 19(4), 397–423 (2017). https://doi.org/10.1177/1099636215603034

    Article  Google Scholar 

  17. Sofiyev, A.H.: The vibration and buckling of sandwich cylindrical shells covered by different coatings subjected to the hydrostatic pressure. Compos. Struct. 117, 124–134 (2014). https://doi.org/10.1016/j.compstruct.2014.06.025

    Article  Google Scholar 

  18. Ashok, R.B., Srinivasa, C.V., Suresh, Y.J., Prema Kumar, W.P.: Buckling behaviour of cylindrical panels. Nonlinear Eng. 4(2), 67–75 (2015). https://doi.org/10.1515/nleng-2014-0019

    Article  Google Scholar 

  19. Lopatin, A.V., Morozov, E.V.: Buckling analysis of the SSCC composite sandwich cylindrical panel under axial compression. J. Sand. Struct. Mat. 23(4), 1292–1310 (2019). https://doi.org/10.1177/1099636219857172

    Article  Google Scholar 

  20. Sadighi, M., Abouhamzeh, M.: Buckling optimisation of sandwich cylindrical panels. Curved Layer. Struct. 3(1), 137–145 (2016). https://doi.org/10.1515/cls-2016-0011

    Article  Google Scholar 

  21. Ahmadia, S.A., Pashaeia, M.H., Jafari-Talookolaei, R.A.: Buckling analysis of sandwich orthotropic cylindrical shells by considering the geometrical imperfection in face-sheets. Appl. Comput. Mech. 13(1), 5–20 (2019)

    Google Scholar 

  22. Mohammadi, F., Sedaghati, R.: Linear and nonlinear vibration analysis of sandwich cylindrical shell with constrained viscoelastic core layer. Int. J. Mech. Sci. 54, 156–171 (2012). https://doi.org/10.1016/j.ijmecsci.2011.10.006

    Article  Google Scholar 

  23. Ansari, R., Shahabodini, A., Faghih Shojaei, M.: Nonlocal three-dimensional theory of elasticity with application to free vibration of functionally graded nanoplates on elastic foundations. Phys E: Low-Dimen. Sys. Nanostruct. 76, 70–81 (2016). https://doi.org/10.1016/j.physe.2015.09.042

    Article  Google Scholar 

  24. Bouazza, M., Becheri, T., Boucheta, A., Benseddiq, N.: Thermal buckling analysis of nanoplates based on nonlocal elasticity theory with four-unknown shear deformation theory resting on Winkler-Pasternak elastic foundation. Inter. J. Comput. Meth. Engineer. Sci. Mech. 17(5–6), 362–373 (2016). https://doi.org/10.1080/15502287.2016.1231239

    Article  MathSciNet  Google Scholar 

  25. Zenkour, A.M., Arefi, M., Alshehri, N.A.: Size-dependent analysis of a sandwich curved nanobeam integrated with piezomagnetic face-sheets. Results. Phys. 7, 2172–2182 (2017). https://doi.org/10.1016/j.rinp.2017.06.032

    Article  Google Scholar 

  26. Arefi, M., Civalek, O.: Static analysis of functionally graded composite shells on elastic foundations with nonlocal elasticity theory. Archiv. Civ. Mech. Eng. 20, 22 (2020). https://doi.org/10.1007/s43452-020-00032-2

    Article  Google Scholar 

  27. Sahmani, S., Aghdam, M.M.: Size dependency in axial postbuckling behavior of hybrid FGM exponential shear deformable nanoshells based on the nonlocal elasticity theory. Compos. Struct. 166, 104–113 (2017). https://doi.org/10.1016/j.compstruct.2017.01.051

    Article  Google Scholar 

  28. Sun, J., Wang, Z., Zhou, Z., Xu, X.: Surface effects on the buckling behaviors of piezoelectric cylindrical nanoshells using nonlocal continuum model. Appl. Math. Model. 59, 341–356 (2018). https://doi.org/10.1016/j.apm.2018.01.032

    Article  MathSciNet  MATH  Google Scholar 

  29. Ashoori, A., Salari, E., Sadough Vanini, S.A.E.: Size-dependent thermal stability analysis of embedded functionally graded annular nanoplates based on the nonlocal elasticity theory. Int. J. Mech. Sci. 119, 396–411 (2016). https://doi.org/10.1016/j.ijmecsci.2016.10.035

    Article  Google Scholar 

  30. Czekanski, A., Zozulya, V.V.: Vibration analysis of nonlocal beams using higher-order theory and comparison with classical models. Mech. Adv. Mater. Struct. 28(12), 1293–1309 (2021). https://doi.org/10.1080/15376494.2019.1665761

    Article  Google Scholar 

  31. Yang, L., Lianzhi, Y., Liangliang, Z., Gao, Y.: Nonlocal free and forced vibration of multilayered two-dimensional quasicrystal nanoplates. Mech. Adv. Mater. Struct. 28(12), 1216–1226 (2021). https://doi.org/10.1080/15376494.2019.1655687

    Article  Google Scholar 

  32. Fang, J., Zheng, S., Xiao, J., Zhang, X.: Vibration and thermal buckling analysis of rotating nonlocal functionally graded nanobeams in thermal environment. Aerosp. Sci. Technol. 106, 106146 (2020). https://doi.org/10.1016/j.ast.2020.106146

    Article  Google Scholar 

  33. Nami, M.R., Janghorban, M., Damadam, M.: Thermal buckling analysis of functionally graded rectangular nanoplates based on nonlocal third-order shear deformation theory. Aerosp. Sci. Technol. 41, 7–15 (2015). https://doi.org/10.1016/j.ast.2014.12.001

    Article  Google Scholar 

  34. Hadjesfandiari, A.R., Dargush, G.F.: Couple stress theory for solids. Int. J. Solids Struct. 48, 2496–2510 (2011)

    Article  Google Scholar 

  35. Dargush, G.F., Apostolakis, G., Hadjesfandiari, A.R.: Two- and three-dimensional size-dependent couple stress response using a displacement-based variational method. Eur. J. Mech. A. Solids 88, 104268 (2021). https://doi.org/10.1016/j.euromechsol.2021.104268

    Article  MathSciNet  MATH  Google Scholar 

  36. Ghadiri, M., SafarPour, H.: Free vibration analysis of size-dependent functionally graded porous cylindrical microshells in thermal environment. J. Thermal. Stress. 40(1), 55–71 (2017). https://doi.org/10.1080/01495739.2016.1229145

    Article  Google Scholar 

  37. Mehralian, F., Beni, Y.T., Ansari, R.: Size dependent buckling analysis of functionally graded piezoelectric cylindrical nanoshell. Compos. Struct. 152, 45–61 (2015). https://doi.org/10.1016/j.compstruct.2016.05.024

    Article  Google Scholar 

  38. Ghayesh, M.H., Farokhi, H., Amabili, M.: Nonlinear dynamics of a microscale beam based on the modified couple stress theory. Compos Part B: Eng. 50, 318–324 (2013). https://doi.org/10.1016/j.compositesb.2013.02.021

    Article  MATH  Google Scholar 

  39. Akgöz, B., Civalek, Ö.: Free vibration analysis of axially functionally graded tapered Bernoulli-Euler microbeams based on the modified couple stress theory. Compos. Struct. 98, 314–322 (2013). https://doi.org/10.1016/j.compstruct.2012.11.020

    Article  Google Scholar 

  40. Farokhi, H., Ghayesh, M.H.: Nonlinear dynamical behaviour of geometrically imperfect microplates based on modified couple stress theory. Int. J. Mech. Sci. 90, 133–144 (2015). https://doi.org/10.1016/j.ijmecsci.2014.11.002

    Article  Google Scholar 

  41. Ansari, R., Faghih Shojaei, M., Mohammadi, V., Gholami, R., Darabi, M.A.: Nonlinear vibrations of functionally graded Mindlin microplates based on the modified couple stress theory. Compos. Struct. 114, 124–134 (2014). https://doi.org/10.1016/j.compstruct.2014.04.013

    Article  Google Scholar 

  42. Shafiei, N., Kazemi, M.: Buckling analysis on the bi-dimensional functionally graded porous tapered nano-/micro-scale beams. Aerosp. Sci. Technol. 66, 1–11 (2017). https://doi.org/10.1016/j.ast.2017.02.019

    Article  Google Scholar 

  43. Farzam, A., Hassani, B.: Isogeometric analysis of in-plane functionally graded porous microplates using modified couple stress theory. Aerosp. Sci. Technol. 91, 508–524 (2019). https://doi.org/10.1016/j.ast.2019.05.012

    Article  Google Scholar 

  44. Anoop Krishnan, N.M., Ghosh, D.: Buckling analysis of cylindrical thin-shells using strain gradient elasticity theory. Meccanica 52, 1369–1379 (2017). https://doi.org/10.1007/s11012-016-0468-1

    Article  MathSciNet  MATH  Google Scholar 

  45. Lazopoulos, K., Lazopoulos, A.K.: Nonlinear strain gradient elastic thin shallow shells. Eur. J. Mech. A/Solids. 30(3), 286–292 (2011). https://doi.org/10.1016/j.euromechsol.2010.12.011

    Article  MathSciNet  MATH  Google Scholar 

  46. Shahraki, H., Riahi, H.T., Izadinia, M., Talaeitaba, S.B.: Mindlin’s strain gradient theory for vibration analysis of FG-CNT-reinforced composite nanoplates resting on Kerr foundation in thermal environment. J. Therm. Compos. Mater. 34(1), 68–101 (2019). https://doi.org/10.1177/0892705719843175

    Article  Google Scholar 

  47. Cornacchia, F., Fabbrocino, F., Fantuzzi, N., Luciano, R., Penna, R.: Anlalytical solution of cross- and angle-ply nano plates with strain gradient theory for linear vibration and buckling. Mech. Adv. Mater. Struct. 28(12), 1201–1215 (2021). https://doi.org/10.1080/15376494.2019.1655613

    Article  Google Scholar 

  48. Lim, C.W., Zhang, G., Reddy, J.N.: A higher-order nonlocal elasticity and strain gradient theory and its applications in wave propagation. J. Mech. Phys. Solids. 78, 298–313 (2015). https://doi.org/10.1016/j.jmps.2015.02.001

    Article  MathSciNet  MATH  Google Scholar 

  49. Li, L., Li, L., Hu, Y.: Free vibration analysis of nonlocal strain gradient beams made of functionally graded material. Int. J. Eng. Sci. 102, 77–92 (2016). https://doi.org/10.1016/j.ijengsci.2016.02.010

    Article  MATH  Google Scholar 

  50. Şimşek, M.: Nonlinear free vibration of a functionally graded nanobeam using nonlocal strain gradient theory and a novel Hamiltonian approach. Int. J. Eng. Sci. 105, 12–27 (2016). https://doi.org/10.1016/j.ijengsci.2016.04.013

    Article  MathSciNet  MATH  Google Scholar 

  51. Ebrahimi, F., Barati, M.R.: A nonlocal strain gradient refined beam model for buckling analysis of size-dependent shear-deformable curved FG nanobeams. Compos. Struct. 159, 174–182 (2017). https://doi.org/10.1016/j.compstruct.2016.09.058

    Article  Google Scholar 

  52. Hieu, D.V., Chan, D.Q.: Nonlinear vibration and stability of functionally graded porous microbeam under electrostatic actuation. Arch. Appl. Mech. 91, 2301–2329 (2021). https://doi.org/10.1007/s00419-021-01884-7

    Article  Google Scholar 

  53. Hieu, D.V., Sedighi, H.M., Chan, D.Q., Civalek, O., Abouelregal, A.E.: Nonlinear vibration and stability of FG nanotubes conveying fluid via nonlocal strain gradient theory. Struct. Eng. Mech. 78(1), 103–116 (2021)

    Google Scholar 

  54. Ebrahimi, F., Barati, M.R., Dabbagh, A.: A nonlocal strain gradient theory for wave propagation analysis in temperature-dependent inhomogeneous nanoplates. Int. J. Eng. Sci. 107, 169–182 (2016). https://doi.org/10.1016/j.ijengsci.2016.07.008

    Article  Google Scholar 

  55. Farajpour, A., Haeri Yazdi, M.R., Rastgoo, A., Mohammadi, M.: A higher-order nonlocal strain gradient plate model for buckling of orthotropic nanoplates in thermal environment. Acta. Mech. 227, 1849–1867 (2016). https://doi.org/10.1007/s00707-016-1605-6

    Article  MathSciNet  MATH  Google Scholar 

  56. Hossein, G.S.M., Shirko, F.: Free vibration and wave propagation of thick plates using the generalized nonlocal strain gradient theory. J. Theor. Appl. Vib. Acous. 3(2), 165–198 (2017)

    Google Scholar 

  57. Barati, M.R., Shahverdi, H.: Hygro-thermal vibration analysis of graded double-refined-nanoplate systems using hybrid nonlocal stress-strain gradient theory. Compos. Struct. 176, 982–995 (2017). https://doi.org/10.1016/j.compstruct.2017.06.004

    Article  Google Scholar 

  58. Tai, T.H., Jalaei, M.H.J.: Dynamic stability of viscoelastic porous FG nanoplate under longitudinal magnetic field via a nonlocal strain gradient quasi-3D theory. Compos. Part B: Eng. 175, 107164 (2019). https://doi.org/10.1016/j.compositesb.2019.107164

    Article  Google Scholar 

  59. Ebrahimi, F., Barati, M.R.: Hygrothermal effects on static stability of embedded single-layer graphene sheets based on nonlocal strain gradient elasticity theory. J. Ther. Stress. 42(12), 1535–1550 (2019). https://doi.org/10.1080/01495739.2019.1662352

    Article  Google Scholar 

  60. Sharifi, Z., Khordad, R., Gharaati, A., Forozani, G.: An analytical study of vibration in functionally graded piezoelectric nanoplates: nonlocal strain gradient theory. Appl. Math. Mech. -Engl. Ed. 40(12), 1723–1740 (2019). https://doi.org/10.1007/s10483-019-2545-8

    Article  MathSciNet  MATH  Google Scholar 

  61. Abazid, M.A.: The nonlocal strain gradient theory for hygro-thermo-electro-magnetic effects on buckling, vibration and wave propagation in piezo-electro-magnetic nanoplates. Int. J. Appl. Mech. 11(7), 1950067 (2019). https://doi.org/10.1142/S1758825119500674

    Article  Google Scholar 

  62. Arefi, M., Kiani, M., Rabczuk, T.: Application of nonlocal strain gradient theory to size dependent bending analysis of a sandwich porous nanoplate integrated with piezomagnetic face-sheets. Compos. Part B: Eng. 168, 320–333 (2019). https://doi.org/10.1016/j.compositesb.2019.02.057

    Article  Google Scholar 

  63. Barati, M.R.: Vibration analysis of porous FG nanoshells with even and uneven porosity distributions using nonlocal strain gradient elasticity. Acta. Mech. 229, 1183–1196 (2018). https://doi.org/10.1007/s00707-017-2032-z

    Article  MathSciNet  MATH  Google Scholar 

  64. Sahmani, S., Aghdam, M.M.: Nonlocal strain gradient shell model for axial buckling and postbuckling analysis of magneto-electro-elastic composite nanoshells. Compos. Part B 132, 258–274 (2018). https://doi.org/10.1016/j.compositesb.2017.09.004

    Article  Google Scholar 

  65. Sahmani, S., Fattahi, A.M.: Small scale effects on buckling and postbuckling behaviors of axially loaded FGM nanoshells based on nonlocal strain gradient elasticity theory. Appl. Math. Mech. -Engl. Ed. 39, 561–580 (2018). https://doi.org/10.1007/s10483-018-2321-8

    Article  MathSciNet  MATH  Google Scholar 

  66. Mehralian, F., Beni, Y.T.: A nonlocal strain gradient shell model for free vibration analysis of functionally graded shear deformable nanotubes. Int. J. Eng. Appl. Sci. (IJEAS) 9(2), 88–102 (2017)

    Google Scholar 

  67. Lu, L., Zhu, L., Zhao, J., Liu, G.: A nonlocal strain gradient shell model incorporating surface effects for vibration analysis of functionally graded cylindrical nanoshells. Appl. Math. Mech. -Engl. Ed. 40(12), 1695–1722 (2019). https://doi.org/10.1007/s10483-019-2549-7

    Article  MathSciNet  MATH  Google Scholar 

  68. Ma, L.H., Ke, L.L., Reddy, J.N., Yang, J., Kitipornchai, S., Wang, Y.S.: Wave propagation characteristics in magneto-electro-elastic nanoshells using nonlocal strain gradient theory. Compos. Struct. 19, 10–23 (2018). https://doi.org/10.1016/j.compstruct.2018.05.061

    Article  Google Scholar 

  69. Babaei, H., Eslami, M.R.: On nonlinear vibration and snap-through buckling of long FG porous cylindrical panels using nonlocal strain gradient theory. Compos. Struct. 256, 113125 (2021). https://doi.org/10.1016/j.compstruct.2020.113125

    Article  Google Scholar 

  70. Zhao, X., Liew, K.M.: A mesh-free method for analysis of the thermal and mechanical buckling of functionally graded cylindrical shell panels. Comput. Mech. 45, 297–310 (2010). https://doi.org/10.1007/s00466-009-0446-8

    Article  MATH  Google Scholar 

  71. Brush, D.O., Almroth, B.O.: Buckling of bars, plates and shells. McGraw-Hill, New York (1975)

    Book  Google Scholar 

  72. Eslami, M. R., Buckling and postbuckling of beams, plates, and shells, Springer International Publishing AG, Gewerbestrasse 11, 6330 Cham, Switzerland, 2018.

  73. Timoshenko, S., Gere, J., Theory of elastic stability, 2nd edn, New York: McGraw-Hill, 1961.

Download references

Acknowledgements

This research is funded by the Project number QG.21.25 of VNU Hanoi “Nonlinear stability of laminated smart composite plates and shells”. The authors are grateful for this support.

Author information

Authors and Affiliations

  1. Faculty of Mechanical Engineering and Mechatronics, Phenikaa University, Ha Dong, Hanoi, 12116, Vietnam

    Do Quang Chan & Tran Quoc Quan

  2. PHENIKAA Research and Technology Institute (PRATI), A&A Green Phoenix Group JSC, No. 167 Hoang Ngan, Trung Hoa, Cau Giay, Hanoi, 11313, Vietnam

    Do Quang Chan

  3. Phenikaa Institute for Advanced Study (PIAS), Phenikaa University, Ha Dong, Hanoi, 12116, Vietnam

    Tran Quoc Quan

  4. University of Transport Technology, 54 Trieu Khuc, Thanh Xuan, Hanoi, Vietnam

    Bui Gia Phi

  5. Thainguyen University of Technology, Thainguyen, Vietnam

    Dang Van Hieu

  6. Departemnt of Engineering and Technology in Constructions and Transportation, VNU Hanoi - University of Engineering and Technology, 144 Xuan Thuy, Cau Giay, Hanoi, Vietnam

    Nguyen Dinh Duc

Authors
  1. Do Quang Chan
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Tran Quoc Quan
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Bui Gia Phi
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Dang Van Hieu
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Nguyen Dinh Duc
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

NDD: Conceptualization, Funding acquisition, Methodology, Supervision, Writing—review and editing; Corresponding author. DQC: Investigation, Methodology, Validation, Writing—original draft. TQQ: Investigation, Methodology. BGP: Investigation, Methodology, Software, Validation. DVH: Investigation, Methodology, Software, Validation.

Corresponding author

Correspondence to Nguyen Dinh Duc.

Ethics declarations

Conflict of interest

The authors declared no potential conflicts of interest with respect to the research, authorship, and/or publication of this article.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendices

Appendix 1

1.1 FG porous type 1

$$E_{1} = E_{c} \left( {h - h_{{{\text{core}}}} } \right) + \frac{{E_{mc} \left( {h - h_{{{\text{core}}}} } \right)}}{k + 1} + \frac{{E_{m} h_{{{\text{core}}}} {\mkern 1mu} \left( {\pi - 2{\mkern 1mu} e_{0} } \right)}}{\pi }$$
(52)
$$E_{2} = 0$$
$$\begin{gathered} E_{3} = {\mkern 1mu} \frac{{E_{c} \left( {h^{3} - h_{{{\text{core}}}}^{3} } \right)}}{12} + \frac{{E_{mc} \left( {2{\mkern 1mu} h^{3} + 2{\mkern 1mu} kh^{2} h_{{{\text{core}}}} + k\left( {k + 1} \right)hh_{{{\text{core}}}}^{2} - \left( {k\left( {k + 2} \right) + 1} \right)h_{{{\text{core}}}}^{3} } \right)}}{{4k\left( {k + 2} \right)\left( {k + 3} \right) + 1}} \hfill \\ \,\qquad\qquad + {\mkern 1mu} \frac{{E_{m} h_{{{\text{core}}}}^{3} \left( {\pi^{3} - 6{\mkern 1mu} e_{0} \pi^{2} + 48{\mkern 1mu} e_{0} } \right)}}{{12\pi^{3} }} \hfill \\ \end{gathered}$$

1.2 FG porous type 2

$$E_{1} = E_{c} \left( {h - h_{{{\text{core}}}} } \right) + \frac{{E_{mc} \left( {h - h_{{{\text{core}}}} } \right)}}{k + 1} + \frac{{E_{m} h_{{{\text{core}}}} {\mkern 1mu} \left( {\pi - 2{\mkern 1mu} e_{0} } \right)}}{\pi }$$
$$E_{2} = - \frac{{E_{m} h_{{{\text{core}}}}^{2} e_{0} \left( {\pi - 4} \right)}}{{\pi^{2} }}$$
(53)
$$\begin{gathered} E_{3} = {\mkern 1mu} \frac{{E_{c} \left( {h^{3} - h_{{{\text{core}}}}^{3} } \right)}}{12} + {\mkern 1mu} \frac{{E_{mc} \left( {2{\mkern 1mu} h^{3} + 2{\mkern 1mu} kh^{2} h_{{{\text{core}}}} + k\left( {k + 1} \right)hh_{{{\text{core}}}}^{2} - \left( {k\left( {k + 2} \right) + 1} \right)h_{{{\text{core}}}}^{3} } \right)}}{{4k\left( {k + 2} \right)\left( {k + 3} \right) + 1}} \hfill \\ \qquad\qquad+ {\mkern 1mu} \frac{{E_{m} h_{{{\text{core}}}}^{3} \left( {\pi^{3} - 6{\mkern 1mu} e_{0} \pi^{2} + 192{\mkern 1mu} e_{0} - 48{\mkern 1mu} \pi {\mkern 1mu} e_{0} } \right)}}{{12\pi^{3} }} \hfill \\ \end{gathered}$$

1.3 FG porous type 3

$$E_{1} = E_{c} \left( {h - h_{{{\text{core}}}} } \right) + \frac{{E_{mc} \left( {h - h_{{{\text{core}}}} } \right)}}{k + 1} + E_{m} h_{{{\text{core}}}} {\mkern 1mu} \left( {1 - 2\lambda_{0} e_{0} } \right)$$
$$E_{2} = 0$$
$$\begin{gathered} E_{3} = {\mkern 1mu} \frac{{E_{c} \left( {h^{3} - h_{{{\text{core}}}}^{3} } \right)}}{12} + {\mkern 1mu} \frac{{E_{mc} \left( {2{\mkern 1mu} h^{3} + 2{\mkern 1mu} kh^{2} h_{{{\text{core}}}} + k\left( {k + 1} \right)hh_{{{\text{core}}}}^{2} - \left( {k\left( {k + 2} \right) + 1} \right)h_{{{\text{core}}}}^{3} } \right)}}{{4k\left( {k + 2} \right)\left( {k + 3} \right) + 1}} \hfill \\ \qquad\qquad+ {\mkern 1mu} \frac{{E_{m} h_{{{\text{core}}}}^{3} \left( {1 - {\mkern 1mu} e_{0} \lambda_{0} } \right)}}{{12\pi^{3} }} \hfill \\ \end{gathered}$$
(54)

Appendix 2

$$X_{11} = - {\mkern 1mu} \frac{{\pi^{2} \left[ {R^{2} \theta_{0}^{2} \left( {L^{2} + \pi^{2} l^{2} m^{2} } \right) + n^{2} \pi^{2} l^{2} L^{2} } \right]}}{{4L^{3} R^{3} \theta_{0}^{3} }}\left( {m^{2} R^{2} \theta_{0}^{2} A_{11} + n^{2} L^{2} A_{33} } \right)$$
(55)
$$X_{12} = - {\mkern 1mu} \frac{{n\pi^{2} m\left[ {R^{2} \theta_{0}^{2} \left( {L^{2} + \pi^{2} l^{2} m^{2} } \right) + n^{2} \pi^{2} l^{2} L^{2} } \right]}}{{4R^{2} \theta_{0}^{2} L^{2} }}\left( {A_{12} + A_{33} } \right)$$
(56)
$$X_{13} = {\mkern 1mu} \frac{{\pi {\mkern 1mu} m\left[ {R^{2} \theta_{0}^{2} \left( {L^{2} + \pi^{2} l^{2} m^{2} } \right) + n^{2} \pi^{2} l^{2} L^{2} } \right]}}{{4L^{4} R^{3} \theta_{0}^{3} }}\left[ {\left( {\pi^{2} m^{2} RB_{11} - L^{2} A_{12} } \right)R\theta_{0}^{2} + n^{2} \pi^{2} L^{2} \left( {B_{12} + B_{33} } \right)} \right]$$
(57)
$$X_{21} = - {\mkern 1mu} \frac{{n\pi^{2} m\left[ {\left( {\pi^{2} l^{2} m^{2} + L^{2} } \right)R^{2} \theta_{0}^{2} + n^{2} \pi^{2} l^{2} L^{2} } \right]}}{{4L^{2} R^{2} \theta_{0}^{2} }}\left( {A_{21} + A_{33} } \right)$$
(58)
$$X_{22} = - {\mkern 1mu} \frac{{\pi^{2} \left[ {\left( {\pi^{2} l^{2} m^{2} + L^{2} } \right)R^{2} \theta_{0}^{2} + n^{2} \pi^{2} l^{2} L^{2} } \right]}}{{4L^{3} R^{3} \theta_{0}^{3} }}\left( {n^{2} L^{2} A_{22} + m^{2} R^{2} \theta_{0}^{2} A_{33} } \right)$$
(59)
$$X_{23} = {\mkern 1mu} {\mkern 1mu} \frac{{n\pi {\mkern 1mu} \left[ {\left( {\pi^{2} l^{2} m^{2} + L^{2} } \right)R^{2} \theta_{0}^{2} + n^{2} \pi^{2} l^{2} L^{2} } \right]}}{{4L^{3} R^{4} \theta_{0}^{4} }}\left[ {L^{2} \left( {n^{2} \pi^{2} B_{22} - R\theta_{0}^{2} A_{22} } \right) + \pi^{2} m^{2} R^{2} \theta_{0}^{2} \left( {B_{21} + B_{33} } \right)} \right]$$
(60)
$$X_{31} = {\mkern 1mu} \frac{{\pi {\mkern 1mu} m\left[ {\left( {L^{2} + \pi^{2} l^{2} m^{2} } \right)\theta_{0}^{2} R^{2} + n^{2} \pi^{2} l^{2} L^{2} } \right]}}{{4L^{4} R^{3} \theta_{0}^{3} }}\left[ {\left( {m^{2} R\pi^{2} B_{11} - L^{2} A_{21} } \right)R\theta_{0}^{2} + L^{2} \pi^{2} n^{2} \left( {B_{21} + 2{\mkern 1mu} B_{66} } \right)} \right]$$
(61)
$$X_{32} = {\mkern 1mu} \frac{{n\pi {\mkern 1mu} \left( {\left( {L^{2} + \pi^{2} l^{2} m^{2} } \right)\theta_{0}^{2} R^{2} + n^{2} \pi^{2} l^{2} L^{2} } \right)}}{{4L^{3} R^{4} \theta_{0}^{4} }}\left[ {L^{2} \left( {n^{2} \pi^{2} B_{22} - R\theta_{0}^{2} A_{22} } \right) + \pi^{2} m^{2} R^{2} \theta_{0}^{2} \left( {B_{12} + 2{\mkern 1mu} B_{66} } \right)} \right]$$
(62)
$$X_{33} = {\mkern 1mu} - \frac{{\left[ {\left( {L^{2} + \pi^{2} l^{2} m^{2} } \right)\theta_{0}^{2} R^{2} + n^{2} \pi^{2} l^{2} L^{2} } \right]}}{{4L^{5} R^{5} \theta_{0}^{5} }}\left[ \begin{gathered} L^{4} R^{2} \theta_{0}^{4} A_{22} + n^{2} \pi^{2} L^{4} \left( {n^{2} \pi^{2} D_{22} - 2{\mkern 1mu} R\theta_{0}^{2} B_{22} } \right) \hfill \\ - \pi^{2} L^{2} \theta_{0}^{4} m^{2} R^{3} \left( {B_{12} + B_{21} } \right) + m^{4} R^{4} \theta_{0}^{4} \pi^{4} D_{11} \hfill \\ + L^{2} \theta_{0}^{2} m^{2} n^{2} R^{2} \pi^{4} \left( {D_{12} + D_{21} + 2{\mkern 1mu} D_{66} } \right) \hfill \\ \end{gathered} \right]$$
(63)
$$X_{34} = {\mkern 1mu} \frac{{h\pi^{2} m^{2} \left[ {\mu^{2} \pi^{2} n^{2} L^{2} + \left( {\mu^{2} \pi^{2} m^{2} + L^{2} } \right)R^{2} \theta_{0}^{2} } \right]}}{{4RL^{3} \theta_{0} }}$$
(64)
$$X_{35} = {\mkern 1mu} \frac{{R^{2} L^{2} \theta_{0}^{2} + \mu^{2} \pi^{2} m^{2} R^{2} \theta_{0}^{2} + \mu^{2} \pi^{2} n^{2} L^{2} }}{{4RL\theta_{0} }}$$
(65)
$$X_{36} = {\mkern 1mu} \frac{{\pi^{2} \left( {R^{2} \theta_{0}^{2} m^{2} + n^{2} L^{2} } \right)\left( {R^{2} L^{2} \theta_{0}^{2} + \mu^{2} \pi^{2} m^{2} R^{2} \theta_{0}^{2} + \mu^{2} \pi^{2} n^{2} L^{2} } \right)}}{{4R^{3} L^{3} \theta_{0}^{3} }}$$
(66)
$$X_{37} = {\mkern 1mu} - \frac{{LR\theta_{0} \left[ { - 1 + ( - 1)^{m} } \right]\left[ { - 1 + ( - 1)^{n} } \right]}}{{nm\pi^{2} }}$$
(67)

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Chan, D.Q., Quan, T.Q., Phi, B.G. et al. Buckling analysis and dynamic response of FGM sandwich cylindrical panels in thermal environments using nonlocal strain gradient theory. Acta Mech 233, 2213–2235 (2022). https://doi.org/10.1007/s00707-022-03212-8

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00707-022-03212-8

Search

Navigation