Bending and buckling analysis of FGM plates resting on elastic foundations in hygrothermal environment

Abstract

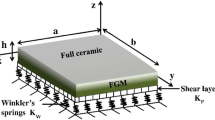
The present paper deals with the effect of exponential temperature and moisture concentration on the bending and buckling analysis of functionally graded plates resting on two-parameter elastic foundations via a four-variable exponential shear deformation theory. The mechanical properties of the plates are assumed to vary through the thickness. The equations of equilibrium are derived using Hamilton’s principle. The present solutions are derived using Navier’s method. Using Navier’s solution the numerical results are presented and compared well with those available in the literature. Discussions are made to show how the foundation stiffness, hygrothermal loading and other parameters have a significant influence on the bending and buckling analysis of FG plates under hygrothermal and mechanical loading.

Appendices

Appendix A

The elements ${D}_{ij}={D}_{ji}$ and ${A}_{ij}$ presented in Eq. (12) are given by:

$$\left\{{D}_{11},{D}_{13},{D}_{15}\right\}={\int }_{-h/2}^{h/2}{Q}_{11}\left\{1,z,f\left(z\right)\right\}\mathrm{d}z,$$

$$\left\{{D}_{12},{D}_{14},{D}_{16}\right\}={\int }_{-h/2}^{h/2}{Q}_{12}\left\{1,z,f\left(z\right)\right\}\mathrm{d}z,$$

$$\left\{{D}_{22},{D}_{24},{D}_{26}\right\}={\int }_{-h/2}^{h/2}{Q}_{22}\left\{1,z,f\left(z\right)\right\}\mathrm{d}z,$$

$$\left\{{D}_{44},{D}_{45},{D}_{46}\right\}={\int }_{-h/2}^{h/2}z\left\{z{Q}_{22},f\left(z\right){Q}_{12},f\left(z\right){Q}_{22}\right\}\mathrm{d}z,$$

$$\left\{{D}_{33},{D}_{35},{D}_{34},{D}_{36}\right\}={\int }_{-h/2}^{h/2}z\left\{z{Q}_{11},f\left(z\right){Q}_{11},z{Q}_{12},f\left(z\right){Q}_{12}\right\}\mathrm{d}z,$$

$$\left\{{D}_{55},{D}_{56},{D}_{66}\right\}={\int }_{-h/2}^{h/2}{\left[f\left(z\right)\right]}^{2}\left\{{Q}_{11},{Q}_{12},{Q}_{22}\right\}\mathrm{d}z,$$

$$\left\{{D}_{23},{D}_{25}\right\}={\int }_{-h/2}^{h/2}{Q}_{12}\left\{z,f\left(z\right)\right\}\mathrm{d}z,$$

$$\left\{{A}_{11},{A}_{12},{A}_{13}\right\}={\int }_{-h/2}^{h/2}{Q}_{66}\left\{1,z,f\left(z\right)\right\}\mathrm{d}z,$$

$$\left\{{A}_{22},{A}_{23},{A}_{33}\right\}={\int }_{-h/2}^{h/2}{Q}_{66}\left\{{z}^{2},zf\left(z\right),{\left[f\left(z\right)\right]}^{2}\right\}\text{d}z,$$

$$\left\{{A}_{44},{A}_{55}\right\}={\int }_{-h/2}^{h/2}{\left[1-{f}^{{^{\prime}}}\left(z\right)\right]}^{2}\left\{{Q}_{55},{Q}_{44}\right\}\text{d}z.$$

Appendix B

The elements ${p}_{ij}={p}_{ji}$ presented in Eq. (16) are given by:

$${p}_{11}={D}_{11}{\partial }_{xx}^{2}+{A}_{11}{\partial }_{yy}^{2},$$

$${p}_{12}=\left({D}_{12}+{A}_{11}\right){\partial }_{yx}^{2},$$

$${p}_{13}=-[{D}_{13}{\partial }_{xx}^{2}+\left({D}_{14}+2{A}_{12}\right){\partial }_{yy}^{2}]{\partial }_{x},$$

$${p}_{14}=-[{D}_{15}{\partial }_{xx}^{2}+\left({D}_{16}+2{A}_{13}\right){\partial }_{yy}^{2}]{\partial }_{x},$$

$${p}_{22}={A}_{11}{\partial }_{xx}^{2}+{D}_{22}{\partial }_{yy}^{2},$$

$${p}_{23}=-[{D}_{24}{\partial }_{yy}^{2}+\left({D}_{23}+2{A}_{12}\right){\partial }_{xx}^{2}]{\partial }_{y},$$

$${p}_{24}=-[{D}_{26}{\partial }_{yy}^{2}+\left({D}_{25}+2{A}_{13}\right){\partial }_{xx}^{2}]{\partial }_{y},$$

$${p}_{33}=\left[{D}_{33}{\partial }_{xx}^{2}+2\left({D}_{34}+2{A}_{22}\right){\partial }_{yy}^{2}-{K}_{P}+{\mathcal{R}}_{11}\right]{\partial }_{xx}^{2}+\left({D}_{33}{\partial }_{yy}^{2}-{K}_{P}+{\mathcal{R}}_{22}\right){\partial }_{yy}^{2}+{K}_{W},$$

$${p}_{34}=-\left[{D}_{46}{\partial }_{yy}^{2}+\left({D}_{36}+{D}_{45}+4{A}_{23}\right){\partial }_{xx}^{2}+{K}_{P}+{\mathcal{R}}_{22}\right]{\partial }_{yy}^{2}-\left({D}_{33}{\partial }_{xx}^{2}+{K}_{P}+{\mathcal{R}}_{22}\right){\partial }_{xx}^{2}-{K}_{W},$$

$${p}_{44}=\left[{D}_{55}{\partial }_{xx}^{2}+2\left({D}_{56}+2{A}_{33}\right){\partial }_{yy}^{2}-{A}_{44}-{K}_{P}+{\mathcal{R}}_{11}\right]{\partial }_{xx}^{2}$$

$$+\left[{D}_{66}{\partial }_{yy}^{2}-{A}_{55}-{K}_{P}+{\mathcal{R}}_{22}\right]{\partial }_{yy}^{2}+{K}_{W}.$$

Appendix C

The elements ${P}_{ij}={P}_{ji}$ and ${e}_{ji}^{\Theta }$ presented in Eqs. (22) and (24) are given by:

$${P}_{11}=-{D}_{11}{\lambda }_{k}^{2}-{A}_{11}{\mu }_{l}^{2},$$

$${P}_{12}=-\left({D}_{12}+{A}_{11}\right){\lambda }_{k}{\mu }_{l},$$

$${P}_{13}=[{D}_{13}{\lambda }_{k}^{2}+\left({D}_{14}+2{A}_{12}\right){\mu }_{l}^{2}]{\lambda }_{k},$$

$${P}_{14}=[{D}_{15}{\lambda }_{k}^{2}+\left({D}_{16}+2{A}_{13}\right){\mu }_{l}^{2}]{\lambda }_{k},$$

$${P}_{22}=-{A}_{11}{\lambda }_{k}^{2}-{D}_{22}{\mu }_{l}^{2},$$

$${P}_{23}=[{D}_{24}{\mu }_{l}^{2}+\left({D}_{23}+2{A}_{12}\right){\lambda }_{k}^{2}]{\mu }_{l},$$

$${P}_{24}=[{D}_{26}{\mu }_{l}^{2}+\left({D}_{25}+2{A}_{13}\right){\lambda }_{k}^{2}]{\mu }_{l},$$

$${P}_{33}=-\left[{D}_{33}{\lambda }_{k}^{2}+2\left({D}_{34}+2{A}_{22}\right){\mu }_{l}^{2}+{K}_{P}+{\mathcal{R}}_{11}\right]{\lambda }_{k}^{2}-\left({D}_{33}{\mu }_{l}^{2}+{K}_{P}+{\mathcal{R}}_{22}\right){\mu }_{l}^{2}-{K}_{W},$$

$${P}_{34}=-\left[{D}_{46}{\mu }_{l}^{2}+\left({D}_{36}+{D}_{45}+4{A}_{23}\right){\lambda }_{k}^{2}+{K}_{P}+{\mathcal{R}}_{22}\right]{\mu }_{l}^{2}-\left({D}_{33}{\lambda }_{k}^{2}+{K}_{P}+{\mathcal{R}}_{22}\right){\lambda }_{k}^{2}-{K}_{W},$$

$${P}_{44}=-\left[{D}_{55}{\lambda }_{k}^{2}+2\left({D}_{56}+2{A}_{33}\right){\mu }_{l}^{2}+{A}_{44}+{K}_{P}+{\mathcal{R}}_{11}\right]{\lambda }_{k}^{2}-\left[{D}_{66}{\mu }_{l}^{2}+{A}_{55}+{K}_{P}+{\mathcal{R}}_{22}\right]{\mu }_{l}^{2}+{K}_{W},$$

$${e}_{1i}^{\Theta }={\int }_{-h/2}^{h/2}\left({Q}_{11}+{Q}_{12}\right){\eta }_{i}\xi \left(z\right)\mathrm{d}z,$$

$${e}_{2i}^{\Theta }={\int }_{-h/2}^{h/2}\left({Q}_{12}+{Q}_{22}\right){\eta }_{i}\xi \left(z\right)\mathrm{d}z,$$

$${e}_{3i}^{\Theta }={\int }_{-h/2}^{h/2}z\left({Q}_{11}+{Q}_{12}\right){\eta }_{i}\xi \left(z\right)\mathrm{d}z,$$

$${e}_{4i}^{\Theta }={\int }_{-h/2}^{h/2}z\left({Q}_{12}+{Q}_{22}\right){\eta }_{i}\xi \left(z\right)\mathrm{d}z,$$

$${e}_{5i}^{\Theta }={\int }_{-h/2}^{h/2}f\left(z\right)\left({Q}_{11}+{Q}_{12}\right){\eta }_{i}\xi \left(z\right)\mathrm{d}z,$$

$${e}_{6i}^{\Theta }={\int }_{-\frac{h}{2}}^\frac{h}{2}f\left(z\right)\left({Q}_{12}+{Q}_{22}\right){\eta }_{i}\xi \left(z\right)\mathrm{d}z, i=\mathrm{1,2},3,$$

$${\eta }_{1}=1, {\eta }_{2}=\frac{z}{h}, {\eta }_{3}=\frac{f\left(z\right)}{h}, \Theta =\left\{\begin{array}{c}C \mathrm{if} \xi =\beta ,\\ T \mathrm{if} \xi =\alpha .\end{array}\right.$$