Comprehensive investigation of vibration of sigmoid and power law FG nanobeams based on surface elasticity and modified couple stress theories

Abstract

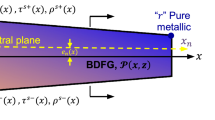
Based on the modified couple stress theory and Gurtin–Murdoch surface elasticity theory, a size-dependent Timoshenko beam model is developed for investigating the nonlinear vibration response of functionally graded (FG) micro-/nanobeams. The model is capable of capturing the simultaneous effects of microstructure couple stress, surface energy, and von Kármán’s geometric nonlinearity. Sigmoid function and power law homogenization schemes are used to model the material gradation of the beam. Hamilton’s principle is exploited to establish the nonclassical nonlinear governing equations and corresponding higher-order boundary conditions. To account for the nonhomogeneity in boundary conditions, the solution of the problem is split into two parts: the nonlinear static response with the nonhomogeneous boundary conditions and the nonlinear dynamic response. The resulting boundary conditions for the dynamic response are homogeneous, and so Galerkin’s approach is applied to reduce the set of PDEs to a nonlinear system of ODEs. The generalized differential quadrature method in terms of spatial variables is applied to obtain the static response and linear vibration mode. Considering the nonlinear system of ODEs in terms of time-related variables, both pseudo-arclength continuation and Runge–Kutta methods are used to obtain the nonlinear free vibration behavior of FG Timoshenko micro-/nanobeams with simply supported and clamped ends. Verification of the proposed model and solution procedure is performed by comparing the obtained results with those available in the open literature. The effects of the nonhomogeneous boundary conditions, surface elasticity modulus, surface residual stress, material length scale parameter, gradient index, and thickness on the characteristics of linear and nonlinear free vibrations of sigmoid function and power law FG micro-/nanobeams are discussed in detail.

Appendices

Appendix A: Coefficients of Eq. (41.1)

The coefficients $\mathbb {K}_{1}:\mathbb {K}_{5},\mathbb {P}_{1}:\mathbb {P}_{5}$, and $\mathbb {M}_{1}:\mathbb {M}_{8}$ that appear in Eq. (41.1) are defined as follows:

$$\begin{aligned} \left\{ \begin{array}{c} {{\mathbb {K}}}_{1}\\ {{\mathbb {K}}}_{2}\\ {\begin{array}{l@{\quad }l} {{\mathbb {K}}}_{3}\\ {{\mathbb {K}}}_{4}\\ {{\mathbb {K}}}_{5}\\ \end{array} }\\ \end{array} \right\}= & {} \int _0^1 \left\{ {\begin{array}{c} {\mathbb {k}}_{1}\phi _{u}\\ {\mathbb {k}}_{2}\phi _{u}^{''}\\ {\begin{array}{c} {\mathbb {k}}_{\mathrm {4}}\left( w_{s}^{'}\phi _{w}^{''}+w_{s}^{''}\phi _{w}^{'} \right) +{\mathbb {k}}_{\mathrm {5}}\phi _{w}^{'}+{\mathbb {k}}_{\mathrm {6}}\phi _{w}^{'''}\\ {\mathbb {k}}_{\mathrm {3}}\phi _{\psi }^{''}+{\mathbb {k}}_{\mathrm {7}}\phi _{\psi }\\ {\mathbb {k}}_{\mathrm {4}}\phi _{w}^{'}\phi _{w}^{''}\\ \end{array} }\\ \end{array} } \right\} \phi _{u}\hbox {d}x, \end{aligned}$$

(A.1)

$$\begin{aligned} \left\{ {\begin{array}{c} {\mathbb {P}}_{1}\\ {\mathbb {P}}_{2}\\ {\begin{array}{c} {\mathbb {P}}_{3}\\ {\mathbb {P}}_{4}\\ {\mathbb {P}}_{5}\\ \end{array} }\\ \end{array} } \right\}= & {} \int _0^1 \left\{ {\begin{array}{c} {\mathbb {P}}_{1}\phi _{\psi }\\ {\mathbb {P}}_{2}\phi _{\psi }^{''}+{\mathbb {P}}_{\mathrm {7}}\phi _{\psi }\\ {\begin{array}{c} {\mathbb {P}}_{\mathrm {3}}\phi _{u}^{''}\\ {\mathbb {P}}_{\mathrm {4}}\left( w_{s}^{'}\phi _{w}^{''}+w_{s}^{''}\phi _{w}^{'} \right) +{\mathbb {P}}_{\mathrm {5}}\phi _{w}^{'}+{\mathbb {P}}_{\mathrm {6}}\phi _{w}^{'''}\\ {\mathbb {P}}_{\mathrm {4}}\phi _{w}^{'}\phi _{w}^{''}\\ \end{array} }\\ \end{array} } \right\} \phi _{\psi }\hbox {d}x, \end{aligned}$$

(A.2)

$$\begin{aligned} \left\{ {\begin{array}{c} {\mathbb {M}}_{1}\\ {\mathbb {M}}_{2}\\ {\begin{array}{c} {\mathbb {M}}_{\mathrm {3}}\\ {\mathbb {M}}_{\mathrm {4}}\\ \end{array} }\\ \end{array} } \right\}= & {} \int _0^1 \left\{ {\begin{array}{c} {\mathbb { I}}_{w}\phi _\mathrm{w}\\ \mathbb {c}_{0}\phi _{w}^{''}+\mathbb {c}_{1}\phi _{w}^{'''}+\mathbb {c}_{\mathrm {4}}\left( u_{s}^{''}\phi _{w}^{'}+u_{s}^{'}\phi _{w}^{''} \right) +\mathbb {c}_{\mathrm {5}}\left( \psi _{s}^{''}\phi _{w}^{'}+\psi _{s}^{'}\phi _{w}^{''} \right) +\mathbb {c}_{\mathrm {6}}\left( w_{s}^{\mathrm {'2}}\phi _{w}^{''}+{2w}_{s}^{'}w_{s}^{''}\phi _{w}^{'} \right) \\ {\begin{array}{c} \mathbb {c}_{2}\phi _{\psi }^{'''}+\mathbb {c}_{\mathrm {3}}\phi _{\psi }^{'}+\mathbb {c}_{\mathrm {5}}\left( w_{s}^{''}\phi _{\psi }^{'}+w_{s}^{'}\phi _{\psi }^{''} \right) \\ \mathbb {c}_{\mathrm {4}}\left( w_{s}^{''}\phi _{u}^{'}+w_{s}^{'}\phi _{u}^{''} \right) \\ \end{array} }\\ \end{array} } \right\} \phi _{w}\hbox {d}x, \nonumber \\\end{aligned}$$

(A.3)

$$\begin{aligned} \left\{ {\begin{array}{c} {\mathbb {M}}_{\mathrm {5}}\\ {\mathbb {M}}_{\mathrm {6}}\\ {\begin{array}{c} {\mathbb {M}}_{\mathrm {7}}\\ {\mathbb {M}}_{\mathrm {8}}\\ \end{array} }\\ \end{array} } \right\}= & {} \int _0^1 \left\{ {\begin{array}{c} \mathbb {c}_{\mathrm {4}}\left( \phi _{u}^{''}\phi _{w}^{'}+\phi _{u}^{'}\phi _{w}^{''} \right) \\ \mathbb {c}_{\mathrm {5}}\left( \phi _{\psi }^{''}\phi _{w}^{'}+\phi _{\psi }^{'}\phi _{w}^{''} \right) \\ {\begin{array}{c} \mathbb {c}_{\mathrm {6}}\left( {2w}_{s}^{'}\phi _{w}^{'}\phi _{w}^{''}+w_{s}^{''}\phi _{w}^{{'2}} \right) \\ \mathbb {c}_{\mathrm {6}}\phi _{w}^{\mathrm {'2}}\phi _{w}^{''}\\ \end{array} }\\ \end{array} } \right\} \phi _{w}\hbox {d}x. \end{aligned}$$

(A.4)

Appendix B: Pseudo-arclength continuation

The pseudo-arclength continuation is used to find the steady-state periodic response of a Timoshenko nanobeam, Eq. (41), in a time period $T={2\pi } /{\varOmega }_{\mathrm {NL}}$. In order to obtain this response, we define ${\bar{\tau }}=t /T$; afterward using a spectral collocation method, the system is discretized over the time domain ${\bar{\tau }}$ into an even number of periodic grid points $(N_{t})$ given by Eq. (B.1),

$$\begin{aligned} {\bar{\tau }}=\frac{i}{N_\mathrm{t}}, 0<i<1, \quad i=1,2,\ldots , N_\mathrm{t}. \end{aligned}$$

(B.1)

Then, the discretized system will be

$$\begin{aligned}&\left( \frac{{\varOmega }_{\mathrm {NL}}}{2\pi } \right) ^{2} {{\mathbb {K}}}_{1}D_\mathrm{t}^{\left( 2 \right) }Q_{u}+{{\mathbb {K}}}_{2}Q_{u}+{{\mathbb {K}}}_{3}Q_{w}+{{\mathbb {K}}}_{4}Q_{\psi }+{{\mathbb {K}}}_{5}\left( Q_{w} \right) ^{\circ 2}=0, \end{aligned}$$

(B.2)

$$\begin{aligned}&\left( \frac{{\varOmega }_{\mathrm {NL}}}{2\pi } \right) ^{2}{\mathbb {M}}_{1}D_\mathrm{t}^{\left( 2 \right) }Q_{w}+{\mathbb {M}}_{2}Q_{w}+{\mathbb {M}}_{3}Q_{\psi }+{\mathbb {M}}_{4}Q_{u}+{\mathbb {M}}_{5}\left( Q_{u}\circ Q_{w} \right) \nonumber \\&\quad +{\mathbb {M}}_{6}\left( Q_{\psi }\circ Q_{w} \right) + {\mathbb {M}}_{7}\left( Q_{w} \right) ^{\circ 2}+ {\mathbb {M}}_{8}\left( Q_{w} \right) ^{\circ 3}=0, \end{aligned}$$

(B.3)

$$\begin{aligned}&\left( \frac{{\varOmega }_{\mathrm {NL}}}{2\pi } \right) ^{2}{\mathbb {P}}_{1}D_\mathrm{t}^{(2)}Q_{\psi }+{\mathbb {P}}_{2}Q_{\psi }+{\mathbb {P}}_{3}Q_{u}+{\mathbb {P}}_{4}Q_{w}+{\mathbb {P}}_{5}\left( Q_{w} \right) ^{\circ 2}=0 \end{aligned}$$

(B.4)

where $\left( \circ \right)$ is the Hadamard product operator, ${\varOmega }_{\mathrm {NL}}$ is the nonlinear frequency to be calculated, the column vectors $\left\{ Q_{w},Q_{u},Q_{\psi }\right\}$ are defined as:

$$\begin{aligned} {\begin{array}{lcc} Q_{u}=\left[ q_{u_{1}}, q_{u_{2}}, \ldots q_{u_{N_\mathrm{t}}} \right] ^\mathrm{T}, &{} Q_{w}=\left[ q_{w_{1}}, q_{w_{2}}, \ldots q_{w_{N_\mathrm{t}}} \right] ^\mathrm{T}, &{} Q_{\psi }=\left[ q_{\psi _{1}}, q_{\psi _{2}}, \ldots q_{\psi _{N_\mathrm{t}}} \right] ^\mathrm{T},\\ \end{array} } \end{aligned}$$

(B.5)

and $D_\mathrm{t}^{(2)}$ is spectral differentiation matrix operator [65].

Equations (B.2–B.4) can be rewritten in the form

$$\begin{aligned} {\mathcal {F}}\left( {{\varvec{Q}}},{\varOmega }_{\mathrm {NL}} \right) =0 \end{aligned}$$

(B.6)

where ${\varvec{Q}}={{[}Q_{u}{,}Q_{w}{,}Q_{\psi }{]}}_{3N_\mathrm{t}\times 1}$. In order to employ the pseudo-arclength continuation method, Eq. (B.6) is parameterized by the arclength s, such that

$$\begin{aligned} {\mathcal {F}}\left( {{\varvec{Q}}}(s),{\varOmega }_{\mathrm {NL}}(s) \right) =0. \end{aligned}$$

(B.7)

To obtain a new, fully determined system for the two-vector $\left( {{\varvec{Q}}},{\varOmega }_{\mathrm {NL}} \right)$, a restriction is added such that

$$\begin{aligned} \left\| {\dot{{{\varvec{Q}}}}} \right\| ^{2}+\left\| {\dot{\varOmega }}_{\mathrm {NL}}^{2} \right\| ^{2}=1 \end{aligned}$$

(B.8)

where ${\dot{{{\varvec{Q}}}}}=\frac{\hbox {d}Q}{\hbox {d}s}$ and $\dot{\varOmega }_{\mathrm {NL}}=\frac{\hbox {d}\varOmega }{\hbox {d}s}$. With this restriction, we obtain a new, fully determined system for the two-vector $\left( {{\varvec{Q}}},{\varOmega }_{\mathrm {NL}} \right)$, as a function of s,

$$\begin{aligned} \left\{ {\begin{array}{cc} {\mathcal {F}}_{{{\varvec{Q}}}}{\dot{{{\varvec{Q}}}}}+ {\mathcal {F}}_{{\varOmega }_{\mathrm {NL}}}{\dot{\varOmega }}_{\mathrm {NL}}=0,\\ \left\| {\dot{Q}} \right\| ^{2}+\left\| {\dot{\varOmega }}_{\mathrm {NL}}^{2} \right\| ^{2}=1.\\ \end{array} } \right. \end{aligned}$$

(B.9)

Then, Eq. (B.9) can be solved by a predictor–corrector method, where the Newton-type iterations in the corrector are typically restricted to be perpendicular to the solution curve being continued. Afterward, the frequency response curves, i.e., nonlinear frequency ${\varOmega }_{\mathrm {NL}}$ versus nonlinear amplitude $Q_{w}$, are obtained.