Skip to main content
SpringerLink
Log in

Exact solutions for the buckling and postbuckling of shear-deformable beams

  • Published:
Acta Mechanica Aims and scope Submit manuscript

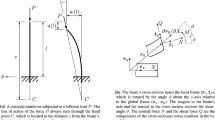
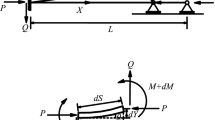
Abstract

The buckling and postbuckling of beams is revisited taking into account both the influence of axial compressibility and shear deformation. A theory based on Reissner’s geometrically exact relations for the plane deformation of beams is adopted, in which the stress resultants depend linearly on the generalized strain measures. The equilibrium equation is derived in a general form that holds for the statically determinate and indeterminate combinations of boundary conditions representing the four fundamental buckling cases. The eigenvalue problem is recovered by consistent linearization of the governing equations, the critical loads at which the trivial solution bifurcates are determined, and the influence of shear on the buckling behavior is investigated. By a series of transformations, the equilibrium equation is rearranged such that it allows a representation of the solution in terms of elliptic integrals. Additionally, closed-form relations are provided for the displacement of the axis, from which buckled shapes are eventually obtained. Even for slender beams, for which shear deformation can usually be neglected, both the buckling and the postbuckling behavior turn out to be affected by shear not only quantitatively, but also qualitatively.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Euler L.: Methodus inveniendi lineas curvas maximi minimive proprietate gaudentes, sive solutio problematis isoperimetrici lattissimo sensu accepti. Marc-Michel Bousquet et Soc, Lausanne (1744)

    Google Scholar 

  2. Truesdell, C.: The Rational Mechanics of Flexible or Elastic Bodies, 1638—1788: Introduction to Leonhardi Euleri Opera Omnia Vol. X et XI Seriei Secundae. O. Füssli (1960)

  3. Reissner E.: On one-dimensional finite-strain beam theory: the plane problem. Z. Angew. Math. Phys. 23(5), 795–804 (1972)

    Article  MATH  Google Scholar 

  4. Antman S.: The theory of rods. In: Truesdell, C. Handbuch der Physik, Vol. VIa/2, Springer, Berlin (1972)

  5. Timoshenko S.: On the transverse vibrations of bars of uniform cross-section. Philos. Mag. 43(6), 125–131 (1922)

    Google Scholar 

  6. Timoshenko S., Gere J.: Theory of Elastic Stability. McGraw-Hill, New York (1961)

    Google Scholar 

  7. Simitses G., Hodges D.: Fundamentals of Structural Stability. Butterworth-Heinemann, Oxford (2006)

    MATH  Google Scholar 

  8. Kirchhoff, G.: Über das Gleichgewicht und die Bewegung eines unendlich dünnen elastischen Stabes. J. Reine Angew. Math. 56, 285–343 (1859)

    Article  MATH  Google Scholar 

  9. Love A.: A Treatise on the Mathematical Theory of Elasticity. Dover Publications Inc., New York (1944)

    MATH  Google Scholar 

  10. Saalschütz, L.: Der belastete Stab unter Einwirkung einer seitlichen Kraft: Auf Grundlage des strengen Ausdrucks für den Krümmungsradius. Teubner (1880)

  11. Bisshopp K., Drucker D.: Large deflection of cantilever beams. Q. Appl. Math. 3(3), 272–275 (1945)

    MathSciNet  MATH  Google Scholar 

  12. Frisch-Fay R.: Flexible Bars. Butterworths, London (1962)

    MATH  Google Scholar 

  13. Stern J.: Der Gelenkstab bei großen elastischen Verformungen. Arch. Appl. Mech. 48(3), 173–184 (1979)

    MATH  Google Scholar 

  14. Stern J.: Der Gelenkstab mit Vorverformung. Arch. Appl. Mech. 54(2), 152–160 (1984)

    Google Scholar 

  15. Domokos G., Holmes P., Royce B.: Constrained Euler buckling. J. Nonlinear Sci. 7(3), 281–314 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  16. Mikata Y.: Complete solution of elastica for a clamped-hinged beam, and its applications to a carbon nanotube. Acta Mech. 190(1), 133–150 (2007)

    Article  MATH  Google Scholar 

  17. Bažant Z.: A correlation study of formulations of incremental deformation and stability of continuous bodies. J. Appl. Mech. 38(197), 9–19 (1971)

    Google Scholar 

  18. Bažant Z., Cedolin L.: Stability of Structures: Elastic, Inelastic, Fracture and Damage Theories. Oxford University Press, New York (1991)

    MATH  Google Scholar 

  19. Engesser F.: Die Knickfestigkeit gerader Stäbe. Centralblatt der Bauverwaltung 11(49), 483– (1891)

    Google Scholar 

  20. Haringx, J.: On the buckling and lateral rigidity of helical springs. I, II. Proc. Konink. Ned. Akad. Wetenschappen 45, 533–539, 650–654 (1942)

  21. Antman S., Rosenfeld G.: Global behavior of buckled states of nonlinearly elastic rods. Siam Rev. 20(3), 513–566 (1978)

    Article  MathSciNet  MATH  Google Scholar 

  22. Pflüger A.: Stabilitätsprobleme der Elastostatik. Springer, Berlin (1964)

    MATH  Google Scholar 

  23. Goto Y., Yamashita T., Matsuura S.: Elliptic integral solutions for extensional elastica with constant initial curvature. Struct. Engng./Earthquake Engng. 4(2), 299–309 (1987)

    Google Scholar 

  24. Goto Y., Yoshimitsu T., Obata M.: Elliptic integral solutions of plane elastica with axial and shear deformations. Int. J. Solids Struct. 26(4), 375–390 (1990)

    Article  MathSciNet  MATH  Google Scholar 

  25. Humer A.: Elliptic integral solution of the extensible elastica with a variable length under a concentrated force. Acta Mech. 222(3–4), 209–223 (2011)

    Article  MATH  Google Scholar 

  26. Magnusson A., Ristinmaa M., Ljung C.: Behaviour of the extensible elastica solution. Int. J. Solids Struct. 38(46–47), 8441–8457 (2001)

    Article  MATH  Google Scholar 

  27. Wang C.: Post-buckling of a clamped-simply supported elastica. Int. J. Non-Linear Mech. 32(6), 1115–1122 (1997)

    Article  MATH  Google Scholar 

  28. Vaz M., Silva D.: Post-buckling analysis of slender elastic rods subjected to terminal forces. Int. J. Non-Linear Mech. 38(4), 483–492 (2003)

    Article  MATH  Google Scholar 

  29. Mazzilli C.: Buckling and post-buckling of extensible rods revisited: a multiple-scale solution. Int. J. Non-Linear Mech. 44(2), 200–208 (2009)

    Article  MATH  Google Scholar 

  30. Nayfeh A., Mook D.: Nonlinear Oscillations. Wiley, New York (1979)

    MATH  Google Scholar 

  31. Simo J., Vu-Quoc L.: On the dynamics of flexible beams under large overall motionsthe plane case: part I. J. Appl. Mech. 53(4), 849–854 (1986)

    Article  MATH  Google Scholar 

  32. Simo J., Vu-Quoc L.: On the dynamics of flexible beams under large overall motionsthe plane case: part II. J. Appl. Mech. 53(4), 855–863 (1986)

    Article  MATH  Google Scholar 

  33. Gerstmayr J., Matikainen M., Mikkola A.: A geometrically exact beam element based on the absolute nodal coordinate formulation. Multibody Syst. Dyn. 20(4), 359–384 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  34. Nachbagauer K., Pechstein A., Irschik H., Gerstmayr J.: A new locking-free formulation for planar, shear deformable, linear and quadratic beam finite elements based on the absolute nodal coordinate formulation. Multibody Syst. Dyn. 26(3), 245–263 (2011)

    Article  MATH  Google Scholar 

  35. Irschik H., Gerstmayr J.: A continuum mechanics based derivation of Reissner’s large-displacement finite-strain beam theory: the case of plane deformations of originally straight Bernoulli-Euler beams. Acta Mech. 206(1-2), 1–21 (2009)

    Article  MATH  Google Scholar 

  36. Irschik H., Gerstmayr J.: A continuum-mechanics interpretation of Reissner’s non-linear shear-deformable beam theory. Math. Comput. Model. Dyn. Syst. 17(1), 19–29 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  37. Reissner E.: Some remarks on the problem of column buckling. Arch. Appl. Mech. 52(1-2), 115–119 (1982)

    MATH  Google Scholar 

  38. Lakes R.: Foam structures with a negative poisson’s ratio. Science 235, 1038–1040 (1987)

    Article  Google Scholar 

  39. Prall D., Lakes R.: Properties of a chiral honeycomb with a poisson’s ratio of −1. Int. J. Mech. Sci. 39(3), 305–314 (1997)

    Article  MATH  Google Scholar 

  40. Attard M., Hunt G.: Column buckling with shear deformations—a hyperelastic formulation. Int. J. Solids Struct. 45(14), 4322–4339 (2008)

    Article  MATH  Google Scholar 

  41. Abramowitz M., Stegun I.: Handbook of Mathematical Functions. Dover Publications Inc., New York (1972)

    MATH  Google Scholar 

  42. Kuznetsov V., Levyakov S.: Complete solution of the stability problem for elastica of Euler’s column. Int. J. Non-Linear Mech. 37(6), 1003–1009 (2002)

    Article  MATH  Google Scholar 

  43. Levyakov S., Kuznetsov V.: Stability analysis of planar equilibrium configurations of elastic rods subjected to end loads. Acta Mech. 211(1), 73–87 (2010)

    Article  MATH  Google Scholar 

  44. Gröbner W., Hofreiter N.: Integraltafel. 1. Teil: Unbestimmte Integrale. Springer, Wien (1975)

    MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Institute of Technical Mechanics, Johannes Kepler University Linz, Altenberger Str. 69, 4040, Linz, Austria

    Alexander Humer

Authors
  1. Alexander Humer
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Alexander Humer.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Humer, A. Exact solutions for the buckling and postbuckling of shear-deformable beams. Acta Mech 224, 1493–1525 (2013). https://doi.org/10.1007/s00707-013-0818-1

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00707-013-0818-1

Keywords

Search

Navigation