Skip to main content
SpringerLink
Log in

Central-upwind scheme for shallow water equations with discontinuous bottom topography

  • Published:
Bulletin of the Brazilian Mathematical Society, New Series Aims and scope Submit manuscript

Abstract

Finite-volume central-upwind schemes for shallow water equations were proposed in [A. Kurganov and G. Petrova, Commun. Math. Sci., 5 (2007), 133–160]. These schemes are capable of maintaining “lake-at-rest” steady states and preserving the positivity of the computed water depth. The well-balanced and positivity preserving features of the central-upwind schemes are achieved, in particular, by using continuous piecewise linear interpolation of the bottom topography function. However, when the bottom function is discontinuous or a model with a moving bottom topography is studied, the continuous piecewise linear approximationmay not be sufficiently accurate and robust.

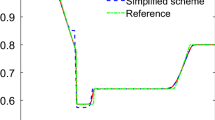
In this paper, we modify the central-upwind scheme by approximating the bottom topography function using a discontinuous piecewise linear reconstruction (the same approximation used to reconstruct evolved quantities in the finite-volume setting) as well as implementing a special quadrature for the geometric source term and draining time step technique. We prove that the new central-upwind scheme possesses the wellbalanced and positivitypreserving properties and illustrate its performance on a number of numerical examples.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. E. Audusse, F. Bouchut, M.-O. Bristeau, R. Klein and B. Perthame. A fast and stable well-balanced scheme with hydrostatic reconstruction for shallow water flows. SIAM J. Sci. Comput., 25 (2004), 2050–2065.

    Article  MathSciNet  MATH  Google Scholar 

  2. C. Berthon and F. Marche. A positive preserving high order VFRoe scheme for shallow water equations: a class of relaxation schemes. SIAM J. Sci. Comput., 30(5) (2008), 2587–2612.

    Article  MathSciNet  MATH  Google Scholar 

  3. A. Bollermann, G. Chen, A. Kurganov and S. Noelle. A well-balanced reconstruction ofwet/dry fronts for the shallowwater equations. J. Sci. Comput., 56(2) (2013), 267–290.

    Article  MathSciNet  MATH  Google Scholar 

  4. A. Bollermann, S. Noelle and M. Lukácová-Medvidová. Finite volume evolution Galerkin methods for the shallow water equations with dry beds. Commun. Comput. Phys., 10(2) (2011), 371–404.

    MathSciNet  MATH  Google Scholar 

  5. A. Chertock, S. Cui, A. Kurganov and T. Wu. Well-balanced positivity preserving centralupwind scheme for the shallow water system with friction terms. Internat. J. Numer. Meth. Fluids, Submitted (1871).

    Google Scholar 

  6. A.J.C. de Saint-Venant. Thèorie du mouvement non-permanent des eaux, avec application aux crues des rivière at à l’introduction des marées dans leur lit. C.R. Acad. Sci. Paris, 73 (1871), 147–154.

    MATH  Google Scholar 

  7. S. Gottlieb, D. Ketcheson and C.-W. Shu. Strong stability preserving Runge-Kutta andmultistep time discretizations. World Scientific Publishing Co. Pte. Ltd., Hackensack, NJ (2011).

    Book  MATH  Google Scholar 

  8. S. Gottlieb, C.-W. Shu and E. Tadmor. Strong stability-preserving high-order time discretization methods. SIAM Rev., 43 (2001), 89–112.

    Article  MathSciNet  MATH  Google Scholar 

  9. A. Kurganov. Well-balanced central-upwind scheme for compressible two-phase flows. Proceedings of the European Conference on ComputationalFluidDynamics ECCOMAS CFD (2006).

    Google Scholar 

  10. A. Kurganov and C.-T. Lin. On the reduction of numerical dissipation in centralupwind schemes. Commun. Comput. Phys., 2 (2007), 141–163.

    MathSciNet  MATH  Google Scholar 

  11. A. Kurganov, S. Noelle and G. Petrova. Semi-discrete central-upwind scheme for hyperbolic conservation laws and Hamilton-Jacobi equations. SIAM J. Sci. Comput., 23 (2001), 707–740.

    Article  MathSciNet  MATH  Google Scholar 

  12. A. Kurganov and G. Petrova. A second-order well-balanced positivity preserving centralupwind scheme for the saint-venant system. Commun. Math. Sci., 5 (2007), 133–160.

    Article  MathSciNet  MATH  Google Scholar 

  13. A. Kurganov and E. Tadmor. New high resolution central schemes for nonlinear conservation laws and convection-diffusion equations. J. Comput. Phys., 160 (2000), 241–282.

    Article  MathSciNet  MATH  Google Scholar 

  14. P.G. LeFloch and M.D. Thanh. A Godunov-type method for the shallow water equations with discontinuous topography in the resonant regime. Journal of Computational Physics, 230 (2011), 7631–7660.

    Article  MathSciNet  MATH  Google Scholar 

  15. K.-A. Lie and S. Noelle. On the artificial compression method for second-order nonoscillatory central difference schemes for systems of conservation laws. SIAM J. Sci. Comput., 24(4) (2003), 1157–1174.

    Article  MathSciNet  MATH  Google Scholar 

  16. H. Nessyahu and E. Tadmor. Nonoscillatory central differencing for hyperbolic conservation laws. J. Comput. Phys., 87(2) (1990), 408–463.

    Article  MathSciNet  MATH  Google Scholar 

  17. B. Perthame and C. Simeoni. A kinetic scheme for the Saint-Venant system with a source term. Calcolo, 38(4) (2001), 201–231.

    Article  MathSciNet  MATH  Google Scholar 

  18. P.K. Sweby. High resolution schemes using flux limiters for hyperbolic conservation laws. SIAM J. Numer. Anal., 21(5) (1984), 995–1011.

    Article  MathSciNet  MATH  Google Scholar 

  19. B. van Leer. Towards the ultimate conservative difference scheme. V. A secondorder sequel to Godunov’s method. J. Comput. Phys., 32(1) (1979), 101–136.

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, North Carolina State University, Raleigh, NC, 27695, USA

    Andrew Bernstein & Alina Chertock

  2. Mathematics Department, Tulane University, New Orleans, LA, 70118, USA

    Alexander Kurganov

Authors
  1. Andrew Bernstein
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Alina Chertock
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Alexander Kurganov
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Alexander Kurganov.

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Bernstein, A., Chertock, A. & Kurganov, A. Central-upwind scheme for shallow water equations with discontinuous bottom topography. Bull Braz Math Soc, New Series 47, 91–103 (2016). https://doi.org/10.1007/s00574-016-0124-3

Download citation

  • Received:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00574-016-0124-3

Keywords

Mathematical subject classification

Search

Navigation