How Daphnia copes with excess carbon in its food

Abstract

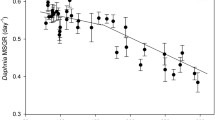
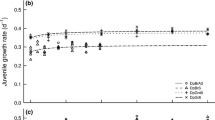
Animals that maintain near homeostatic elemental ratios may get rid of excess ingested elements from their food in different ways. C regulation was studied in juveniles of Daphnia magna feeding on two Selenastrum capricornutum cultures contrasting in P content (400 and 80 C:P atomic ratios). Both cultures were labelled with ¹⁴C in order to measure Daphnia ingestion and assimilation rates. No significant difference in ingestion rates was observed between P-low and P-rich food, whereas the net assimilation of ¹⁴C was higher in the treatment with P-rich algae. Some Daphnia were also homogeneously labelled over 5 days on radioactive algae to estimate respiration rates and excretion rates of dissolved organic C (DOC). The respiration rate for Daphnia fed with high C:P algae (38.7% of body C day^-1) was significantly higher than for those feeding on low C:P algae (25.3% of body C day^-1). The DOC excretion rate was also higher when animals were fed on P-low algae (13.4% of body C day^-1) than on P-rich algae (5.7% of body C day^-1) . When corrected for respiratory losses, total assimilation of C did not differ significantly between treatments (around 60% of body C day^-1). Judging from these experiments, D. magna can maintain its stoichiometric balance when feeding on unbalanced diets (high C:P) primarily by disposing of excess dietary C via respiration and excretion of DOC.

Appendices

Appendix A

Demonstration of equations modelling evolution over time of total radioactivity in homogeneously labelled Daphnia feeding on unlabelled algae

The kinetics of the tracer in each pool is described by a system of two differential equations (see Table 1 for explanation of symbols):

$$ \left\{ {\matrix{ {{{d\left( m \right)} \over {dt}} = {{\rho _{21} } \over S}s - {{\rho _{12} } \over M}m - {{\rho _{10} } \over M}m} \hfill \cr {{{d\left( s \right)} \over {dt}} = {{\rho _{12} } \over M}m - {{\rho _{21} } \over S}s} \hfill \cr } } \right. $$

We know that this kind of differential system has a solution of the type:

$$ \left\{ {\matrix{ {m = a\exp \left( {\lambda _j t} \right)} \hfill \cr {s = b\exp \left( {\lambda _j t} \right)} \hfill \cr } } \right. $$

(8)

In using this type of solution in the system, we found:

$$ \left\{ {\matrix{ {\lambda _j a\exp \left( {\lambda _j t} \right) = \left[ { - \left( {{{\rho _{12} + \rho _{10} } \over M}} \right)\;a + {{\rho _{21} } \over S}b} \right]\exp \left( {\lambda _j t} \right)} \hfill \cr {\lambda _j b\exp \left( {\lambda _j t} \right) = \left( {{{\rho _{12} } \over M}a - {{\rho _{21} } \over S}b} \right)\exp \left( {\lambda _j t} \right)} \hfill \cr } } \right. $$

In simplifying this by exp(λ _j t), we found algebraic equations for a and b:

$$ \left\{ {\matrix{ { - \left( {\lambda _j + {{\rho _{12} + \rho _{10} } \over M}} \right)\;a + {{\rho _{21} } \over S}b = 0} \hfill \cr {{{\rho _{12} } \over M}a - \left( {\lambda _j + {{\rho _{21} } \over S}} \right)\;b = 0} \hfill \cr } } \right. $$

(9)

This system will give a non-trivial solution only if the next determinant

$$ \left| {\matrix{ { - \left( {\lambda _j + {{\rho _{12} + \rho _{10} } \over M}} \right)} & {{{\rho _{21} } \over S}} \cr {{{\rho _{12} } \over M}} & { - \left( {\lambda _j + {{\rho _{21} } \over S}} \right)} \cr } } \right| = \lambda _j^2 + \left( {{{\rho _{12} } \mathord{\left/ {\vphantom {{\rho _{12} } M}} \right. \kern-\nulldelimiterspace} M} + {{\rho _{21} } \mathord{\left/ {\vphantom {{\rho _{21} } S}} \right. \kern-\nulldelimiterspace} S} + {{\rho _{10} } \mathord{\left/ {\vphantom {{\rho _{10} } M}} \right. \kern-\nulldelimiterspace} M}} \right)\;\lambda _j + {{\rho _{10} \rho _{21} } \mathord{\left/ {\vphantom {{\rho _{10} \rho _{21} } {MS}}} \right. \kern-\nulldelimiterspace} {MS}} = 0 $$

is equal to 0. This gives a quadratic equation in λ _j with two solutions:

$$ \lambda _1 = - {1 \over 2}\left( {{{\rho _{12} } \mathord{\left/ {\vphantom {{\rho _{12} } M}} \right. \kern-\nulldelimiterspace} M} + {{\rho _{21} } \mathord{\left/ {\vphantom {{\rho _{21} } S}} \right. \kern-\nulldelimiterspace} S} + {{\rho _{10} } \mathord{\left/ {\vphantom {{\rho _{10} } M}} \right. \kern-\nulldelimiterspace} M}} \right) + {1 \over 2}\sqrt {\left( {{{\rho _{12} } \mathord{\left/ {\vphantom {{\rho _{12} } M}} \right. \kern-\nulldelimiterspace} M} + {{\rho _{21} } \mathord{\left/ {\vphantom {{\rho _{21} } S}} \right. \kern-\nulldelimiterspace} S} + {{\rho _{10} } \mathord{\left/ {\vphantom {{\rho _{10} } M}} \right. \kern-\nulldelimiterspace} M}} \right)^2 - {{4\rho _{10} \rho _{21} } \mathord{\left/ {\vphantom {{4\rho _{10} \rho _{21} } {MS}}} \right. \kern-\nulldelimiterspace} {MS}}} $$

(10)

and

$$ \lambda _2 = - {1 \over 2}\left( {{{\rho _{12} } \mathord{\left/ {\vphantom {{\rho _{12} } M}} \right. \kern-\nulldelimiterspace} M} + {{\rho _{21} } \mathord{\left/ {\vphantom {{\rho _{21} } S}} \right. \kern-\nulldelimiterspace} S} + {{\rho _{10} } \mathord{\left/ {\vphantom {{\rho _{10} } M}} \right. \kern-\nulldelimiterspace} M}} \right) - {1 \over 2}\sqrt {\left( {{{\rho _{12} } \mathord{\left/ {\vphantom {{\rho _{12} } M}} \right. \kern-\nulldelimiterspace} M} + {{\rho _{21} } \mathord{\left/ {\vphantom {{\rho _{21} } S}} \right. \kern-\nulldelimiterspace} S} + {{\rho _{10} } \mathord{\left/ {\vphantom {{\rho _{10} } M}} \right. \kern-\nulldelimiterspace} M}} \right)^2 - {{4\rho _{10} \rho _{21} } \mathord{\left/ {\vphantom {{4\rho _{10} \rho _{21} } {MS}}} \right. \kern-\nulldelimiterspace} {MS}}} $$

(11)

We then rewrite the equations in system 8 (Eq. 8) under their decomposed form:

$$ \left\{ \matrix{ m = a_1 \exp \left( {\lambda _1 t} \right) + a_2 \exp \left( {\lambda _2 t} \right) \hfill \cr s = b_1 \exp \left( {\lambda _1 t} \right) + b_2 \exp \left( {\lambda _2 t} \right) \hfill \cr} \right. $$

(12)

We then rewrite the second equation in system 9 (Eq. 9) with decomposition of λ _j into λ ₁ and λ ₂:

$$ {{\rho _{12} } \over M}a_1 - \left( {\lambda _1 + {{\rho _{21} } \over S}} \right)\;b_1 = 0 \Leftrightarrow b_1 = {{{{\rho _{12} a_1 } \mathord{\left/ {\vphantom {{\rho _{12} a_1 } M}} \right. \kern-\nulldelimiterspace} M}} \over {\lambda _1 + {{\rho _{21} } \mathord{\left/ {\vphantom {{\rho _{21} } S}} \right. \kern-\nulldelimiterspace} S}}} $$

and

$$ {{\rho _{12} } \over M}a_2 - \left( {\lambda _2 + {{\rho _{21} } \over S}} \right)\;b_2 = 0 \Leftrightarrow b_2 = {{{{\rho _{12} a_2 } \mathord{\left/ {\vphantom {{\rho _{12} a_2 } M}} \right. \kern-\nulldelimiterspace} M}} \over {\lambda _2 + {{\rho _{21} } \mathord{\left/ {\vphantom {{\rho _{21} } S}} \right. \kern-\nulldelimiterspace} S}}} $$

If we insert these two equations into system 12 (Eq. 12), we obtain a new system of two linear equations with two variables a ₁ and a ₂:

$$ \eqalign{ & \left\{ \matrix{ m = a_1 \exp \left( {\lambda _1 t} \right) + a_2 \exp \left( {\lambda _2 t} \right) \hfill \cr s = {{{{\rho _{12} a_1 } \mathord{\left/ {\vphantom {{\rho _{12} a_1 } M}} \right. \kern-\nulldelimiterspace} M}} \over {\lambda _1 + {{\rho _{21} } \mathord{\left/ {\vphantom {{\rho _{21} } S}} \right. \kern-\nulldelimiterspace} S}}}\exp \left( {\lambda _1 t} \right) + {{{{\rho _{12} a_2 } \mathord{\left/ {\vphantom {{\rho _{12} a_2 } M}} \right. \kern-\nulldelimiterspace} M}} \over {\lambda _2 + {{\rho _{21} } \mathord{\left/ {\vphantom {{\rho _{21} } S}} \right. \kern-\nulldelimiterspace} S}}}\exp \left( {\lambda _2 t} \right) \hfill \cr} \right. \cr & \Rightarrow m + s = a_1 \exp \left( {\lambda _1 t} \right)\;\left( {1 + {{{{\rho _{12} } \mathord{\left/ {\vphantom {{\rho _{12} } M}} \right. \kern-\nulldelimiterspace} M}} \over {\lambda _1 + {{\rho _{21} } \mathord{\left/ {\vphantom {{\rho _{21} } S}} \right. \kern-\nulldelimiterspace} S}}}} \right) + a_2 \exp \left( {\lambda _2 t} \right)\;\left( {1 + {{{{\rho _{12} } \mathord{\left/ {\vphantom {{\rho _{12} } M}} \right. \kern-\nulldelimiterspace} M}} \over {\lambda _2 + {{\rho _{21} } \mathord{\left/ {\vphantom {{\rho _{21} } S}} \right. \kern-\nulldelimiterspace} S}}}} \right) \cr} $$

(13)

Under initial conditions, system 13 (Eq. 13) becomes:

$$ \left\{ \matrix{ m_0 = a_1 + a_2 \Leftrightarrow a_1 = m_0 - a_2 \hfill \cr s_0 = {{{{\rho _{12} a_1 } \mathord{\left/ {\vphantom {{\rho _{12} a_1 } M}} \right. \kern-\nulldelimiterspace} M}} \over {\lambda _1 + {{\rho _{21} } \mathord{\left/ {\vphantom {{\rho _{21} } S}} \right. \kern-\nulldelimiterspace} S}}} + {{{{\rho _{12} a_2 } \mathord{\left/ {\vphantom {{\rho _{12} a_2 } M}} \right. \kern-\nulldelimiterspace} M}} \over {\lambda _2 + {{\rho _{21} } \mathord{\left/ {\vphantom {{\rho _{21} } S}} \right. \kern-\nulldelimiterspace} S}}} \hfill \cr} \right. $$

Thus,

$$ \matrix{ {s_0 = {{\rho _{12} } \over M}\left( {{{m_0 - a_2 } \over {\lambda _1 + {{\rho _{21} } \mathord{\left/ {\vphantom {{\rho _{21} } S}} \right. \kern-\nulldelimiterspace} S}}} + {{a_2 } \over {\lambda _2 + {{\rho _{21} } \mathord{\left/ {\vphantom {{\rho _{21} } S}} \right. \kern-\nulldelimiterspace} S}}}} \right)} \hfill \cr { \Leftrightarrow a_2 = \left[ {{{\lambda _1 + {{\rho _{21} } \mathord{\left/ {\vphantom {{\rho _{21} } S}} \right. \kern-\nulldelimiterspace} S}} \over {{{\rho _{12} } \mathord{\left/ {\vphantom {{\rho _{12} } M}} \right. \kern-\nulldelimiterspace} M}}}s_0 - m_0 } \right]{{\lambda _2 + {{\rho _{21} } \mathord{\left/ {\vphantom {{\rho _{21} } S}} \right. \kern-\nulldelimiterspace} S}} \over {\lambda _1 - \lambda _2 }}} \hfill \cr } $$

Appendix B

Demonstration of equations modelling evolution over time of radioactivity in unlabelled Daphnia feeding on labelled algae

The kinetics of the tracer in each pool is described by (see Table 1 for explanation of symbols):

$$ \left\{ {\matrix{ {{{d\left( m \right)} \over {dt}}} \hfill & = \hfill & {{{\rho _{21} } \over S}s - {{\rho _{12} } \over M}m - {{\rho _{10} } \over M}m + {{\rho _{01} } \over {100}}W\;SA} \hfill \cr {{{d\left( s \right)} \over {dt}}} \hfill & = \hfill & {{{\rho _{12} } \over M}m - {{\rho _{21} } \over S}s} \hfill \cr } } \right. $$

(14)

Let us define

$$ m_t = o_t + \alpha $$

(15)

and

$$ s_t = u_t + \beta $$

(16)

that we insert in the system of differential equations:

$$ \left\{ {\matrix{ {{{d\left( o \right)} \over {dt}}} \hfill & = \hfill & { - {{\left( {\rho _{12} + \rho _{10} } \right)} \over M}o + {{\rho _{21} } \over S}u - {{\left( {\rho _{12} + \rho _{10} } \right)} \over M}\alpha + {{\rho _{21} } \over S}\beta + {{\rho _{01} } \over {100}}W\;SA} \hfill \cr {{{d\left( u \right)} \over {dt}}} \hfill & = \hfill & {{{\rho _{12} } \over M}o - {{\rho _{21} } \over S}u + {{\rho _{12} } \over M}\alpha - {{\rho _{21} } \over S}\beta } \hfill \cr } } \right. $$

(17)

We can now determine α et β which nullify the non-homogeneous terms:

$$ \eqalign{ & \left\{ {\matrix{ {{{\left( {\rho _{12} + \rho _{10} } \right)} \over M}\alpha - {{\rho _{21} } \over S}\beta = {{\rho _{01} } \over {100}}W\;SA} \hfill \cr {{{\rho _{12} } \over M}\alpha - {{\rho _{21} } \over S}\beta = 0} \hfill \cr } } \right. \cr & \Leftrightarrow \left\{ {\matrix{ {\alpha = {{\rho _{01} } \over {\rho _{10} 100}}W\;M\;SA} \hfill \cr {\beta = {{\rho _{12} \rho _{01} } \over {\rho _{10} \rho _{21} 100}}W\;S\;SA} \hfill \cr } } \right. \cr} $$

Both equations of system 17 (Eq. 17) become homogeneous:

$$ \left\{ \matrix{ {{d\left( o \right)} \over {dt}} = - {{\left( {\rho _{12} + \rho _{10} } \right)} \over M}o + {{\rho _{21} } \over S}u \hfill \cr {{d\left( u \right)} \over {dt}} = {{\rho _{12} } \over M}o - {{\rho _{21} } \over S}u \hfill \cr} \right. $$

As for the problem defined in Appendix A, we know that the solution of this linear differential system is a linear combination:

$$ \left\{ \matrix{ o_t = a_1 \exp \left( {\lambda _1 t} \right) + a_2 \exp \left( {\lambda _2 t} \right) \hfill \cr u_t = b_1 \exp \left( {\lambda _1 t} \right) + b_2 \exp \left( {\lambda _2 t} \right) \hfill \cr} \right. $$

(18)

where, as in Appendix A, b ₁ and b ₂ are defined, respectively, by $b_1 = {{{{\rho _{12} a_1 } \mathord{\left/ {\vphantom {{\rho _{12} a_1 } M}} \right. \kern-\nulldelimiterspace} M}} \over {\lambda _1 + {{\rho _{21} } \mathord{\left/ {\vphantom {{\rho _{21} } S}} \right. \kern-\nulldelimiterspace} S}}}$, and $b_2 = {{{{\rho _{12} a_2 } \mathord{\left/ {\vphantom {{\rho _{12} a_2 } M}} \right. \kern-\nulldelimiterspace} M}} \over {\lambda _2 + {{\rho _{21} } \mathord{\left/ {\vphantom {{\rho _{21} } S}} \right. \kern-\nulldelimiterspace} S}}}$, and λ ₁ and λ ₂, respectively, by Eqs. 10 and 11.

Under initial conditions, m ₀=0 and s ₀=0.

The insertion of Eqs. 15 and 16 into system 18 (Eq. 18) gives:

$$ \left\{ \matrix{ - \alpha = a_1 + a_2 \Leftrightarrow a_1 = - \left( {a_2 + \alpha } \right) \hfill \cr - \beta = b_1 + b_2 \hfill \cr} \right. $$

Thus,

$$ {\matrix{ {{ - \beta = {{\rho _{{12}} } \over M}{\left[ {{{ - {\left( {a_{2} + \alpha } \right)}} \over {\lambda _{1} + {\rho _{{21}} } \mathord{\left/ {\vphantom {{\rho _{{21}} } S}} \right. {\kern-\nulldelimiterspace} S}}} + {{a_{2} } \over {\lambda _{2} + {\rho _{{21}} } \mathord{\left/ {\vphantom {{\rho _{{21}} } S}} \right. {\kern-\nulldelimiterspace} S}}}} \right]}} \hfill} \cr {{ \Leftrightarrow a_{2} = {\left( {\alpha - {{\lambda _{1} + {\rho _{{21}} } \mathord{\left/ {\vphantom {{\rho _{{21}} } S}} \right. {\kern-\nulldelimiterspace} S}} \over {{\rho _{{12}} } \mathord{\left/ {\vphantom {{\rho _{{12}} } M}} \right. {\kern-\nulldelimiterspace} M}}}\beta } \right)}{{\lambda _{2} + {\rho _{{21}} } \mathord{\left/ {\vphantom {{\rho _{{21}} } S}} \right. {\kern-\nulldelimiterspace} S}} \over {\lambda _{1} - \lambda _{2} }}} \hfill} \cr } } $$