Plane strain gradient elastic rectangle in bending

Abstract

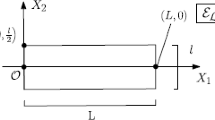
The present paper can be considered as an extension of the work (Charalambopoulos and Polyzos in Arch Appl Mech 85:1421–1438, 2015). The simplest possible elastostatic version of Mindlin’s strain gradient elastic (SGE) theory is employed for the solution of a SGE rectangle in bending under plane strain conditions. The equilibrium equations as well as expressions for all types of stresses and boundary conditions appearing in the considered rectangle are explicitly provided. An improved version of Mindlin’s solution procedure via potentials is proposed. Besides, an elegant solution representation that contains the solution of the corresponding classical elastic problem is demonstrated. Results of six plane strain bending problems, which reveal a significant diversification from the classical elasticity theory and specific features of the underlying microstructure, are addressed and discussed.

Appendix A

This appendix deals with the proof of Theorem 1 stated in Section 4 of the present work.

We consider the vector Helmholtz decomposition of the displacement vector, i.e.,

$$\begin{aligned} \mathbf{u}=\nabla \varphi +\nabla \times \mathbf{A},\quad \nabla \cdot \mathbf{A}=0 \end{aligned}$$

(A.1)

Inserting (A.1) into (24), one obtains

$$\begin{aligned} \left( {1-g^{2}\nabla ^{2}} \right) \nabla ^{2}\left[ {(\lambda +2\mu )\nabla \varphi +\mu \nabla \times \mathbf{A}} \right] +\mathbf{F}=\mathbf{0} \end{aligned}$$

(A.2)

Defining as

$$\begin{aligned} \mathbf{B}=\frac{\lambda +2\mu }{\mu }\nabla \varphi +\nabla \times \mathbf{A} \end{aligned}$$

(A.3)

Equation (A.2) is simplified to

$$\begin{aligned} \mu \left( {1-g^{2}\nabla ^{2}} \right) \nabla ^{2}{} \mathbf{B}=-\mathbf{F} \end{aligned}$$

(A.4)

From (A.3) and (A.4), we obtain the relations

$$\begin{aligned}&\nabla \cdot \mathbf{B}=\frac{\lambda +2\mu }{\mu }\nabla ^{2}\varphi \end{aligned}$$

(A.5)

$$\begin{aligned}&(\lambda +2\mu )\left( {1-g^{2}\nabla ^{2}} \right) \nabla ^{2}\nabla ^{2}\varphi =-\nabla \cdot \mathbf{F} \end{aligned}$$

(A.6)

In the sequel, taking the inner product of Eq. (A.4) with the position vector $\mathbf{r}$, utilizing the identity $\nabla ^{2}\left( {\mathbf{r}\cdot \mathbf{a}} \right) =\mathbf{r}\cdot \nabla ^{2}{} \mathbf{a}+2\nabla \cdot \mathbf{a}$ and making use of Eq. (A.5), one yields

$$\begin{aligned}&\mu \nabla ^{2}\left[ {\mathbf{r}\cdot \left( {1-g^{2}\nabla ^{2}} \right) \mathbf{B}} \right] -2(\lambda +2\mu )\nabla ^{2}\phi \nonumber \\&\quad +2g^{2}(\lambda +2\mu )\nabla ^{2}\nabla ^{2}\varphi =-\mathbf{r}\cdot \mathbf{F} \end{aligned}$$

(A.7)

Applying the operator $\left( {1-g^{2}\nabla ^{2}} \right)$ on Eq. (A.7) and taking into account Eq. (A.6), Eq. (A.7) is transformed to

$$\begin{aligned} \begin{array}{l} \mu \left( {1-g^{2}\nabla ^{2}} \right) \nabla ^{2}\left[ {\mathbf{r}\cdot \left( {1-g^{2}\nabla ^{2}} \right) \mathbf{B}-2\frac{\lambda +2\mu }{\mu }\varphi } \right] \\ =-\left( {1-g^{2}\nabla ^{2}} \right) \left( {\mathbf{r}\cdot \mathbf{F}} \right) +2g^{2}\nabla \cdot \mathbf{F} \\ \end{array} \end{aligned}$$

(A.8)

Making use of the identity

$$\begin{aligned} \left( {1-g^{2}\nabla ^{2}} \right) \left( {\mathbf{r}\cdot \mathbf{F}} \right) =\mathbf{r}\cdot \left( {1-g^{2}\nabla ^{2}} \right) \mathbf{F}-2g^{2}\nabla \cdot \mathbf{F} \end{aligned}$$

(A.9)

Eq. (A.8) reads

$$\begin{aligned} \mu \left( {1-g^{2}\nabla ^{2}} \right) \nabla ^{2}B_0 =\mathbf{r}\cdot \left( {1-g^{2}\nabla ^{2}} \right) \mathbf{F}-4g^{2}\nabla \cdot \mathbf{F} \end{aligned}$$

(A.10)

with

$$\begin{aligned} B_0 =-\mathbf{r}\cdot \left( {1-g^{2}\nabla ^{2}} \right) \mathbf{B}+2\frac{\lambda +2\mu }{\mu }\varphi \end{aligned}$$

(A.11)

Finally, starting from Eq. (A.1) we have the following algebra

$$\begin{aligned} \mathbf{u}&=\nabla \varphi +\nabla \times \mathbf{A}\mathop =\limits ^{Eq.(B.3)} \nabla \varphi +\mathbf{B}-\frac{\lambda +2\mu }{\mu }\nabla \varphi \nonumber \\&=\mathbf{B}-\frac{\lambda +\mu }{\mu }\nabla \varphi \nonumber \\&\mathop =\limits ^{Eq.(B.11)} \mathbf{B}-\frac{\lambda +\mu }{\mu }\nabla \frac{\mu }{2(\lambda +2\mu )}[\mathbf{r}\cdot (1-g^{2}\nabla ^{2})\mathbf{B}+B_0 ] \nonumber \\&=\mathbf{B}-\frac{\lambda +\mu }{2(\lambda +2\mu )}\nabla [\mathbf{r}\cdot (1-g^{2}\nabla ^{2})\mathbf{B}+B_0 ] \end{aligned}$$

(A.12)

Equation (A.12) in conjunction with (A.4) and (A.10) readily shows the validity of the present theorem 1.