An Asymptotic Analysis of a 2-D Model of Dynamically Active Compartments Coupled by Bulk Diffusion

Abstract

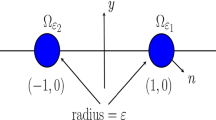
A class of coupled cell–bulk ODE–PDE models is formulated and analyzed in a two-dimensional domain, which is relevant to studying quorum-sensing behavior on thin substrates. In this model, spatially segregated dynamically active signaling cells of a common small radius $\epsilon \ll 1$ are coupled through a passive bulk diffusion field. For this coupled system, the method of matched asymptotic expansions is used to construct steady-state solutions and to formulate a spectral problem that characterizes the linear stability properties of the steady-state solutions, with the aim of predicting whether temporal oscillations can be triggered by the cell–bulk coupling. Phase diagrams in parameter space where such collective oscillations can occur, as obtained from our linear stability analysis, are illustrated for two specific choices of the intracellular kinetics. In the limit of very large bulk diffusion, it is shown that solutions to the ODE–PDE cell–bulk system can be approximated by a finite-dimensional dynamical system. This limiting system is studied both analytically, using a linear stability analysis and, globally, using numerical bifurcation software. For one illustrative example of the theory, it is shown that when the number of cells exceeds some critical number, i.e., when a quorum is attained, the passive bulk diffusion field can trigger oscillations through a Hopf bifurcation that would otherwise not occur without the coupling. Moreover, for two specific models for the intracellular dynamics, we show that there are rather wide regions in parameter space where these triggered oscillations are synchronous in nature. Unless the bulk diffusivity is asymptotically large, it is shown that a diffusion-sensing behavior is possible whereby more clustered spatial configurations of cells inside the domain lead to larger regions in parameter space where synchronous collective oscillations between the small cells can occur. Finally, the linear stability analysis for these cell–bulk models is shown to be qualitatively rather similar to the linear stability analysis of localized spot patterns for activator–inhibitor reaction–diffusion systems in the limit of long-range inhibition and short-range activation.

Appendix: Non-Dimensionalization of the Coupled Cell–Bulk System

In this appendix we non-dimensionalize (1.1) into the dimensionless form (1.2). If we let $\left[ \gamma \right]$ denote the dimensions of the variable $\gamma$, then the dimensions of the various quantities in (1.1) are as follows:

$$\begin{aligned} \begin{aligned} \left[ {\mathcal {U}}\right]&= \frac{\text{ moles }}{\text{(length) }^2} \,, \qquad \left[ \varvec{\mu }_j\right] = \text{ moles } \,, \qquad \left[ \mu _c \right] = \text{ moles } \,, \qquad \left[ D_B \right] =\frac{\text{(length) }^2}{\text{ time }} \,, \qquad \\ \left[ k_B \right]&=\left[ k_R\right] = \frac{1}{\text{ time }} \,, \qquad \left[ \beta _1 \right] =\frac{\text{ length }}{\text{ time }} \,, \qquad \ \left[ \beta _2 \right] =\frac{1}{\text{ length }\times \text{ time }} \,. \end{aligned} \end{aligned}$$

(8.1)

We now non-dimensionalize (1.1) by introducing the dimensionless variables t, $\varvec{x}$, U, $\varvec{u}$, and D, defined by

$$\begin{aligned} t = k_R T \,, \qquad \varvec{x} = {\varvec{X}/L} \,, \qquad U= \frac{L^2}{\mu _c} {\mathcal {U}} \,, \qquad \varvec{u}_j = \frac{\varvec{\mu }_j}{\mu _c} \,, \qquad D \equiv \frac{D_B}{k_B L^2} \,, \end{aligned}$$

(8.2)

where L is a typical radius of $\Omega$. In terms of these variables, (1.1) becomes

$$\begin{aligned} \begin{aligned} \frac{k_R}{k_B} { U}_t&= D \Delta _{\varvec{x}} {U} - {U}\,, \qquad \varvec{x} \in \tilde{\Omega }\backslash \cup _{j=1}^m\Omega _{\epsilon _j}\,; \qquad \partial _{n_{\varvec{x}}} {U}= 0\,,\qquad \varvec{x} \in \partial \tilde{\Omega }\,,\\ D \partial _{n_{\varvec{x}}} {U}&= \frac{\beta _1}{k_B L} { U} - \frac{\beta _2 L}{k_B} u_j^1 \,, \;\;\quad \varvec{x}\in \partial \Omega _{\epsilon _j}\,, \\ \end{aligned} \end{aligned}$$

(8.3a)

which is coupled to the intracellular dynamics for each $j=1,\ldots ,m$ by

$$\begin{aligned} \frac{\hbox {d} \varvec{u}_j}{\hbox {d}t} = \varvec{F}_j\left( \varvec{u}_j\right) + \frac{k_B\varvec{e}_1}{k_R} \int _{\partial \Omega _{\epsilon _j}} \left( \frac{\beta _1}{k_B L} {U} - \frac{\beta _2 L}{k_B} u_j^1\right) \, dS_{\varvec{x}} \,. \end{aligned}$$

(8.3b)

For each j, $\Omega _{\epsilon _j}$ is a disk centered at some $\varvec{x}_j$ of a common radius ${\sigma /L}$. In our non-dimensionalization the timescale is chosen based on the timescale of the reaction kinetics, and D is an effective dimensionless diffusivity. In this way, and upon dropping the tilde variables, we obtain the dimensionless problem (1.2) with dimensionless parameters as in (1.3). We remark that, upon using the divergence theorem, we can readily establish from (8.3) that

$$\begin{aligned} \frac{\hbox {d}}{\hbox {d}t} \left( \int _{\tilde{\Omega }\backslash \cup _{j=1}^m\Omega _{\epsilon _j}} U \, \hbox {d}\varvec{x} + \sum _{j=1}^{m} \varvec{e}^T \varvec{u}_j \right) = -\frac{k_B}{k_R}\int _{\tilde{\Omega }\backslash \cup _{j=1}^m\Omega _{\epsilon _j}} U \, \hbox {d}\varvec{x} + \sum _{j=1}^{m} \varvec{e}^T \varvec{F}_j(\varvec{u}_j) \,, \end{aligned}$$

(8.4)

where $\varvec{e} \equiv (1,\ldots ,1)^T$. The left-hand side of this expression is the total amount of material inside the cells and in the bulk, while the right-hand side characterizes the bulk degradation and production within the cells.