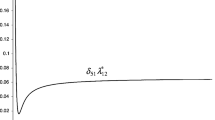
Abstract
We investigate the nonlinear stability of the icosahedral relative equilibrium configuration of point vortices on a sphere. The relative equilibrium problem is formulated as a problem of finding the nullspace of the configuration matrix that encodes the geometry of the icosahedron, as in Jamaloodeen and Newton (Proc. Royal Soc. A, Math. Phys. Eng. Sci. 462(2075):3277, 2006). The seven-dimensional nullspace of the configuration matrix, A, associated with the icosahedral geometry gives rise to a basis set of vortex strengths for which the icosahedron stays in relative formation, and we use these values to form the augmented Hamiltonian governing the stability. We choose the basis set made up of (i) one element with equal strength vortices on every vertex of the icosahedron (the uniform icosahedron); (ii) six elements made up of equal and opposite antipodal pairs. We start by proving nonlinear stability of the antipodal vortex pair (by direct methods). Following the methods laid out in Simo et al. (Arch. Ration. Mech. Anal. 115(1):15–59, 1991) and Pekarsky and Marsden (J. Math. Phys. 39(11):5894–5907, 1998) and more generally in Marsden and Ratiu (Introduction to Mechanics and Symmetry, 1999), we then combine our knowledge of the nullspace structure of A with the structure of the underlying Hamiltonian, and analyze the stability of the icosahedron using the energy-momentum method. Because the parameter space is large, we focus on the physically motivated and important case obtained by combining the basis elements into (i) the uniform icosahedron; (ii) a von Kármán vortex street configuration of equal and opposite staggered, evenly spaced latitudinal rows equidistant from the equator (Chamoun et al. in Phys. Fluids 21:116603, 2009), and (iii) the North Pole–South Pole equal and opposite vortex pair. Stability boundaries in a three-parameter space are calculated for linear combinations of these grouped basis configurations.






Similar content being viewed by others
Notes
A symmetry induced basis is a basis of the invariant subspaces of the dihedral group D 5 (symmetry group of regular 5-gon). As such, it is the biggest symmetry subgroup of the configuration under investigation; i.e., the configuration will not change if we do cyclic rotations by an angle \(\frac{2\pi}{5}\) about the z-axis or flip about any plane which goes through the z-axis and any vertex.
References
Aref, H., Newton, P.K., Stremler, M.A., Tokieda, T., Vainchtein, D.L.: Vortex crystals. Adv. Appl. Mech. 39, 1–79 (2003)
Boatto, S., Cabral, H.E.: Non-linear stability of a latitudinal ring of point vortices on a non-rotating sphere. SIAM J. Appl. Math. 64(1), 216–230 (2003)
Bogomolov, V.A.: Dynamics of the vorticity on a sphere. Izv. Akad. Nauk SSSR Mekh. Zhidk. Gaza, pp. 57–65 (1977)
Borisov, A.V., Kilin, A.A.: Stability of Thomson’s configurations of vortices on a sphere. Regul. Chaotic Dyn. 5, 189–200 (2000)
Cabral, H.E., Schmidt, D.S.: Stability of relative equilibria in the problem of N+1 vortices. SIAM J. Math. Anal. 31(2), 231–250 (1999)
Cabral, H.E., Meyer, K.R., Schmidt, D.S.: Stability and bifurcations for the N+1 vortex problem on the sphere. Regul. Chaotic Dyn. 8(3), 259–282 (2003)
Chamoun, G., Kanso, E., Newton, P.K.: Von Kármán vortex streets on a sphere. Phys. Fluids 21, 116603 (2009)
Crowdy, D.G.: Stuart vortices on a sphere. J. Fluid Mech. 498, 381–402 (2004)
Hally, D.: Stability of streets of vortices on surfaces of revolution with a reflection symmetry. J. Math. Phys. 21, 211 (1980)
Humphreys, T., Marcus, P.S.: Vortex street dynamics: the selection mechanism for the areas and locations of Jupiter’s vortices. J. Atmos. Sci. 64, 1318–1333 (2008)
Jamaloodeen, M.I., Newton, P.K.: The N-vortex problem on a rotating sphere. II. Heterogeneous Platonic solid equilibria. Proc. R. Soc. A, Math. Phys. Eng. Sci. 462(2075), 3277 (2006)
Kidambi, R., Newton, P.K.: Motion of three point vortices on a sphere. Physica D 116, 143–175 (1998)
Kidambi, R., Newton, P.K.: Point vortex motion on a sphere with solid boundaries. Phys. Fluids 12(3), 581–588 (2000)
Kimura, Y., Okamoto, H.: Vortex motion on a sphere. J. Phys. Soc. Jpn. 56, 4203–4206 (1987)
Kurakin, L.G.: On nonlinear stability of the regular vortex systems on a sphere. Chaos 14(3), 592–602 (2004)
Laurent-Polz, F.: Point vortices on the sphere: a case with opposite vortices. Nonlinearity 15, 143–171 (2002)
Lewis, D., Ratiu, T.: Rotating n-gon/kn-gon vortex configurations. J. Nonlinear Sci. 6, 385–414 (1996)
Lim, C.C., Montaldi, J., Roberts, M.R.: Relative equilibria of point vortices on a sphere. Physica D 148, 97–135 (2001)
Lim, C.C., Ding, X., Nebus, J.: Vorticity Dynamics, Statistical Mechanics, and Planetary Atmospheres. World Scientific, Singapore (2009)
Marsden, J.E., Ratiu, T.S.: Introduction to Mechanics and Symmetry, 2nd edn. Texts in Applied Mathematics, vol. 17. Springer, New York (1999)
Meyer, C.D.: Matrix Analysis and Applied Linear Algebra. Society for Industrial Mathematics, Philadelphia (2000)
Newton, P.K.: The N-Vortex Problem. Analytical Techniques. Applied Mathematical Sciences, vol. 145. Springer, New York (2001)
Newton, P.K.: The N-vortex problem on a sphere: geophysical mechanisms that break integrability. Theor. Comput. Fluid Dyn. (2009). doi:10.1007/s00162-009-0109-6
Newton, P.K., Sakajo, T.: Point vortex equilibria on the sphere via Brownian ratchets. Proc. R. Soc. A 465(2102), 437 (2009)
Newton, P.K., Sakajo, T.: Point vortex equilibria and optimal packings of circles on a sphere. Proc. R. Soc. A 467(2102), 1468–1490 (2011)
Patrick, G.: Relative equilibria in Hamiltonian systems: the dynamic interpretation of nonlinear stability on a reduced phase space. J. Geom. Phys. 9, 111–119 (1992)
Pekarsky, S., Marsden, J.E.: Point vortices on a sphere: stability of relative equilibria. J. Math. Phys. 39(11), 5894–5907 (1998)
Polvani, L.M., Dritschel, D.G.: Wave and vortex dynamics on the surface of a sphere. J. Fluid Mech. 255, 35–64 (1993)
Sakajo, T.: Transition of global dynamics of a polygonal vortex ring on a sphere with pole vortices. Physica D 196, 243–264 (2004)
Serre, J.P.: Linear Representations of Finite Groups. Springer, New York (1977)
Simo, J.C., Lewis, D., Marsden, J.E.: Stability of relative equilibria. Part I: the reduced energy-momentum method. Arch. Ration. Mech. Anal. 115(1), 15–59 (1991)
Surana, A., Crowdy, D.G.: Vortex dynamics in a complex domain on a spherical surface. J. Comput. Phys. 227, 6058–6070 (2008)
Zandi, R., Reguera, D., Bruinsma, R.F., Gelbart, W.M., Rudnick, J.: Origin of icosahedral symmetry in viruses. Proc. Natl. Acad. Sci. USA 101, 15556–15560 (2004)
Acknowledgements
We dedicate this paper to the memory of Jerry Marsden, whose work in Hamiltonian mechanics and stability theory laid the groundwork for much that is described in this paper. Support from the National Science Foundation, grant NSF-DMS-0804629, is greatly appreciated.
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by Anthony Bloch.
Appendix
Appendix
Matrix C can be written as
where C i ,i=1,…,10 are 2×2 matrices:

Matrix D can be written as
where D i ,i=1,…,15 are 2×2 matrices:

Rights and permissions
About this article
Cite this article
Newton, P.K., Ostrovskyi, V. Energy-Momentum Stability of Icosahedral Configurations of Point Vortices on a Sphere. J Nonlinear Sci 22, 499–515 (2012). https://doi.org/10.1007/s00332-012-9142-5
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00332-012-9142-5