An analytical study of PPP-RTK corrections: precision, correlation and user-impact

Abstract

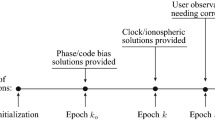
PPP-RTK extends the PPP concept by providing single-receiver users, next to orbits and clocks, also information about the satellite phase and code biases, thus enabling single-receiver ambiguity resolution. It is the goal of the present contribution to provide an analytical study of the quality of the PPP-RTK corrections as well as of their impact on the user ambiguity resolution performance. We consider the geometry-free and the geometry-based network derived corrections, as well as the impact of network ambiguity resolution on these corrections. Next to the insight that is provided by the analytical solutions, the closed form expressions of the variance matrices also demonstrate how the corrections depend on network parameters such as number of epochs, number of stations, number of satellites, and number of frequencies. As a result we are able to describe in a qualitative sense how the user ambiguity resolution performance is driven by the data from the different network scenarios.

Appendix

Proof of Theorem 1

To prove (51), we apply the least-squares conditional adjustment (Teunissen 2000) to the single-station correction ‘${\mathrm{I}}$’. Given the GF ambiguity-float network redundancy (Table 3), the following uncorrelated sets of misclosures are formed

$$\begin{aligned}&t = (I_{n-1}\otimes E^-\otimes D_m^T)\,[p_{12}^T(\bar{i}),\ldots ,p_{1n}^T(\bar{i})]^T, \nonumber \\&t_l = \left( I_{k-1}\otimes \left[ \begin{array}{cc}{\varLambda }^{-1}, &{} M\\ 0, &{}E^-\end{array}\right] \otimes \, D_m^T\right) \, \left[ \begin{array}{cc} \left[ \begin{array}{c}\phi _{l}(12)\\ p_{l}(12)\end{array}\right] ^T,\ldots , \left[ \begin{array}{c}\phi _{l}(1k)\\ p_{l}(1k)\end{array}\right] ^T \end{array}\right] ^T\nonumber \\ \end{aligned}$$

(99)

$l=1,\ldots ,n$. The first set of misclosures t is due to the fact that all single-station solutions of the estimable code biases $\tilde{d}^{ps}$ have the same mean. The n sets of misclosures $t_l$ are due to the fact that all single-station solutions of the estimable ambiguities $\tilde{a}_l^{ps}$ and code biases $\tilde{d}^{ps}$ are assumed constant over k epochs. According to the least-squares conditional adjustment, the GF ambiguity-float network correction (51) is obtained as

$$\begin{aligned} \left[ \begin{array}{c} \hat{\tilde{c}}_{\phi , \scriptscriptstyle \mathrm{GF}}(i) \\ \hat{\tilde{c}}_{p, \scriptscriptstyle \mathrm{GF}}(i) \end{array}\right]= & {} {\mathrm{I}}-Q_{{\mathrm{I}},t}Q_{tt}^{-1}t-\sum \limits _{l=1}^n Q_{{\mathrm{I}},t_l}Q_{t_lt_l}^{-1}t_l \end{aligned}$$

(100)

This, together with the following equalities

$$\begin{aligned} Q_{{\mathrm{I}},t}Q_{tt}^{-1}t = \hat{{\mathrm{I}\!\mathrm{I}\!\mathrm{I}}},\quad \text {and}\quad Q_{{\mathrm{I}},t_l}Q_{t_lt_l}^{-1}t_l=\left\{ \begin{array}{l@{\quad }l} \hat{{\mathrm{I}\!\mathrm{I}}}, &{} l=r\\ 0, &{} l\ne r \end{array}, \right. \end{aligned}$$

(101)

completes the proof. $\square$

Proof of Theorem 2

The proof goes along the same lines as the proof of Theorem 1. The GF ambiguity-fixed network correction (57) is obtained through replacing the role of the misclosure vector t in (100) by its higher-dimension counterpart (cf. Table 3)

$$\begin{aligned} \tilde{t}= & {} \left( I_{n-1}\otimes \left[ \begin{array}{c@{\quad }c}{\varLambda }^{-1}, &{} M\\ 0, &{}E^-\end{array}\right] \otimes D_m^T\right) \nonumber \\&\times \left[ \begin{array}{cc} \left[ \begin{array}{c}\tilde{\phi }_{12}(\bar{i})\\ p_{12}(\bar{i})\end{array}\right] ^T,\ldots , \left[ \begin{array}{c}\tilde{\phi }_{1n}(\bar{i})\\ p_{1n}(\bar{i})\end{array}\right] ^T \end{array}\right] ^T, \end{aligned}$$

(102)

together with the equality

$$\begin{aligned} Q_{{\mathrm{I}},\tilde{t}}\,Q_{\tilde{t}\tilde{t}}^{-1}\tilde{t} = \check{{\mathrm{I}\!\mathrm{I}}}-\hat{{\mathrm{I}\!\mathrm{I}}} \end{aligned}$$

(103)

$\square$

Proof of Theorem 3

We apply the least-squares conditional adjustment to the GF ambiguity-float network correction (51). Given the extra redundancy by the geometry-based network model (Table 6), the following sets of misclosures are formed

$$\begin{aligned}&t_{g} = (I_{n-1}\otimes (D_m^TG)^{\bot T})\,[\hat{\tilde{\rho }}_{12}^T(\bar{i}),\ldots ,\hat{\tilde{\rho }}_{1n}^T(\bar{i})]^T, \nonumber \\&t_{g_{1l}} = (I_{k-1}\otimes D_m^T)\,[\hat{\tilde{\rho }}_{1l}^T(12),\ldots ,\hat{\tilde{\rho }}_{1l}^T(1k)]^T \end{aligned}$$

(104)

$l=2,\ldots ,n$. The first set of misclosures $t_g$ is due to the ‘geometry-parametrization’ of (72). The $(n-1)$ sets of misclosures $t_{g_{1l}}$ are due to the fact that the relative position increments and ZTDs $({\varDelta } x_l - {\varDelta } x_1)$ are assumed constant over k epochs. According to the least-squares conditional adjustment, the GB ambiguity-float network correction (75) is obtained as

$$\begin{aligned} \begin{array}{lcl} \left[ \begin{array}{c} \hat{\tilde{c}}_{\phi , \scriptscriptstyle \mathrm{GB}}(i) \\ \hat{\tilde{c}}_{p, \scriptscriptstyle \mathrm{GB}}(i) \end{array}\right]= & {} y-Q_{y,t_g}Q_{t_gt_g}^{-1}t_g-Q_{y,t_{g_L}}Q_{t_{g_L}t_{g_L}}^{-1}t_{g_L} \end{array}\end{aligned}$$

(105)

with $y=[\hat{\tilde{c}}_{\phi , \scriptscriptstyle \mathrm{GF}}^T (i),\hat{\tilde{c}}_{p, \scriptscriptstyle \mathrm{GF}}^T (i)]^T$ and $t_{g_L}=[t_{g_{12}}^T,\ldots ,t_{g_{1n}}^T]^T$. Equation (75) follows then by substituting

$$\begin{aligned} Q_{y,t_g}Q_{t_gt_g}^{-1}t_g = \hat{{\mathrm{V}}},\quad \text {and}\quad Q_{y,t_{g_L}}Q_{t_{g_L}t_{g_L}}^{-1}t_{g_L} = \hat{{\mathrm{I}\!\mathrm{V}}} \end{aligned}$$

(106)

into (105). $\square$

Proof of Theorem 4

We apply the least-squares conditional adjustment to the GF ambiguity-fixed network correction (57), on the basis of the extra geometry-based misclosures given in (104). The GB ambiguity-fixed network correction (81) follows then through replacing the role of y in (105) by $\tilde{y}=[\check{\tilde{c}}_{\phi , \scriptscriptstyle \mathrm{GF}}^T (i),\check{\tilde{c}}_{p, \scriptscriptstyle \mathrm{GF}}^T (i)]^T$, together with the equalities

$$\begin{aligned} Q_{\tilde{y},t_g}Q_{t_gt_g}^{-1}t_g = \check{{\mathrm{V}}},\quad \text {and}\quad Q_{\tilde{y},t_{g_L}}Q_{t_{g_L}t_{g_L}}^{-1}t_{g_L} = \hat{{\mathrm{I}\!\mathrm{V}}} \end{aligned}$$

(107)

$\square$