Skip to main content
SpringerLink
Log in

Modeling Large-deformation-induced Microflow in Soft Biological Tissues

  • Original Article
  • Published:
Theoretical and Computational Fluid Dynamics Aims and scope Submit manuscript

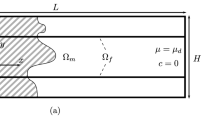
Abstract

The homogenization approach to multiscale modeling of soft biological tissues is presented. The homogenized model describes the relationship between the macroscopic hereditary creep behavior and the microflow in a fluid-saturated dual-porous medium at the microscopic level. The micromodel is based on Biot’s system for quasistatic deformation processes, modified for the updated Lagrangian formulation to account for coupling the fluid diffusion through a porous solid undergoing large deformation. Its microstructure is constituted by fluid-filled inclusions embedded in the porous matrix. The tangential stiffness coefficients and the retardation stress for the macromodel are derived for a time-stepping algorithm. Numerical examples are discussed, showing the strong potential of the model for simulations of deformation-driven physiological processes at the microscopic scale.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Allaire G. (1992). Homogenization and two-scale convergence. SIAM J. Math. Anal. 23:1482–1518

    Article  MATH  MathSciNet  Google Scholar 

  2. Baek S., Srinivasa A.S. (2004). Diffusion of a fluid through an elastic solid undergoing large deformation. Nonlin. Mech. 39:201–218

    Article  Google Scholar 

  3. de Boer R. (2000). Theory of Porous Media. Springer, Berlin Heidelberg New York

    MATH  Google Scholar 

  4. Bourgeat A., Chechkin G.A., Piatnitski L. (2003). Singular double porosity model. Applic. Anal. 82:103–116

    Article  MATH  MathSciNet  Google Scholar 

  5. Brezzi F., Fortin M. (1991). Mixed and Hybrid Finite Element Methods. Springer, Berlin Heidelberg New York

    MATH  Google Scholar 

  6. Campen D.H., Huyghe J.M., Bovendeerd P.H.M., Arts T. (1994). Biomechanics of the heart muscle. Eur. J. Mech. A/Solids 13:19–41

    MATH  Google Scholar 

  7. Cimrman R., Rohan E. (2003). Modeling heart tissue using a composite muscle model with blood perfusion. In: Bathe K.J. (eds) Computational Fluid and Solid Mechanics 2003. Elsevier, Amsterdam, pp 1642–1646

    Google Scholar 

  8. Cioranescu D., Donato P. (1999). An Introduction to Homogenization. Oxford Lecture Series in Mathematics and its Applications 17. Oxford University Press, Oxford

    MATH  Google Scholar 

  9. Cioranescu D., Saint Jean Paulin J. (1999). Homogenization of Reticulated Structures. Springer, Berlin Heidelberg New York Applied Mathematical Sciences, vol. 136

    MATH  Google Scholar 

  10. Clopeau Th., Ferrín J.L., Gilbert R.P., Mikelić A. (2001). Homogenizing the acoustic properties of the seabed, part II. Math. Comput. Modell. 33:821–841

    Article  MATH  Google Scholar 

  11. Frijns, A.J.H.: A four-component theory applied to cartilaginous tissues: numerical modelling and experiments. Ph.D. Thesis, Eindhoven University of Technology (2000)

  12. Frijns A.J.H., Huyghe J.M., Janssen J.D. (1997). A validation of the quadriphasic mixture theory for intervertebral disc tissue. Int. J. Eng. Sci. 35:1419–1429

    Article  MATH  Google Scholar 

  13. Hiroshi M., Yoshitaka H., Hayashi K. (2000). A newly designed tensile tester for cells and its application to fibroblast. J. Biomech. 33:97–104

    Article  Google Scholar 

  14. Holecek, M., Moravec, F.: Hyperelastic model of a material whose microstructure is formed by “balls and springs”. Accepted by Int. J. Solids Struct. (2006)

  15. Holzapfel G.A. (2000). Nonlinear Solid Mechanics. Wiley, Chichester

    MATH  Google Scholar 

  16. Hornung U. (1997). Homogenization and Porous Media. Springer, Berlin Heidelberg New York

    MATH  Google Scholar 

  17. Huyghe J.M., van Campen D.H. (1995). Finite deformation theory of hierarchically arranged porous solids I, II. Int. J. Eng. Sci. 33:1861–1886

    Article  MATH  Google Scholar 

  18. Huyghe J.M., Janssen J.D. (1997). Quadriphasic mechanics of swelling incompressible porous media. Int. J. Eng. Sci. 35:793–802

    Article  MATH  Google Scholar 

  19. Ingber D.E. (2002). Mechanical signaling and the cellular response to extracellular matrix in angiogenesis and cardiovascular physiology. Circ. Res. 91:877–887

    Article  Google Scholar 

  20. Keener J., Sneyd J. (1998). Mathematical Physiology. Interdisciplinary Applied Mathematics 8. Springer, Berlin Heidelberg New York

    MATH  Google Scholar 

  21. Kouznetsova V., Geers M.G.D., Breklemans W.A.M. (2002). Multi-scale constitutive modelling of heterogeneous materials with a gradient-enhanced computational homogenization scheme. Int. J. Numer. Methods Eng. 54:1235–1260

    Article  MATH  Google Scholar 

  22. Murad M.A., Cushman J.H. (1996). Multiscale flow and deformation in hydrophilic swelling media. Int. J. Eng. Sci. 34:13–338

    Article  MathSciNet  Google Scholar 

  23. Murad A.M., Guerreiro J.N., Loula A.F.D. (2001). Micromechanical computational modelling of secondary consolidation and hereditary creep in soils. Comput. Methods Appl. Mech. Eng. 190:1985–2016

    Article  MATH  MathSciNet  Google Scholar 

  24. Rohan E. (2002a). Mathematical modelling of soft tissues. Habilitation Thesis, University of West Bohemia, Plzeň

    Google Scholar 

  25. Rohan, E.: Homogenization of highly deformed media. In: Proceedings of Modelován a měření v mechanice kontinua, Konstitutivní vztahy. VTS Škoda výzkum, Pilsen, Nečtiny (2002b)

  26. Rohan, E.: A two scale updated Lagrangian algorithm for large deformation problems in heterogeneous media. In: Plešek J, Praha (ed.) Proceedings of Euromech Colloquium 430: Formulations and constitutive laws for very large strains. Institute of Termomechanics, 199–201 (2002c)

  27. Rohan E. (2003a). Sensitivity strategies in modelling heterogeneous media undergoing finite deformation. Math. Comput. Simul. 61:261–270

    Article  MathSciNet  Google Scholar 

  28. Rohan, E.: On homogenization of double diffusion problem in deforming porous media. In: Proceedings of the 19th Conference Computational Mechanics. pp. 389–396, Nečtiny (2003b)

  29. Rohan, E., Cimrman, R.: Homogenization based modelling of microstructural interactions in soft tissues. In: Proceedings of WCCM 2002, electronic publication: http://wccm.tuwien.ac.at (2002)

  30. Rohan, E., Cimrman, R.: Numerical modelling and homogenization of large deforming porous media. In: Proceedings of the 7th International Conference on Computational Structures Technology (2004)

  31. Rohan E., Cimrman R., Lukeš V. (2006). Numerical modelling and homogenized constitutive law of large deforming fluid saturated heterogeneous solids. Comput. Struct. 84:1095–1114

    Article  Google Scholar 

  32. Sanchez–Palencia E. (1978). Non-homogeneous media and vibration theory. Lecture Notes in Physics 127. Springer, Berlin Heidelberg New York

    Google Scholar 

  33. Showalter R.E. (2000). Diffusion in poro-elastic media. J. Math. Anal. Appl. 251:310–340

    Article  MATH  MathSciNet  Google Scholar 

  34. Showalter R.E., Momken B. (2002). Single-phase flow in composite poro-elastic media. Math. Methods Appl. Sci. 25:115–139

    Article  MATH  MathSciNet  Google Scholar 

  35. Showalter, R.E., Visarraga, D.B.: Double-diffusion models from a highly heterogeneous medium. http://www.ticam.utexas.edu/reports/2002.html (2002)

  36. Simon B.R., Liable J.P., Pflaster D., Yuan Y., Krag M.H. (1996). A poroelastic finite element formulation including transport and swelling in soft tissue structures. J. Biomech. Eng. 118:1–9

    MATH  Google Scholar 

  37. Terada K., Kikuchi N. (2001). A class of general algorithm for multi-scale analyses of heterogeneous media. Comput. Methods Appl. Mech. Eng. 190:5427–5464

    Article  MATH  MathSciNet  Google Scholar 

  38. Terada K., Ito T., Kikuchi N. (1998). Characterization of the mechanical behaviors of solid-fluid mixture by the homogenization method. Comput. Methods Appl. Mech. Eng. 153:223–257

    Article  MATH  MathSciNet  Google Scholar 

  39. Terada K., Hori M., Kyoya T., Kikuchi N. (2000). Simulation of the multi-scale convergence in computational homogenization approaches. Int. J. Solids Struct. 37:2285–2311

    Article  MATH  Google Scholar 

  40. Tada S., Tarbell J.M. (2000). Interstitial flow through the internal elastic lamina affects shear stress on arterial smooth muscle cells. Am. J. Physiol. Heart Circ. Physiol. 278:H1589–H1597

    Google Scholar 

  41. Takano N., Ohnishi Y., Zako M., Nishiyabu K. (2000). The formulation of homogenization method applied to large deformation problem for composite materials. Int. J. Solids Struct. 37:6517–6535

    Article  MATH  MathSciNet  Google Scholar 

  42. Takano N., Zako M., Okazaki T., Terada K. (2002). Microstructure-based evaluation of the influence of woven architecture on permeability by asymptotic homogenization theory. Compos. Sci. Technol. 62:1347–1356

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mechanics and New Technologies Research Centre, University of West Bohemia, Univerzitní 22, 306 14, Plzeň, Czech Republic

    Eduard Rohan

Authors
  1. Eduard Rohan
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Eduard Rohan.

Additional information

Communicated by O.E. Jensen and J. Malek

Rights and permissions

Reprints and permissions

About this article

Cite this article

Rohan, E. Modeling Large-deformation-induced Microflow in Soft Biological Tissues. Theor. Comput. Fluid Dyn. 20, 251–276 (2006). https://doi.org/10.1007/s00162-006-0020-3

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00162-006-0020-3

Keywords

Search

Navigation