Skip to main content
SpringerLink
Log in

A geometrically exact Cosserat shell-model including size effects, avoiding degeneracy in the thin shell limit. Part I: Formal dimensional reduction for elastic plates and existence of minimizers for positive Cosserat couple modulus

  • Original article
  • Published:
Continuum Mechanics and Thermodynamics Aims and scope Submit manuscript

Abstract.

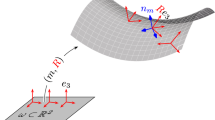
This contribution is concerned with a consistent formal dimensional reduction of a previously introduced finite-strain three-dimensional Cosserat micropolar elasticity model to the two-dimensional situation of thin plates and shells. Contrary to the direct modelling of a shell as a Cosserat surface with additional directors, we obtain the shell model from the Cosserat bulk model which already includes a triad of rigid directors. The reduction is achieved by assumed kinematics, quadratic through the thickness. The three-dimensional transverse boundary conditions can be evaluated analytically in terms of the assumed kinematics and determines exactly two appearing coefficients in the chosen ansatz. Further simplifications with subsequent analytical integration through the thickness determine the reduced model in a variational setting. The resulting membrane energy turns out to be a quadratic, elliptic, first order, non degenerate energy in contrast to classical approaches. The bending contribution is augmented by a curvature term representing an additional stiffness of the Cosserat model and the corresponding system of balance equations remains of second order. The lateral boundary conditions for simple support are non-standard. The model includes size-effects, transverse shear resistance, drilling degrees of freedom and accounts implicitly for thickness extension and asymmetric shift of the midsurface. The formal thin shell “membrane” limit without classical h 3-bending term is non-degenerate due to the additional Cosserat curvature stiffness and control of drill rotations. In our formulation, the drill-rotations are strictly related to the size-effects of the bulk model and not introduced artificially for numerical convenience. Upon linearization with zero Cosserat couple modulus \(\mu_c = 0\) we recover the well known infinitesimal-displacement Reissner-Mindlin model without size-effects and without drill-rotations. It is shown that the dimensionally reduced Cosserat formulation is well-posed for positive Cosserat couple modulus \(\mu_c > 0\) by means of the direct methods of variations along the same line of argument which showed the well-posedness of the three-dimensional Cosserat bulk model [72].

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Antman, S.: Nonlinear Problems of Elasticity, volume 107 of Applied Mathematical Sciences. Springer, Berlin 1995

  2. Babuska, I., Li, L.: The problem of plate modelling: theoretical and computational results. Comp. Meth. Appl. Mech. Engrg. 100, 249-273 (1992)

    Article  MATH  Google Scholar 

  3. Badur, J., Pietraszkiewicz, W.: On geometrically non-linear theory of elastic shells derived from pseudo-cosserat continuum with constrained micro-rotations. In: Pietraszkiewicz, W. (ed.) Finite Rotations in Structural Mechanics, no. 19, pp. 19-32. Springer, 1985

  4. Ball, J.M.: Constitutive inequalities and existence theorems in nonlinear elastostatics. In: Knops, R.J. (ed.) Herriot Watt Symposion: Nonlinear Analysis and Mechanics., vol. 1, pp. 187-238. Pitman, London, 1977

  5. Basar, Y.: A consistent theory of geometrically non-linear shells with independent rotation vector. Int. J. Solids Struct. 23, 1401-1415 (1987)

    MATH  Google Scholar 

  6. Basar, Y., Weichert, D.: A finite rotation theory for elastic-plastic shells under consideration of shear deformations. Z. Ang. Math. Mech. 71, 379-389 (1991)

    MathSciNet  MATH  Google Scholar 

  7. Betsch, P., Gruttmann, F., Stein, E.: A 4-node finite shell element for the implementation of general hyperelastic 3d-elasticity at finite strains. Comp. Meth. Appl. Mech. Engrg. 130, 57-79

  8. Betsch, P., Stein, E.: Numerical implementation of multiplicative elasto-plasticity into assumed strain elements with applications to shells at large strains. Comp. Meth. Appl. Mech. Engrg. 179, 215-245 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  9. Bhattacharya, K., James, R.D.: A theory of thin films of martensitic materials with applications to microactuators. J. Mech. Phys. Solids 47, 531-576 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  10. Bischoff, M., Ramm, E.: Shear deformable shell elements for large strains and rotations. Int. J. Num. Meth. Engrg. 40, 4427-4449 (1997)

    Article  MATH  Google Scholar 

  11. Bischoff, M., Ramm, E.: On the physical significance of higher order kinematic and static variables in a three-dimensional shell formulation. Int. J. Solids Struct. 37, 6933-6960 (2000)

    Article  MATH  Google Scholar 

  12. Braess, D.: Finite Elemente. Springer, Heidelberg, 1992

  13. Braun, M., Bischoff, M., Ramm, E.: Nonlinear shell formulations for complete three-dimensional constitutive laws including composites and laminates. Comp. Mech. 15, 1-18 (1994)

    MATH  Google Scholar 

  14. Buechter, N., Ramm, E.: Shell theory versus degeneration-a comparison in large rotation finite element analysis. Int. J. Num. Meth. Engrg. 34, 39-59 (1992)

    MATH  Google Scholar 

  15. Chernykh, K.: Nonlinear theory of isotropically elastic thin shells. Mechanics of Solids, Transl. of Mekh. Tverdogo Tela 15(2), 118-127 (1980)

    Google Scholar 

  16. Ciarlet, P.G., Lods, V.: Asymptotic analysis of linearly elastic shells. I. Justification of membrane shell equations. Arch. Rat. Mech. Anal. 136, 119-161 (1996)

    MATH  Google Scholar 

  17. Ciarlet, P.G., Lods, V.: Asymptotic analysis of linearly elastic shells. III. Justification of Koiter’s shell equations. Arch. Rat. Mech. Anal. 136, 191-200 (1996)

    MATH  Google Scholar 

  18. Ciarlet, P.G., Lods, V., Miara, B.: Asymptotic analysis of linearly elastic shells. II. Justification of flexural shell equations. Arch. Rat. Mech. Anal. 136, 163-1190 (1996)

    MATH  Google Scholar 

  19. Ciarlet, P.G.: Mathematical Elasticity, Vol II: Theory of Plates. North-Holland, Amsterdam, 1st edn., 1997

  20. Ciarlet, P.G.: Introduction to Linear Shell Theory. Series in Applied Mathematics. Gauthier-Villars, Paris, 1st edn., 1998

  21. Ciarlet, P.G.: Mathematical Elasticity, Vol III: Theory of Shells. North-Holland, Amsterdam, 1st edn., 1999

  22. Cirak, F., Cisternas, J.E., Cuitino, A.M., Ertl, G., Holmes, P., Kevrekidis, I.G., Ortiz, M., Rotermund, H.H., Schunack, M., Wolff, J.: Oscillatory thermomechanical instability of an ultrathin catalyst. Science 300, 1932-1936 (2003)

    Article  Google Scholar 

  23. Cirak, F., Ortiz, M.: Fully C 1-conforming subdivision elements for finite deformation thin-shell analysis. Int. J. Numer. Meth. Engrg. 51(7), 813-833 (2001)

    Article  MATH  Google Scholar 

  24. Cirak, F., Ortiz, M., Schroeder, P.: Subdivision surfaces: a new paradigm for thin-shell finite element analysis. Int. J. Num. Meth. Engrg. 47, 2039-2072 (2000)

    Article  MATH  Google Scholar 

  25. Cohen, H., DeSilva, C.N.: Nonlinear theory of elastic directed surfaces. J. Mathematical Phys. 7, 960-966 (1966)

    MATH  Google Scholar 

  26. Cohen, H., DeSilva, C.N.: Nonlinear theory of elastic surfaces. J. Mathematical Phys. 7, 246-253 (1966)

    MATH  Google Scholar 

  27. Cohen H., Wang, C.C.: A mathematical analysis of the simplest direct models for rods and shells. Arch. Rat. Mech. Anal. 108, 35-81 (1989)

    MathSciNet  MATH  Google Scholar 

  28. Cosserat E., Cosserat, F.: Théorie des corps déformables. Librairie Scientifique A. Hermann et Fils, Paris, 1909

  29. Dacorogna, B.: Direct Methods in the Calculus of Variations, volume 78 of Applied Mathematical Sciences. Springer, Berlin, 1st edn., 1989

  30. Destuynder, P., Salaun, M.: Mathematical Analysis of Thin Plate Models. Springer, Berlin 1996

  31. Ben Dhia, H.: Analyse mathematique de models de plaques non lineaires de type Mindlin-Naghdi-Reissner. Existence de solutions et convergence sous des hypotheses optimales. C. R. Acad. Sci. Paris, Ser. I 320, 1545-1552 (1995)

    Google Scholar 

  32. Dikmen, M.: Theory of Thin Elastic Shells. Pitman, London 1982

  33. Le Dret, H., Raoult, A.: From three-dimensional elasticity to nonlinear membranes. In: Ciarlet, P.G., Trabucho, L., Viano, J.M. (eds.) Asymptotique Methods for Elastic Structures, Proceedings of the International Conference. Walter de Gruyter, Berlin 1995

  34. Le Dret, H., Raoult, A.: The nonlinear membrane model as a variational limit of nonlinear three-dimensional elasticity. J. Math. Pures Appl. 74, 549-578 (1995)

    MATH  Google Scholar 

  35. Le Dret, H., Raoult, A.: The quasiconvex envelope of the Saint Venant-Kirchhoff stored energy function. Proc. Roy. Soc. Edinb. A 125, 1179-1192 (1995)

    MATH  Google Scholar 

  36. Le Dret H., Raoult, A.: The membrane shell model in nonlinear elasticity: a variational asymptotic derivation. J. Nonlinear Science 6, 59-84 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  37. Le Dret, H., Raoult, A.: Variational convergence for nonlinear shell models with directors and related semicontinuity and relaxation results. Arch. Rat. Mech. Anal. 154, 101-134 (2000)

    Article  MATH  Google Scholar 

  38. Ebenfeld, S.: A comparison of the shell theories in the sense of Kirchhoff-Love and Reissner-Mindlin, Preprint Nr. 2023, TU Darmstadt. Math. Meth. Appl. Sci. 22(17), 1505-1534 (1999)

  39. Ericksen, J.L., Truesdell, C.: Exact theory of stress and strain in rods and shells. Arch. Rat. Mech. Anal. 1, 295-323 (1958)

    MATH  Google Scholar 

  40. Eringen, A.C.: Theory of micropolar plates. Z. Angew. Math. Phys. 18, 12-30 (1967)

    Google Scholar 

  41. Fox, D.D., Raoult, A., Simo, J.C.: A justification of nonlinear properly invariant plate theories. Arch. Rat. Mech. Anal. 124, 157-199 (1993)

    MathSciNet  MATH  Google Scholar 

  42. Fox, D.D., Simo, J.C.: A drill rotation formulation for geometrically exact shells. Comp. Meth. Appl. Mech. Eng. 98, 329-343 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  43. Friesecke, G., James, R.D., Müller, S.: A theorem on geometric rigidity and the derivation of nonlinear plate theory from three-dimensional elasticity. Comm. Pure Appl. Math. LV(11), 1461-1506 (2002)

  44. Ge, Z., Kruse, H.P., Marsden, J.E.: The limits of Hamiltonian structures in three-dimensional elasticity, shells, and rods. J. Nonl. Science 6, 19-57 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  45. Green, A.E., Naghdi, P.M.: Shells in the light of generalized Cosserat continua. In: Niordson, F.I. (ed.), Theory of Thin Shells., IUTAM Symposium Copenhagen 1967, pp. 39-58. Springer, Heidelberg (1969)

  46. Green, A.E., Naghdi, P.M., Wainwright, W.L.: A general theory of a Cosserat surface. Arch. Rat. Mech. Anal. 20, 287-308 (1965)

    Google Scholar 

  47. Gruttmann, F., Stein, E., Wriggers, P.: Theory and numerics of thin elastic shells with finite rotations. Ing. Arch. 59, 54-67 (1989)

    Google Scholar 

  48. Gruttmann, F., Taylor, R.L.: Theory and finite element formulation of rubberlike membrane shells using principle stretches. Int. J. Num. Meth. Engrg. 35, 1111-1126 (1992)

    MATH  Google Scholar 

  49. Hartmann, S., Neff, P.: Polyconvexity of generalized polynomial type hyperelastic strain energy functions for near incompressibility. Int. J. Solids Struct. 40, 2767-2791 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  50. Hughes, T.J.R., Brezzi, F.: On drilling degrees of freedom. Comp. Meth. Appl. Mech. Engrg. 72, 105-121 (1989)

    Article  MathSciNet  MATH  Google Scholar 

  51. John, F.: Estimates for the derivatives of the stresses in a thin shell and interior shell equations. Comm. Pure Appl. Math. 18, 235-267 (1965)

    Google Scholar 

  52. John, F.: Refined interior equations for thin elastic shells. Comm. Pure Appl. Math. 24, 583-615 (1971)

    MATH  Google Scholar 

  53. Kilchevsky, N.A.: Fundamentals of the Analytical Mechanics of Shells, volume NASA TT F-292. NASA, Washington D.C., 1965

  54. Klingenberg, W.: A Course in Differential Geometry, vol. 51 of Graduate Texts in Mathematics. Spinger, New York, 1978

  55. Koiter, W.T.: A consistent first approximation in the general theory of thin elastic shells. In: Koiter, W.T. (ed.), The Theory of Thin Elastic Shells., IUTAM Symposium Delft 1960, pp. 12-33. North-Holland, Amsterdam (1960)

  56. Koiter, W.T.: Foundations and basic equations of shell theory. A survey of recent progress. In: Niordson, F.I. (ed.) Theory of Thin Shells., IUTAM Symposium Copenhagen 1967, pp. 93-105. Springer, Heidelberg, 1969

  57. Koiter, W.T.: On the foundations of the linear theory of thin elastic shells. Proc. Kon. Ned. Akad. Wetenschap B73, 169-195 (1970)

  58. Lew, A., Neff, P., Sulsky, D., Ortiz, M.: Optimal BV-estimates for a discontinuous Galerkin method in linear elasticity. Applied Mathematics Research Express (to appear), http://www.amrx.hindawi.com, 2004

  59. Li, Z.: Existence theorem and finite element method for static problems of a class of nonlinear hyperelastic shells. Chin. Ann. of Math 10B(2), 169-189 (1989)

    Google Scholar 

  60. Libai, A., Simmonds, J.G.: The Nonlinear Theory of Elastic Shells. Cambridge University Press, Cambridge, 1998

  61. Lods, V., Miara, B.: Nonlinearly elastic shell models: a formal asymptotic approach. II. The flexural model. Arch. Rat. Mech. Anal. 142, 355-374 (1998)

    Article  MATH  Google Scholar 

  62. Marsden, J.E., Hughes, J.R.: Mathematical Foundations of Elasticity. Prentice-Hall, Englewood Cliffs, New Jersey, 1983

  63. B. Miara. Nonlinearly elastic shell models: a formal asymptotic approach. I. The membrane model. Arch. Rat. Mech. Anal. 142, 331-353 (1998)

    Google Scholar 

  64. Mielke, A.: On the justification of plate theories in linear elasticity theory using exponential decay estimates. J. Elasticity 38, 165-208 (1995)

    MathSciNet  MATH  Google Scholar 

  65. Monneau, R.: Justification of the nonlinear Kirchhoff-Love theory of plates as the application of a new singular inverse method. Arch. Rat. Mech. Anal. 169, 1-34 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  66. Naghdi, P.M.: The theory of shells. In Handbuch der Physik, Mechanics of Solids, vol. VI a/2. Springer (1972)

  67. Neff, P.: A geometrically exact Cosserat-plate including size effects, avoiding degeneracy in the thin plate limit. Modelling and mathematical analysis. Preprint 2301, http://wwwbib.mathematik.tu-darmstadt.de/Math-Net/Preprints/Listen/pp03.html , 10/2003

  68. Neff, P.: On Korn’s first inequality with nonconstant coefficients. Proc. Roy. Soc. Edinb. 132A, 221-243 (2002)

    MathSciNet  MATH  Google Scholar 

  69. Neff, P.: Finite multiplicative plasticity for small elastic strains with linear balance equations and grain boundary relaxation. Cont. Mech. Thermodynamics 15(2)(DOI 10.1007/s00161-002-0190-x), 161-195 (2003)

    Google Scholar 

  70. Neff, P.: A geometrically exact micromorphic elastic solid. Modelling and existence of minimizers. Preprint 23xx, http://wwwbib.mathematik.tu-darmstadt.de/Math-Net/Preprints/Listen/pp04.html, submitted to Calculus of Variations, 2/2004

  71. Neff, P.: A geometrically exact Cosserat shell-model including size effects, avoiding degeneracy in the thin shell limit. Existence of minimizers for zero Cosserat couple modulus. Preprint 23xx, http://wwwbib.mathematik.tu-darmstadt.de/Math-Net/Preprints/Listen/pp03.html , 4/2004, submitted

  72. Neff, P.: Finite multiplicative elastic-viscoplastic Cosserat micropolar theory for polycrystals with grain rotations. Modelling and mathematical analysis. Preprint 2297, http://wwwbib.mathematik.tu-darmstadt.de/Math-Net/Preprints/Listen/pp03.html, submitted, 9/2003

  73. Neff, P., Wieners, C.: Comparison of models for finite plasticity. A numerical study. Comput. Visual. Sci. 6, 23-35 (2003)

    Google Scholar 

  74. Pietraszkiewicz, W.: Finite Rotations in Structural Mechanics. Number 19 in Lectures Notes in Engineering. Springer, Berlin, 1985

  75. Pipkin, A.C.: Relaxed energy densities for large deformations of membranes. IMA J. Appl. Math. 52, 297-308 (1994)

    MATH  Google Scholar 

  76. Podio-Guidugli, P., Vergara Caffarelli, G.: Extreme elastic deformations. Arch. Rat. Mech. Anal. 115, 311-328 (1991)

    MATH  Google Scholar 

  77. Pompe, W.: Korn’s first inequality with variable coefficients and its generalizations. Comment. Math. Univ. Carolinae 44,1, 57-70 (2003)

    Google Scholar 

  78. Roehl, D., Ramm, E.: Large elasto-plastic finite element analysis of solids and shells with the enhanced assumed strain concept. Int. J. Solids Struct. 33, 3215-3237 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  79. Rössle, A.: On the derivation of an asymptotically correct shear correction factor for the Reissner-Mindlin plate model. C. R. Acad. Sci. Paris, Ser. I, Math. 328(3), 269-274 (1999)

    Google Scholar 

  80. Rössle, A., Bischoff, M., Wendland, W., Ramm, E.: On the mathematical foundation of the (1,1,2)-plate model. Int. J. Solids Struct. 36, 2143-2168 (1999)

    Article  MathSciNet  Google Scholar 

  81. Rubin, M.B.: Cosserat Theories: Shells, Rods and Points. Kluwer Academic Publishers, Dordrecht, 2000

  82. Sansour, C.: A theory and finite element formulation of shells at finite deformations including thickness change: circumventing the use of a rotation tensor. Arch. Appl. Mech. 10, 194-216 (1995)

    Article  Google Scholar 

  83. Sansour, C., Bednarczyk, H.: The Cosserat surface as a shell model, theory and finite element formulation. Comp. Meth. Appl. Mech. Eng. 120, 1-32 (1995)

    Article  MathSciNet  MATH  Google Scholar 

  84. Sansour, C., Bocko, J.: On hybrid stress, hybrid strain and enhanced strain finite element formulations for a geometrically exact shell theory with drilling degrees of freedom. Int. J. Num. Meth. Engrg. 43, 175-192 (1998)

    Article  MATH  Google Scholar 

  85. Sansour, C., Bufler, H.: An exact finite rotation shell theory, its mixed variational formulation and its finite element implementation. Int. J. Num. Meth. Engrg. 34, 73-115 (1992)

    MathSciNet  MATH  Google Scholar 

  86. Schmidt, R.: Polar decomposition and finite rotation vector in first order finite elastic strain shell theory. In: Pietraszkiewicz, W. (ed.) Finite Rotations in Structural Mechanics, no. 19 in Lecture Notes in Engineering. Springer, Berlin, 1985

  87. Schröder, J., Neff, P.: Invariant formulation of hyperelastic transverse isotropy based on polyconvex free energy functions. Int. J. Solids Struct. 40, 401-445 (2002)

    Article  Google Scholar 

  88. Schwab, C.: A-posteriori modelling error estimation for hierarchic plate models. Numer. Math. 74, 221-259 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  89. Simmonds, J.G., Danielson, D.A.: Nonlinear shell theory with finite rotation and stress-function vectors. J. Appl. Mech. Trans. ASME. 39, 1085-1090 (1972)

    MATH  Google Scholar 

  90. Simo, J.C., Fox, D.D.: On a stress resultant geometrically exact shell model. Part I: Formulation and optimal parametrization. Comp. Meth. Appl. Mech. Eng. 72, 267-304 (1989)

    Google Scholar 

  91. Simo, J.C., Fox, D.D.: On a stress resultant geometrically exact shell model. Part VI: Conserving algorithms for non-linear dynamics. Comp. Meth. Appl. Mech. Eng. 34, 117-164 (1992)

    MATH  Google Scholar 

  92. Simo, J.C., Fox, D.D., Hughes, T.J.R.: Formulations of finite elasticity with independent rotations. Comp. Meth. Appl. Mech. Engrg. 95, 277-288 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  93. Simo, J.C., Fox, D.D., Rifai, M.S.: On a stress resultant geometrically exact shell model. Part II: The linear theory; computational aspects. Comp. Meth. Appl. Mech. Eng. 73, 53-92 (1989)

    Google Scholar 

  94. Simo, J.C., Fox, D.D., Rifai, M.S.: On a stress resultant geometrically exact shell model. Part III: Computational aspects of the nonlinear theory. Comp. Meth. Appl. Mech. Eng. 79, 21-70 (1990)

    Google Scholar 

  95. Simo, J.C., Kennedy, J.G.: On a stress resultant geometrically exact shell model. Part V: Nonlinear plasticity: formulation and integration algorithms. Comp. Meth. Appl. Mech. Eng. 96, 133-171 (1992)

    Article  MATH  Google Scholar 

  96. Simo, J.C., Rifai, M.S., Fox. D.D.: On a stress resultant geometrically exact shell model. Part IV: Variable thickness shells with through the thickness stretching. Comp. Meth. Appl. Mech. Eng. 81, 91-126 (1990)

    Article  MATH  Google Scholar 

  97. Steigmann, D.J.: Tension-field theory. Proc. R. Soc. London A 429, 141-173 (1990)

    MathSciNet  MATH  Google Scholar 

  98. Stenberg, R.: A new finite element formulation for the plate bending problem. In: Ciarlet, P.G., Trabucho, L., Viano, J.M. (eds.), Asymptotique Methods for Elastic Structures, Proceedings of the International Conference. Walter de Gruyter, Berlin, 1995

  99. Wisniewski, K., Turska, E.: Kinematics of finite rotation shells with in-plane twist parameter. Comp. Meth. Appl. Mech. Engng. 190, 1117-1135, (2000)

    Article  MATH  Google Scholar 

  100. Wisniewski, K., Turska, E.: Warping and in-plane twist parameters in kinematics of finite rotation shells. Comp. Meth. Appl. Mech. Engng. 190, 5739-5758, (2001)

    Article  MATH  Google Scholar 

  101. Wisniewski, K., Turska, E.: Second-order shell kinematics implied by rotation constraint-equation. J. Elasticity 67, 229-246 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  102. Wriggers, P., Gruttmann, F.: Thin shells with finite rotations formulated in Biot stresses: Theory and finite element formulation. Int. J. Num. Meth. Engrg. 36, 2049-2071 (1993)

    MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, Darmstadt University of Technology, Schlossgartenstrasse 7, 64289, Darmstadt, Germany

    P. Neff

Authors
  1. P. Neff
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to P. Neff.

Additional information

Communicated by K. Hutter

Received: 16 April 2004, Accepted: 3 May 2004, Published online: 17 September 2004

Rights and permissions

Reprints and permissions

About this article

Cite this article

Neff, P. A geometrically exact Cosserat shell-model including size effects, avoiding degeneracy in the thin shell limit. Part I: Formal dimensional reduction for elastic plates and existence of minimizers for positive Cosserat couple modulus. Continuum Mech. Thermodyn. 16, 577–628 (2004). https://doi.org/10.1007/s00161-004-0182-4

Download citation

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00161-004-0182-4

Keywords:

Search

Navigation