Numerical and experimental case study on simultaneous optimization of blank shape and variable blank holder force trajectory in deep drawing

Abstract

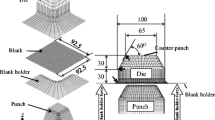
This paper shows a case study for a simultaneous optimization of blank shape and variable blank holder force (VBHF) trajectory in deep drawing, which is one of the challenging issues in sheet metal forming in industry. Blank shape directly affects the material cost. To reduce the material cost, it is important to determine an optimal blank shape minimizing earing. In addition, VBHF approach is recognized as an attractive and crucial technology for successful sheet metal forming, but the practical application is rarely reported. To resolve these issues, the simultaneous optimization of blank shape and VBHF trajectory is performed. First, the experiment to identify the wrinkling region is carried out. Based on the experimental results, the finite element analysis (FEA) model is developed. The validity of the FEA model is examined by using the FLD. Numerical simulation in deep drawing is so intensive that a sequential approximate optimization (SAO) using a radial basis function (RBF) network is used for the numerical optimization. Based on the numerical result, the experiment using the AC servo press is carried out. It is found from the experimental results that the successful sheet metal forming is performed. In addition, it is confirmed from the numerical and experimental result that both the material cost and the forming energy are simultaneously reduced by using the design optimization technique.

Appendices

Appendix I

In this appendix, two optimal VBHF trajectories are determined with different objective functions. Note that the blank shape is not optimized, and the full blank size shown in Table 1 is used. The optimal VBHF trajectory is determined under tearing and wrinkling conditions. Therefore, the tearing and wrinkling are handled as the design constraints, and are evaluated as described in section 3.3. We consider the following two objective functions: (Case I) the forming energy, and (Case II) the thickness deviation. The optimal VBHF trajectory is determined through the numerical optimization. Based on the numerical result, the experiment is carried out using the AC servo press. The total stroke is divided into 4 sub-stroke steps, as shown in Fig. 21. Therefore, in both cases, the number of the design variables is 4.

Fig. 21

Design variables for VBHF trajectory in Cases I and II

1.1 Case I

The forming energy represented by the gray area in Fig. 21 is taken as the objective function. The optimal VBHF trajectory is shown in Fig. 22, in which the red line and the dashed line represent the optimal VBHF trajectory in the experiments and in the numerical simulation, respectively. Figure 23 shows the experimental result. Figure 24 shows the lengths for dimension accuracy, and the result is summarized in Table 5.

Fig. 22

Optimal VBHF trajectory in numerical simulation and experiment of Case I

Fig. 23

Experimental result in Case I

Fig. 24

Evaluation for dimension accuracy in Case I

Table 5 Dimension accuracy between numerical simulation and experiment in Case I

1.2 Case II

Second case considers minimizing the thickness deviation, which is defined as the following equation:

$$ f\left(\boldsymbol{x}\right)={\left\{{\displaystyle {\sum}_{i=1}^{nelm}{\left(\frac{t_i}{t_0}-1\right)}^p}\right\}}^{1/p}\to \min $$

(A1)

where t _i denotes the thickness of the i-th element of the blank, t ₀ the initial thickness of the blank, respectively. p in (A1) is the parameter, and is set to 4. The optimal VBHF trajectory is shown in Fig. 25, in which the red line and the dashed line represent the optimal VBHF trajectory in the experiments and in the numerical simulation, respectively. Figure 26 shows the experimental result. Figure 27 shows the lengths for dimension accuracy, and the result is summarized in Table 6.

Fig. 25

Optimal VBHF trajectory in numerical simulation and experiment of Case II

Fig. 26

Experimental result in Case II

Fig. 27

Evaluation for dimension accuracy in Case II

Fig. 28

Excel VBA code for numerically evaluating risk of wrinkling and tearing

Table 6 Dimension accuracy between numerical simulation and experiment in Case II

Appendix II

Two critical lines for wrinkling and tearing of the FLD shown in Fig. 6 are drawn as follows:

The normal anisotropy coefficient r (called Lankford value) is used for the critical line for wrinkling, which is simply given by the following equation:

$$ \frac{\varepsilon_2}{\varepsilon_1}=-\frac{1+r}{r} $$

(A2)

Next, the critical curve for tearing is defined as follows:

$$ {\varepsilon}_1=\left\{\begin{array}{cc}\hfill \frac{N}{\left(1+\beta \right)\left\{\frac{1-N}{2}+{\left[\frac{{\left(1+N\right)}^2}{4}-\frac{\beta N}{{\left(1+\beta \right)}^2}\right]}^{\frac{1}{2}}\right\}}\hfill & \hfill -1\le \beta \le 0\hfill \\ {}\hfill \frac{3{\beta}^2+N{\left(2+\beta \right)}^2}{2\left(2+\beta \right)\left(1+\beta +{\beta}^2\right)}\hfill & \hfill 0\le \beta \le 1\hfill \end{array}\right. $$

(A3)

where β = ε ₂/ε ₁, N denotes the strain hardening coefficient. A3 is solved with respect to ε ₁, and finally the following equations can be obtained for the critical curve for tearing:

$$ {\varepsilon}_1=\left\{\begin{array}{cc}\hfill \frac{1}{2}\left(-1-{\varepsilon}_2+N+\sqrt{A}\right)\hfill & \hfill {\varepsilon}_2<0\hfill \\ {}\hfill \frac{1}{6}\left[\left(-3{\varepsilon}_2+2N\right)-\frac{9{\varepsilon}_2^2-4{N}^2}{B}+B\right]\hfill & \hfill {\varepsilon}_2\ge 0\hfill \end{array}\right. $$

(A4)

where

$$ \left.\begin{array}{l}A=1-2{\varepsilon}_2-3{\varepsilon}_2^2+2N+2{\varepsilon}_2N+{N}^2\\ {}B={\left\{C+9\sqrt{D}\right\}}^{\frac{1}{3}}\\ {}C=81{\varepsilon}_2^2-27{\varepsilon}_2^2N+8{N}^3\\ {}D=81{\varepsilon}_2^4+9{\varepsilon}_2^6-54{\varepsilon}_2^4N-3{\varepsilon}_2^4{N}^2+16{\varepsilon}_2^2{N}^3\end{array}\right\} $$

(A5)

For better understanding, Excel VBA code to numerically evaluate the risk of wrinkling and tearing is listed below (See Fig. 28), in which the following symbols are used:

nelm:

the number of finite elements of blank

r:

the normal anisotropy coefficient r (Lankford value)

n:

the strain hardening coefficient

sfact:

safety tolerance in (12)

eps1(i):

major strain of the i-th element of blank, which is obtained from LS-DYNA

eps2(i):

minor strain of the i-th element of blank, which is obtained from LS-DYNA.