Improved incremental transfer matrix method for nonlinear rotor-bearing system

Abstract

Rotor system supported by nonlinear bearing such as squeeze film damper (SFD) is widely used in practice owing to its wide range of damping capacity and simplicity in structure. In this paper, an improved and effective Incremental transfer matrix method (ITMM) is first presented by combining ITMM and fast Fourier transform (FFT). Afterwards this method is applied to calculate the dynamic characteristics of a Jeffcott rotor system with SFD. The convergence difficulties incurred caused by strong nonlinearities of SFD has been dealt by adopting a control factor. It is found that for the more general boundary problems where the boundary conditions are not at input and output ends of a chain system, the supplementary equation is necessarily added. Additionally, the Floquet theory is used to analyze the stability and bifurcation type of the obtained periodic solution. The semi-analytical results, including the periodic solutions of the system, the bifurcation points and their types, are in good agreement with the numerical method. Furthermore, the involution mechanism of the quasi-periodic and chaotic motions near the first-order translational mode and the second order bending mode of this system is also clarified by this method with the aid of Floquet theory.

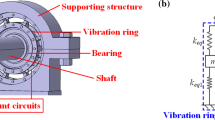
Graphical abstract

Appendices

Appendix I

1.1 Expressions of derivatives of the oil force

In this section, the expressions of derivatives of the oil force generated by squeeze film damper in Sect. 2.2 are given as follows,

$$\frac{{\partial \bar{F}_{x} }}{\partial x} = \frac{{\partial \bar{F}_{t} }}{\partial x}\sin \psi + \bar{F}_{t} \frac{{\partial \sin \psi_{t} }}{\partial x} - \frac{{\partial \bar{F}_{r} }}{\partial x}\cos \psi - \bar{F}_{r} \frac{\partial \cos \psi }{\partial x},$$

$$\frac{{\partial \bar{F}_{x} }}{\partial y} = \frac{{\partial \bar{F}_{t} }}{\partial y}\sin \psi + \bar{F}_{t} \frac{{\partial \sin \psi_{t} }}{\partial y} - \frac{{\partial \bar{F}_{r} }}{\partial y}\cos \psi - \bar{F}_{r} \frac{\partial \cos \psi }{\partial y},$$

$$\frac{{\partial \bar{F}_{x} }}{{\partial x^{\prime}}} = \frac{{\partial \bar{F}_{t} }}{{\partial x^{\prime}}}\sin \psi - \frac{{\partial \bar{F}_{r} }}{{\partial x^{\prime}}}\cos \psi ,$$

$$\frac{{\partial \bar{F}_{x} }}{{\partial y^{\prime}}} = \frac{{\partial \bar{F}_{t} }}{{\partial y^{\prime}}}\sin \psi - \frac{{\partial \bar{F}_{r} }}{{\partial y^{\prime}}}\cos \psi ,$$

$$\frac{{\partial \bar{F}_{x} }}{\partial \omega } = \frac{{\partial \bar{F}_{t} }}{\partial \omega }\sin \psi - \frac{{\partial \bar{F}_{r} }}{\partial \omega }\cos \psi ,$$

$$\frac{{\partial \bar{F}_{y} }}{\partial x} = - \frac{{\partial \bar{F}_{t} }}{\partial x}\cos \psi - \bar{F}_{t} \frac{{\partial \cos \psi_{t} }}{\partial x} - \frac{{\partial \bar{F}_{r} }}{\partial x}\sin \psi - \bar{F}_{r} \frac{\partial \sin \psi }{\partial x},$$

$$\frac{{\partial \bar{F}_{y} }}{\partial y} = - \frac{{\partial \bar{F}_{t} }}{\partial y}\cos \psi - \bar{F}_{t} \frac{{\partial \cos \psi_{t} }}{\partial y} - \frac{{\partial \bar{F}_{r} }}{\partial y}\sin \psi - \bar{F}_{r} \frac{\partial \sin \psi }{\partial y},$$

$$\frac{{\partial \bar{F}_{y} }}{{\partial x^{\prime}}} = - \frac{{\partial \bar{F}_{t} }}{{\partial x^{\prime}}}\cos \psi - \frac{{\partial \bar{F}_{r} }}{{\partial x^{\prime}}}\sin \psi ,$$

$$\frac{{\partial \bar{F}_{y} }}{{\partial y^{\prime}}} = - \frac{{\partial \bar{F}_{t} }}{{\partial y^{\prime}}}\cos \psi - \frac{{\partial \bar{F}_{r} }}{{\partial y^{\prime}}}\sin \psi ,$$

$$\frac{{\partial \bar{F}_{y} }}{\partial \omega } = - \frac{{\partial \bar{F}_{t} }}{\partial \omega }\cos \psi - \frac{{\partial \bar{F}_{r} }}{\partial \omega }\sin \psi .$$

The analytical expressions for $F_{t}$ and $F_{r}$ have been derived in Sect. 2.3, where the specific expressions for $\varepsilon$, $\dot{\varepsilon }$, $\psi$ and $\dot{\psi }$ in them are

$$\varepsilon = \sqrt {(x^{2} + y^{2} )} ,\;\dot{\varepsilon } = \frac{{x\dot{x} + y\dot{y}}}{\varepsilon },\;\psi = \arctan \left(\frac{y}{x}\right),\;\dot{\psi } = - \frac{{\dot{x}y}}{{x^{2} + y^{2} }} + \frac{{x\dot{y}}}{{x^{2} + y^{2} }}.$$

Appendix II

2.1 Transfer matrices

In Sect. 2, the incremental transfer matrix of bearing supported by SFD has been derived. As a supplement, the other matrices of components are directly given here.

• (1) Disk

  Figure 14 shows a simplified model of the disk, the transfer equation is

  $$\left\{ {\begin{array}{*{20}c} {\Delta {\mathbf{A}}_{x} } \\ {\Delta {\mathbf{B}}_{\beta } } \\ {\Delta {\mathbf{H}}_{y} } \\ {\Delta {\mathbf{G}}_{x} } \\ {\Delta {\mathbf{A}}_{y} } \\ {\Delta {\mathbf{B}}_{\alpha } } \\ {\Delta {\mathbf{H}}_{x} } \\ {\Delta {\mathbf{G}}_{y} } \\ {\Delta \omega } \\ 1 \\ \end{array} } \right\}^{\text{R}} = \left[ {\begin{array}{*{20}c} {\mathbf{I}} & {} & {} & {} & {} & {} & {} & {} & {} & {} \\ {} & {\mathbf{I}} & {} & {} & {} & {} & {} & {} & {} & {} \\ {} & { - {\mathbf{P}}_{14}^{\prime } } & {\mathbf{I}} & {} & {} & { - {\mathbf{P}}_{15}^{\prime } } & {} & {} & { - {\mathbf{P}}_{16}^{\prime } } & { - {\mathbf{P}}_{13}^{\prime } } \\ {{\mathbf{P}}_{4}^{\prime } } & {} & {} & {\mathbf{I}} & {} & {} & {} & {} & {{\mathbf{P}}_{5}^{\prime } } & {{\mathbf{P}}_{3}^{\prime } + {\mathbf{P}}_{6}^{\prime } } \\ {} & {} & {} & {} & {\mathbf{I}} & {} & {} & {} & {} & {} \\ {} & {} & {} & {} & {} & {\mathbf{I}} & {} & {} & {} & {} \\ {} & {{\mathbf{P}}_{20}^{\prime } } & {} & {} & {} & { - {\mathbf{P}}_{19}^{\prime } } & {\mathbf{I}} & {} & { - {\mathbf{P}}_{21}^{\prime } } & {{\mathbf{P}}_{18}^{\prime } } \\ {} & {} & {} & {} & { - {\mathbf{P}}_{9}^{\prime } } & {} & {} & {\mathbf{I}} & {{\mathbf{P}}_{10}^{\prime } } & {{\mathbf{P}}_{8}^{\prime } + {\mathbf{P}}_{11}^{\prime } } \\ {} & {} & {} & {} & {} & {} & {} & {} & 1 & {} \\ {} & {} & {} & {} & {} & {} & {} & {} & {} & 1 \\ \end{array} } \right]\left\{ {\begin{array}{*{20}c} {\Delta {\mathbf{A}}_{x} } \\ {\Delta {\mathbf{B}}_{\beta } } \\ {\Delta {\mathbf{H}}_{y} } \\ {\Delta {\mathbf{G}}_{x} } \\ {\Delta {\mathbf{A}}_{y} } \\ {\Delta {\mathbf{B}}_{\alpha } } \\ {\Delta {\mathbf{H}}_{x} } \\ {\Delta {\mathbf{G}}_{y} } \\ {\Delta \omega } \\ 1 \\ \end{array} } \right\}^{\text{L}} ,$$

  where ${\mathbf{P}}_{1} = \int_{0}^{{2{\uppi}}} {{\mathbf{S}}^{\text{T}} {\mathbf{S}}{\text{d}}\tau }$, ${\mathbf{P}}_{2} = \int_{0}^{{2{\uppi}}} {{\mathbf{S}}^{\text{T}} {\mathbf{S}}{\text{d}}\tau }$, ${\mathbf{P}}_{3} = \int_{0}^{{2{\uppi}}} {{\mathbf{S}}^{\text{T}} (q_{x10} - m\omega_{0}^{2} x_{0}^{\prime \prime } + u\omega_{0}^{2} \cos \tau - q_{x20} ){\text{d}}\tau }$, ${\mathbf{P}}_{4} = \int_{0}^{{2{\uppi}}} {{\mathbf{S}}^{\text{T}} \omega_{0}^{2} m{\mathbf{S^{\prime\prime}}}{\text{d}}\tau }$, ${\mathbf{P}}_{5} = \int_{0}^{{2{\uppi}}} {{\mathbf{S}}^{\text{T}} (2u\omega_{0} \cos \tau - 2\omega_{0} mx_{0}^{\prime \prime } ){\text{d}}\tau }$, ${\mathbf{P}}_{7} = \int_{0}^{{2{\uppi}}} {{\mathbf{S}}^{\text{T}} {\mathbf{S}}{\text{d}}\tau }$, ${\mathbf{P}}_{8} = \int_{0}^{{2{\uppi}}} {{\mathbf{S}}^{\text{T}} (q_{y10} - m\omega_{0}^{2} y_{0}^{\prime \prime } + u\omega_{0}^{2} \sin \tau - q_{y20} ){\text{d}}\tau }$, ${\mathbf{P}}_{9} = \int_{0}^{{2{\uppi}}} {{\mathbf{S}}^{\text{T}} \omega_{0}^{2} m{\mathbf{S^{\prime\prime}}}{\text{d}}\tau }$, ${\mathbf{P}}_{10} = \int_{0}^{{2{\uppi}}} {{\mathbf{S}}^{\text{T}} (2u\omega_{0} \sin \tau - 2\omega_{0} my_{0}^{\prime \prime } ){\text{d}}\tau }$, ${\mathbf{P}}_{12} = \int_{0}^{{2{\uppi}}} {{\mathbf{S}}^{\text{T}} {\mathbf{S}}{\text{d}}\tau }$, ${\mathbf{P}}_{13} = \int_{0}^{{2{\uppi}}} {{\mathbf{S}}^{\text{T}} (M_{y10} - J_{d} \omega_{0}^{2} \beta_{0}^{\prime \prime } - J_{p} \omega_{0}^{2} a_{0}^{\prime } - M_{y20} ){\text{d}}\tau }$, ${\mathbf{P}}_{14} = \int_{0}^{{2{\uppi}}} {{\mathbf{S}}^{\text{T}} \omega_{0}^{2} J_{d} {\mathbf{S^{\prime\prime}}}{\text{d}}\tau }$, ${\mathbf{P}}_{15} = \int_{0}^{{2{\uppi}}} {{\mathbf{S}}^{\text{T}} \omega_{0}^{2} J_{p} {\mathbf{S^{\prime}}}{\text{d}}\tau }$, ${\mathbf{P}}_{16} = \int_{0}^{{2{\uppi}}} {{\mathbf{S}}^{\text{T}} (2\omega_{0} J_{d} \beta_{0}^{\prime \prime } + 2\omega_{0} J_{p} \alpha^{\prime}){\text{d}}\tau }$, ${\mathbf{P}}_{17} = \int_{0}^{{2{\uppi}}} {{\mathbf{S}}^{\text{T}} {\mathbf{S}}{\text{d}}\tau }$, ${\mathbf{P}}_{18} = \int_{0}^{{2{\uppi}}} {{\mathbf{S}}^{\text{T}} (M_{x10} - J_{d} \omega_{0}^{2} \alpha_{0}^{\prime \prime } + J_{p} \omega_{0}^{2} \beta_{0}^{\prime } - M_{x20} ){\text{d}}\tau }$, ${\mathbf{P}}_{19} = \int_{0}^{{2{\uppi}}} {{\mathbf{S}}^{\text{T}} \omega_{0}^{2} J_{d} {\mathbf{S^{\prime\prime}}}{\text{d}}\tau }$, ${\mathbf{P}}_{20} = \int_{0}^{{2{\uppi}}} {{\mathbf{S}}^{\text{T}} \omega_{0}^{2} J_{p} {\mathbf{S^{\prime}}}{\text{d}}\tau }$, ${\mathbf{P}}_{21} = \int_{0}^{{2{\uppi}}} {{\mathbf{S}}^{\text{T}} (2\omega_{0} J_{d} \alpha_{0}^{\prime \prime } - 2\omega_{0} J_{p} \beta^{\prime}){\text{d}}\tau }$.

  Fig. 14

  

  Model of disk

• (2) Massless beam

  $$\left\{ {\begin{array}{*{20}c} {\Delta {\mathbf{A}}_{x} } \\ {\Delta {\mathbf{B}}_{\beta } } \\ {\Delta {\mathbf{H}}_{y} } \\ {\Delta {\mathbf{G}}_{x} } \\ {\Delta {\mathbf{A}}_{y} } \\ {\Delta {\mathbf{B}}_{\alpha } } \\ {\Delta {\mathbf{H}}_{x} } \\ {\Delta {\mathbf{G}}_{y} } \\ {\Delta \omega } \\ 1 \\ \end{array} } \right\}^{\text{R}} = \left[ {\begin{array}{*{20}c} {{\mathbf{U}}_{1} } & {} & {} \\ {} & {{\mathbf{U}}_{2} } & {} \\ {} & {} & {{\mathbf{I}}_{2} } \\ \end{array} } \right]\left\{ {\begin{array}{*{20}c} {\Delta {\mathbf{A}}_{x} } \\ {\Delta {\mathbf{B}}_{\beta } } \\ {\Delta {\mathbf{H}}_{y} } \\ {\Delta {\mathbf{G}}_{x} } \\ {\Delta {\mathbf{A}}_{y} } \\ {\Delta {\mathbf{B}}_{\alpha } } \\ {\Delta {\mathbf{H}}_{x} } \\ {\Delta {\mathbf{G}}_{y} } \\ {\Delta \omega } \\ 1 \\ \end{array} } \right\}^{\text{L}} ,$$

  where ${\mathbf{U}}_{1} = \left[ {\begin{array}{*{20}c} {\mathbf{I}} & {{\mathbf{B}}_{1}^{\prime } } & {{\mathbf{B}}_{2}^{\prime } } & {{\mathbf{B}}_{3}^{\prime } } \\ {} & {\mathbf{I}} & {{\mathbf{B}}_{4}^{\prime } } & {{\mathbf{B}}_{5}^{\prime } } \\ {} & {} & {\mathbf{I}} & {{\mathbf{B}}_{6}^{\prime } } \\ {} & {} & {} & {\mathbf{I}} \\ \end{array} } \right]$, ${\mathbf{B}}_{1} = \int_{0}^{{2{\uppi}}} {{\mathbf{S}}^{\text{T}} l{\mathbf{S}}{\text{d}}\tau }$, ${\mathbf{B}}_{2} = \int_{0}^{{2{\uppi}}} {{\mathbf{S}}^{\text{T}} \frac{{l^{2} }}{EI}{\mathbf{S}}{\text{d}}\tau }$, ${\mathbf{B}}_{3} = \int_{0}^{{2{\uppi}}} {{\mathbf{S}}^{\text{T}} \frac{{l^{3} (1 - \nu )}}{6EI}{\mathbf{S}}{\text{d}}\tau }$, ${\mathbf{B}}_{4} = \int_{0}^{{2{\uppi}}} {{\mathbf{S}}^{\text{T}} \frac{l}{EI}{\mathbf{S}}{\text{d}}\tau }$, ${\mathbf{B}}_{5} = \int_{0}^{{2{\uppi}}} {{\mathbf{S}}^{\text{T}} \frac{{l^{2} }}{2EI}{\mathbf{S}}{\text{d}}\tau }$, ${\mathbf{B}}_{6} = \int_{0}^{{2{\uppi}}} {{\mathbf{S}}^{\text{T}} l{\mathbf{S}}{\text{d}}\tau }$, ${\mathbf{U}}_{2} = {\mathbf{R}}^{\text{T}} {\mathbf{U}}_{1} {\mathbf{R}}$, ${\mathbf{R}} = {\text{diag}}({\mathbf{I}},\; - {\mathbf{I}},\; - {\mathbf{I}},\;{\mathbf{I}})$.

• (3) Bearing supported by linear-elastic spring

  $$\left\{ {\begin{array}{*{20}c} {\Delta {\mathbf{A}}_{x} } \\ {\Delta {\mathbf{B}}_{\beta } } \\ {\Delta {\mathbf{H}}_{y} } \\ {\Delta {\mathbf{G}}_{x} } \\ {\Delta {\mathbf{A}}_{y} } \\ {\Delta {\mathbf{B}}_{\alpha } } \\ {\Delta {\mathbf{H}}_{x} } \\ {\Delta {\mathbf{G}}_{y} } \\ {\Delta \omega } \\ 1 \\ \end{array} } \right\}^{\text{R}} = \left[ {\begin{array}{*{20}c} {{\mathbf{U}}_{1} } & {} & {} \\ {} & {{\mathbf{U}}_{2} } & {} \\ {} & {} & {{\mathbf{I}}_{2} } \\ \end{array} } \right]\left\{ {\begin{array}{*{20}c} {\Delta {\mathbf{A}}_{x} } \\ {\Delta {\mathbf{B}}_{\beta } } \\ {\Delta {\mathbf{H}}_{y} } \\ {\Delta {\mathbf{G}}_{x} } \\ {\Delta {\mathbf{A}}_{y} } \\ {\Delta {\mathbf{B}}_{\alpha } } \\ {\Delta {\mathbf{H}}_{x} } \\ {\Delta {\mathbf{G}}_{y} } \\ {\Delta \omega } \\ 1 \\ \end{array} } \right\}^{\text{L}} ,$$

  where ${\mathbf{U}}_{1} = \left[ {\begin{array}{*{20}c} {\mathbf{I}} & {} & {} & {} \\ {} & {\mathbf{I}} & {} & {} \\ {} & {} & {\mathbf{I}} & {} \\ { - ({\mathbf{L}}_{1} + {\mathbf{L}}_{2} )} & {} & {} & {\mathbf{I}} \\ \end{array} } \right]$, ${\mathbf{L}}_{1} + {\mathbf{L}}_{2} = \frac{{\int_{0}^{{2{\uppi}}} {{\mathbf{S}}^{\text{T}} m\omega^{2} {\mathbf{S^{\prime\prime}}}{\text{d}}\tau } + \int_{0}^{{2{\uppi}}} {{\mathbf{S}}^{\text{T}} k{\mathbf{S}}{\text{d}}\tau } }}{{\int_{0}^{{2{\uppi}}} {{\mathbf{S}}^{\text{T}} {\mathbf{S}}d\tau } }}$, ${\mathbf{U}}_{2} = {\mathbf{R}}^{\text{T}} {\mathbf{U}}_{1} {\mathbf{R}}$, ${\mathbf{R}} = {\text{diag}}({\mathbf{I}},\; - {\mathbf{I}},\; - {\mathbf{I}},\;{\mathbf{I}})$.