Abstract
Mathematical modelling is a cyclic process in which a modeller evaluates a real-life scenario using mathematics. It is rarely included in the curriculum for pupils prior to secondary school in the United States and is thus unfamiliar to most elementary teachers . In this study, we begin by describing our perspectives and stance on professional development for elementary school teachers in mathematical modelling from both content and pedagogical aspects. We then describe how engaging teachers and students in mathematical modelling promoted mathematical communities of practice through classroom values of relevance, access, and engagement. Findings from a narrative analysis of field notes and transcripts from teacher study groups suggest that when teachers create modelling tasks with these values in mind, modelling provides opportunities for all students to use mathematics to solve problems that matter to them in a way that fosters and benefits community.
You have full access to this open access chapter, Download chapter PDF
Similar content being viewed by others
Keywords
7.1 Introduction
The idea that mathematical modelling provides powerful learning opportunities for students is not new. In Principles and Standards for School Mathematics (2000), the National Council of Teachers of Mathematics (NCTM) asserted, “One of the powerful uses of mathematics is the mathematical modelling of phenomena. Students at all levels should have opportunities to model a wide variety of phenomena mathematically in ways that are appropriate to their level” (p. 39). Yet models and modelling perspectives on teaching and learning mathematics have yet to take hold in U.S. classrooms, especially at the elementary school level. There is, however, reason to believe this trend will change. A growing body of research highlights the affordances of mathematical modelling across grades K–12 (e.g. Brown 2013; Doerr and English 2006; Doerr and Lesh 2011; English 2004, 2006; Lesh and Doerr 2003). The Common Core State Standards for School Mathematics (National Governors Association Center for Best Practices & Council of Chief State School Officers 2010), wherein modelling is included as both a high school content strand and a Standard for Mathematical Practice, has led to a renewed focus on teaching and learning mathematical modelling in the USA.
A critical next step in developing and promoting high-quality modelling experiences for children and youth is to understand the learning opportunities teachers need in order to facilitate such experiences. Modelling certainly will not become an integral part of students’ mathematical learning if their teachers are not prepared to provide classroom leadership in this area. This chapter describes our efforts to engage a community of elementary school teachers in professional development focused on mathematical modelling and our own discoveries as we followed the teachers into their classrooms to implement what they had learnt.
We envisioned mathematical modelling as a tool that elementary teachers could use to help students see mathematics, early in their academic careers, as open, rich, creative, and purposeful. As we enacted modelling tasks with teachers and as they engaged students in mathematical modelling, we found that modelling promoted mathematical communities of practice (Wenger 1998) in which modellers developed a shared purpose, relied on shared knowledge resources to determine solutions, and respected each other’s mathematical contributions. We know that developing meaningful mathematical tasks and facilitating mathematical discussions where all students are heard is not easy (Smith and Stein 2001) and we investigated what it was about modelling that promoted these practices.
In this chapter, we describe why we believe modelling helps to foster communities of practice . We describe our research journey by providing rich description of professional development provided to our teachers. Next, we describe our theoretical framework, communities of practice (Wenger 1998) in relation to three teacher-developed modelling tasks to understand what attributes of the task may have led to community building. Lastly, we look across the teacher-developed modelling tasks to consider implications for our work and for future professional development.
7.2 Perspectives and Stance on Modelling Professional Development
Teaching mathematical modelling is demanding work. Teachers must draw on multiple dimensions of knowledge (Koellner-Clark and Lesh 2003) including, but not limited to pedagogical content knowledge (Blum 2011, 2015; Doerr and Lesh 2011), knowledge of the modelling process, knowledge of students’ backgrounds and experiences, and knowledge of instructional practices that facilitate individual and group learning (Zawojewski et al. 2003). Moreover, the open nature of many modelling activities means that teachers must allow for, and respond to, student conceptions as they emerge.
Explicit attention to models and modelling is not typical in U.S. teacher preparation programs (Doerr 2007). Even experienced and skilled teachers may not automatically transfer their knowledge of teaching mathematics to teaching mathematical modelling (Niss et al. 2007). Thus, teachers need a variety of experiences and support in order to effectively engage students in mathematical modelling.
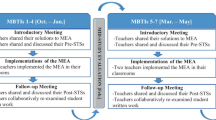
Professional development is most effective when it is sustained, intensive, and integrated into teachers’ daily work (Garet et al. 2001). It should also be “learner centered” (Hawley and Valli 1999, p. 137), accounting for teachers’ existing knowledge, experiences, and beliefs. We adopted a two-phase format for our professional development. First, teachers engaged in a week-long intensive summer institute focused on mathematical modelling . Then, during the school year, teachers were organized into study groups that worked together to develop and implement tasks in their own classrooms.
For our project, situating teachers’ initial learning experiences outside their regular classrooms was important. We wanted our participants to begin to think about mathematics from new and different perspectives, perspectives that might conflict with, or call into question, their current classroom routines and environments. Putnam and Borko (2000) argue that such goals may require teacher learning to be removed from teachers’ own classrooms, at least initially, because “the classroom is a powerful environment for shaping and constraining how teachers act” (p. 6). We did not want teachers’ existing classroom routines, assumptions, and curricula to inhibit their initial experiences with mathematical modelling .
At the same time, our understanding of mathematical modelling as an open, exploratory, and dynamic process meant that learning to engage children in mathematical modelling had to include opportunities for teachers to learn from their own practice. All classroom teaching is relational work (Lampert 2010), and modelling, which involves ongoing negotiations around the meaning and importance of contexts, assumptions, representations, and mathematical strategies, intensifies the relational work between and among the teacher and the students. Thus, it was essential to engage teachers in the process of reflecting on modelling from two perspectives: that of a student who is learning to model and also as a teacher teaching others to model.
To support both of these needs, we adopted a situated (e.g., Greeno 1997), social (Wenger 1998) perspective on teacher learning in which the teacher took on roles as both modeller and teacher. When viewing learning as situated, educators acknowledge the “interactive systems that are composed of groups of individuals together with the material and representational resources they use” (Cobb and Bowers 1999). Individuals within such systems learn by “engaging in and contributing to the practices of their communities” (Wenger 1998, p. 7). From these perspectives, designing professional development is less about finding ways to convey information, strategies, and practices to teachers and more about examining the contexts, activities, and interactions that may facilitate shifts in teachers’ perspectives, understandings, and classroom practices.
We established the following content and pedagogical goal for our professional development :
-
Teachers will understand what mathematical modelling is and the processes involved.
-
Teachers will experience modelling as learners of mathematics by investigating tasks relevant to their own experiences.
-
Teachers will experience different types of models and will understand that different models emphasize different values.
-
Teachers will relate mathematical modelling to other types of mathematical problems to analyse cognitive demand and attributes of the task.
-
Teachers will learn about and experience classrooms routines and structures they can use to facilitate the modelling process.
-
Teachers will develop modelling tasks that are mathematically appropriate for their students.
As highlighted in these goals, we wanted teachers to understand and experience the modelling process as learners and then use their understanding of modelling, coupled with pedagogical supports, to design and enact mathematically appropriate modelling tasks for their students.
7.2.1 Preparing Teachers as Modellers
In designing modelling tasks for teachers, we began with the phases in the modelling process (Borromeo Ferri 2006). To transition between these phases the following steps could be involved (1) Examining the situation and setting up a problem to be solved, (2) Identifying variables in the situation and selecting those that are essential, (3) Creating a model that best describes the relationships among the variables using geometric, graphical, tabular, algebraic, or statistical representations, (4) Formulating conclusions, (5) Interpreting the results for accuracy and relevance, (6) Refining the model through validating its potential to account for all relevant variables, (7) Testing model generalizability to other situations. However, we did not want teachers to see the modelling process as a pedagogical checklist where students simply needed to complete steps 1 through 7 to have ‘done’ mathematical modelling . We also wanted to give teachers opportunities to practice and experience broader features of mathematical modelling that stretch across the modelling cycle. We then identified four features of modelling practice that could be developed and used by novice, as well as experienced, modellers:
-
Wrestling with openness in modelling
-
Posing mathematical problems to address real world situations
-
Making choices creatively whilst modelling
-
Revisiting ideas and revising solutions during the modelling process
We believed that these features were unlikely to be a part of most teachers as learners’ experiences in teaching and learning mathematics.
Many of the mathematical tasks used in elementary schools are word problems—applications in which either the real world does not affect the problem or there is a clear solution strategy (Tran and Dougherty 2014; Zbiek and Conner 2006). In mathematical modelling , problems can be open at the beginning of the investigation, allowing modellers to ask different mathematical questions about a scenario, open in the middle as modellers investigate different solution strategies, and open at the end as modellers consider ways the models do, or do not, apply to other situations. Wrestling with openness in modelling is a feature that conveys the idea that real-world situations do not always have a single, clear-cut beginning, approach, or solution.
Posing mathematical problems to address real world situations involves determining if, and how, mathematics can be used to investigate issues originating from lived experiences. In school mathematics, students are usually asked to solve problems, but rarely asked to determine and articulate the range of mathematical problems they could pose. Problem posing is a central feature not only of mathematical modelling , but of mathematical activity in general and “can occur before, during, or after the solution of a problem” (Silver 1994). Although often neglected in school mathematics, problem posing is as important as problem solving (Cai et al. 2015; Kilpatrick 1987) and warranted explicit attention in professional development focused on modelling.
Many students believe that mathematics consists of fragmented bits of information transmitted from teachers or textbooks, and that students are not capable of “constructing mathematics knowledge and solving problems on their own” (Muis 2004, p. 329). To be successful modellers, students need experiences that challenge these perspectives and reveal mathematics as a creative enterprise, wherein problem solvers are empowered to make choices as they pursue solutions. Making choices creatively whilst modelling focuses on the ability to determine what mathematics the modeller will use or develop to make progress on a task. It empowers students as mathematical thinkers whose perspectives, ideas, and decisions matter, both to the ways problems are formulated and to the solutions pursued.
Finally, revisiting ideas and revising solutions during the modelling process involves stepping back and considering whether the solution (either in progress or complete) makes sense in light of the initial problem. In the context of teaching and learning, revisiting ideas and revising solutions also involves returning to both the contexts and mathematical content that played a critical role in previous modelling tasks and applying them to new situations.
Keeping our four features in mind, we designed five modelling tasks for teachers during professional development . When determining what tasks to pose, we considered that modelling is a challenging process that requires persistence and time. In designing tasks, we considered four pedagogical features:
-
Attributes of Modelling: What modelling practices (wrestling with openness in modelling, posing mathematical problems to address real world situations, making choices creatively whilst modelling, revisiting ideas and revising solutions during the modelling process) are made visible through this task?
-
Variation: Does this task highlight a particular type of model?
-
Access: Do the modellers have the appropriate mathematical tools to approach and solve this task?
-
Relevance: Will the modeller care about this problem or situation?
To highlight each of these pedagogical features, we will describe them through the Water Usage Task adapted from Hoffman (2014). The water usage task asks problem solvers to quantify the amount of water used to grow, process, and distribute the ingredients needed to make one slice of cheese pizza. We asked our participants, “How much water is needed to make pizza?”
Hoffman (2014) suggests a specific solution based on critical assumptions and decisions about the meaning of the situation and the purpose in asking the question. Our purpose in using the Water Usage Task was to introduce teachers to wrestling with openness in modelling. We wanted teachers to consider the notion that a problem could be approached from multiple perspectives and reinforce the notion that the perspective and knowledge of the modeller matters (English and Watters 2005). We also wanted teachers to see that perspective can cause variation in the types of models that are produced and that situations and questions exist in which more than one solution could make sense. We anticipated teachers could use a variety of mathematical tools, at different levels of complexity, to make sense of the situation and knew all of the teachers had experiences making and eating pizza.
7.2.2 Preparing Teachers to Teach Modelling
Using mathematical modelling to solve a problem is markedly different from supporting students as they work through a modelling task. Elsewhere, we described the work teachers must engage in as they develop and enact modelling tasks (Carlson et al. 2016). This work involves three teaching phases: developing the task and anticipating student strategies, enacting the task alongside students, and revisiting the task as opportunities arise. As the phases suggest, enacting modelling tasks with students involves many of the demanding teaching practices that have been associated with tasks that have high cognitive demand (Stein et al. 2000). Teachers must anticipate the mathematics students might use as they approach the task and anticipate mathematics students might find confusing or challenging. If the teachers plan for students to work in small groups, they must manage group work. During task enactment, teachers facilitate classroom discourse, selecting and sequencing student work that will be shared in a whole-group discussion. In addition, teachers must consider what contexts will be interesting and engaging for students and try to predict how students’ cultural and community-based “funds of knowledge” (e.g. González et al. 2001) give students access to, and agency within, the problem. In order to respond to the pedagogical demands of teaching mathematical modelling, we set aside time each day to focus on and develop teachers’ capacity in areas like differentiating classroom instruction, facilitating classroom discourse, and managing group work.
7.3 Theoretical Framework: Mathematical Modelling as a Community of Practice
As teachers translated their experiences in professional development into their own classrooms, we wondered how attributes of modelling and associated pedagogical practices might influence their classroom instruction. Our qualitative analyses suggested that modelling fostered outreach and empowerment across the classroom, school, and local communities. We looked to the communities of practice literature as a way to make sense of the communities that formed when teachers enacted modelling tasks as well as the attributes of modelling that seemed to promote community knowledge and ownership.
Wenger (1998) described a community of practice as a “simple social system” (p. 1). Participants in a community of practice exhibit certain competencies, including:
-
Understanding what matters, what the enterprise of the community is, and how it gives rise to a perspective on the world.
-
Being able (and allowed) to engage productively with others in the community.
-
Using appropriately the repertoire of resources that the community has accumulated through its history of learning. (p. 2)
Wenger went on to explain that communities of practice exist across broader systems that involve other communities.
In this chapter, we look across three teacher-developed modelling tasks to consider what attributes the teachers transitioned into practice that fostered mathematical modelling as a community practice. We address the following research questions:
-
1.
In what ways does mathematical modelling promote mathematical communities of practice in the elementary classroom?
-
2.
How do attributes of modelling and pedagogical practices help foster communities of practice as teachers engage elementary school students in mathematical modelling ?
7.4 Setting and Method
This investigation was part of a National Science Foundation-funded study aimed at examining mathematical modelling in the elementary and middle grades. Working alongside two other universities and three school districts, we engaged in a three-year, multi-state project. Our overarching goal was to provide and study the effects of professional development in mathematical modelling for grades Kindergarten to grade 8 (K–8) teachers. We developed and implemented a week-long, intensive summer professional development course, described above, as well as facilitated semester-long teacher study groups for 28 elementary grades teachers at a U.S. university in the Rocky Mountain West. We set up study groups of grade level teams and each teacher team implemented at least one modelling task. Each of the authors as well as two teacher leaders facilitated a teacher study group of 4–6 teachers during implementation of the modelling tasks. In this investigation, we draw on data collected from classrooms during teacher study groups.
7.4.1 Data Collection
The 28 teachers worked in their study groups with a facilitator to discuss, debrief, and modify modelling tasks designed during the summer professional development . These study groups met six times through the autumn school semester. We audio-recorded teacher-study group sessions and took notes describing the nature of the discussions. In addition, we visited classrooms as the tasks were enacted and wrote field notes following each day of implementation. Our main data sources were video and audio of classroom tasks and teacher study groups as well as first-hand observations of, and field notes taken during, implementation of the modelling tasks.
7.4.2 Data Analysis
We used narrative analysis (Clandinin and Connelly 2000; Riessman 2008) to examine the data, primarily by examining the modelling tasks themselves. We used communities of practice (Wenger 1998) as a guiding framework. Narrative analysis uses artefacts such as field notes and conversations to understand the ways in which meaning is created. Using our notes, paired with transcripts from the teacher study groups, we first sought to describe each modelling task, the rationale, and the teachers’ mathematical goals for the task. We found evidence that a majority of teachers used mathematical modelling tasks in their classrooms as a way to highlight community—classroom community, school community, or the civic community. Next, we considered the ways in which students worked through the task and decisions teachers made as students worked. We looked for ways, both in the design and in the enactment of the task, that modelling fostered community across the tasks and how this occurred. From this analysis, we constructed rich descriptions of the modelling tasks and teacher accounts.
As we analysed each of the modelling tasks, we looked for these three features of communities of practice :
-
Relevance: Understanding what matters, what the enterprise of the community is, and how it gives rise to a perspective on the world (Wenger 1998, p. 2). We looked for ways that the modelling task was meaningful to the modeller.
-
Engagement: Being able (and allowed) to engage productively with others in the community (Wenger 1998, p. 2). We looked for ways that multiple modellers’ perspectives were heard, considered, and valued.
-
Access: Using appropriately the repertoire of resources that the community has accumulated through its history of learning (Wenger 1998, p. 2). We looked for ways that all modellers in the class could make contributions to the creation of a model.
To help answer the second research question, we also analysed across modelling tasks considering how these attributes fostered modelling as a community of mathematical practice.
7.5 Results
In this section, we chronicle three different modelling tasks designed by teacher teams selected on the basis that they demonstrate fostering of classroom community (Lunch Planning Task ), school community (Pizza Party Task ), and the civic community (City Park Ice Rink Design Task ) and discuss how the themes of relevance, engagement, and access emerged in each lesson and how these themes fostered modelling as a community endeavour.
7.5.1 The Lunch Planning Task
Third and fourth-grade teachers collaborated on the Lunch Planning Task . The purpose of the task was for students to design a lunch, within budget, for several classes to enjoy and use as a team-building experience. Students discussed that they needed to determine what to serve, how much food to order, and what the cost of the lunch would be. In addition, since the focus was on team building, several of the classes also researched how to spend their time during the lunch and what activities they could organize that would help to build community within the classroom.
The teachers worked with students on this task over the span of four weeks, addressing the task a few times each week. As the students worked on the task, the teachers allowed students to choose which portions of the task they wanted to help with. They described that all parts of the task were accessible to someone in the classroom. Initially, the students worked on determining what should be served at lunch and the quantity of food they should order. Students primarily used surveys, multiplication, counting, and measurement as mathematical tools to aid them in making decisions. Once students determined what should be served and how much they would need to order, they needed to determine where the food would come from and if the meal was in budget. The teachers helped by providing grocery store and restaurant advertisements, and students used multiplication and repeated addition in determining the total cost.
In planning the activity, the teachers discussed that the community lunch planning happens every year, but the teachers usually take on the responsibility of designing and planning the lunch. Since students usually enjoy the lunch and make suggestions on what should happen, they determined that the students would find the task relevant. As students worked on the task, the teachers commented that it promoted excitement, motivation, and perseverance because students felt they were given ownership of an important decision. In describing relevance, one teacher stated:
I would also really encourage them [other teachers] to think about not just problems outside of school, like building a house or something but problems that are more real to the students. For fourth-grade, that has been really successful, you know? Our lunch was really successful and really motivating. There is no work I have to do, you know, no encouragement. We just started the process…and they were really excited. They knew what was going on. [It’s important] to attack problem that they have some sort of connection to.
Engagement emerged quickly in this modelling task. When determining what lunch to serve, students had opinions on which meal was their favourite and why, however, they found that their opinions varied greatly. The students realized that 50 different meals was not a cost-effective model. Teachers encouraged students to consider how to best hear and acknowledge everyone’s perspective, which eventually resulted in learning about surveying and implementing surveys in order to hear from all students. Even after a decision was made, according to the survey, students asked if it was fair to serve a meal that a few people did not like or could not eat. The teacher encouraged them to go back and consider these perspectives as they were making choices in the modelling process. For example, after pizza was chosen as the most popular meal, students decided they needed to find a solution for several lactose-intolerant students.
In describing the Lunch Planning Task , one teacher described that it was one of the few times where all students could feel included and access the mathematics together. She stated:
The most amazing thing to me is that everybody is able, no matter who you are, can enter the process where you need to enter it. I just, my entire life, as a person, I have always had a hard time not including everyone and not having everyone feel like they are valued or important. And, I’ve, when I decided to become a teacher, as much as we like to think public education is inclusive, it’s not. We have groups, pullouts and things because we need to service everybody. I totally understand, but it has always made me a little uncomfortable because I see the dynamics because of that. Roles are created…It’s just reality. This was the first time that I had that “aha” moment in the class this summer when we were reading those articles. I was like ohhh, if this is how math could be in my classroom where everyone was doing math and didn’t have a status role so to speak as the really smart math kid or the not so smart kid. If we all just had a part in this, that was totally mind-blowing for me. I got so excited.
The Lunch Planning Task promoted a community of practice through relevance, engagement, and access. Students found the task relevant because they were allowed to assume ownership of a classroom activity usually reserved for the teacher to design and enact on her own. Engagement was evident as the students wanted to make sure the model satisfied their wants and needs as a classroom. All students were able to access the task and share solutions because the teachers carefully thought about the mathematics involved in the task in relation to their grade level.
7.5.2 The Pizza Party Task
The Pizza Party Task involved first-grade and fifth-grade students. Traditionally, first-grade students (age 6–7) at the school have a pizza party on the last day of school and this year they wanted to invite their fifth-grade (age 10–11) “buddies” to join.
Similar to the Lunch Planning Task , this task was relevant to both ages of students and the teachers discussed the importance of relevance in motivating students. They also discussed how many mathematics problems they typically work on that are set in the “real-world” but the choices students make in solving the problem do not actually mean anything in the context of the problem. One teacher addressed relevance this way:
The real worldliness of what we were doing was key. Because a lot of math that I teach on a daily basis I feel like has no connection to the real world. I mean maybe you can stretch it where we are talking about candy or in a story problem dividing it up, but it kind of loses something because it’s not connected to a real-world thing that means something to the kids… The fact that they were actually going to get food at the end was important. It was engaging.
Engagement emerged in several different ways. First, the teachers did not reserve all of the mathematics for the older students. Instead, they allowed both age groups to be experts in their own right and describe solutions to parts of the problem to each other. Second, echoing what we found in the Lunch Planning Task , the students had to understand the wants and needs of one another as they ordered pizza.
Concerning access, the teachers were very thoughtful about considering how a modelling task could have multiple questions that could be approached in an appropriate way by students at varying grade levels. For example, the fifth-grade class determined where to buy the pizza by considering the area of the pizzas in relation to their size and price. They presented their findings to the first-graders, identifying the place that they had determined was the best, showing them the size of the pizza slices, and explaining the topping choices for a particular cost. The first-graders addressed a different question by determining how many of each pizza to buy and what kind. The first-graders also constructed surveys to gather data about how many pieces of each type of pizza each student wanted and determined how many pizzas they should order for their class. Using repeated addition, they extrapolated from their class to all of the classes who would be at the party. They concluded the task by presenting how many pizzas and what type should be ordered. Although different ages, each class was able to contribute to the overarching question in a way that was appropriate for their mathematical understanding.
The Pizza Party Task promoted a mathematical community of practice across grade levels through relevance, access, and engagement. In this case, students found the task relevant because the task was real and the choices they made had consequences that mattered to them. In terms of access, the teachers divided the task so that students, who varied in age by four years, could answer a question at their appropriate level of mathematical understanding. Lastly, engagement emerged as the students, across grade-levels, were able to take ownership and expertise of the task and share their solutions to parts of the problem.
7.5.3 City Park Ice Rink Design Task
The City Park Ice Rink Design Task emerged from fifth-grade students’ discussion about the use of an ice rink at City Park. The students felt that the area currently designated for the skating rink was not being used appropriately. From their perspective, the hockey space was too small, causing hockey players to enter the free-skate area. Students who enjoyed free-skating felt unsafe because of the hockey equipment, and students who enjoyed hockey felt they did not have enough space to play. The teacher commented that she heard the students complain about the use of the park on a daily basis and felt it would have relevance for her students to explore the use of the park. She stated:
We have a local park that they all use and they all talk about how the use of it isn’t always appropriate, or appropriate as they see it as ten-year olds. So my thought was that we could explore the uses and see why the uses aren’t equitable….I think we can use it [Google Earth]. That’s part of the discovery that I really want them to be thinking about. How are you going to figure out how big the park is? How are you going to measure and do that?
Unlike the other two tasks we chronicled, the teacher described that for some students, lack of relevance impacted students’ perseverance and interest in the problem. Several of the students were engaged in the task and solving the problem but a few seemed to lose interest because they did not use the park nor did they like ice-skating.
The teacher worked carefully to consider how students would access the task, examining what mathematics would be addressed in the activity and how it was connected to grade-level standards. Because students were studying area measurement, she wanted them to investigate the area of the skating rink and how they might fairly partition it for different types of skaters. During the task, the students found the area of the current skating space using tools like Google Earth and then researched appropriate areas for skating activities.
Engagement was represented in a different way in this task. The City Park Ice Rink Design Task intersects with the broader community. When students proposed changes, the teachers and students could not actually enact them. The teacher proposed that they first present and listen to each other’s solutions to determine which model might be the best overall. After determining which solution might be best, the students were asked to voice their opinion to the town hall through a letter. The students were asked to describe the problem and the mathematical process they went through in determining a solution. Through this process, the students were given a voice in a larger community space. The teacher stated:
They use that park all the time. It is very much part of their daily lives… Even if the city doesn’t do anything, they have been able to voice their opinion in a constructive way with evidence, which is so important.
The City Park Ice Rink Design Task promoted a mathematical community of practice across grade levels through relevance, access, and engagement. In this case, many students found the task relevant because they cared about the city park and ice rink usage. The task also highlights that if the modelling task is not relevant to some students then they may not fully participate in the task. In terms of access, the teacher thought carefully about the mathematics involved in the task and how it aligned with grade level standards. Lastly, engagement emerged as the students were able to describe their solutions both inside their classroom and in the broader community.
7.5.4 Looking Across Tasks
Looking across the tasks, we found that the elements of communities of practice emerge in different ways. Relevance is evident where a community of practice has a shared vision or purpose and understands its role in relation to the greater community. Also, when problems had relevance to the students, this made the problem important and motivating for students to work to solve the task. Relevance was apparent in these modelling tasks when students would take on a coveted responsibility, take action toward an important problem, or work toward a goal that everyone valued.
Access is apparent when a community of practice draws on their shared experience and history of learning. In each of these tasks, the teachers thought carefully about the mathematics the students might use in the task to make sure everyone could contribute in a way that made sense to them. The Pizza Party Task highlights that the same modelling task could be approached using different questions and content knowledge to address the task in an appropriate way.
Engagement is perceptible when all members of a community of practice have the right and ability to be heard. Teachers fostered this in several ways as they implemented modelling tasks. First, the openness of the task and the variety of tasks embedded in one project allowed for students to solve the Lunch Planning Task in multiple ways. In the Pizza Party Task , teachers designated tasks to different grade levels to provide engagement by, and expertise to, all. Communities of practice acknowledge that multiple communities and spaces exist together at once and, within the City Park Ice Rink Design Task , the teacher helped students to transition their engagement to a community that encompassed the local town.
7.6 Discussion and Implications
In this research, we asked in what ways mathematical modelling promotes mathematical communities of practice in the elementary classroom and how attributes of modelling help foster communities of practice as teachers engage elementary school students in mathematical modelling. Our narrative analysis of the tasks designed and taught by teachers provides a chronicle of how teachers use the relevance of a task to build communities and how the nature of modelling allows for multiple access points and student engagement.
We chose tasks for teachers to engage in during professional development that were intended to help teachers experience and reflect on learning opportunities made available through mathematical modelling : (1) wrestling with openness in modelling; (2) posing mathematical problems to address real world situations; (3) making choices creatively whilst modelling; and (4) revisiting ideas and revising solutions during the modelling process. As a stance, the professional development reinforced the nature of mathematical modelling as a tool to solve problems important to the community and enacted by a community of learners. In the teacher study groups, teachers worked together to create tasks for their students that would exhibit these features. We found that the tasks teachers enacted in their classrooms promoted classroom, school, and civic community through mathematical modelling. In addition, we know that teaching mathematical modelling is demanding work and that teachers must coordinate knowledge of mathematical and pedagogical content knowledge to facilitate a modelling task (Blum 2011, 2015; Doerr and Lesh 2011; Zawojewski et al. 2003). Teachers saw opportunities to motivate mathematical thinking and perseverance by engaging students in solving problems that made a difference for themselves and for others.
In our professional development , we created opportunities for teachers to experience being modellers, to engage in relevant tasks, to work together as a community of learners, and to be thoughtful practitioners in teaching mathematical modelling . In this combination of goals in professional development, we framed how teachers saw community as an important component of modelling, which they then extended to their own tasks. We are interested to notice that the teachers and their students made the most of these opportunities to make social connections and to use modelling to find ways to make decisions that were for the good of the group. In the Lunch Planning Task , students tackled a task they understood and felt connected to. Their teachers, in that case, recognized the power of students using mathematics in ways that directly impact their own classrooms. In the Pizza Party Task, students investigated and analysed a situation that fostered connections between themselves and other students. In the City Park Ice Rink Design Task , students considered the role mathematics can play not only in solving problems, but in developing convincing arguments that can be shared with civic leaders. One teacher gave insight about why this might be when discussing a community-based task in her classroom of second-graders by saying, “[students] are really interested in these ideas and problem solving these big things. They don’t feel incapable because they are young. I want them to understand that their work, and their work through math, has an impact.”
7.7 Conclusion
The teachers responded to our conception of modelling as a tool used to solve relevant problems. They created tasks for their students that would engage students as modellers in using mathematics to solve problems that mattered to them.
Our approach to the mathematical modelling professional development was one in which we relied on a community of practice (Wenger 1998). This message about modelling, and how best to accomplish the teaching of modelling among those unfamiliar with it, may have permeated teachers’ notions about how to teach modelling. It might also be that in their search for contexts with meaningful problems that lend themselves to classroom modelling, teachers found that school students were most interested in pursuing problems that affect themselves.
This narrative analysis leaves us with several lines of inquiry open to investigation. Why do teachers gravitate towards opportunities to model problems directly affecting community? Is there something inherent in the way we defined mathematical modelling in classrooms—as solving a problem that is based in authentic, lived experiences—that leads to tasks based in community improvement?
The way we approached modelling as an activity that takes place within a community of practice involved classroom practices of discourse, including making compromises and active listening. Does modelling in classrooms amplify this sense of negotiation and agreement because problems begin and remain open? Critical to modelling in elementary grades is the teacher facilitating community agreements about modelling decisions so that the class can move forward together in productive work (Carlson et al. 2016). It is not practical to think that in elementary grades teachers can let students pursue individual solutions to modelling problems. This means modelling in an elementary classroom requires the teacher to facilitate community agreement and progress in a way that is not inherent to modelling outside of classrooms.
Finally, we remain interested in the affordances and limitations of mathematical modelling in elementary grades, especially viewed in light of an already demanding elementary school curriculum. Our selection of modelling tasks in the professional development was a careful balance of mathematical topics we wanted to engage teachers in and modelling practices and values we wanted to expose them to. What kinds of mathematical decisions do teachers make when pursuing modelling opportunities for students? Do they hold high standards for mathematical work? There can be much classroom activity when students are engaged in solving problems with modelling, but how can teachers keep the activity focused on mathematical learning? Does inserting modelling in elementary curriculum sacrifice curricular coherence?
Mathematical modelling in elementary grade classrooms in the USA is a new arena—new to practice and new to research. We continue to pursue an understanding of how to best support teachers in their enactment of mathematical modelling .
References
Blum W. (2011). Can modelling be taught and learnt? Some answers from empirical research. In G. Kaiser, W. Blum., R. Borromeo Ferri, & G. Stillman (Eds.), Trends in teaching and learning of mathematical modelling (pp. 15–30). Dordrecht: Springer.
Blum W. (2015). Quality teaching of mathematical modeling: What do we know, what can we do? In S. Cho (Ed.), The Proceedings of the 12th International Congress on Mathematical Education (pp. 73–96). Cham: Springer.
Borromeo Ferri, R. (2006). Theoretical and empirical differentiations of phases in the modeling process. ZDM Mathematics Education, 38(2), 86–95.
Brown, J. P. (2013). Inducting Year 6 students into “A culture of mathematising as a practice”. In G. A. Stillman, G. Kaiser, W. Blum, & J. P. Brown (Eds.), Teaching mathematical modelling: Connecting to research and practice (pp. 295–305). Dordrecht: Springer.
Cai, J., Hwang, S., Jiang, C., & Silber, S. (2015). Problem-posing research in mathematics education: Some answered and unanswered questions. In F. Singer, N. F. Ellerton, & J. Cai (Eds.), Mathematical problem posing research in mathematics education (pp. 3–34). New York, NY: Springer.
Carlson, M. A., Wickstrom, M. H., Burroughs, E. A., & Fulton, E. W. (2016). A case for mathematical modeling in the elementary school classroom. In C. R. Hirsch & A. R. McDuffie (Eds.), Mathematical modeling and modeling mathematics (pp. 121–129). Reston, VA: National Council of Teachers of Mathematics.
Clandinin, D. J., & Connelly, F. M. (2000). Narrative inquiry: Experience and story in qualitative research. San Francisco, CA: Jossey-Bass.
Cobb, P., & Bowers, J. (1999). Cognitive and situated learning perspectives in theory and practice. Educational Researcher, 28(2), 4–15.
Doerr, H. M. (2007). What knowledge do teachers need for teaching mathematics through applications and modelling? In W. Blum, P. L. Galbraith, H. W. Henn, & M. Niss (Eds.), Modelling and applications in mathematics education. New ICMI Study Series (Vol. 10, pp. 69–78). New York, NY: Springer.
Doerr, H. M., & English, L. D. (2006). Middle grade teachers’ learning through students’ engagement with modeling tasks. Journal of Mathematics Teacher Education, 9(1), 5–32.
Doerr, H. M., & Lesh, R. (2011). Models and modelling perspectives on teaching and learning mathematics in the twenty-first century. In G. Kaiser, W. Blum, R. Borromeo Ferri, & G. Stillman (Eds.), Trends in teaching and learning of mathematical modelling (pp. 247–268). Dordrecht: Springer.
English, L. (2004). Mathematical modelling in the primary school. In I. Putt, R. Faragher, & M. McLean (Eds.), Mathematics education for the third millennium: Towards 2010 (pp. 207–214). Adelaide: Mathematics Education Research Group of Australasia.
English, L. D. (2006). Mathematical modeling in the primary school: Children’s construction of a consumer guide. Educational Studies in Mathematics, 63(3), 303–323.
English, L. D., & Watters, J. J. (2005). Mathematical modelling in the early school years. Mathematics Education Research Journal, 16(3), 58–79.
Garet, M. S., Porter, A. C., Desimone, L., Birman, B. F., & Yoon, K. S. (2001). What makes professional development effective? Results from a national sample of teachers. American Educational Research Journal, 38(4), 915–945.
González, N., Andrade, R., Civil, M., & Moll, L. (2001). Bridging funds of distributed knowledge: Creating zones of practices in mathematics. Journal of Education for Students Placed at Risk, 6(1–2), 115–132.
Greeno, J. G. (1997). On claims that answer the wrong questions. Educational Researcher, 26(1), 5–17.
Hawley, W. D., & Valli, L. (1999). The essentials of effective professional development: A new consensus. In L. Darling-Hammond & G. Sykes (Eds.), Teaching as the learning profession (pp. 127–159). San Francisco, CA: Jossey-Bass.
Hoffman, B. (2014). 42 Gallons of water to make one slice of pizza, and other facts we need to know. Forbes. https://www.forbes.com/sites/bethhoffman/2014/02/03/42-gallons-of-water-to-make-one-slice-of-pizza-and-other-facts-we-need-to-know/#478f39156fe9.
Kilpatrick, J. (1987). Problem formulating: Where do good problems come from? In A. H. Schoenfeld (Ed.), Cognitive science and mathematics education (pp. 123–147). New York: Routledge.
Koellner-Clark, K., & Lesh, R. (2003). A modeling approach to describe teacher knowledge. In R. Lesh & H. M. Doerr (Eds.), Beyond constructivism: Models and modeling perspectives on mathematics problem solving, learning, and teaching (pp. 159–174). Mahwah, NJ: Erlbaum.
Lampert, M. (2010). Learning teaching in, from, and for practice: What do we mean? Journal of Teacher Education, 61(1–2), 21–34.
Lesh, R., & Doerr, H. M. (Eds.). (2003). Beyond constructivism: A models & modeling perspective on mathematics problem solving, learning & teaching. Mahwah, NJ: Erlbaum.
Muis, K. (2004). Personal epistemology and mathematics: A critical review and synthesis of research. Review of Educational Research, 74(3), 317–377.
National Council of Teachers of Mathematics. (2000). Principles and standards for school mathematics. Reston, VA: National Council of Teachers of Mathematics.
National Governors Association Center for Best Practices & Council of Chief State School Officers. (2010). Common core standards for mathematics. Washington, DC: National Governors Association Center for Best Practices & Council of Chief State School Officers.
Niss, M., Blum, W., & Galbraith, P. (2007). Introduction. In W. Blum, P. L. Galbraith, H.-W. Henn, & M. Niss (Eds.), Modelling and applications in mathematics education: The 14th ICMI study (pp. 1–32). New York: Springer.
Putnam, R. T., & Borko, H. (2000). What do new views of knowledge and thinking have to say about research on teacher learning? Educational Researcher, 29(1), 4–15.
Riessman, C. K. (2008). Narrative methods for the human sciences. Thousand Oaks, CA: Sage.
Silver, E. (1994). On mathematical problem posing. For the Learning of Mathematics, 14(1), 19–28. Retrieved from http://www.jstor.org/stable/40248099.
Smith, M. S., & Stein, M. K. (2001). Five practices for orchestrating productive mathematics discussion. Reston, VA: National Council of Teachers of Mathematics.
Stein, M. K., Smith, M. S., Henningsen, M. A., & Silver, E. A. (2000). Implementing standards-based mathematics instruction: A casebook for professional development. Columbia University: Teachers College Press.
Tran, D., & Dougherty, B. J. (2014). Authenticity of mathematical modeling. Mathematics Teacher, 107(9), 672–678.
Wenger, E. (1998). Communities of practice: Learning, meaning, and identity. New York, NY: Cambridge University Press.
Zawojewski, J. S., Lesh, R., & English, L. (2003). A models and modeling perspectives on the role of small group learning activities. In R. A. Lesh & H. Doerr (Eds.), Beyond constructivism: Models and modeling perspectives on mathematics problem solving, learning, and teaching (pp. 337–358). Mahwah, NJ: Erlbaum.
Zbiek, R. M., & Conner, A. (2006). Beyond motivation: Exploring mathematical modeling as a context for deepening students’ understandings of curricular mathematics. Educational Studies in Mathematics, 63(1), 89–112.
Acknowledgements
This material is based upon work supported by the National Science Foundation under Grant No. 1441024.
Author information
Authors and Affiliations
Corresponding author
Editor information
Editors and Affiliations
Rights and permissions
Open Access This chapter is licensed under the terms of the Creative Commons Attribution 4.0 International License (http://creativecommons.org/licenses/by/4.0/), which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license and indicate if changes were made.
The images or other third party material in this chapter are included in the chapter's Creative Commons license, unless indicated otherwise in a credit line to the material. If material is not included in the chapter's Creative Commons license and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder.
Copyright information
© 2019 The Author(s)
About this chapter
Cite this chapter
Fulton, E.W., Wickstrom, M.H., Carlson, M.A., Burroughs, E.A. (2019). Teachers as Learners: Engaging Communities of Learners in Mathematical Modelling Through Professional Development. In: Stillman, G., Brown, J. (eds) Lines of Inquiry in Mathematical Modelling Research in Education. ICME-13 Monographs. Springer, Cham. https://doi.org/10.1007/978-3-030-14931-4_7
Download citation
DOI: https://doi.org/10.1007/978-3-030-14931-4_7
Published:
Publisher Name: Springer, Cham
Print ISBN: 978-3-030-14930-7
Online ISBN: 978-3-030-14931-4
eBook Packages: EducationEducation (R0)