Skip to main content
SpringerLink
Log in

A Piecewise Memory Principle for Fractional Derivatives

  • Research Paper
  • Published:
Fractional Calculus and Applied Analysis Aims and scope Submit manuscript

Abstract

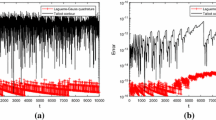
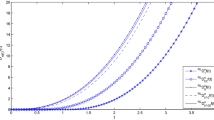
In the numerical approximation of fractional order derivatives, the crucial point is to balance the computing complexity and the computing accuracy. We proposed a piecewise memory principle for fractional derivatives, in which the past history is divided into several segments instead of discarded. The piecewise approximation is performed on each segment. Error estimation of piecewise memory principle is analyzed also. Numerical examples show that the contradiction of computing accuracy and complexity is effectively relaxed and the piecewise memory principle is superior to the existing short, variable and equal-weight memory principles. The impacts of the memory length, step size and segment size are also discussed.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. A. Bhrawy, Y. Alhamed, D. Baleanu, A. Al-Zahrani, New spectral techniques for systems of fractional differential equations using fractional-order generalized Laguerre orthogonal functions. Fract. Calc. Appl. Anal. 17, No 4 (2014), 1137–1157; 10.2478/s13540-014-0218-9; https://www.degruyter.com/view/j/fca.2014.17.issue-4/issue-files/fca.2014.17.issue-4.xml.

    Article  MathSciNet  Google Scholar 

  2. T. Blaszczyk, M. Ciesielski, Numerical solution of fractional Sturm-Liouville equation in integral form. Fract. Calc. Appl. Anal. 17, No 2 (2014), 307–320; 10.2478/s13540-014-0170-8; https://www.degruyter.com/view/j/fca.2014.17.issue-2/issue-files/fca.2014.17.issue-2.xml.

    Article  MathSciNet  Google Scholar 

  3. H. Cao, X. Li, Z. Deng, Y. Qin, Control-oriented fast numerical approaches of fractional-order models. Control Theory & Applications (in Chinese) 28 (2011), 715–721.

    Google Scholar 

  4. W. Deng, Short memory principle and a predictor–corrector approach for fractional differential equations. J. of Computational and Applied Mathematics. 206, No 1 (2007), 174–188; 10.1016/j.cam.2006.06.008.

    Article  MathSciNet  Google Scholar 

  5. K. Diethelm, An efficient parallel algorithm for the numerical solution of fractional differential equations. Fract. Calc. Appl. Anal. 14, No 3 (2011), 475–490; 10.2478/s13540-011-0029-1; https://www.degruyter.com/view/j/fca.2011.14.issue-3/issue-files/fca.2011.14.issue-3.xml.

    Article  MathSciNet  Google Scholar 

  6. H. Ding, C. Li, Y. Chen, High-order algorithms for Riesz derivative and their applications (ii). J. of Computational Physics. 293 (2015), 218–237; 10.1016/j.jcp.2014.06.007.

    Article  MathSciNet  Google Scholar 

  7. X. Ding, J. Nieto, Analytical solutions for the multi-term time-space fractional reaction-diffusion equations on an infinite domain. Fract. Calc. Appl. Anal. 18, No 3 (2015), 697–716; 10.1515/fca-2015-0043; https://www.degruyter.com/view/j/fca.2015.18.issue-3/issue-files/fca.2015.18.issue-3.xml.

    Article  MathSciNet  Google Scholar 

  8. N. Ford, A. Simpson, The numerical solution of fractional differential equations: Speed versus accuracy. Numerical Algorithms 26 (2001), 333–346; 10.1023/A:1016601312158.

    Article  MathSciNet  Google Scholar 

  9. C. Gong, W. Bao, G. Tang, A parallel algorithm for the Riesz fractional reaction-diffusion equation with explicit finite difference method. Fract. Calc. Appl. Anal. 16, No 3 (2013), 654–669; 10.2478/s13540-013-0041-8; https://www.degruyter.com/view/j/fca.2013.16.issue-3/issue-files/fca.2013.16.issue-3.xml.

    Article  MathSciNet  Google Scholar 

  10. C. Gong, W. Bao, G. Tang, B. Yang, J. Liu, An efficient parallel solution for Caputo fractional reaction-diffusion equation. J. of Supercomputing 68, No 3 (2014), 1521–1537; 10.1007/s11227-014-1123-z.

    Article  Google Scholar 

  11. C. Gong, W. Bao, G. Tang, Y. Jiang, J. Liu, Computational challenge of fractional differential equations and the potential solutions: A survey. Mathematical Problems in Engineering 2015 (2015), Art. # 258265; 10.1155/2015/258265.

  12. C. Gong, J. Liu, L. Chi, H. Huang, J. Fang, Z. Gong, GPU accelerated simulations of 3D deterministic particle transport using discrete ordinates method. J. of Computational Physics 230, No 15 (2011), 6010–6022; 10.1016/j.jcp.2011.04.010.

    Article  Google Scholar 

  13. H. Jiang, F. Liu, I. Turner, K. Burrage, Analytical solutions for the multi-term time-fractional diffusion-wave/diffusion equations in a finite domain. Computers & Math. with Appl. 64, No 10 (2012), 3377–3388; 10.1016/j.camwa.2012.02.042.

    Article  MathSciNet  Google Scholar 

  14. V. Kiryakova, A brief story about the operators of the generalized fractional calculus. Fract. Calc. Appl. Anal. 11, No 2 (2008), 203–220; at http://www.math.bas.bg/~fcaa.

    MathSciNet  MATH  Google Scholar 

  15. C. Li, Numerical Methods for Fractional Calculus. CRC Press (2015).

    Book  Google Scholar 

  16. C. Li, F. Zeng, F. Liu, Spectral approximations to the fractional integral and derivative. Fract. Calc. Appl. Anal. 15, No 3 (2012), 383–406; 10.2478/s13540-012-0028-x; https://www.degruyter.com/view/j/fca.2012.15.issue-3/issue-files/fca.2012.15.issue-3.xml.

    Article  MathSciNet  Google Scholar 

  17. H.-l. Liao, Y.-n. Zhang, Y. Zhao, H.-s. Shi, Stability and convergence of modified Du Fort–Frankel schemes for solving time-fractional subdiffusion equations. J. of Scientific Computing 61, No 3 (2014), 629–648; 10.1007/s10915-014-9841-1.

    Article  MathSciNet  Google Scholar 

  18. L. Liu, L. Zheng, X. Zhang, Fractional anomalous diffusion with cattaneoCchristov flux effects in a comb-like structure. Applied Mathematical Modelling 40, No 13C-14 (2016), 6663–6675; 10.1016/j.apm.2016.02.013.

    Article  MathSciNet  Google Scholar 

  19. C. Lubich, Discretized fractional calculus. SIAM J. on Math. Anal. 17, No 3 (1986), 704–719; 10.1137/0517050.

    Article  MathSciNet  Google Scholar 

  20. J. T. Machado, V. Kiryakova, F. Mainardi, Recent history of fractional calculus. Commun. in Nonlinear Sci. and Numer. Simul. 16, No 3 (2011), 1140–1153; 10.1016/j.cnsns.2010.05.027.

    Article  MathSciNet  Google Scholar 

  21. I. Podlubny, Fractional Differential Equations. Academic Press, San Diego, CA (1999).

  22. P. W. Stokes, B. Philippa, W. Read, R. D. White, Efficient numerical solution of the time fractional diffusion equation by mapping from its Brownian counterpart. J. of Comput. Phys. 282 (2015), 334–344; 10.1016/j.jcp.2014.11.023.

    Article  MathSciNet  Google Scholar 

  23. Y. Xu, Z. He, The short memory principle for solving abel differential equation of fractional order. Computers & Math. with Appl. 62, No 12 (2011), 4796–4805; 10.1016/j.camwa.2011.10.071.

    Article  MathSciNet  Google Scholar 

  24. F. Yin, T. Tian, J. Song, M. Zhu, Spectral methods using legendre wavelets for nonlinear Klein Sine-Gordon equations. J. of Comput. & Appl. Math. 275 (2015), 321–334; 10.1016/j.cam.2014.07.014.

    Article  MathSciNet  Google Scholar 

  25. F. Yin, J. Song, F. Lu, A coupled method of Laplace transform and Legendre wavelets for nonlinear Klein-Gordon equations. Mathematical Methods in Appl. Sci. 37, No 6 (2014), 781–792; 10.1002/mma.2834.

    Article  MathSciNet  Google Scholar 

  26. Q. Zeng, Research on fractional-order control systems and its application to molten carbonate fuel cell (in Chinese). Ph.D. Thesis, Shanghai Jiao Tong University (2008).

    Google Scholar 

  27. X. Zhao, Y. Chen, H. Zhang, Y. Zhang, T. K. Sarkar, A new decomposition solver for complex electromagnetic problems [EM Programmer’s Notebook]. IEEE Antennas and Propagation Magazine 59, No 3 (2017), 131–140; 10.1109/MAP.2017.2687119.

    Article  Google Scholar 

  28. P. Zhuang, F. Liu, V. Anh, I. Turner, Numerical methods for the variable-order fractional advection-diffusion equation with a nonlinear source term. SIAM J. on Numer. Anal. 47, No 3 (2009), 1760–1781; 10.1137/080730597.

    Article  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. School of Computer Science, National University of Defense Technology, Changsha, 410073, China

    Chunye Gong & Jie Liu

  2. Science and Technology on Space Physics Laboratory, Beijing, 100076, China

    Chunye Gong & Weimin Bao

  3. College of Aerospace Science and Engineering, National University of Defense Technology, Changsha, 410073, China

    Chunye Gong & Weimin Bao

Authors
  1. Chunye Gong
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Weimin Bao
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Jie Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Chunye Gong.

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Gong, C., Bao, W. & Liu, J. A Piecewise Memory Principle for Fractional Derivatives. FCAA 20, 1010–1022 (2017). https://doi.org/10.1515/fca-2017-0052

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1515/fca-2017-0052

MSC 2010

Key Words and Phrases

Search

Navigation