Abstract
The prestressed hollow-core slab (PHCS) is a precast concrete member that can maximize productivity and structural performance efficiency of concrete cross-section. For the PHCS members produced by extrusion method, however, it is difficult to provide the shear reinforcement due to its unique production method to form the hollow-cores in concrete section. The recently revised ACI318 Building Code Requirements stipulate that web-shear capacity of thick hollow-core member over 315 mm depth without the minimum shear reinforcement should be reduced in half, which may result in an excessively conservative shear design for the PHCS member compared to conventional prestressed concrete members with thin webs. In this study, shear tests on PHCS members, which have typical thicknesses widely used in precast construction industry, were conducted, and a large number of shear test data were additionally collected from previous studies to evaluate the current shear design criteria for PHCS in detail.
Similar content being viewed by others
1 Background
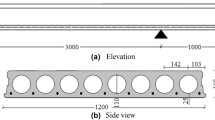
The prestressed hollow-core slabs (PHCS) have multiple hollows in concrete web, as shown in Fig. 1, which enables to save concrete materials and to be lightweight. Since the PHCS members have excellent flexural performances by introducing prestress into concrete, they have been widely used in precast industry as a lightweight floor system. In addition, the PHCS members are produced in precast plants by a mass production method, which is very advantageous for quality control in comparison with conventional cast-in-place concrete members. For these reasons, in Europe and North America, the PHCS has been commonly used for the construction of offices, apartments, parking structures, etc. since the 1950s, and its commercial demands also continue to increase in international markets (Precast/Prestressed Concrete Institute 1998; Pajari 2009; Palmer and Schultz 2010, 2011). The PHCS members are generally produced by the dry-cast method or the high-slump concrete method. In the high-slump concrete method, PHCS are cast by using relatively high-slump concrete in a slip-forming machine, in which shear reinforcement can be placed. However, this type of fabrication method generally needs many labors for formworks, and the size of hollow-cores is relatively small because of its weak compaction performance. On the contrary, as shown in Fig. 2, the dry-cast method, so-called the extrusion method, uses very low slump concrete, and an extruder machine that compacts concrete simultaneously with extruding concrete along the casting bed. Thus, it needs no formwork, and can significantly increase the size of hollow-cores in the concrete section, which is very advantageous for material saving and weight reduction of the products. Due to its excellent productivity, the PHCS members have been produced mostly by the extrusion method in Asia, Europe, and North America. The unique production characteristics of the extrusion method, however, make it difficult to place shear reinforcement in the PHCS members, and the effective prestress (\(f_{se}\)) is not fully developed within the transfer length near the end regions of thin-webbed PHCS members. Thus, the web-shear strength (\(V_{cw}\)) of PHCS member needs to be checked in detail.
In recent years, Hawkins and Ghosh (2006) raised a concern that the web-shear capacity of the thick PHCS members exceeding 315 mm in the member depth (\(h\)) can be unconservatively estimated by ACI318 code provisions (ACI Committee 318 2005). For this reason, it had been stipulated in ACI318-08 code (ACI Committee 318 2008) that the minimum shear reinforcement should be provided in hollow-core slabs with untopped depth over 315 mm when factored shear force exceeds \(0.5\phi V_{cw}\), where \(\phi\) is the strength reduction factor for shear. This means that the web-shear capacity (\(V_{cw}\)) of PHCS members with no shear reinforcement should be reduced by half (Palmer and Schultz 2010, 2011), and as afore-mentioned, it is very difficult to provide shear reinforcement in the PHCS members produced by the extrusion method. According to the recent research conducted by Lee et al. (2014), Im et al. (2014), and Palmer and Schultz (Palmer and Schultz 2010, 2011), however, the strict restriction on the web-shear capacity of the thick PHCS members without the minimum shear reinforcement can be excessively conservative. Because the provisions on the web-shear strength of the PHCS members have a huge impact on precast industry, further experimental investigations on thick PHCS members and detailed reviews on this issue are still required. As an example, Fig. 3 shows a typical underground precast parking structure of a multiplex building constructed with the PHCS units. Due to heavy weight of soil, the top roof floors of the basement are commonly designed with thick PHCS members having a thickness of 300 mm or greater, and the required shear force to the web-shear capacity ratio (\(V_{u} /\phi V_{cw}\)) of the PHCS units at the critical section of the member (i.e., support region) typically ranges from about 0.5–0.7 in the consideration of structural safety and economic efficiency. Due to the reduction of the web-shear capacity of the PHCS members without minimum shear reinforcement specified in ACI318-08 (ACI Committee 318 2008), much thicker PHCS members should be used in this example building, which gives a concern that the shear provision may lead to an uneconomical structural design of the PHCS members.
In this study, shear tests were conducted on the PHCS members with various thicknesses ranged from 200 to 500 mm, which is widely used in precast construction industry, and a large number of the web-shear capacity data of PHCS members were collected from previous studies (Pajari 2009; Palmer and Schultz 2010, 2011; Hawkins and Ghosh 2006; Lee et al. 2014; Im et al. 2014; Walraven and Mercx 1983; Becker and Buettner 1985; Pajari 2005; TNO 2005; Bertagnoli and Mancini 2009; Celal 2011; Rahman et al. 2012; Simasathien and Chao 2015). On this basis, this study evaluates the rationality of the reduction in the web-shear strength of the thick PHCS without shear reinforcement in the ACI318 code, and a simple alternative method is presented considering a proper margin of safety and the economic efficiency.
2 Web-shear Capacity of Precast Prestressed Hollow Core Slab
The shear strength of the PHCS is typically determined by the web-shear capacity at the end regions. The web-shear capacity of prestressed concrete (PSC) members without shear reinforcement can be defined as the shear force acting on the cross-section when the principal tensile stress of the web concrete (\(\sigma_{1}\)) reaches the cracking strength of concrete (\(f_{cr}\)), assuming that the web-shear capacity is close enough to the web-shear cracking strength. Thus, the web-shear capacity (\(V_{cw}\)) of PHCS can be expressed, based on theory of elasticity (Ugural and Fenster 2003), as follows:
where \(Q\) is the first moment about the centroidal axis of the part of the cross-sectional area lying farther from the centroidal axis than the point where the shear stresses are being calculated, \(I_{g}\) is the moment of inertia of the gross section, \(b_{w}\) is the sum of the total web widths of the PHCS, \(f_{ct}\) is the tensile strength of the concrete, \(\alpha\) is the coefficient for the reduced effective prestress at the critical section, \(f_{se}\) is the effective prestress, and \(A_{ps}\) and \(A_{g}\) are the cross-sectional area of tendon and concrete, respectively.
The design codes in North America, such as ACI318-08 (ACI Committee 318 2008) and AASHTO-LRFD (AASHTO 2007) assume an average shear stress distribution in the cross-section, and thus the web-shear capacity (\(V_{cw}\)) of PSC members can be expressed, as follows:
where \(f_{c} '\) is the specified compressive strength of concrete, \(\lambda\) is the light-weight concrete coefficient, \(f_{pc}\) is the compressive stress in concrete at centroid of the cross-section resisting externally applied loads or at junction of web and flange when the centroid lies within the flange, \(d_{p}\) is the distance from extreme compression fiber to centroid of prestressing steel, \(d_{v}\) is the effective depth, and \(V_{p}\) is the vertical component of the effective prestress force. In ACI318-08 (ACI Committee 318 2008) and AASHTO-LRFD (AASHTO 2007), it is also specified that the effective prestress (\(f_{se}\)) at the critical section should be reduced by accounting for its linear change within the transfer length that is \(50d_{b}\) or \(60d_{b}\), respectively. ACI318-08 (ACI Committee 318 2008) also specifies that the critical sections of PSC members are located at the distance \(h/2\) from the member ends, while, in AASHTO-LRFD (AASHTO 2007), the critical section is assumed to be located at the effective shear depth (\(d_{v}\)) or \(0.5d_{v} \cot \theta\) from the member ends, where \(\theta\) is the diagonal crack angle. In addition, as afore-mentioned, the current ACI318 code (ACI Committee 318 2014) specifies that the minimum shear reinforcement should be provided, if the factored shear (\(V_{u}\)) exceeds \(0.5\phi V_{cw}\) for the hollowed-section members with the untopped height exceeding 315 mm. In other words, the web-shear capacity of the PHCS with the net member height over 315 mm, produced by the extrusion method, should be reduced by half, as follows:
3 Experimental Study
3.1 Specimens and Test Set-up
In this study, shear tests were conducted on a total of 10 PHCS test specimens, whose thicknesses were 200, 265, 400, and 500 mm. All the PHCS specimens tested in this study were produced by the extrusion method in a long-line precast bed. Table 1 shows the material mixing ratio of concrete used in this study. The water-cement ratio (w/c) was 36.2%, the slump of the concrete was almost zero, and the maximum aggregate size was 13.0 mm. The design strength of concrete was 40.0 MPa, and the concrete compressive strength (\(f_{c} '\)) was measured at 60.5 MPa. Seven-wire low-relaxation tendons with the diameters of 9.5 or 12.7 mm were used in this study, and their tensile strengths (\(f_{pu}\)) were approximately 1860 MPa.
Figure 4 shows dimensional details of test specimens. The S2 and S2.65 series were 200 mm and 265 mm deep, respectively, and two prestressing tendons with a diameter of 9.5 mm were provided in the compression zone of the hollow-cored section, while four prestressing tendons with a 12.7 mm diameter were placed in the tension zone. The S4 series had a thickness of 400 mm, and two 9.5 mm and eight 12.7 mm prestressing tendons were provided in the compression zone and tension zone, respectively. The S5 series were 500 mm in depth, and two 9.5 mm and ten 12.7 mm prestressing tendons were placed in the compression zone and tension zone, respectively. The top and bottom tendons were pre-tensioned at the same time, and the magnitude of the effective prestress (\(\,f_{se}\)) was about \(0.65f_{pu}\). As shown in Table 2, the magnitudes of the compressive stresses at the centroid of the concrete section (\(f_{pc}\)) were ranged from 4.0 to 5.0 MPa. The area ratio between the hollow cores and the concrete gross section without hollow-cores were 49 and 52% in the S2 and S2.65 series, respectively, and those of the S4 and S5 series were 54 and 55%, respectively. The S2 and S2.65 series are divided into E and F specimens. As shown in Fig. 5a, the S2-E and S2.65-E specimens were tested at the end regions within the transfer length, where the effective prestress was not fully developed. As shown in Fig. 5b, the S2-F and S2.65-F specimens were supported at 80 times the diameter (\(\,d_{b}\)) of the prestressing tendon from the one end of the members, where the effective prestress was expected to be fully developed. The shear span-depth ratio (\(a/d\)) of the S2 and S2.65 series was 3.0, and the one point load was applied on the top of the specimens. The S4 and S5 series were also tested within the transfer length with a shear-span ratio (a/d) of 2.8 as done in the S2-E and S2.65-E specimens, as shown in Fig. 5a.
During the tests, vertical deflections were measured at the loading point, as shown in Fig. 5, but no strain gage were installed in the prestressing tendons because all the specimens were produced by the extrusion method in a commercial precast plant having a tight production schedule.
3.2 Experimental Results
All the PHCS specimens tested in this study failed under shear, as shown in Figs. 6 and 7, having the critical diagonal tension cracks developed in the web concrete between the loading point and the supporting point. Figure 8 shows the load–deflection behaviors of the S2 series specimens. As shown in Fig. 8a, the S2-E and S2-F specimens with 200 mm thickness had almost same stiffness up to diagonal tension cracking, and the shear-resisting forces were reduced right after the diagonal tension cracking. The S2-F specimen tested in the region where the effective prestress (\(f_{se}\)) was fully developed showed about two times higher shear capacity than that of the S2-E specimen tested within the transfer length. In the S2-F specimen, about 10% of the maximum load decreased right after the occurrence of shear cracks, and in the S2-E specimen, about 25% of the maximum load was reduced right after shear cracking.
Figure 8b shows a comparison of the load–deflection behaviors between the S2.65-E and S2.65-F specimens with 265 mm thickness. The S2.65-F specimen with the full effective prestress (\(\,f_{se}\)), which was tested at the outside of the transfer length, showed slightly higher stiffness compared to the S2.65-E specimen, and its shear capacity was also about 1.8 times higher than the S2.65-E specimen. In addition, the S2.65-F specimen showed more stable post-peak responses compared to the S2.65-E specimen.
All the S4 series specimens, i.e., S4-1, S4-2, and S4-3 specimens, showed a perfectly linear load–deflection response until the web-shear cracks occurred, as shown in Fig. 9a, and they were failed in shear at 279.2, 261.3 and 294.0 kN, respectively, due to the significant diagonal tension cracks developed in the web concrete with loud noises. The average value of the shear capacities of the three test specimens (\(\,V_{n,ave}\)) was 278.1 kN with a less than 10% variation, and their average shear strength (\(\,v_{n} = V_{n,ave} /b_{w} d_{p}\)) was 2.80 MPa. Unlike the S2 and S2.65 series specimens, the S4 series specimens showed much more brittle failure modes right after reaching the maximum loads without any post-peak response. Their shear capacities were sufficiently larger than the web-shear capacity estimated by the ACI318-05 code model though, which means that the reduction in shear strength due to the size effect was not observed from these specimens with 400 mm in depth. As shown in Fig. 9b, the S5 series specimens, i.e., S5-1, S5-2, and S5-3 specimens, also demonstrated the almost linear load–deflection responses until the diagonal tension cracking, which were very similar to the S4 series specimens. The S5 series specimens also showed brittle web-shear failures at 427.2, 454.4, and 369.8 kN, respectively. The average shear capacity was 417.1 kN, which is almost identical to that estimated by the ACI318-05 shear equation. The average shear strength of the specimens (\(\,v_{n}\)) was 3.06 MPa, which is approximately 10% higher than that of the S4 series specimens. Thus, the shear strength reduction due to the size effect was not observed in the S5 series specimens as well as in the S4 specimen.
4 Evaluation of Web-shear Capacity of PHCS Members
4.1 Shear Database for PHCS Members
In addition to the test results reported in this study, the shear test results of PHCS members were collected from the literature (Palmer and Schultz 2011; Walraven and Mercx 1983; Becker and Buettner 1985; Pajari 2005; TNO 2005; Bertagnoli and Mancini 2009; Celal 2011; Rahman et al. 2012; Simasathien and Chao 2015), and their detailed information can be found in Appendix. All the collected specimens were the PHCS members with no cast-in-place topping concrete, and it was thoroughly confirmed that all of them were failed in web-shear. The cross-sectional heights (\(h\)) of the collected test specimens ranged from 151 to 508 mm, and the concrete compressive strengths (\(f_{c} '\)) ranged from 36 to 114 MPa. In addition, the average compressive stresses due to the prestress at the centriod of section (\(f_{pc}\)) were mostly distributed in the range from 1.0 to 8.0 MPa, except some specimens exceeding this range.
Figure 10 shows the shear test results of all the collected PHCS test specimens and those tested in this study, in which their normalized average shear strength divided by \(\sqrt {f^{\prime}_{c} }\) were plotted with respect to the key influential factors on the web-shear capacity. As shown in Fig. 10, the web-shear capacity of the PHCS members showed a uniform distribution without a specific bias against the key influential factors, such as the cross-sectional height (\(h\)), the concrete compressive strength (\(f_{c} '\)), and the magnitude of the prestress (\(f_{pc}\)). Especially, it can be confirmed that there is no significant size effect in the web-shear strengths of the thick PHCS members with thicknesses over 315 mm.
4.2 Evaluation of Code Models
Figure 11 and Table 3 show comparisons of the web-shear capacities of the PHCS specimens and those estimated by the web-shear strength model presented in the ACI318-05 (ACI Committee 318 2005) and ACI318-08 (ACI Committee 318 2008) i.e., Eq. 2 in this study. As shown in Fig. 11a, the web-shear strength model specified in ACI318-05 (ACI Committee 318 2005) provided unsafe estimations on the shear strengths of the PHCS members with thicknesses over 315 mm (\(h > 315{\text{ mm}}\)), as pointed out by Hawkins and Ghosh (2006). However, it is also confirmed that ACI318-05 (ACI Committee 318 2005) provided unsafe estimations for the thin PHCS members with depth under 315 mm (\(h \le 315{\text{ mm}}\)). In fact, the shear strength ratios (\(V_{pre} /V_{u,test}\)) of the thin PHCS members (\(h \le 315{\text{ mm}}\)) were more scattered than those of the thick PHCS members (\(h > 315{\text{ mm}}\)), which indicates that the accuracy of the web-shear strength specified in ACI318-05 (ACI Committee 318 2005) is worse for the thin PHCS members than the thick PHCS members. Thus, it is now concerned that ACI318-05 (ACI Committee 318 2005) provides unsafe web-shear capacities not only for the thick PHCS members but also for the thin PHCS members.
Figure 11b shows the evaluation results of ACI318-08 provisions (ACI Committee 318 2008), considering that the web-shear capacity of the PHCS members with thicknesses greater than 315 mm without the minimum shear reinforcement should be reduced by half. It appears that the ACI318-08 (ACI Committee 318 2008) equation provided the overly conservative results for all the specimens exceeding 315 mm in height, and consequently it gave an excessive margin of safety for those thick PHCS members. It should be noted, however, that ACI318-08 still provided unsafe estimations for the web-shear capacities of the thin PHCS members (\(h \le 315{\text{ mm}}\)). The AASHTO-LRFD simplified model (AASHTO 2007), as shown in Fig. 11c, provided conservative estimations on the shear strengths of all the PHCS members regardless of their thicknesses, but its accuracy turned out to be slightly lower than ACI318-05 (ACI Committee 318 2005) as shown in Table 3.
4.3 Alternative Approach
The concept of the average shear stress analogy used in North American standards is based on Kani’s tooth model (Kani 1964) as shown in Fig. 12. However, according to the authors’ previous study (Lee et al. 2014), the thin-webbed prestressed concrete (PSC) members without shear reinforcement were typically failed in the web-shear right after diagonal tension cracking at near supports without any flexural crack, where the external shear force acting on the cross-section is more significant than flexural moment. Thus, it is not likely that the PHCS members without shear reinforcement have the average shear stress distributions shown in Fig. 12 at the web-shear failure. For this reason, Eurocode2 (European Committee for Standardisation, CEN 2004) adopts different shear stress distribution from ACI318 for the estimation of the web-shear capacity of the PSC member without shear reinforcement, and in the authors’ previous study (Lee et al. 2014), the web-shear capacity of the PHCS members was calculated with the basis of a parabolic shear stress distribution. In addition, authors attempted to simplify the calculation procedure, examining the ratio of the average to the parabolic shear stress (\(\,I_{g} /Qd_{p}\)) of the collected PHCS specimens, which was approximately converged to 0.76 as shown in Fig. 13. Thus, defining the average value of \(\,I_{g} /Qd_{p}\) of the collected PHCS specimens as the conversion factor (\(\eta\)) to change from the average shear stress to the maximum shear stress of the parabolic shear distribution, the web-shear capacity in Eq. (2) can be modified, as follows:
where \(\eta\) is 1.0 and 0.76 for the PHCS members with and without shear reinforcement, respectively, and \(V_{p}\) is the vertical components of prestressing forces due to draped strands, if any. It should be noted that Eq. (5) is to estimate the web-shear capacity (\(V_{cw}\)) of the PHCS members without shear reinforcement, not for other types of PSC members.

(Kani 1964).
Concept of average shear stress assumption
Figure 14a shows the comparison between the test results of the PHCS specimens and the analysis results calculated by Eq. (5). It shows that Eq. (5) can provide a uniform level of safety margin for the PHCS members with all the different thicknesses, while it gives a similar level of accuracy with ACI318-05 (ACI Committee 318 2005). It should be noted, however, that the experimental shear test data on the thick PHCS members with a cross-sectional height exceeding 500 mm are still very limited, and their brittle shear failure modes should be also considered with a big concern. On this basis, it seems to be desirable to apply the conversion factor (\(\eta\)) of 0.5 in Eq. (5) for the thick PHCS members over 500 mm deep. Figure 14b shows the analysis results by Eq. (5) reflecting the shear strength reduction of the PHCS members with the heights over 500 mm. It is shown that the proposed simple approach can secure an improved accuracy and a proper margin of safety for the web-shear design of the PHCS members.
5 Conclusions
In this study, the web-shear tests on the PHCS members without shear reinforcement were conducted, and the shear test data on PHCS members were also collected from literature. The web-shear strength equations in design code provisions were evaluated by comparing to the test data of the PHCS members. Based on this study, the following conclusions can be drawn:
-
1.
The thick PHCS specimens over 315 mm in depth tested in this study showed very brittle shear failure modes, but their web-shear capacities were not reduced by the size effect.
-
2.
The web-shear capacity estimated by the ACI318-05 (ACI Committee 318 2005) provided unconservative results not only for the thick PHCS members (\(h > 315{\text{ mm}}\)) but also the thin PHCS members (\(h \le 315{\text{ mm}}\)).
-
3.
The analysis results of the PHCS web-shear database showed that the ACI318-08 code (ACI Committee 318 2008) model, imposing the web-shear capacity reduction on the PHCS members with the heights greater than 315 mm, can provide excessively conservative estimations on the shear capacities of the thick PHCS members without shear reinforcement.
-
4.
In order to secure sufficient margin of safety and economical structural design of PHCS members, authors introduced a simple method to estimate their shear strength, and it provided fairly accurate analysis results.
-
5.
Further experimental investigations on the PHCS members exceeding 500 mm in depth are still encouraged because of the lack of experimental data on these members, and their brittle failure modes also need to be considered in their design.
References
AASHTO. (2007). AASHTO LRFD bridge design specifications. Washington D.C: American Association of State Highway and Transportation Officials (AASHTO).
ACI Committee 318. (2005). Building code requirements for structural concrete (ACI 318M-05) and commentary. Farmington Hills: American Concrete Institute.
ACI Committee 318. (2008). Building code requirements for structural concrete (ACI 318M-08) and commentary. Farmington Hills: American Concrete Institute.
ACI Committee 318. (2014). Building code requirements for structural concrete (ACI 318-14) and commentary. Farmington Hills: American Concrete Institute.
Becker, R. J., & Buettner, D. R. (1985). Shear tests of extruded hollow core slabs. PCI Journal, 30(2), 40–54.
Bertagnoli, G., & Mancini, G. (2009). Failure analysis of hollow-core slabs tested in shear. Structural Concrete, 10(3), 139–152.
Celal, M. S. (2011). Shear behaviour of precast/prestressed hollow-core slabs. M.Sc. Dissertation, University of Manitoba, Canada.
European Committee for Standardisation, CEN. (2004). Eurocode 2: Design of concrete structures—part 1-1: General rules and rules for buildings. Brussels: European Standard, EN 1992-1-1, CEN.
Hawkins, N. M., & Ghosh, S. K. (2006). Shear strength of hollow-core slabs. PCI Journal, 51(1), 110–115.
Im, J. H., Park, M. K., Lee, D. H., Kim, K. S., & Seo, S. Y. (2014). Evaluation of web-shear capacity of thick hollow-core slabs. The Regional Association of Architectural Institute of Korea, 16(6), 139–145. (In Korean).
Kani, G. N. J. (1964). The riddle of shear failure and its solution. ACI Structural Journal, 61(4), 441–467.
Lee, D. H., Park, M. K., Oh, J. Y., Kim, K. S., Im, J. H., & Seo, S. Y. (2014). Web-shear capacity of prestressed hollow-core slab unit with consideration on the minimum shear reinforcement requirement. Computers and Concrete, 14(3), 211–231.
Pajari, M. (2005). Resistance of prestressed hollow core slabs against web shear failure. Espoo: Technical Research Centre of Finland (VTT).
Pajari, M. (2009). Web shear failure in prestressed hollow core slabs. Journal of Structural Mechanics, 42(4), 207–217.
Palmer, K. D., & Schultz, A. E. (2010). Factors affecting web-shear capacity of deep hollow-core units. PCI Journal, 55(2), 123–146.
Palmer, K. D., & Schultz, A. E. (2011). Experimental investigation of the web-shear strength of deep hollow-core units. PCI Journal, 56(3), 83–104.
Precast/Prestressed Concrete Institute. (1998). PCI manual for the design of hollow core slabs. Chicago: Precast/Prestressed Concrete Institute.
Rahman, M. K., Baluch, M. H., Said, M. K., & Shazali, M. A. (2012). Flexural and shear strength of prestressed precast hollow-core slabs. Arabian Journal for Science and Engineering, 37(2), 443–455.
Simasathien, S., & Chao, S. H. (2015). Shear strength of steel-fiber-reinforced deep hollow-core slabs. PCI Journal, 60(4), 85–101.
TNO. (2005). TNO Report: Standard shear tests on prestressed hollow core slabs according to EN 1168. Hague: TNO Building and Constructions Research.
Ugural, A. C., & Fenster, S. K. (2003). Advanced strength and applied elasticity. Upper Saddle River: Prentice Hall Inc.
Walraven, J. C., & Mercx, W. P. M. (1983). The bearing capacity of prestressed hollow-core slabs. Heron, 28(3), 1–46.
Authors’ contribution
M-KP wrote the initial draft of manuscript. DHL and S-JH conducted the experiments and analyzed test results. KSK supervised the experiments and reviewed all the versions of the manuscript. All authors read and approved the final manuscript.
Acknowledgements
This research was supported by Basic Science Research Program through the National Research Foundation of Korea (NRF) funded by the Ministry of Science and ICT (2016R1A2B2010277). Authors also would like to give sincere thanks to Samsung C&T Corporation for supporting this work by the project K2012-07, “Developments of enhancement techniques on shear strength of hollow-core slab and its composite action with topping concrete”.
Availability of data and material
Not applicable.
Competing interests
The authors declare no competing interests.
Funding
Not applicable.
Publisher’s Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Author information
Authors and Affiliations
Corresponding author
Additional information
Journal information: ISSN 1976-0485 / eISSN 2234-1315
Appendix
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 4.0 International License (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted use, distribution, and reproduction in any medium, provided you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made.
About this article
Cite this article
Park, MK., Lee, D.H., Han, SJ. et al. Web-Shear Capacity of Thick Precast Prestressed Hollow-Core Slab Units Produced by Extrusion Method. Int J Concr Struct Mater 13, 7 (2019). https://doi.org/10.1186/s40069-018-0288-x
Received:
Accepted:
Published:
DOI: https://doi.org/10.1186/s40069-018-0288-x