Approximation theorems of a solution of amperometric enzymatic reactions based on Green’s fixed point normal-S iteration

Abstract

In this paper, the authors present a strategy based on fixed point iterative methods to solve a nonlinear dynamical problem in a form of Green’s function with boundary value problems. First, the authors construct the sequence named Green’s normal-S iteration to show that the sequence converges strongly to a fixed point, this sequence was constructed based on the kinetics of the amperometric enzyme problem. Finally, the authors show numerical examples to analyze the solution of that problem.

1 Introduction

The development of a mathematical model based on diffusion has received a great deal of attention in recent years, many scientist and mathematician have tried to apply basic knowledge about the differential equation and the boundary condition to explain and approximate the diffusion and reaction model [1–11].

In 2017, Abukhaled and Khuri [12] solved a solution of amperometric enzymatic reaction based on Green’s function by using the fixed point iteration

$$ \frac{\partial ^{2} s}{\partial x^{2}} - \frac{Ks}{1 + \alpha s} = 0,\quad 0 < s \leq 1, $$

(1)

subject to

$$ s'(0) = 0, \qquad s(0)= a, \quad \text{and} \quad s(1)= b. $$

(2)

They defined an operator based on the Picard iteration and proved that the operator is a contraction mapping that shows the sequence convergence with regard to Banach’s theorem.

Theorem Banach

([13])

Let $(M, d)$ be a complete metric space and $P : M \rightarrow M$ be Banach’s contraction map (that is, there exists $a \in [0, 1)$ such that

$$ d(Px, Py) \leq a d(x, y) $$

for all $x, y \in M$. Then P has a unique fixed point $p \in M$. Furthermore, for each $x_{0} \in M$, the sequence $\lbrace x_{n}\rbrace$ defined by

$$ x_{n+1}=Px_{n} $$

for each $n\geq 0$ converges to the fixed point p.

In 2018, Khuri and Louhichi [14] presented a new numerical approach for the numerical solution of boundary value problems. The algorithm is defined in terms of Green’s function into the Ishikawa fixed point iteration [15]

$$ \textstyle\begin{cases} x_{0} \in M, \\ y_{n} = (1-\beta _{n}) x_{n}+\beta _{n}Px_{n}, \\ x_{n+1} = (1-\alpha _{n})x_{n}+\alpha _{n}Py_{n},\quad \forall n \in \mathbb{N}, \end{cases} $$

where $\{\beta _{n}\}$ and $\{\alpha _{n}\}$ are sequences in $[0,1]$. Note that the step of $y_{n}$ is called Mann’s iteration [16].

Further, the converge theorem was proved by using the theorem of Berinde [17].

Theorem Berinde

Let M be an arbitrary Banach space, K is a closed convex subset of M and $P: K \to K$, which the operator satisfies the Zamfirescu operator. Let $\{x_{n}\}_{n=0}^{\infty }$ be an Ishikawa iteration and $x_{0} \in K$, where $\{\alpha \}$ and $\{\beta \}$ are sequences of positive numbers in $[0,1]$ with $\{\beta _{n}\}$ satisfying $\sum_{n=0}^{\infty }\beta _{n} = \infty$. Then $\{x_{n}\}_{n=0}^{\infty }$ strongly converges to the fixed point of P.

The above operator is sometimes called Zamfirescu operator [18].

Theorem Zamfirescu

Let $(M, d)$ be a complete metric space and $P: M \to M$ be a map for there exist the real numbers $a_{1}$, $a_{2}$, and $a_{3}$ satisfying $0 \leq a_{1} < 1$, $0 \leq a_{2}$, $a_{c} < 0.5$ such that, for each pair x, y in M, at least one of the following is true:

$(z_{1})$:

$d(Px,Py) \leq a_{1}d(x,y)$;

$(z_{2})$:

$d(Px,Py) \leq a_{2}[d(x,Px) + d(y,Py)]$;

$(z_{3})$:

$d(Px,Py) \leq a_{3}[d(x,Py) + d(y,Px)]$.

Then P has a unique fixed point p and the sequence $\{x_{n}\}_{n=0}^{\infty }$ defined by Picard iteration

$$ x_{n+1} = Px_{n}, \quad n=0,1,2,\ldots $$

converges to p for any $x_{0} \in M$.

In this paper, the authors use the motivation above to construct Green’s normal-S iteration based on the sequence of normal-S iteration of Sahu [19]. Let K be a convex subset of the normed space M and a nonlinear mapping P, the sequence $\{x_{n}\}$ in K is call normal-S if it is defined by

$$ \textstyle\begin{cases} x_{0} \in M, \\ y_{n} =(1-\beta _{n})x_{n}+\beta _{n}Px_{n}, \\ x_{n+1} =P(y_{n}) \end{cases} $$

(3)

for each $n \geq 1$, where $\lbrace \beta _{n}\rbrace$ is the sequence in $[0,1]$.

The proof of the convergence theorem is based on Berinde’s idea. Finally, the authors use the sequence to approximate problem (1) subject to (2) by showing a numerical example.

2 Preliminaries

2.1 The mathematical model

Diffusion equations were presented by a mathematical model related to Michaelis–Menten kinetics (4) of the enzymatic reaction

$$ E + S \quad \iff\quad ES \rightarrow E + P, $$

(4)

where E is an enzyme, S is a substrate, ES is a complex between enzyme and substrate, and P is a product of reaction.

In biochemistry, the enzyme kinetics in n-dimension Ω is modeled by the reaction-diffusion equation [20]

$$ \frac{\partial S}{\partial t} = D_{S}\nabla (\nabla S) - \nu (t, X),\quad X \in \Omega , $$

(5)

where $D_{S}$ is the diffusion coefficient of a substrate and ν is the initial reaction velocity. By using the Michaelis–Menton hypothesis, the velocity ν for simple reaction processes without competitive inhibition is given by [20, 21]

$$ \nu (t, X) = \frac{KS}{1 + S/K_{M}}, $$

(6)

where $K = k_{2}E_{0}/K_{M}$ represents a pseudo first order, in which $k_{2}$ is the unimolecular rate constant, $E_{0}$ is the total amount of enzymes, and $K_{M}$ is the Michaelis constant. The one-dimensional form of (5) is given by

$$ \frac{\partial S}{\partial t} = D_{S} \frac{\partial ^{2} S}{\partial X^{2}} - \frac{KS}{1 + S/K_{M}},\quad X\in \Omega , $$

(7)

with the initial condition given by

$$ S(0, X) = S_{0}(X). $$

(8)

By introducing the parameters

$$ s = \frac{S}{KS^{\infty }}, \qquad x = \frac{X}{L}, \qquad \tau = \frac{D_{S}}{L^{2}},\qquad K=\frac{kL^{2}}{D_{S}}=\phi ^{2},\qquad \alpha = \frac{kS^{\infty }}{K_{M}}, $$

(9)

we obtain the nonlinear reaction-diffusion equation at steady state

$$ \frac{\partial ^{2} s}{\partial x^{2}} - \frac{Ks}{1 + \alpha s} = 0,\quad 0 < s \leq 1, $$

(10)

where $S^{\infty }$ is the substrate concentration in bulk solution (mol dm⁻³), $\phi ^{2}$ is the Thiele modulus.

2.2 Green’s function

Consider the second order differential equation decomposed into a linear term $\operatorname{Li}[y]$ and a nonlinear term $f(t,y,y')$ as follows:

$$ \operatorname{Li}[y] \equiv y'' = f \bigl(t, y, y' \bigr), $$

(11)

subject to the boundary conditions

$$ \textstyle\begin{cases} \operatorname{BC}_{a}[y] \equiv a_{0}y(a) + a_{1}y'(a) = \alpha , \\ \operatorname{BC}_{b}[y] \equiv b_{0}y(b) + b_{1}y'(b) = \beta , \end{cases} $$

(12)

where $a \leq t \leq b$. Bernfeld and Lakshmikantham [22] presented the existence and uniqueness theorems for solutions of (11).

The Green’s function $G(t,s)$ corresponding to the linear term $\operatorname{Li}[y]$ is defined as the solution of the following boundary value problem:

$$ \operatorname{Li} \bigl[G(t, s) \bigr] = \delta (t - s) , \qquad \operatorname{BC}_{a} \bigl[G(t, s) \bigr] = \operatorname{BC}_{b} \bigl[G(t, s) \bigr] = 0, $$

(13)

and has the piecewise form

$$ G(t, s) = \textstyle\begin{cases} c_{1}y_{1} +c_{2}y_{2},& a \leq t < s, \\ d_{1}y_{1} +d_{2}y_{2},& s < t \leq b, \end{cases} $$

(14)

where $y_{1}$ and $y_{2}$ form a fundamental set of solutions for $\operatorname{Li}[y] = 0$. The unknowns could be found using the homogeneous conditions given in (12) and the fact that the Green’s function is continuous and its first derivative has a unit jump discontinuity. More precisely, the constants are determined using the following properties:

• A. G satisfies the corresponding homogeneous boundary conditions

  $$ \operatorname{BC}_{a} \bigl[G(t, s) \bigr] = \operatorname{BC}_{b} \bigl[G(t, s) \bigr] = 0; $$

  (15)

• B. G is continuous at $t = s$, i.e.,

  $$ c_{1}y_{1}(s) + c_{2}y_{2}(s) = d_{1}y_{1}(s) + d_{2}y_{2}(s); $$

  (16)

• C. $G'$ has a unit jump discontinuity at $t = s$, i.e.,

  $$ d_{1}y'_{1}(s)+d_{2}y'_{2}(s) - c_{1}y'_{1}(s) - c_{2}y'_{2}(s) = 1. $$

  (17)

A particular solution to $y'' = f(t, y, y', y'')$ is expressed in terms of G and is given by the following structure:

$$ u_{p} = \int _{a}^{b}G(t,s)f \bigl(s,u_{p},u'_{p} \bigr)\,ds. $$

(18)

We construct the Green’s function for the differential operator $\operatorname{Li}[y] = y'' = 0$, which has two linearly independent solutions $y_{1}(t) = 1$ and $y_{2}(t) = t$. From (14), the Green’s function will have the form

$$ G(t, s) = \textstyle\begin{cases} c_{1} +c_{2}t, & 0 \leq t \leq s , \\ d_{1} +d_{2}t, & s \leq t \leq 1, \end{cases} $$

(19)

where the unknowns are found by the properties A, B, and C listed above. To find the homogeneous boundary conditions, we have

$$ c_{1} = 0, \qquad d_{1} + d_{2} = 0. $$

(20)

$G(t, s)$ is continuous and $G'(t, s)$ discontinues at $t = s$ then

$$ \textstyle\begin{cases} c_{1} + c_{2}s = d_{1} + d_{2}s, \\ c_{2} - d_{2} = 1. \end{cases} $$

(21)

From (19)–(21), we obtain the following Green’s function:

$$ G(t, s) = \textstyle\begin{cases} s(1-t), & 0 \leq s \leq t , \\ t(1-s), & t \leq s \leq 1 . \end{cases} $$

(22)

2.3 Green’s normal-S iteration

Applying the Green’s function to the normal-S iterative method, we recall the following differential equation:

$$ \operatorname{Li}[y] + \operatorname{No}[y] = f(t, y), $$

(23)

where $\operatorname{Li}[u]$ is a linear operator in y, $\operatorname{No}[y]$ is a nonlinear operator in y, and $f(t, y)$ is a linear or nonlinear function in y. Let $y_{p}$ be a particular solution of (23). We define the linear integral operator in terms of the Green’s function and the particular solution $y_{p}$ as follows:

$$ K[y_{p}] = \int _{a}^{b}G(t, s)\operatorname{Li}[y_{p}] \,ds. $$

(24)

Here, G is the Green’s function corresponding to the linear differential operator $\operatorname{Li}[y]$. For convenience, we set $y_{p} = v$. Adding and subtracting $\operatorname{No}[v] - f(s, v)$ from within the integral in (24) yield

$$\begin{aligned} K[v]&= \int _{a}^{b}G(t, s) \bigl(\operatorname{Li}[v]+ \operatorname{No}[v]-f(s,v) \bigr)\,ds + \int _{a}^{b}G(t, s) \bigl(f(s,v) - \operatorname{No}[v] \bigr)\,ds \\ \end{aligned}$$

(25)

$$\begin{aligned} &= v + \int _{a}^{b}G(t, s) \bigl(\operatorname{Li}[v]+ \operatorname{No}[v]-f(s,v) \bigr)\,ds. \end{aligned}$$

(26)

We then apply the normal-S fixed point iterative form

$$ \textstyle\begin{cases} v_{0} \in M, \\ w_{n} = (1-\beta _{n})v_{n} + \beta _{n}K[v_{n}], \\ v_{n+1} = K[w_{n}], \end{cases} $$

(27)

where $n \geq 0$, $(\beta _{n})$ is a sequence of real numbers in $[0,1]$. That is,

$$ \textstyle\begin{cases} v_{0} \in M, \\ w_{n} = (1-\beta _{n})v_{n} + \beta _{n} [ v_{n} + \int _{a}^{b}G(t,s)(\operatorname{Li}[v_{n}] + \operatorname{No}[v_{n}] - f(s,v_{n}))\,ds ], \\ v_{n+1} = w_{n} + \int _{a}^{b}G(t,s)(\operatorname{Li}[w_{n}] + \operatorname{No}[w_{n}] - f(s,w_{n}))\,ds, \end{cases} $$

(28)

which is reduced to

$$ \textstyle\begin{cases} v_{0} \in M, \\ w_{n} = v_{n} + \beta _{n} \int _{a}^{b}G(t,s)(\operatorname{Li}[v_{n}] + \operatorname{No}[v_{n}] - f(s,v_{n}))\,ds, \\ v_{n+1} = w_{n} + \int _{a}^{b}G(t,s)(\operatorname{Li}[w_{n}] + \operatorname{No}[w_{n}] - f(s,w_{n}))\,ds. \end{cases} $$

(29)

3 Main results

3.1 Constructing the normal-S Green’s iterative scheme

Let $\operatorname{Li}[s] = \frac{\partial ^{2} s}{\partial x^{2}}$ and $f(\alpha ,K,s) = \frac{Ks}{1+\alpha s}$, consider the enzyme substrate reaction equation, which takes the form of the following nonlinear equation:

$$ \operatorname{Li}[s] = s''(x) = f \bigl(x,s(x), s'(x) \bigr), $$

(30)

with boundary condition (2), then the required Green’s function

$$ \operatorname{Li} \bigl[G(x,z) \bigr] = \delta (x-z), $$

(31)

subject to the corresponding homogenous boundary conditions

$$ \frac{d}{dx}G(x,z)|_{x=0} = 0 \quad \text{and}\quad G(x,z)|_{x=1} = 0. $$

(32)

Using boundary condition (32) in Green’s function (19) then $G(x,z)$, we obtain the equations

$$ c_{2} = 0 \quad \text{and}\quad d_{1} + d_{2} =0. $$

(33)

The continuity of G implies that

$$ c_{2}z + c_{1} = d_{2}z + d_{1}, $$

(34)

and $\frac{d}{dx}G(x,z)$ jump discontinuity implies that

$$ d_{2} - c_{2} = 1. $$

(35)

Hence,

$$ G(x, z) = \textstyle\begin{cases} z-1, & 0 \leq x \leq z, \\ x-1, & z \leq x \leq 1. \end{cases} $$

(36)

From (25), we introduce the following continuous functions on $[0, 1]$ into itself:

$$ P_{G}(s_{n}) = s_{n} + \int _{0}^{1}G(x,z) \bigl(s''_{n}(z) - f(\alpha ,K,s_{n}) \bigr)\,dz, $$

(37)

then equations (27)–(29) become

$$ \textstyle\begin{cases} s_{0} \in M, \\ w_{n}=(1-\beta _{n})s_{n} + \beta _{n} P_{G}(s_{n}), \\ s_{n+1}=P_{G}(w_{n}). \end{cases} $$

(38)

3.2 Convergence theorems

In Theorem 1 we show that the operator $P_{G}$ is a contraction mapping, and in Theorem 2 we show that if the operator P satisfies condition Z, then the sequence $\{s_{n}\}_{n=0}^{\infty }$ defined by normal-S (29) converges strongly to the fixed point of P.

Theorem 1

Assume that the function f, which appears in the definition of the operator $P_{G}$, is such that

$$ C = C_{c} < 1, $$

where $C_{c} = \max_{x\in [0,1]}|f'(s(x))|$. Then $P_{G}$ is a contraction, and hence the sequence $\{s_{n}\}$ is defined by normal-S iteration (29).

Proof

Performing integration by parts in equations (29), (36)–(38), the product is

$$ P_{G}(s) = s- \int _{0}^{1}G(x,z)f \bigl(z,s,s' \bigr)\,dz, $$

(39)

where $s = w_{n}$ of (38). Thus

$$\begin{aligned} \bigl\vert P_{G}(s)-P_{G}(v) \bigr\vert &= \biggl\vert \int _{0}^{1}G(x,z) \bigl[f \bigl(z,v,v' \bigr)-f \bigl(z,s,s' \bigr) \bigr]\,dz \biggr\vert \\ &\leq \biggl( \int _{0}^{1} \bigl\vert G(x,z) \bigr\vert \,dz \biggr) \biggl( \int _{0}^{1} \bigl\vert f \bigl(z,v,v' \bigr)-f \bigl(z,s,s' \bigr) \bigr\vert \,dz \biggr) \\ &\leq \biggl( \int _{0}^{1} \bigl\vert f \bigl(z,v,v' \bigr)-f \bigl(z,s,s' \bigr) \bigr\vert \,dz \biggr). \end{aligned}$$

By applying the mean value theorem for $f(s)$ and using the condition that $C_{c} = \max_{x\in [0,1]}|f'(s(x))|$, we consider the last inequality

$$\begin{aligned} \bigl\vert P_{G}(s)-P_{G}(v) \bigr\vert &\leq \max _{x\in [0,1]} \bigl\vert f \bigl(v(x) \bigr) - f \bigl(s(x) \bigr) \bigr\vert \\ &\leq C_{c} \Vert s-v \Vert , \end{aligned}$$

where $\|s-v\| = \max_{x\in [0,1]}|s(x)-v(x)|$ and $C =C_{c} < 1$. So, we obtain the following:

$$ \bigl\Vert P_{G}(s)-P_{G}(v) \bigr\Vert \leq C \Vert s-v \Vert $$

such that $0 \leq C < 1$. Hence $P_{G}$ is a contraction mapping. □

Theorem 2

Let M be an arbitrary Banach space, K be a closed convex subset of M, and $P : K \rightarrow K$ be an operator satisfying the condition of Zamfirescu. Let $\{s_{n}\}_{n=0}^{\infty }$ be defined by normal-S (3) and $s_{0} \in K$, where $\{\beta _{n}\}$ is a sequence in $[0,1]$. Then $\{s_{n}\}_{n=0}^{\infty }$ converges strongly to the fixed point of P.

Proof

By Zamfirescu’s theorem, we know that P has a unique fixed point in K that is p. Consider $s, m \in K$. Since P is a Zamfirescu operator, at least one of conditions $(z_{1})$, $(z_{2})$, and $(z_{3})$ is satisfied. If $(z_{2})$ holds, then

$$\begin{aligned} \Vert Ps-Pm \Vert &\leq a_{2} \bigl[ \Vert s-Ps \Vert + \Vert m-Pm \Vert \bigr] \\ &\leq b \bigl[ \Vert s-Ps \Vert + \Vert m-s \Vert + \Vert s-Ps \Vert + \Vert Ps-Pm \Vert \bigr], \end{aligned}$$

so

$$ (1-a_{2}) \Vert Ps-Pm \Vert \leq a_{2} \Vert s-m \Vert + 2a_{2} \Vert s-Ps \Vert $$

from $0 \leq a_{2} <1$

$$ \Vert Ps-Pm \Vert \leq \frac{a_{2}}{1-a_{2}} \Vert s-m \Vert + \frac{2a_{2}}{1-a_{2}} \Vert s-Ps \Vert . $$

(40)

Similarly, if $(z_{3})$ holds

$$ \Vert Ps-Pm \Vert \leq \frac{a_{3}}{1-a_{3}} \Vert s-m \Vert + \frac{2a_{3}}{1-a_{3}} \Vert s-Ps \Vert . $$

(41)

Denote $\delta = \max \{a_{1}, \frac{a_{2}}{1-a_{2}}, \frac{a_{3}}{1-a_{3}} \}$. Then we have $0 \leq \delta < 1$ and get

$$ \Vert Ps-Pm \Vert \leq \delta \Vert s-m \Vert + 2\delta \Vert s-Ps \Vert . $$

(42)

The sequence $\{s_{n}\}_{n=0}^{\infty }$ is defined by normal-S iteration (3) and $s_{0} \in K$, by (42) we get

$$ \Vert s_{n+1}-p \Vert = \Vert Pp - Pm_{n} \Vert \leq \delta \Vert m_{n} -p \Vert . $$

Consider again

$$\begin{aligned} \Vert s_{n}-p \Vert &= \bigl\Vert (1-\beta _{n})s_{n} + \beta _{n}Ps_{n} - p \bigr\Vert \\ &= \bigl\Vert (1-\beta _{n}) (s_{n}-p) + \beta _{n}(Ps_{n} - p) \bigr\Vert \\ &= (1-\beta _{n}) \Vert s_{n}-p \Vert + \beta _{n} \Vert Ps_{n} - p \Vert . \end{aligned}$$

By (42) again,

$$ \Vert p-Ps_{n} \Vert \leq \delta \Vert s_{n} -p \Vert . $$

So, we have

$$\begin{aligned} \Vert s_{n+1}-p \Vert &\leq \delta \Vert m_{n} - p \Vert \\ &\leq \delta \bigl[(1-\beta _{n}) \Vert s_{n}-p \Vert + \beta _{n}Ps_{n} - p \Vert \bigr] \\ &\leq \delta \bigl[(1-\beta _{n}) \Vert s_{n}-p \Vert + \beta _{n} \delta \Vert s_{n} - p \Vert \bigr] \\ &= \delta (1-\beta _{n} + \beta _{n} \delta ) \Vert s_{n}-p \Vert , \end{aligned}$$

by induction

$$ \Vert s_{n+1}-p \Vert \leq \prod _{k=0}^{n} \delta (1-\beta _{k} + \beta _{k} \delta ) \Vert s_{0}-p \Vert \quad \text{for } n=0, 1, 2, \ldots . $$

(43)

From $\delta (1-\beta _{k} + \beta _{k} \delta ) < 1$,

$$ \lim_{n\to \infty }\prod _{k=0}^{n} \delta (1-\beta _{k} + \beta _{k} \delta ) = 0, $$

(44)

which implies

$$ \lim_{n\to \infty } \Vert s_{n+1} - p \Vert = 0. $$

(45)

Therefore $\{s_{n}\}_{n=0}^{\infty }$ converges strongly to the fixed point of P. This is completes the proof. □

4 Numerical examples

In the first example, we show a simple example to compare the solution with three iterative methods to explain the convergence of the sequences. In the last example, we present the main example to analyze the main problem (10).

Example 1

Consider the following differential equation $x(t)$:

$$ x''(t) = \frac{3}{2}x(t)^{2}, $$

(46)

where $0 \leq t \leq 1$ and subject to

$$ x(0)=4,\qquad x(1)=1. $$

(47)

The exact solution is $x(t) = \frac{4}{(1+t)^{2}}$. The initial iterate satisfies $x'' =0$ and boundary conditions (47). This $x_{0} = 4-3t$. By normal-S Green’s iteration (29),

$$ \begin{aligned} &w_{n}=x_{n} + \beta _{n} \int _{0}^{t}s(1-t) \biggl(x_{n}^{\prime \prime }(s) - \frac{3}{2}x(s)^{2} \biggr)\,ds+ \beta _{n} \int _{t}^{1}t(1-s) \biggl(x_{n}^{\prime \prime }(s) - \frac{3}{2}x(s)^{2} \biggr)\,ds, \\ &x_{n+1}=w_{n} + \int _{0}^{t}s(1-t) \biggl(w_{n}^{\prime \prime }(s) - \frac{3}{2}w(s)^{2} \biggr)\,ds + \int _{t}^{1}t(1-s) \biggl(w_{n}^{\prime \prime }(s) - \frac{3}{2}w(s)^{2} \biggr)\,ds. \end{aligned} $$

(48)

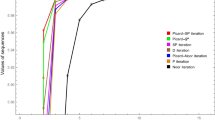
Table 1 shows the convergence step, Fig. 1 shows the convergence step and the error step of sequence $\{x_{n}\}$, which the error is calculated from $(\int _{a}^{b}|x_{n} - x_{\mathrm{exact}}|^{2})^{1/2}$.

Figure 1

The convergence step of sequence $\{x_{n}\}$ with $\alpha _{n} = 0.1 + \frac{0.000001}{n^{2}}$ and $\beta _{n} = 0.005+\frac{0.0000001}{n^{2}}$

Table 1 The convergence step of $\lbrace x_{n} \rbrace$ for example with $\alpha _{n} = 0.1 + \frac{0.000001}{n^{2}}$ and $\beta _{n} = 0.005+\frac{0.0000001}{n^{2}}$

Figure 1 shows a sequence of functions to compare three iterative methods. From the boundary condition, the value of problem starts at 4 and stops at 1. The back line is the solution of function, while red, blue, and green lines are Mann, normal-S, and Ishikawa sequences, respectively. The figure concludes that Mann and normal-S are converging faster than Ishikawa and converging nearly to the solution of the function.

Figure 2 shows the error of three iterative sequences to compare the error value. Red, blue, and green lines mark Mann, Normal-S, and Ishikawa sequences, respectively. The figure concludes that normal-S sequence is decreasing to 0 faster than the error of Mann and Ishikawa sequences.

Figure 2

The convergence and the error step of sequence $\{x_{n}\}$ with $\alpha _{n} = 0.1 + \frac{0.000001}{n^{2}}$ and $\beta _{n} = 0.005+\frac{0.0000001}{n^{2}}$

Example 2

Consider the differential equation (10)

$$ s''(x) = \frac{Ks}{1+\alpha s}, $$

where $0 \leq x \leq 1$ and subject to

$$ s'(0) = 0,\qquad s(0)= 4 \quad \text{and}\quad s(1)= 1. $$

The initial iterate satisfies $s'' =0$ and the boundary conditions. Then $s_{0} = 4-3x$. By normal-S Green’s iteration (29) and from (36), (37), and (38), the sequence is defined by

$$ \begin{aligned} &w_{n}=s_{n} + \beta _{n} \int _{0}^{x}(z-1) \biggl(s_{n}^{\prime \prime }(z) - \frac{Ks_{n}(z)}{1+\alpha s_{n}(z)}\biggr)\,dz \\ &\hphantom{w_{n}={}}{}+ \beta _{n} \int _{x}^{1}(x-1) \biggl(s_{n}^{\prime \prime }(z) - \frac{Ks_{n}(z)}{1+\alpha s_{n}(z)}\biggr)\,dz, \\ &s_{n+1}=w_{n} + \int _{0}^{x}(z-1) \biggl(w_{n}^{\prime \prime }(z) - \frac{Kw_{n}(z)}{1+\alpha w_{n}(z)}\biggr)\,dz \\ &\hphantom{s_{n+1}={}}{}+ \int _{x}^{1}(x-1) \biggl(w_{n}^{\prime \prime }(z) - \frac{Kw_{n}(z)}{1+\alpha w_{n}(z)}\biggr)\,dz, \end{aligned} $$

(49)

where K and α are constants of substrate concentration, and set $\beta _{n} = 0.005+\frac{0.0000001}{n^{2}}$.

Table 2 and Fig. 3 show approximation of substrate concentration sequence $S(x)$ for different values of α and K.

Figure 3

The error step of the sequence $\{s_{n}\}$ with different values of α with $K = 0.00001$ and different values of K with $\alpha = 1000$

Table 2 Approximation of substrate concentration sequence $S(x)$ for different values of α and K

Explanation of Fig. 3: Firstly, the error of normal-S sequence $S(x)$ compares with different values of α with $K = 0.00001$, the error sequence of large α converges faster than that of small α. Secondly, the error of normal-S sequence $S(x)$ which compared by different values of K with $\alpha = 1000$, the error sequence of small K converges faster than that of large K.

5 Conclusion

This paper presents a strategy based on fixed point iterative methods with normal-S iteration (38) to solve a nonlinear dynamical problem in a form of Green’s function with boundary conditions used in Theorem 1 and Theorem 2 to guarantee the solution. Example 2 explains two constants K and α in the nonlinear reaction-diffusion equation at steady state (1). Therefore, the values of K must be small, while the values of α should be large, so the error value of sequence will converge to 0 faster than the other cases.