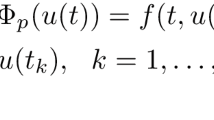Abstract
Applying the eigenvalue theory and theory of α-concave operator, we establish some new sufficient conditions to guarantee the existence and continuity of positive solutions on a parameter for a second-order impulsive differential equation. Furthermore, two nonexistence results of positive solutions are also given. In particular, we prove that the unique solution \(u_{\lambda}(t)\) of the problem is strongly increasing and depends continuously on the parameter λ.
Similar content being viewed by others
1 Introduction
We consider the second-order impulsive differential equation
where \(\lambda>0, \omega\in L^{p}[0,1]\) for some \(1\leq p\leq+\infty \), \(f\in C(R^{+}, R^{+}), R^{+}=[0,+\infty], t_{k} \) (\(k=1, 2, \dots, n\)) are fixed points with \(0< t_{1}< t_{2}<\cdots<t_{k}<\cdots <t_{n}<1, a, b>0\), \(\{c_{k}\}\) is a real sequence with \(c_{k}>-1, k=1, 2, \dots, n\), \(x(t_{k}^{+})\) (\(k=1, 2, \dots, n\)) denotes the right-hand limit of \(x(t)\) at \(t=t_{k}\), and \(g\in C[0,1]\) is a nonnegative function. In addition, we assume that \(\omega, f, c_{k}\), and g satisfy
- (H1):
-
\(\omega\in L^{p}[0,1]\) for some \(1\leq p\leq+\infty\), and there exists \(\xi>0\) such that \(\omega(t)\geq\xi\) a.e. on J;
- (H2):
-
\(f\in C([0,+\infty), [0,+\infty))\) with \(f(0)=0\) and \(f(u)>0\) for \(u>0\), \(\{c_{k}\}\) is a real sequence with \(c_{k}>-1\), \(k=1, 2, \dots, n\), and \(c(t):=\Pi_{0< t_{k}< t}(1+c_{k})\);
- (H3):
-
\(g\in C[0,1]\) is nonnegative with
$$ \mu:= \int_{0}^{1}g(t)c(t)\,dt\in\bigl[0,ac(1)\bigr). $$(1.2)
Remark 1.1
We always assume that the product \(c(t):=\Pi _{0< t_{k}< t}(1+c_{k})\) equals unity if the number of factors is equal to zero, and let
Remark 1.2
Combining (H2) and the definition of \(c(t)\), we know that \(c(t)\) is a step function, which is bounded on J, and
Such problems were first studied by Zhang and Feng [1]. By using transformation technique to deal with impulse term of second-order impulsive differential equations, the authors obtained existence results of positive solutions by using fixed point theorems in a cone. However, they only considered the case \(\omega(t)\equiv1\) on \(t\in [0,1]\). The other related results can be found in [2–14]. However, there are almost no papers on second-order boundary value problems, especially second-order boundary value problems with impulsive effects, using the eigenvalue theory. In this paper, we solve this problem.
The first goal of this paper is to establish several criteria for the optimal intervals of the parameter λ so as to ensure the existence of positive solutions for problem (1.1). Our method is based on transformation technique, Hölder’s inequality, and the eigenvalue theory and is completely different from those used in [1–14].
Another contribution of this paper is to study the expression and properties of Green’s function associated with problem (1.1). It is interesting to point out that the Green’s function associated with problem (1.1) is positive, which is different from that of [15].
Moreover, we give two nonexistence results. The arguments that we present here are based on geometric properties of the super-sublinearity of f at zero and infinity, which was first used by Sánchez in [16] (see Properties 1.1-1.2).
For convenience, we introduce the following notations:
The following geometric Properties 1.1-1.2 will be very important in our arguments.
Property 1.1
If \(f_{0}=+\infty\) and \(f_{\infty}=+\infty\), then there exists \(R>0\) such that
Let R̄ be a point where f attains its maximum on the interval \((0,R]\).
Property 1.2
If \(f_{0}=0\) and \(f_{\infty}=0\), then there exists \(R>0\) such that
Finally, we are able to obtain the uniqueness results of problem (1.1) by using theory of α-concave operators. We also obtain the following analytical properties: the unique solution \(u_{\lambda}(t)\) of the above problem is strongly increasing and depends continuously on the parameter λ.
The rest of this paper is organized as follows. In Section 2, we provide some necessary background. In particular, we introduce some lemmas and definitions associated with the eigenvalue theory and theory of α-concave (or −α-convex) operators. Several technical lemmas are given in Section 3. In Section 4, we establish the existence and nonexistence of positive solutions for problem (1.1). In Section 5, we prove the uniqueness of a positive solution for problem (1.1) and its continuity on a parameter . In Section 6, we offer some remarks and comments on the associated problem (1.1). Finally, in Section 7, two examples are also included to illustrate the main results.
2 Preliminaries
In this section, we collect some known results, which can be found in the book by Guo and Lakshmikantham [17].
Definition 2.1
Let E be a real Banach space over R. A nonempty closed set \(P \subset E\) is said to be a cone if
-
(i)
\(au+bv \in P\) for all \(u, v \in P\) and all \(a\geq0, b\geq0\), and
-
(ii)
\(u, -u \in P \) implies \(u=0\).
Definition 2.2
A cone P of a real Banach space E is a solid cone if \(P^{\circ}\) is not empty, where \(P^{\circ}\) is the interior of P.
Every cone \(P \subset E\) induces a semiorder in E given by “≤”. That is, \(x\leq y\) if and only if \(y-x \in P\). If a cone P is solid and \(y-x\in P^{\circ}\), then we write \(x\ll y\).
Definition 2.3
A cone P is said to be normal if there exists a positive constant δ such that
Geometrically, normality means that the angle between two positive unit vectors is bounded away from π. In other words, a normal cone cannot be too large.
Lemma 2.1
Let P be a cone in E. Then the following assertions are equivalent:
-
(i)
P is normal;
-
(ii)
There exists a constant \(\gamma>0\) such that
$$\|x+y\|\geq\gamma\max\bigl\{ \|x\|,\|y\|\bigr\} ,\quad \forall x, y\in P; $$ -
(iii)
There exists a constant \(\eta>0\) such that \(0\leq x\leq y\) implies that \(\|x\|\leq\eta\|y\|\), that is, the norm \(\|\cdot\|\) is semimonotone;
-
(iv)
There exists an equivalent norm \(\|\cdot\|_{1}\) on E such that \(0\leq x\leq y\) implies that \(\|x\|_{1}\leq\|y\|_{1}\), that is, the norm \(\|\cdot\|_{1}\) is semimonotone;
-
(v)
\(x_{n}\leq z_{n}\leq y_{n}\) (\(n=1,2,3,\ldots\)) and \(\| x_{n}-x\|\rightarrow0, \|y_{n}-x\|\rightarrow0\) imply that \(\| z_{n}-x\|\rightarrow0\);
-
(vi)
The set \((B+P)\cap(B-P)\) is bounded, where
$$B=\bigl\{ x\in E:\|x\|\leq1\bigr\} ; $$ -
(vii)
Every order interval \([x,y]=\{z\in E:x\leq z\leq y\}\) is bounded.
Remark 2.1
Some authors use assertion (iii) as the definition of normality of a cone P and call the smallest number η the normal constant of P.
Definition 2.4
Let P be a solid cone of a real Banach space E. An operator \(A: P^{\circ}\rightarrow P^{\circ}\) is called an α-concave operator (−α-convex operator) if
where \(0\leq\alpha<1\). The operator A is increasing (decreasing) if \(x_{1}, x_{2}\in P^{\circ}\) and \(x_{1}\leq x_{2}\) imply \(Ax_{1}\leq Ax_{2} \) (\(Ax_{1}\geq Ax_{2}\)), and further, the operator A is strongly increasing (decreasing) if \(x_{1}, x_{2}\in P^{\circ}\) and \(x_{1}< x_{2}\) imply \(Ax_{2}- Ax_{1}\in P^{\circ}\) (\(Ax_{1}- Ax_{2}\in P^{\circ}\)). Let \(x_{\lambda}\) be a proper element of an eigenvalue λ of A, that is, \(Ax_{\lambda}=\lambda x_{\lambda}\). Then \(x_{\lambda}\) is called strongly increasing (decreasing) if \(\lambda _{1}>\lambda_{2}\) implies that \(x_{\lambda_{1}}-x_{\lambda_{2}}\in P^{\circ}\) (\(x_{\lambda_{2}}-x_{\lambda_{1}}\in P^{\circ}\)), which is denoted by \(x_{\lambda_{1}}\gg x_{\lambda_{2}}\) (\(x_{\lambda_{2}}\gg x_{\lambda _{1}}\)).
Definition 2.5
An operator is called completely continuous if it is continuous and maps bounded sets into precompact sets.
Lemma 2.2
(Arzelà-Ascoli)
A set \(M\subset C(J,R)\) is said to be a precompact set if the following two conditions are satisfied:
-
(i)
All the functions in the set M are uniformly bounded, which means that there exists a constant \(r>0\) such that \(|u(t)|\leq r, \forall t\in J, u\in M\);
-
(ii)
All the functions in the set M are equicontinuous, which means that for every \(\varepsilon>0\), there is \(\delta=\delta (\varepsilon)>0\), which is independent of the function \(u\in M\), such that
$$\bigl|u(t_{1})-u(t_{2})\bigr|< \varepsilon $$
whenever \(|t_{1}-t_{2}|<\delta, t_{1}, t_{2}\in J\).
Lemma 2.3
Suppose D is an open subset of an infinite-dimensional real Banach space E, \(\theta\in D\), and P is a cone of E. If the operator \(\Gamma: P\cap D\rightarrow P\) is completely continuous with \(\Gamma\theta=\theta\) and satisfies
then Γ has a proper element on \(P\cap\partial D\) associated with a positive eigenvalue. That is, there exist \(x_{0}\in P\cap\partial D\) and \(\mu_{0}\) such that \(\Gamma x_{0}=\mu _{0}x_{0}\).
Lemma 2.4
Suppose that P is a normal cone of a real Banach space and \(A: P^{\circ}\rightarrow P^{\circ}\) is an α-concave increasing (or −α-convex decreasing) operator. Then A has exactly one fixed point in \(P^{\circ}\).
3 Some lemmas
Let \(J=[0,1]\). A function \(u(t)\) is said to be a solution of problem (1.1) on J if:
-
(i)
\(u(t)\) is absolutely continuous on each interval \((0,t_{1}]\) and \((t_{k},t_{k+1}]\), \(k=1, 2, \dots, n\);
-
(ii)
for any \(k=1, 2, \dots, n\), \(u(t_{k}^{+})\) and \(u(t_{k}^{-})\) exist, and \(u(t_{k}^{-})=u(t_{k})\);
-
(iii)
\(u(t)\) satisfies (1.1).
We shall reduce problem (1.1) to a system without impulse. To this goal, firstly, by means of the transformation
we convert problem (1.3) into
The following lemmas will be used in the proof of our main results.
Lemma 3.1
Assume that (H1)-(H3) hold. Then
-
(i)
If \(y(t)\) is a solution of problem (3.2) on J, then \(u(t)=c(t)y(t)\) is a solution of problem (1.1) on J;
-
(ii)
If \(u(t)\) is a solution of problem (1.1) on J, then \(y(t)=c^{-1}(t)u(t)\) is a solution of problem (3.2) on J.
Proof
(i) Let \(y(t)\) be a solution of (3.2) on J. It is easy to see that \(u(t)=c(t)y(t)\) is absolutely continuous on each interval \((t_{k},t_{k+1}]\), \(k=1,2,\dots,n\). By the definition of \(c(t)\) we have \(c^{\prime}(t)=0\) for \(t\neq t_{k}\). Then, for \(t\neq t_{k}\), we have
It follows that
For \(t=t_{k}\), we have
By (ii) of Definition 2.2, \(u(t_{k}^{-})=u(t_{k})\), so we have
Thus, \(u(t_{k}^{+})-u(t_{k})=c_{k}u(t_{k})\).
It is obvious that \(u(t)\) satisfies the boundary conditions.
Then \(u(t)\) is a solution of problem (1.1) on J.
(ii) It is easy to see that, for \(t\in J\),
For \(t=t_{k}\),
Then \(y(t)\) is continuous on J. It is easy to prove that \(y(t)\) is absolutely continuous on J and satisfies the boundary conditions.
Then \(y(t)\) is a solution of problem (3.2) on J. □
Lemma 3.2
If (H1)-(H3) hold, then problem (3.2) has a solution y, and y can be expressed in the form
where
Proof
First, suppose that y is a solution of problem (3.2). Integrating problem (3.2) from 0 to t, by the boundary conditions we obtain that
where \(z(s)=\lambda\omega(s)c^{-1}(s)f(c(s)y(s))\).
Integrating (3.6) from 0 to t, we have
Letting \(t=1\) in (3.6) and (3.7), we find
Combining these equalities with (3.7) and the boundary conditions \(ac(1)y(1)+bc(1)y^{\prime}(1)=\int_{0}^{1}g(t)c(t)y(t)\,dt\), we obtain
and further
Therefore, we have
Substituting (3.10) into (3.8), we obtain
Then
and the proof of sufficiency is complete.
Conversely, from (3.3) it is easy to obtain
Lemma 3.2 is proved. □
Lemma 3.3
Let \(\mu\in[0,ac(1))\), G, and H be given as in Lemma 3.2. Then we have the following results:
where \(0\leq e(t)=1-t\leq1\), and
where
Proof
Relation (3.13) is simple to prove. For \(0\leq s\leq t\leq1\), we have
For \(0\leq t\leq s\leq1\), we have
Then,
This gives the proof of (3.14).
For any \(t, s\in J\), by (3.13), (3.14), and (3.16) we have
On the other hand,
Therefore, the proof of (3.15) is complete. □
To obtain some of the norm inequalities in our main results, we employ Hölder’s inequality.
Lemma 3.4
(Hölder)
Let \(e\in L^{p}[a,b]\) with \(p>1\), \(h\in L^{q}[a,b]\) with \(q>1\), and \(\frac{1}{p}+\frac{1}{q}=1\). Then \(eh\in L^{1}[a,b]\) and
Let \(e\in L^{1}[a,b]\), \(h\in L^{\infty}[a,b]\). Then \(eh\in L^{1}[a,b]\), and
Let \(E=C[0,1]\). Then E is a real Banach space with the norm \(\|\cdot\| \) defined by
Define two cones K and \(K_{1}\) in E by
and
where \(\delta=\frac{\alpha^{*}}{\beta^{*}}=\frac{b}{a+b}\). It is easy to see that K and \(K_{1}\) are two solid normal cones and
For \(r>0\), define \(\Omega_{r}\) by
Define T: \(K\rightarrow K\) by
Lemma 3.5
Assume that (H1)-(H3) hold. Then \(T(K)\subset K\), and \(T: K\rightarrow K\) is completely continuous.
Proof
For \(y\in K\), it follows from (3.7) and (3.12) that
Thus, \(T(K)\subset K\).
Next, we prove that the operator \(T: K\rightarrow K\) is completely continuous by standard methods and the Arzelà-Ascoli theorem.
Let \(B_{r}= \{y\in E\mid\|y\|\leq r \}\) be a bounded set. Then, for all \(y\in B_{r}\), we have
where \(L=\max_{\|c(s)y(s)\|\leq c_{M}r}f(c(s)y(s))\). Therefore, \(T(B_{r})\) is uniformly bounded.
On the other hand, noticing that \(H(t,s)\) is uniformly continuous on \(J\times J\), we have that, for any \(\varepsilon>0\), there exists \(\delta_{1}>0\) such that if \(| t_{1}-t_{2}|<\delta_{1}\), then
Then, for any \(y\in B_{r}\), taking \(| t_{1}-t_{2}|<\delta_{1}\), we get
Thus, the set \(\{T: y\in B_{r} \}\) is equicontinuous. The Arzelà-Ascoli theorem implies that T is completely continuous, and Lemma 3.5 is proved. □
4 Existence and nonexistence of positive solutions on a parameter
In this section, we establish some sufficient conditions for the existence and nonexistence of positive solutions of problem (1.1). We consider the following three cases for \(\omega\in L^{p}[0,1]: p>1, p=1\), and \(p=\infty\). The case \(p>1\) is treated in the following theorem.
Theorem 4.1
Assume that (H1)-(H3) hold. If \(0< f_{\infty }<+\infty\), then there exists \(R_{0}>0\) such that for any \(r>R_{0}\), problem (1.1) has a positive solution \(u_{r}(t)\) satisfying \(\| u_{r}(t)\|=c_{M}r\) for any
where \(\lambda_{1}\) and \(\lambda_{2}\) are two positive finite numbers.
Proof
By (3.3) and (3.18) problem (1.1) has a positive solution \(u_{r}(t)\) associated with \(\lambda>0\) if and only if the operator T has a proper element \(y_{r}\) associated with the eigenvalue \(\frac {1}{\lambda}>0\).
Since \(0< f_{\infty}<+\infty\), there exist \(l_{2}>l_{1}>0\) and \(\eta>0\) such that
Now, we prove that \(R_{0}=\frac{\eta}{c_{m}\delta}\) is required. Thus, for all \(r>R_{0}\), if \(y\in K\cap\partial\Omega_{r}\), we have
Noticing \(r>R_{0}\), we have
Together with Lemma 3.5, we have that \(T: K\cap\bar{\Omega }_{r}\rightarrow K\) is completely continuous with \(T\theta=\theta\). In addition,
Therefore, for any \(r>R_{0}\) and \(y\in K\cap\partial\Omega_{r}\), we have
By Lemma 2.3, for any \(r>R_{0}\), the operator T has a proper element \(y_{r}\in K\) associated with the eigenvalue \(\gamma>0\); further, \(y_{r}\) satisfies \(\|y_{r}\|=r\). Let \(\lambda=\frac{1}{\gamma}\). Then problem (3.2) has a positive solution \(y_{r}(t)\) associated with λ.
Hence, it follows from Lemma 3.1 that problem (1.1) has a positive solution \(u_{r}(t)\) associated with λ and satisfying \(\|u_{r}\|=c_{M}r\).
From the proof above, for any \(r>R_{0}\), there exists a positive solution \(y_{r}\in K\cap\partial\Omega_{r}\) associated with \(\lambda >0\), that is,
with \(\|y_{r}\|=r\).
On the one hand,
and, further,
which means that
On the other hand,
and thus
which leads to
It is easy to see by calculating that \(\lambda_{1}<\lambda_{2}\).
In conclusion, \(\lambda\in[\lambda_{1},\lambda_{2}]\). The proof is complete. □
The following Corollary 4.1 deals with the case \(p=\infty\).
Corollary 4.1
Assume that (H1)-(H3) hold. If \(0< f_{\infty}<+\infty\), then there exists \(R_{1}>0\) such that for any \(r>R_{1}\), problem (1.1) has a positive solution \(u_{r}(t)\) satisfying \(\|u_{r}(t)\|=c_{M}r\) for any
where
Proof
Replacing \(\|h\|_{q}\|\omega\|_{p}\) by \(\|h\|_{1}\|\omega\| _{\infty}\) and repeating the argument above, we get the corollary. □
Finally, we consider the case of \(p=1\).
Corollary 4.2
Assume that (H1)-(H3) hold. If \(0< f_{\infty}<+\infty\), then there exists \(R_{2}>0\) such that for any \(r>R_{2}\), problem (1.1) has a positive solution \(u_{r}(t)\) satisfying \(\|u_{r}(t)\|=c_{M}r\) for any
where
Proof
Replacing \(\beta^{\prime}\|h\|_{q}\|\omega\|_{p}\) by \(\beta ^{*}\|\omega\|_{1}\) and repeating the argument above, we get the corollary. □
In the following theorems, we only consider the case \(1< p<+\infty\).
Theorem 4.2
Assume that (H1)-(H3) hold. If \(f_{\infty }=+\infty\), then there exists \(R_{3}>0\) such that for any \(r>R_{3}\), problem (1.1) has a positive solution \(u_{r}(t)\) satisfying \(\|u_{r}\| =c_{M}r\) for any
where \(\lambda_{3}\) is a positive finite number.
Proof
Similarly to the proof of Theorem 4.1, it is easy to see from (4.2) and (4.3) that Theorem 4.2 is also true. □
Theorem 4.3
Assume that (H1)-(H3) hold. If \(0< f_{0}<+\infty\), then there exists \(r_{0}>0\) such that for any \(0< r< r_{0}\), problem (1.1) has a positive solution \(u_{r}(t)\) satisfying \(\|u_{r}(t)\|=c_{M}r\) for any
where \(\hat{\lambda}_{1}\) and \(\hat{\lambda}_{2}\) are two positive finite numbers.
Proof
By (3.3) and (3.18) problem (1.1) has a positive solution \(u_{r}(t)\) associated with \(\lambda>0\) if and only if the operator T has a proper element \(y_{r}\) associated with the eigenvalue \(\frac {1}{\lambda}>0\).
Since \(0< f_{0}<+\infty\), there exist \(\eta^{\prime}>0\) and constants \(c_{2}>c_{1}>0\) such that
Set
where \(0< r< r_{0}\).
Then \(U_{r}\) is a bounded open subset of the Banach space E, and \(\theta\in U_{r}\).
Now, we prove that \(r_{0}=\frac{\eta'}{c_{M}}\) is required.
Thus, for \(y\in K\cap\partial U_{r}\), noticing \(0< r< r_{0}\), we have
and
Together with Lemma 3.5, we note that \(T: K\cap\bar{U}_{r}\rightarrow K\) is completely continuous with \(T\theta=\theta\) and that
So, for any \(0< r< r_{0}\) and \(y\in K\cap\partial U_{r}\), we have
By Lemma 2.3, for any \(0< r< r_{0}\), the operator T has a proper element \(y_{r}\in K\) associated with the eigenvalue \(\gamma>0\); further, \(y_{r}\) satisfies \(\|y_{r}\|=r\). Letting \(\lambda=\frac {1}{\gamma}\) and following the proof of Theorem 4.1, we complete the proof of Theorem 4.3. □
Theorem 4.4
Assume that (H1)-(H3) hold. If \(f_{0}=+\infty\), then there exists \(r_{1}>0\) such that for any \(0< r< r_{1}\), problem (1.1) has a positive solution \(u_{r}(t)\) satisfying \(\|u_{r}\|=c_{M}r\) for any
where \(\hat{\lambda}_{3}\) is a positive finite number.
Proof
The proof is similar to that of Theorem 4.3, so we omit it here. □
Theorem 4.5
Assume that (H1)-(H3) hold. If \(f_{0}=f_{\infty}=+\infty\), then there exists \(\bar{\lambda}>0\) such that problem (1.1) has no positive solutions for all \(\lambda\in[\bar {\lambda},+\infty)\).
Proof
We argue by contradiction. Suppose that there exists a sequence \(\{\lambda_{n}\}\) with \(\lambda_{n}>n\) such that for each n, problem (3.2) has a positive solution \(y_{n}\in K\). Let \(\mu_{n}=\frac {1}{\lambda_{n}}\). Since \((Ty_{n})(t)=\mu_{n} y_{n}(t)\) for \(t\in J\) and \(f(u)\geq Nu\) for all \(u>0\), where \(N=\frac{f(R)}{R}\), we have
which implies that \(1>n\alpha^{*}\xi c_{M}^{-1}Nc_{m}\delta\).
Since n may be arbitrarily large, we obtain a contradiction.
Therefore, by Lemma 3.1 problem (1.1) has no positive solutions for all \(\lambda\geq\bar{\lambda}\). This gives the proof of Theorem 4.5. □
Theorem 4.6
Assume that (H1)-(H3) hold. If \(f_{0}=f_{\infty}=0\), then there exists \(\underline{\lambda}>0\) such that problem (1.1) has no positive solutions for \(\lambda\in (0,\underline{\lambda})\).
Proof
It follows from \(f_{0}=f_{\infty}=0\) and (1.4) that there exists \(\bar{v}_{0}>0\) such that
Let
Then \(\mathbf{M}>0\) and
Let \(y(t)\) be a positive solution of problem (3.2). We will show that this leads to a contradiction for \(\lambda<\underline{\lambda}\), where \(\underline {\lambda}= (\beta^{\prime}\|h\|_{q}\|\omega\|_{p}c_{m}^{-1}c_{M}\mathbf {M} )^{-1}\). Let \(\mu=\frac{1}{\lambda}\). Since \((Ty)(t)=\mu y(t)\) for \(t\in J\), it follows from (3.18) that
which shows that
which is a contradiction. This finishes the proof. □
Remark 4.1
The method to study the existence and nonexistence results of positive solutions is completely different from those of Zhang and Feng [18].
5 Uniqueness and continuity of positive solution on a parameter
In the previous section, we have established some existence and nonexistence criteria of positive solutions for problem (1.1). Next, we consider the uniqueness and continuity of positive solutions on a parameter for problem (1.1).
Theorem 5.1
Suppose that \(f(u): [0,+\infty)\rightarrow[0,+\infty )\) is a nondecreasing function with \(f(u)>0\) for \(u>0\) and satisfies \(f(\rho u)\geq\rho^{\alpha}f(u)\) for any \(0<\rho<1\), where \(0\leq\alpha <1\). Then, for any \(\lambda\in(0,\infty)\), problem (1.1) has a unique positive solution \(u_{\lambda}(t)\). Furthermore, such a solution \(u_{\lambda}(t)\) satisfies the following properties:
-
(i)
\(u_{\lambda}(t)\) is strongly increasing in λ, that is, \(\lambda_{1}>\lambda_{2}>0\) implies \(u_{\lambda_{1}}(t)\gg u_{\lambda _{2}}(t)\) for \(t\in J\).
-
(ii)
\(\lim_{\lambda\rightarrow0^{+}}\|u_{\lambda}\|=0, \lim_{\lambda\rightarrow+\infty}\|u_{\lambda}\|=+\infty\).
-
(iii)
\(u_{\lambda}(t)\) is continuous with respect to λ, that is, \(\lambda\rightarrow\lambda_{0}>0\) implies \(\|u_{\lambda}-u_{\lambda _{0}}\|\rightarrow0\).
Proof
Set \(\Psi=\lambda T\), where T is the same as in (3.18). Similarly to Lemma 3.5, the operator Ψ maps \(K_{1}\) into \(K_{1}\). In view of \(H(t,s)>0, \omega(s)>0, c^{-1}(s)>0\), and \(f(u)>0\) for \(u>0\), it is easy to see that \(\Psi: K_{1}^{0}\rightarrow K_{1}^{0}\). We assert that \(\Psi: K_{1}^{0}\rightarrow K_{1}^{0}\) is an α-concave increasing operator. Indeed,
where \(0\leq\alpha<1\). Since \(f(u)\) is nondecreasing, we have
In view of Lemma 2.4, Ψ has a unique fixed point \(y_{\lambda}\in K_{1}^{0}\). This shows that problem (3.2) has a unique positive solution \(y_{\lambda}(t)\). It follows from Lemma 3.1 that problem (1.1) has a unique positive solution \(u_{\lambda}(t)\).
Next, we give a proof for (i)-(iii). Let \(\gamma=\frac{1}{\lambda}\) and denote \(\lambda Ty_{\lambda}=y_{\lambda}\) by \(Ty_{\gamma}=\gamma y_{\gamma}\). Assume that \(0<\gamma_{1}<\gamma_{2}\). Then \(y_{\gamma _{1}}\geq y_{\gamma_{2}}\). Indeed, set
We assert \(\bar{\eta}\geq1\). If this is not true, then \(0<\bar{\eta }<1\), and further
which implies
This is a contradiction to (5.1).
In view of the discussion above, we have
Hence, \(y_{\gamma}(t)\) is strongly decreasing in γ. Namely, \(y_{\lambda}(t)\) is strongly increasing in λ. By Lemma 3.1, (i) is proved.
Setting \(\gamma_{2}=\gamma\) and fixing \(\gamma_{1}\) in (5.2), we have \(y_{\gamma_{1}}\geq\frac{\gamma}{\gamma_{1}}y_{\gamma}\) for \(\gamma >\gamma_{1}\). Further,
where \(N_{1}>0\) is a normal constant. Noting that \(\gamma=\frac {1}{\lambda}\), we have \(\lim_{\lambda\rightarrow0^{+}}\| y_{\lambda}(t)\|=0\). Then it follows from Lemma 3.1 that \(\lim_{\lambda\rightarrow 0^{+}}\|u_{\lambda}(t)\|=0\).
Similarly, letting \(\gamma_{1}=\gamma\) and fixing \(\gamma_{2}\), again by (5.2) and the normality of \(K_{1}\) we have \(\lim_{\lambda \rightarrow+\infty}\|y_{\lambda}(t)\|=+\infty\). Then, it follows from Lemma 3.1 that \(\lim_{\lambda\rightarrow +\infty}\|u_{\lambda}(t)\|=+\infty\).
This gives the proof of (ii).
Next, we show the continuity of \(u_{\gamma}(t)\). For given \(\gamma _{0}>0\), by (i),
Let \(l_{\gamma}=\sup \{\nu>0 \mid y_{\gamma}\geq\nu y_{\gamma _{0}}, \gamma>\gamma_{0} \}\). Obviously, \(0< l_{\gamma}<1\) and \(y_{\gamma}\geq l_{\gamma}y_{\gamma_{0}}\). So, we have
and further
By the definition of \(l_{\gamma}\),
Again by the definition of \(l_{\gamma}\), we have
Noticing that \(K_{1}\) is a normal cone, in view of (5.4) and (5.5), we obtain
In the same way,
where \(N_{2}>0\) is a normal constant.
Therefore, by Lemma 3.1 we have
Consequently, (iii) holds. The proof is complete. □
6 Remarks and comments
In this section, we offer some remarks and comments on the associated problem (1.1).
Remark 6.1
Some ideas of the proof of Theorem 5.1 come from Theorem 2.2.7 in [17] and Theorem 6 in [19], but there are almost no papers considering the uniqueness of positive solution for second impulsive differential equations, especially in the case where \(\omega (t)\) is \(L^{p}\)-integrable.
Remark 6.2
Generally, it is difficult to study the uniqueness of a positive solution for nonlinear second-order differential equations with or without impulsive effects (see, e.g., [4, 5, 20] and references therein).
For example, we consider the following problem:
where \(\lambda>0\) is a positive parameter, \(J=[0,1], \omega\in L^{p}[0,1]\) for some \(1\leq p\leq+\infty, f\in C(J\times R^{+},R^{+}), R^{+}=[0,+\infty)\), \(t_{k}\) (\(k=1,2,\dots,n\)) are fixed points with \(0< t_{1}< t_{2}<\cdots <t_{k}<\cdots<t_{n}<1\), \(\{c_{k}\}\) is a real sequence with \(c_{k}>-1, k=1,2,\ldots,n\), \(x(t_{k}^{+})\) (\(k=1,2,\ldots,n\)) is the right-hand limit of \(x(t)\) at \(t_{k}\), and \(h\in C[0,1]\) is nonnegative.
By means of transformation (3.1) we can convert problem (6.1) into
Using a proof similar to that of Lemma 3.2, we can obtain the following results.
Lemma 6.1
If (H1)-(H3) hold, then problem (6.2) has a solution y, and y can be expressed in the form
where
It is not difficult to prove that \(H^{*}(t,s)\) and \(G^{*}(t,s)\) have similar properties to those of \(H(t,s)\) and \(G(t,s)\). However, we cannot guarantee that \(H^{*}(t,s)>0\) for any \(t,s\in J\). This implies that we cannot apply Lemma 2.4 to study the uniqueness of a positive solution for problem (6.1).
Remark 6.3
In Theorem 5.1, even though we do not assume that T is completely continuous or even continuous, we can assert that \(u_{\lambda}\) depends continuously on λ.
Remark 6.4
If we replace \(K_{1}, K_{1}^{0}\) by \(K, K^{0}\), respectively, then Theorem 5.1 also holds.
7 Examples
To illustrate how our main results can be used in practice, we present two examples.
Example 7.1
Let \(n=1, t_{1}=\frac{1}{2}, p=3\). It follows from \(p=3\) that \(q=\frac{3}{2}\). Consider the following boundary value problem:
Conclusion
Problem (7.1) has at least one positive solution for any \(\lambda\in[0.0056, 0.09]\).
Proof
Problem (7.1) can be regarded as a problem of the form (1.1), where
and
We convert problem (7.1) into
where
From \(\omega(t)=\frac{1}{|t-\frac{1}{3}|^{\frac{1}{4}}}, t\in J\), choosing \(p=3, q=\frac{3}{2}\), it follows that
Thus, it is easy to see by calculating that \(\omega(t)\geq\xi=\sqrt [4]{\frac{3}{2}}\) for a.e. \(t\in J\) and that
and
Therefore, it follows from the definitions \(\omega(t), f\), and g that (H1)-(H3) hold and
so \(0< f_{\infty}=6<+\infty\).
Thus, we have
Set \(l_{1}=5\) and \(l_{2}=7\). Then
Hence, by Theorem 4.1 the conclusion follows, and the proof is complete. □
Example 7.2
Let \(n=1, t_{1}=\frac{1}{2}, p=1\). It follows from \(p=1\) that \(q=\infty\). Consider the following boundary value problem:
Conclusion
Problem (7.3) has at least one positive solution for any \(\lambda\in[\frac{1}{504}, \frac{1}{30}]\).
Proof
Problem (7.3) can be regarded as a problem of the form (1.1), where
and
We convert problem (7.3) into
where
Thus, it is easy to see by calculating that \(\omega(t)\geq\xi=3\) for a.e. \(t\in J\) and that
and
Therefore, it follows from the definitions \(\omega(t), f\), and g that (H1)-(H3) hold.
On the other hand, it follows from \(\omega(t)=2t+3\) that
Thus, we have
Set \(l_{1}=5\) and \(l_{2}=7\). Then
Hence, by Corollary 4.2 the conclusion follows, and the proof is complete. □
References
Zhang, X, Feng, M: Transformation techniques and fixed point theories to establish the positive solutions of second-order impulsive differential equations. J. Comput. Appl. Math. 271, 117-129 (2014)
Guo, D: Multiple positive solutions of impulsive nonlinear Fredholm integral equations and applications. J. Math. Anal. Appl. 173, 318-324 (1993)
Agarwal, RP, Franco, D, O’Regan, D: Singular boundary value problems for first and second order impulsive differential equations. Aequ. Math. 69, 83-96 (2005)
Lin, X, Jiang, D: Multiple solutions of Dirichlet boundary value problems for second order impulsive differential equations. J. Math. Anal. Appl. 321, 501-514 (2006)
Feng, M, Xie, D: Multiple positive solutions of multi-point boundary value problem for second-order impulsive differential equations. J. Comput. Appl. Math. 223, 438-448 (2009)
Li, Q, Cong, F, Jiang, D: Multiplicity of positive solutions to second order Neumann boundary value problems with impulse actions. Appl. Math. Comput. 206, 810-817 (2008)
Zhou, Q, Jiang, D, Tian, Y: Multiplicity of positive solutions to period boundary value problems for second order impulsive differential equations. Acta Math. Appl. Sinica (Engl. Ser.) 26, 113-124 (2010)
Liu, Y, O’Regan, D: Multiplicity results using bifurcation techniques for a class of boundary value problems of impulsive differential equations. Commun. Nonlinear Sci. Numer. Simul. 16, 1769-1775 (2011)
Ma, R, Yang, B, Wang, Z: Positive periodic solutions of first-order delay differential equations with impulses. Appl. Math. Comput. 219, 6074-6083 (2013)
Hao, X, Liu, L, Wu, Y: Positive solutions for second order impulsive differential equations with integral boundary conditions. Commun. Nonlinear Sci. Numer. Simul. 16, 101-111 (2011)
Feng, M: Positive solutions for a second-order p-Laplacian boundary value problem with impulsive effects and two parameters. Abstr. Appl. Anal. 2014, 4 (2014)
Feng, M, Qiu, J: Multi-parameter fourth order impulsive integral boundary value problems with one-dimensional m-Laplacian and deviating arguments. J. Inequal. Appl. 2015, 64 (2015)
Zhou, J, Feng, M: Green’s function for Sturm-Liouville-type boundary value problems of fractional order impulsive differential equations and its application. Bound. Value Probl. 2014, 69 (2014)
Liu, X, Guo, D: Method of upper and lower solutions for second-order impulsive integro-differential equations in a Banach space. Comput. Math. Appl. 38, 213-223 (1999)
Lu, G, Feng, M: Positive Green’s function and triple positive solutions of a second-order impulsive differential equation with integral boundary conditions and a delayed argument. Bound. Value Probl. 2016, 88 (2016)
Sánchez, J: Multiple positive solutions of singular eigenvalue type problems involving the one-dimensional p-Laplacian. J. Math. Anal. Appl. 292, 401-414 (2004)
Guo, D, Lakshmikantham, V: Nonlinear Problems in Abstract Cones. Academic Press, New York (1988)
Zhang, X, Feng, M: Existence of a positive solution for one-dimensional singular p-Laplacian problems and its parameter dependence. J. Math. Anal. Appl. 413, 566-582 (2014)
Liu, X, Li, W: Existence and uniqueness of positive periodic solutions of functional differential equations. J. Math. Anal. Appl. 293, 28-39 (2004)
Zhou, J, Feng, M: Triple positive solutions for a second order m-point boundary value problem with a delayed argument. Bound. Value Probl. 2015, 178 (2015)
Acknowledgements
This work is sponsored by the National Natural Science Foundation of China (11301178, 11371117), the Beijing Natural Science Foundation of China (1163007), and the Scientific Research Project of Construction for Scientific and Technological Innovation Service Capacity (71E1610973). The authors are grateful to anonymous referees for their constructive comments and suggestions, which have greatly improved this paper.
Author information
Authors and Affiliations
Corresponding author
Additional information
Competing interests
The authors declare that they have no competing interests.
Authors’ contributions
All results belong to YT and XZ. Both authors read and approved the final manuscript.
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 4.0 International License (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted use, distribution, and reproduction in any medium, provided you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made.
About this article
Cite this article
Tian, Y., Zhang, X. Existence and continuity of positive solutions on a parameter for second-order impulsive differential equations. Bound Value Probl 2016, 163 (2016). https://doi.org/10.1186/s13661-016-0672-x
Received:
Accepted:
Published:
DOI: https://doi.org/10.1186/s13661-016-0672-x