1 Introduction

Let \({\mathcal {H}}\) denote the class of analytic functions in the unit disc \(|z|<1\) on the complex plane \({\mathbb{C}}\). The following lemma is a particular case of the Julia-Wolf theorem. It is known as Jack’s lemma.

Lemma 1.1

([1])

Let \(\omega(z)\in{\mathcal {H}}\) with \(\omega(0)=0\). If for a certain \(z_{0}\), \(|z_{0}|<1\), we have \(|\omega(z)|\leq|\omega(z_{0})|\) for \(|z|\leq|z_{0}|\), then \(z_{0}\omega'(z_{0})=m\omega(z_{0})\), \(m\geq 1\).

In this paper, we consider a related problem. We establish a relation between \(w(z)\) and \(zw'(z)\) at the point \(z_{0}\) such that \(|w(z_{0})|=\min \{|w(z)|:|z|= |z_{0}| \}\) or at the point \(z_{0}\) satisfying (1.1). We consider the p-valent functions.

Lemma 1.2

Let \(w(z) = z^{p} + \sum_{n=p+1} ^{\infty}a_{n} z^{n}\) be analytic in \(|z| < 1\). Assume that there exists a point \(z_{0} \), \(|z_{0}|=R<1\), such that

$$ \min \bigl\{ \big|w(z)\big|: w(z)\in\partial w\bigl(|z|\leq R\bigr) \bigr\} = \big|w(z_{0})\big|>0. $$
(1.1)

If \(w(z)/z^{p}\neq0\) in \(|z| < R\), then

$$ \frac{z_{0}w'(z_{0})}{w(z_{0})}=k_{1}\leq p. $$
(1.2)

If the function \(w(z)/z^{p}\) has a zero in \(|z| < R\) and \(\partial w(|z|\leq R)\) is a smooth curve at \(w(z_{0})\), then

$$ \frac{z_{0}w'(z_{0})}{w(z_{0})}=k_{2}\geq p, $$
(1.3)

where \(k_{1}\), \(k_{2}\) are real.

Proof

If

$$ \min \bigl\{ \big|w(z)\big|: w(z)\in\partial w\bigl(|z|\leq R\bigr) \bigr\} = \big|w(z_{0})\big|>0, $$

then

$$ \bigl\vert w(z) \bigr\vert \geq \bigl\vert w(z_{0}) \bigr\vert \quad\text{for } w(z)\in\partial w\bigl(|z|\leq R\bigr). $$
(1.4)

Then, we also have

$$ \biggl\vert \frac{w(z)}{z^{p}} \biggr\vert \geq \biggl\vert \frac{w(z_{0})}{z^{p}_{0}} \biggr\vert \quad\text{for } w(z)\in\partial w\bigl(|z|\leq R\bigr). $$
(1.5)

Let

$$ \Phi(z) = w(z)/z^{p}, \quad|z|< 1. $$
(1.6)

Then, from (1.5) and from hypothesis (1.1) we have

$$ \min \bigl\{ \big|\Phi(z)\big|:\Phi(z)\in\partial\Phi\bigl(|z|\leq R\bigr) \bigr\} = \big| \Phi(z_{0})\big|. $$
(1.7)

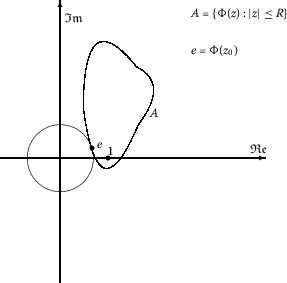
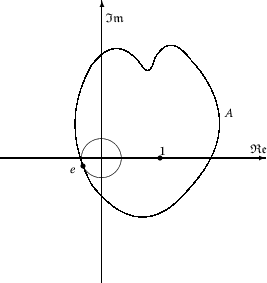
There are two cases: \(\Phi(|z|< R)\) contains the origin (see Figure 2); and \(\Phi(|z|< R)\) does not (see Figure 1).

Figure 1
figure 1

\(\pmb{\Phi(z)}\) in the case \(\pmb{\Phi(z)\neq0}\) .

Full size image

First, suppose that \(\Phi(z)\) does not become 0 in \(|z| < R\). If there exists a point \(z_{0}=R\exp(i\varphi_{0})\), \(0\leq\varphi_{0}<2\pi\), \(0 < R< 1\), such that

$$ \min \bigl\{ \big|\Phi(z)\big|: \Phi(z)\in\partial\Phi\bigl(|z|\leq R\bigr) \bigr\} = \big|\Phi(z_{0})\big|, $$
(1.8)

then the function

$$ F(z)=\frac{z}{\Phi(z)}=\frac{z^{p+1}}{w(z)}, \quad|z| \leq R, $$

satisfies the assumptions of Jack’s lemma (Lemma 1.1),

$$ F(z_{0})=\max_{\theta\in[0,2\pi)} \bigl\{ \big|F(z)\big|: z=Re^{i\theta} \bigr\} , $$

and hence

$$ \frac{z_{0}F'(z_{0})}{F(z_{0})}=p+1-\frac{z_{0}w'(z_{0})}{w(z_{0})}\geq m\geq1. $$

This gives (1.2).

For the case \(0\in\Phi(|z|< R)\) (see Figure 2), for \(\Phi(z)\) given in (1.6), we have that \(|\Phi(z)|\) has an extremum at \(z_{0}\), and so

$$ \frac{\mathrm{d}|\Phi(z)|}{\mathrm{d}\varphi} \bigg\vert _{z=z_{0}}=0. $$
(1.9)

Furthermore, \(\arg \{\Phi(z) \}\) is increasing at \(z_{0}\), and so

$$ \frac{\mathrm{d}\arg \{\Phi(z) \}}{\mathrm{d}\varphi} \bigg\vert _{z=z_{0}}\geq0. $$
(1.10)
Figure 2
figure 2

\(\pmb{\Phi(z)}\) in the case \(\pmb{0\in\Phi(|z|\leq R)}\) .

Full size image

Then we have

$$\begin{aligned}[b] \frac{z_{0}\Phi'(z_{0})}{\Phi(z_{0})} &= \frac{\mathrm{d}\log\Phi (z)}{\mathrm{d}\log z} \bigg\vert _{z=z_{0}} \\ &= \frac{\mathrm{d}\log|\Phi(z)|+i\mathrm{d}\arg \{\Phi(z) \} }{i\mathrm{d}\varphi} \bigg\vert _{z=z_{0}} \\ &= \frac{\mathrm{d}\arg \{\Phi(z) \}}{\mathrm{d}\varphi}- \frac{i}{|\Phi(z)|}\frac{\mathrm{d}|\Phi(z)|}{\mathrm{d}\varphi} \bigg\vert _{z=z_{0}} \\ &= \frac{\mathrm{d}\arg \{\Phi(z) \}}{\mathrm{d}\varphi} \bigg\vert _{z=z_{0}} \\ &\geq0,\end{aligned} $$
(1.11)

because of (1.9). On the other hand, by (1.6) we have \(w'(z)=z^{p}\Phi'(z)+pz^{p-1}\Phi(z)\), and hence

$$ \frac{z_{0}w'(z_{0})}{w(z_{0})}=\frac{z_{0}\Phi'(z_{0})}{\Phi(z_{0})}+p. $$
(1.12)

Relations (1.11) and (1.12) imply that

$$ \frac{z_{0}w'(z_{0})}{w(z_{0})}\geq p. $$

Therefore, by (1.11) we obtain (1.3). □

If we additionally assume that \(w(z)/z^{p}\) is univalent in the unit disc, then we have the following result.

Remark 1.3

Let \(w(z) = z^{p} + \sum_{n=p+1} ^{\infty}a_{n} z^{n}\) be analytic in \(|z| < 1\). Assume that there exists a point \(z_{0} \), \(|z_{0}|=R<1\), such that

$$ \min_{\theta\in[0,2\pi)} \bigl\{ \big|w(z)\big|: z=Re^{i\theta} \bigr\} = \big|w(z_{0})\big|>0. $$
(1.13)

If \(w(z)/z^{p}\) is univalent and \(w(z)/z^{p}\neq0\) in \(|z| \leq R\), then

$$ \frac{z_{0}w'(z_{0})}{w(z_{0})}=k_{1}\leq p, $$
(1.14)

where \(k_{1}\) is real. If \(w(z)/z^{p}\) is univalent and \(w(z)/z^{p}\) vanishes in \(|z| \leq R\), then

$$ \frac{z_{0}w'(z_{0})}{w(z_{0})}=k_{2}\geq p, $$
(1.15)

where \(k_{2}\) is real.

2 Applications

For \(p=0\), then Lemma 1.2 becomes the following corollary.

Corollary 2.1

Let \(w(z) = 1 + \sum_{n=1} ^{\infty}a_{n} z^{n}\) be analytic in \(|z| < 1\). Assume that there exists a point \(z_{0} \), \(|z_{0}|=R<1\), such that

$$ \min \bigl\{ \big|w(z)\big|: w(z)\in\partial w\bigl(|z|\leq R\bigr) \bigr\} = \big|w(z_{0})\big|>0. $$
(2.1)

If \(w(z)\neq0\) in \(|z| < R\), then

$$ \frac{z_{0}w'(z_{0})}{w(z_{0})}=k_{1}\leq0. $$
(2.2)

If the function \(w(z)\) has a zero in \(|z| < R\) and \(\partial w(|z|\leq R)\) is a smooth curve at \(w(z_{0})\), then

$$ \frac{z_{0}w'(z_{0})}{w(z_{0})}=k_{2}\geq0. $$
(2.3)

A simple contraposition of Lemma 1.2 provides the following two corollaries.

Corollary 2.2

Let \(w(z) = z^{p} + \sum_{n=p+1} ^{\infty}a_{n} z^{n}\) be analytic in \(|z| < 1\) and suppose that there exists a point \(z_{0} \), \(|z_{0}|=R<1\), such that

$$ \min \bigl\{ \big|w(z)\big|: w(z)\in\partial w\bigl(|z|\leq R\bigr) \bigr\} = \big|w(z_{0})\big|>0. $$
(2.4)

If

$$ \frac{z_{0}w'(z_{0})}{w(z_{0})}=k_{1}< p $$
(2.5)

and \(\partial w(|z|\leq R)\) is a smooth curve at \(w(z_{0})\), then \(w(z)/z^{p}\) has no zero in \(|z| \leq R\). If

$$ \frac{z_{0}w'(z_{0})}{w(z_{0})}=k_{2}> p, $$
(2.6)

then the function \(w(z)/z^{p}\) has a zero in \(|z| \leq R\).

Corollary 2.3

Let \(q(z)=z^{p}+\sum_{n=p+1}^{\infty}a_{n} z^{n}\) be analytic in \(|z|\leq1\). Assume that \(q(z)/z^{p}\) has a zero in \(|z|<1\). If for given \(c\in[0,1)\),

$$ \big|zq'(z)\big|< \frac{p}{c}\big|q(z)\big|^{2},\quad |z| < 1, $$
(2.7)

then the image domain \(q(|z|<1)\) covers the disc \(|w|< c\).

Proof

If

$$ \min \bigl\{ \big|q(z)\big|: q(z)\in\partial q\bigl(|z|\leq1\bigr) \bigr\} = \big|q(z_{0})\big|< c, $$
(2.8)

then by (1.2) in Lemma 1.2 we have

$$ \frac{z_{0}q'(z_{0})}{q(z_{0})}=k\geq p\quad \Rightarrow \quad\big|z_{0}q'(z_{0})\big| \geq p\big|q(z_{0})\big|. $$
(2.9)

Therefore, by (2.8) and (2.9) we have

$$ \big|z_{0}q'(z_{0})\big|\geq\frac{p}{c}\big|q(z_{0})\big|^{2}, $$

which contradicts hypothesis (2.7) and therefore completes the proof. □

Theorem 2.4

Let \(p(z)\) be analytic in \(|z|<1\) with \(p(z) \neq0\), \(|p(0)|>c\), in \(|z| < 1\) and suppose that

$$ \big|p(z) + zp'(z)\big|>c,\quad |z| < 1, $$
(2.10)

where \(c>0\), and that

$$ \mathfrak{Re} \biggl\{ \frac{zp'(z)}{p(z)} \biggr\} >-2, \quad |z| < 1. $$
(2.11)

Then we have

$$ \big|p(z)\big|> c, \quad |z| < 1. $$
(2.12)

Proof

If there exists a point \(z_{0}\), \(|z_{0}| < 1\), such that

$$ \big|p(z)\big| > c \quad\text{for } |z| < |z_{0}| $$
(2.13)

and \(| p(z_{0}) | = c\), then \(p(|z|\leq|z_{0}|)\) has the shape as in Figure 1 and \(\mathrm{d}|p(z)|/\mathrm{d}\varphi\), \(z=re^{i\varphi}\), vanishes at the point \(z=z_{0}\). Therefore, we have

$$ \begin{aligned}[b]\frac{z_{0}p'(z_{0})}{p(z_{0})}&= \frac{\mathrm{d}\log p(z)}{\mathrm{d}\log z} \bigg\vert _{z=z_{0}} \\ &= \frac{\mathrm{d}\log|p(z)|+i\mathrm{d}\arg \{p(z) \} }{i\mathrm{d}\varphi} \bigg\vert _{z=z_{0}} \\ &= \frac{\mathrm{d}\arg \{p(z) \}}{\mathrm{d}\varphi}- \frac{i}{|p(z)|}\frac{\mathrm{d}|p(z)|}{\mathrm{d}\varphi} \bigg\vert _{z=z_{0}} \\ &= \frac{\mathrm{d}\arg \{p(z) \}}{\mathrm{d}\varphi} \bigg\vert _{z=z_{0}} \\ &\leq0.\end{aligned} $$
(2.14)

From (2.11) and (2.14) we have

$$ -2< \frac{z_{0}p'(z_{0})}{p(z_{0})}\leq0, $$

and hence

$$ 0\leq \biggl\vert 1 + \frac{z_{0}p'(z_{0})}{p(z_{0})} \biggr\vert \leq1. $$

Then it follows that

$$ \big|p(z_{0}) + z_{0}p'(z_{0})\big| =\big|p(z_{0})\big| \biggl\vert 1 + \frac{z_{0}p'(z_{0})}{p(z_{0})} \biggr\vert \leq\big| p(z_{0})\big| = c, $$
(2.15)

which contradicts hypothesis (2.10) and therefore completes the proof. □

For some other geometrical properties of analytic functions, we refer to the papers [2–4].

3 Conclusion

In this paper, we have presented a correspondence between an analytic function \(f(z)\) and \(zf'(z)\) at the point w, \(0<|w|=R< 1\), in the unit disc \(|z|<1\) on the complex plane such that \(|f(w)|=\min \{|f(z)|: f(z)\in\partial f(|z|\leq R) \}\).