1 Introduction

We use Bondy and Murty [1] for terminology and notations not defined in this paper and consider undirected and simple graphs only. Let \(G=(V, E)\) be a graph with order n. Denote by \(m(G,t)\) the number of t-matchings of G. Clearly, \(m(G,1)=e(G)\), the size of G, and \(m(G,t)=0\) for \(t > \lfloor n/2\rfloor\).

Recall that the matching polynomial of a graph G is defined as

$$\alpha(G)=\alpha(G,\lambda)=\sum_{t\geq0}(-1)^{t} m(G,t)\lambda^{n-2t} $$

and its theory is well elaborated in [2–4].

The energy of G is defined as

$$ E(G)=\sum_{i=1}^{n}|\lambda_{i}|, $$

where \(\lambda_{1}, \lambda_{2},\ldots, \lambda_{n}\) are the eigenvalues of the adjacency matrix \(A(G)\) of G. The theory of graph energy is well developed nowadays; for details, see reviews [5, 6], the book [7], and the recent related results [8–23].

The Coulson integral formula [24] plays an important role in the study of the graph energy. For an acyclic graph T, its Coulson integral formula is as follows:

$$ E(T)=\frac{2}{\pi} \int_{0}^{+\infty}\frac{1}{x^{2}}\ln \biggl[\sum _{t\geq 0} m(T,t)x^{2t} \biggr]\,dx. $$
(1)

Motivated by equation (1), Gutman and Wagner [25] defined the matching energy of a graph G as

$$ \mathit{ME}=\mathit{ME}(G)=\frac{2}{\pi} \int^{+\infty}_{0}\frac{1}{x^{2}}\ln \biggl[ \sum _{t\geq0}m(G,t) x^{2t} \biggr]\,dx. $$
(2)

Energy and matching energy of graphs are closely related, and they are two quantities of relevance for chemical applications; for details see [26–28].

We now give an equivalent definition of matching energy.

Definition 1.1

[25]

Let G be a graph of order n, and let \(\mu_{1},\mu_{2}, \ldots, \mu _{n}\) be the roots of its matching polynomial. Then

$$ \mathit{ME}(G)=\sum_{i=1}^{n}|\mu_{i}|. $$

The equation (2) induces a quasi-order relation over the set of all graphs on n vertices: if \(G_{1}\) and \(G_{2}\) are two graphs of order n, then

$$ G_{1}\preceq G_{2} \quad\Leftrightarrow\quad m(G_{1},t)\leq m(G_{2},t) \quad\mbox{for all } t=0, 1, \ldots, \biggl\lfloor \frac{n}{2}\biggr\rfloor . $$
(3)

If \(G_{1}\preceq G_{2}\) and there exists some i such that \(m(G_{1},i) < m(G_{2}, i )\), then we write \(G_{1}\prec G_{2}\). Clearly,

$$G_{1}\prec G_{2}\quad\Rightarrow\quad \mathit{ME}(G_{1})< \mathit{ME}(G_{2}). $$

Recall that the Hosoya index of a graph G is defined as \(Z(G)=\sum_{t\geq0} m(G,t)\) [29]. So we also have

$$G_{1}\prec G_{2}\quad\Rightarrow\quad Z(G_{1})< Z(G_{2}). $$

In [3, 4], the authors gave two fundamental identities for the number of t-matchings of a graph.

Lemma 1.2

Let G be a graph, \(e=uv\) an edge of G, and \(N(u)=\{v_{1}(=v),v_{2},\ldots,v_{j} \}\) the set of all neighbors of u in G. Then we have

$$ m(G,t)=m(G-uv,t)+m(G-u-v,t-1) $$
(4)

and

$$ m(G,t)=m(G-u,t)+\sum_{i=1}^{j}m(G-u-v_{i},t-1). $$
(5)

From Lemma 1.2, it is easy to get the following result.

Lemma 1.3

[25]

Let G be a graph and e one of its edges. Let \(G-e\) be the subgraph obtained from G by deleting the edge e. Then \(G-e\prec G\) and \(\mathit{ME}(G-e)<\mathit{ME}(G)\).

By Lemma 1.3, among all graphs on n vertices, the empty graph \(E_{n}\) and the complete graph \(K_{n}\) have the minimum and the maximum matching energy [25], respectively. It follows from equations (1) and (2) that \(\mathit{ME}(T)=E(T)\) for any tree T [25]. By using the quasi-order relation, some results have been obtained on extremal graphs with respect to matching energy among some classes of connected graphs with n vertices. For example, the extremal graphs in connected unicyclic, bicyclic graphs were determined in [25] and [30, 31], respectively; the maximal matching energy graphs of tricyclic graphs were obtained in [32], the minimal graphs among connected k-cyclic (\(k\leq n-3\)) graphs and bipartite graphs were characterized in [33]; the maximal connected graph with given connectivity (resp. chromatic number) was determined in [34]. For more as regards the latest results on matching energy, we refer the reader to [30, 35–41].

We now introduce some notations which will be used in the next section.

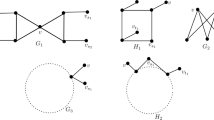
Let \(\mathcal{G}_{n,k}\) be the set of connected graphs of order n (≥2) with edge connectivity k (\(1\leq k\leq n-1\)). Let \(K_{n-1,1}^{k}\) be the graph obtained from \(K_{1}\cup K_{n-1}\) by adding k edges between \(V(K_{1})\) and \(V(K_{n-1})\); see Figure 1. In this paper, we show that \(K_{n-1,1}^{k}\) is the unique graph with the maximum matching energy (resp. Hosoya index) in \(\mathcal{G}_{n,k}\).

Figure 1
figure 1

Left is \(\pmb{K_{n-m,m}^{k}}\) ( \(\pmb{k\le m\le\lfloor\frac{n}{2}\rfloor}\) ) and right is \(\pmb{K_{n-1,1}^{k}}\) .

Full size image

2 Main results

First we recall some notations. We denote by \(\kappa'(G)\) and \(\delta(G)\) the edge connectivity and the minimum degree of a graph G, respectively. Let S be a nonempty proper subset of V. We use \(G[S]\) to denote the subgraph of G induced by S. The edge cut of G, denoted by \(\partial(S)\), is a subset of \(E(G)\) of the form \([S,\bar{S}]\), where \(\bar{S}=V\backslash S\). An edge cut \(\partial(v)\) (\(v\in V\)) is called a trivial edge cut. A k-edge cut is an edge cut of k elements. Let \(G\in\mathcal{G}_{n,k}\). Then G must have a k-edge cut \(\partial(S)\) with \(1\leq|S| \leq\lfloor \frac{n}{2}\rfloor\).

Lemma 2.1

Let \(G\ncong K_{n-1,1}^{k}\) be a graph in \(\mathcal{G}_{n,k}\) with a trivial k-edge cut. Then \(G\prec K_{n-1,1}^{k}\).

Proof

Let \(\partial(S)\) be a trivial k-edge cut of G with \(|S|=1\). Since \(G\ncong K_{n-1,1}^{k}\), \(G[\bar{S}]\) is a proper subgraph of \(K_{n-1}\). Hence G is a proper subgraph of \(K_{n-1,1}^{k}\), and so the result follows from Lemma 1.3. □

Lemma 2.2

Let \(G \in\mathcal{G}_{n,k}\) be a graph without trivial k-edge cuts. Then for any k-edge cut \(\partial(S)\) of G with \(2\leq|S| \leq\lfloor\frac{n}{2}\rfloor\), we have \(|S|\geq k\).

Proof

For \(k\leq2\), the assertion is trivial, so suppose \(k\geq3\). Assume, to the contrary, that G has a k-edge cut \(\partial(S)\) with \(2\leq|S|\leq k-1\). By the facts that \(\delta(G)\geq\kappa'(G)=k\) and G has no trivial k-edge cuts, we have \(\delta(G)\geq k+1\), and thus \(\sum_{v\in S}d_{G}(v)\geq|S|(k+1)\). On the other hand, \(\sum_{v\in S}d_{G}(v)=2e(G[S])+k\leq|S|(|S|-1)+k\). Therefore, we have \(|S|(k+1) \leq|S|(|S|-1)+k\), that is, \((|S|-1)(k-|S|)+|S|\leq0\), which is a contradiction. Therefore the result holds. □

For \(k\leq m\leq\lfloor\frac{n}{2}\rfloor\), let \(K_{n-m,m}^{k}\) be a graph obtained from \(K_{n-m}\cup K_{m}\) by adding k independent edges between \(V(K_{n-m})\) and \(V(K_{m})\), as shown in Figure 1. It is easy to see that \(\kappa'(K_{n-m,m}^{k})=k\) and \(\kappa'(K_{n-1,1}^{k})=k\).

We next show that for a graph \(G \in\mathcal{G}_{n,k}\) without trivial k-edge cuts, \(G\preceq K_{n-m,m}^{k}\) for some m. Before this, we introduce a new graph operation as follows.

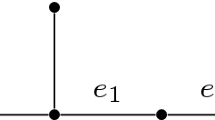
Let \(G_{1}\) be a graph in \(\mathcal{G}_{n,k}\) such that \(G_{1}\) has a k-edge cut \(\partial(S)\) with \(G[S]=K_{m}\), \(G[\bar{S}]=K_{n-m}\), and \(k\leq m\leq\lfloor\frac{n}{2}\rfloor\). Suppose that \(u_{1}, u_{2}\in\bar{S}\), \(v_{1}, v_{2}\in S\), \(e_{1}=u_{1}v_{1}\), \(e_{2}=u_{1}v_{2}\) are two edges of \(\partial(S)\), and \(u_{2}\) is not incident with any edge in \(\partial(S)\). If \(G_{2}\) is obtained from \(G_{1}\) by deleting the edge \(e_{2}\) and adding a new edge \(e'_{2}=u_{2}v_{2}\), we say that \(G_{2}\) is obtained from \(G_{1}\) by Operation I, as shown in Figure 2. Clearly, \(G_{2} \in\mathcal{G}_{n,k}\).

Figure 2
figure 2

The graphs \(\pmb{G_{1}}\) and \(\pmb{G_{2}}\) of \(\pmb{\mathcal{G}_{n,k}}\) in Operation I.

Full size image

Lemma 2.3

If \(G_{2}\) is obtained from \(G_{1}\) by Operation I, then \(G_{1}\prec G_{2}\).

Proof

By equation (4), we have

$$ m(G_{1},t)=m(G_{1}-e_{2},t)+m(G_{1}-u_{1}-v_{2},t-1) $$

and

$$ m(G_{2},t)=m\bigl(G_{2}-e'_{2},t \bigr)+m(G_{2}-u_{2}-v_{2},t-1). $$

Note that \(G_{1}-e_{2}\cong G_{2}-e'_{2}\), and \(G_{1}-u_{1}-v_{2}\) is isomorphic to a proper subgraph of \(G_{2}-u_{2}-v_{2}\). So, \(m(G_{1}-u_{1}-v_{2},t-1)\leq m(G_{2}-u_{2}-v_{2},t-1)\) for all t and \(m(G_{1}-u_{1}-v_{2},1)< m(G_{2}-u_{2}-v_{2},1)\). The result thus follows. □

Lemma 2.4

Let \(G \in\mathcal{G}_{n,k}\) be a graph without trivial k-edge cuts. Then \(G\preceq K_{n-m,m}^{k}\) for some m with \(\max\{k, 2\} \leq m\leq \lfloor\frac{n}{2}\rfloor\).

Proof

Let \(\partial(S)\) be a k-edge cut of G with \(2\leq|S| \leq\lfloor\frac{n}{2}\rfloor\). Let \(|S|=m\). Then \(m\geq k\) by Lemma 2.2. Let \(G_{1}\) be the graph obtained from G, by adding edges if necessary, such that \(G[S]\) and \(G[\bar{S}]\) are complete graphs. Therefore \(G\preceq G_{1}\) by Lemma 1.3. If \(G_{1} \ncong K_{n-m,m}^{k}\), then by using Operation I repeatedly, we can finally get \(K_{n-m,m}^{k}\) from \(G_{1}\). Hence \(G_{1} \preceq K_{n-m,m}^{k}\) by Lemma 2.3. The proof is thus complete. □

In the following, we show that \(K_{n-m,m}^{k}\prec K_{n-1,1}^{k}\) for \(m\geq2\).

Lemma 2.5

Suppose \(\max\{k, 2\} \leq m\leq\lfloor\frac{n}{2}\rfloor\). Then \(e(K^{k}_{n-m,m})< e(K^{k}_{n-1,1})\).

Proof

Note that

$$ e\bigl(K^{k}_{n-m,m}\bigr)= \frac{m(m-1)}{2}+\frac{(n-m)(n-m-1)}{2}+k $$

and

$$ e\bigl(K^{k}_{n-1,1}\bigr)=\frac{(n-1)(n-2)}{2}+k. $$

Hence we have

$$\begin{aligned} e\bigl(K^{k}_{n-1,1}\bigr)-e \bigl(K^{k}_{n-m,m}\bigr) =&\frac{n^{2}-3n+2}{2}-\frac{n^{2}+2m^{2}-2mn-n}{2} \\ =&(m-1) (n-m-1)>0. \end{aligned}$$

The proof is thus complete. □

Lemma 2.6

Let \(m \geq1\) be a positive integer. Then we have

$$ m\bigl(K^{1}_{m,m},t\bigr)\leq m \bigl(K^{1}_{2m-1,1},t\bigr) \quad\textit{for all } t=0, 1, \ldots, m $$
(6)

and

$$ m\bigl(K^{1}_{m+1,m},t\bigr)\leq m \bigl(K^{1}_{2m,1},t\bigr) \quad\textit{for all } t=0, 1, \ldots, m. $$
(7)

Proof

We apply induction on m. For \(m=1\) and \(m=2\), the assertions are trivial since \(K^{1}_{2,2}\) and \(K^{1}_{3,2}\) are proper subgraphs of \(K^{1}_{3,1}\) and \(K^{1}_{4,1}\), respectively. So suppose that \(m\geq3\) and inequalities (6) and (7) hold for smaller values of m. By Lemma 1.2, we obtain

$$\begin{aligned} m\bigl(K^{1}_{m,m},t\bigr) =&m \bigl(K^{1}_{m,m-1},t\bigr)+(m-2)m\bigl(K^{1}_{m,m-2},t-1 \bigr)+m(K_{m}\cup K_{m-2},t-1) \\ =&m\bigl(K^{1}_{m,m-1},t\bigr)+(m-1)m\bigl(K^{1}_{m,m-2},t-1 \bigr)-m(K_{m-1}\cup K_{m-3},t-2) \\ =&m\bigl(K^{1}_{m,m-1},t\bigr)-m(K_{m-1}\cup K_{m-3},t-2)+(m-1)\bigl[m\bigl(K^{1}_{m-1,m-2},t-1\bigr) \\ &{} +(m-1)m\bigl(K^{1}_{m-2,m-2},t-2\bigr)-m(K_{m-3}\cup K_{m-3},t-3)\bigr] \\ \leq& m\bigl(K^{1}_{m,m-1},t\bigr)+(m-1)m\bigl(K^{1}_{m-1,m-2},t-1 \bigr)+(m-1)^{2}m\bigl(K^{1}_{m-2,m-2},t-2\bigr) \\ &{} -m(K_{m-1}\cup K_{m-3},t-2) \end{aligned}$$

and

$$\begin{aligned} m\bigl(K^{1}_{2m-1,1},t\bigr) =&m \bigl(K^{1}_{2m-2,1},t\bigr)+(2m-3)m\bigl(K^{1}_{2m-3,1},t-1 \bigr)+m(K_{2m-3},t-1) \\ =&m\bigl(K^{1}_{2m-2,1},t\bigr)+m(K_{2m-3},t-1)+(2m-3) \bigl[m\bigl(K^{1}_{2m-4,1},t-1\bigr) \\ &{} +(2m-5)m\bigl(K^{1}_{2m-5,1},t-2\bigr)+m(K_{2m-5},t-2) \bigr] \\ \geq& m\bigl(K^{1}_{2m-2,1},t\bigr)+(2m-3)m\bigl(K^{1}_{2m-4,1},t-1 \bigr) \\ &{} +(2m-3) (2m-5)m\bigl(K^{1}_{2m-5,1},t-2\bigr). \end{aligned}$$

By the induction hypothesis, we obtain

$$\begin{aligned}& m\bigl(K^{1}_{m,m-1},t\bigr) \leq m \bigl(K^{1}_{2m-2,1},t\bigr), \\& m\bigl(K^{1}_{m-1,m-2},t-1\bigr) \leq m\bigl(K^{1}_{2m-4,1},t-1 \bigr), \\& m\bigl(K^{1}_{m-2,m-2},t-2\bigr) \leq m\bigl(K^{1}_{2m-5,1},t-2 \bigr). \end{aligned}$$

Since \(m\geq3\), we have \(m-1\leq2m-3\) and \((m-1)^{2}\leq(2m-3)(2m-5)\) when \(m\geq4\). Notice that for \(m=3\), \(K^{1}_{m-2,m-2}=K_{m-1}\cup K_{m-3}\), and \((m-1)^{2}-1=(2m-3)(2m-5)\). Hence inequality (6) holds.

By Lemma 1.2, we get

$$\begin{aligned} m\bigl(K^{1}_{m+1,m},t\bigr) =&m \bigl(K^{1}_{m,m},t\bigr)+(m-1)m\bigl(K^{1}_{m-1,m},t-1 \bigr)+m(K_{m-1}\cup K_{m},t-1) \\ \leq& m\bigl(K^{1}_{m,m},t\bigr)+m \cdot m \bigl(K^{1}_{m-1,m},t-1\bigr) \\ =&m\bigl(K^{1}_{m,m},t\bigr)+m \cdot\bigl[m \bigl(K^{1}_{m-1,m-1},t-1\bigr) \\ &{} +(m-2)m\bigl(K^{1}_{m-1,m-2},t-2\bigr)+m(K_{m-1}\cup K_{m-2},t-2)\bigr] \\ \leq& m\bigl(K^{1}_{m,m},t\bigr)+m\cdot\bigl[m \bigl(K^{1}_{m-1,m-1},t-1\bigr) \\ &{} +(m-1)m\bigl(K^{1}_{m-1,m-2},t-2\bigr)\bigr] \\ =&m\bigl(K^{1}_{m,m},t\bigr)+m \cdot m\bigl(K^{1}_{m-1,m-1},t-1 \bigr) +m(m-1)m\bigl(K^{1}_{m-1,m-2},t-2\bigr) \end{aligned}$$

and

$$\begin{aligned} m\bigl(K^{1}_{2m,1},t\bigr) =&m \bigl(K^{1}_{2m-1,1},t\bigr)+(2m-2)m\bigl(K^{1}_{2m-2,1},t-1 \bigr)+m(K_{2m-2},t-1) \\ =&m\bigl(K^{1}_{2m-1,1},t\bigr)+m(K_{2m-2},t-1)+(2m-2) \bigl[m\bigl(K^{1}_{2m-3,1},t-1\bigr) \\ &{} +(2m-4)m\bigl(K^{1}_{2m-4,1},t-2\bigr)+m(K_{2m-4},t-2) \bigr] \\ \geq& m\bigl(K^{1}_{2m-1,1},t\bigr)+(2m-2)m\bigl(K^{1}_{2m-3,1},t-1 \bigr) \\ &{} +(2m-2) (2m-4)m\bigl(K^{1}_{2m-4,1},t-2\bigr). \end{aligned}$$

By the induction hypothesis and inequality (6), we have

$$\begin{aligned}& m\bigl(K^{1}_{m,m},t\bigr) \leq m \bigl(K^{1}_{2m-1,1},t\bigr), \\& m\bigl(K^{1}_{m-1,m-1},t-1\bigr) \leq m\bigl(K^{1}_{2m-3,1},t-1 \bigr), \\& m\bigl(K^{1}_{m-1,m-2},t-2\bigr) \leq m\bigl(K^{1}_{2m-4,1},t-2 \bigr). \end{aligned}$$

Notice that \(m\leq2m-2\) and \(m(m-1)\leq(2m-2)(2m-4)\). Therefore inequality (7) holds.

The proof is thus complete. □

Lemma 2.7

Suppose \(2\leq m\leq\lfloor\frac{n}{2}\rfloor\). Then

$$m\bigl(K^{1}_{n-m,m},t\bigr)\leq m\bigl(K^{1}_{n-1,1},t \bigr) \quad\textit{for all } t=0, 1, \ldots, \biggl\lfloor \frac{n}{2}\biggr\rfloor . $$

Proof

We apply induction on n. As the two cases \(n=2m\) and \(n=2m+1\) were proved by Lemma 2.6, we proceed to the induction step. By Lemma 1.2 and the induction hypothesis, we have

$$\begin{aligned} m\bigl(K^{1}_{n-m,m},t \bigr) =&m\bigl(K^{1}_{n-m,m-1},t\bigr)+(m-2)m\bigl(K^{1}_{n-m,m-2},t-1 \bigr)+m(K_{n-m}\cup K_{m-2},t-1) \\ \leq& m\bigl(K^{1}_{n-m,m-1},t\bigr)+(m-1)m\bigl(K^{1}_{n-m,m-2},t-1 \bigr) \\ \leq& m\bigl(K^{1}_{n-2,1},t\bigr)+(m-1)m\bigl(K^{1}_{n-3,1},t-1 \bigr) \\ =&m(K_{n-2},t)+m(K_{n-3},t-1) \\ & {}+(m-1) \bigl(m(K_{n-3},t-1)+m(K_{n-4},t-2)\bigr) \\ =&m(K_{n-2},t)+m\cdot m(K_{n-3},t-1)+(m-1)m(K_{n-4},t-2) \end{aligned}$$

and

$$\begin{aligned} m\bigl(K^{1}_{n-1,1},t \bigr) =&m(K_{n-1},t)+m(K_{n-2},t-1) \\ =&m(K_{n-2},t)+(n-2)m(K_{n-3},t-1) \\ &{} +m(K_{n-3},t-1)+(n-3)m(K_{n-4},t-2) \\ =&m(K_{n-2},t)+(n-1)m(K_{n-3},t-1)+(n-3)m(K_{n-4},t-2). \end{aligned}$$

Thus the result follows by the fact that \(m\leq n-2\). □

Lemma 2.8

Suppose \(k\leq m\leq\lfloor\frac{n}{2}\rfloor\). Then

$$m\bigl(K^{k}_{n-m,m},t\bigr)\leq m\bigl(K^{k}_{n-1,1},t \bigr) \quad\textit{for all } t=0, 1, \ldots, \biggl\lfloor \frac{n}{2}\biggr\rfloor . $$

Proof

We apply induction on k. As the case \(k=1\) was proved by Lemma 2.7, we suppose that \(k\geq2\) and the assertion holds for smaller values of k. By equation (4), we have

$$ m\bigl(K^{k}_{n-m,m},t\bigr) = m\bigl(K^{k-1}_{n-m,m},t \bigr)+ m\bigl(K^{k-1}_{n-m-1,m-1},t-1\bigr) $$

and

$$ m\bigl(K^{k}_{n-1,1},t\bigr) = m\bigl(K^{k-1}_{n-1,1},t \bigr)+ m(K_{n-2},t-1). $$

By the induction hypothesis and Lemma 1.3, we obtain \(m(K^{k-1}_{n-m,m},t)\leq m(K^{k-1}_{n-1,1},t)\) and \(m(K^{k-1}_{n-m-1,m-1},t-1) \leq m(K_{n-2},t-1)\). Thus the result follows. □

Combining with Lemmas 2.5 and 2.8, we obtain the following result directly.

Corollary 2.9

Suppose \(\max\{k, 2\} \leq m\leq\lfloor\frac{n}{2}\rfloor\). Then \(K^{k}_{n-m,m}\prec K^{k}_{n-1,1}\).

Theorem 2.10

Let G be a graph in \(\mathcal{G}_{n,k}\). Then \(\mathit{ME}(G)\leq \mathit{ME}(K^{k}_{n-1,1})\). The equality holds if and only if \(G\cong K^{k}_{n-1,1}\).

Proof

Notice that \(K^{k}_{n-1,1} \in\mathcal{G}_{n,k}\). Let \(G\ncong K^{k}_{n-1,1}\) be a graph in \(\mathcal{G}_{n,k}\). It suffices to show that \(G\prec K^{k}_{n-1,1}\). If G has a trivial k-edge cut, then we have \(G\prec K_{n-1,1}^{k}\) by Lemma 2.1. Otherwise, by Lemma 2.4 and Corollary 2.9, we obtain \(G\prec K_{n-1,1}^{k}\) again. The proof is thus complete. □

By the proof of Theorem 2.10 and the definition of the Hosoya index, we can get the following result on the Hosoya index.

Theorem 2.11

Let G be a graph in \(\mathcal{G}_{n,k}\). Then \(Z(G)\leq Z(K^{k}_{n-1,1})\). The equality holds if and only if \(G\cong K^{k}_{n-1,1}\).

3 Conclusion

Chen et al. [30] characterized the extremal graphs of the matching energy in unicyclic, bicyclic graphs with a given diameter, respectively. Li et al. [37] obtained the unique graph having extremal matching energy in unicyclic graphs with fixed girth and the graphs with given clique number. Xu et al. [41] got the extremal graph of the matching energy among all t-apex trees. In [38], the authors obtained the extremal graph with matching energy in the complement of graphs. So it is interesting to characterize a graph having an extremal value of the matching energy with some graph invariants. In the paper, we got the maximal graph of the matching energy in graphs with given edge connectivity.

Therefore, our next work is to continue the study of graphs having extremal values of the matching energy with some graph invariants.