Thermoelectric power of metallic Rb₃C₆₀: phonon-drag and carrier diffusion contributions

Abstract

Thermoelectric power (S) of Rb₃C₆₀ fullerides in the metallic phase is theoretically estimated. We first develop a Hamiltonian model that incorporates the scattering rates within the relaxation time approximation to estimate the phonon-drag thermoelectric power (S_ph^drag) incorporating the scattering of phonons with defects, electrons as carriers, grain boundaries, and phonon-phonon interactions. As a next step, Mott expression within parabolic band approximation is used to analyze the electron diffusive thermoelectric power (S_c^diff) using Fermi energy as electron parameter, and S_c^diff shows a linear temperature dependence. The S_ph^drag is nonzero in both normal and superconducting states. Its behavior is determined by competition among the several operating scattering rates for heat carriers and a balance between diffusive carrier and phonon-drag contributions. Acoustic phonons are effectively scattered by various scatterers for the thermoelectric power. S infers a change in slope above transition temperature and becomes almost linear above 70 K.

Background

Since the revolution of superconductivity in organic materials, initiated by Hebard and researchers [1], the unusual normal state transport properties of alkali-metal-intercalated fullerides have been suspected of giving clues to the basic mechanism responsible for superconductivity [2, 3]. The parent C₆₀ is a typical insulator, and by doping with alkali-metal atoms, M₃C₆₀ (which opt for a face-centered cubic structure) superconductivity in intercalated compounds will appear in limited conditions. Prominent among these materials are the M₃C₆₀ fullerides with M standing for K, Rb, or Cs with a superconducting transition temperature T_c of approximately 40 K (Rb₃C₆₀) at ambient pressure. Alkali-metal-intercalated C₆₀ continues to generate excitement due to the conducting polymeric phase in MC₆₀[4]. It is identified that in alkali-metal-doped fullerenes, the phonon spectrum has a wide frequency region. The vibrational spectrum is classified into two regions. One of them belongs to the rotation of C₆₀ molecule and the intermolecular vibrations. The others belong to the intramolecular vibrations. Actually, the intramolecular phonons span a large frequency range, from 2 × 10² to 2 × 10³ cm⁻¹, while intermolecular vibrations and soccer ball vibrations (restricted rotations) go down to a low energy interval, 10 to 100 cm⁻¹[5]. The electronic structure of alkali-metal-doped fullerenes in the normal state is important in understanding the mechanism of superconductivity. Switching to electronic structure, experimental techniques as NMR measurements [6], photoemission measurements [7], and infrared reflectivity measurements [8] report the bandwidth ranging from 0.2 to 1.2 eV.

It is identified that in alkali-metal-doped fullerenes, the phonon spectrum has a wide frequency region. The vibrational spectrum is classified into two regions. One of them belongs to the rotation of C₆₀ molecule and the intermolecular vibrations. The others are the intramolecular vibrations. Actually, the intramolecular phonons span a large frequency range, 2 × 10² – 2 × 10³ cm^-1, while intermolecular vibrations and soccer ball vibrations (restricted rotations) go down to a low energy interval, 10 – 100 cm^-1[5]. The electronic structure of alkali-metal-doped fullerenes in the normal state is important for understanding the mechanism of superconductivity. Switching to electronic structure, experimental techniques as NMR measurements [6], photoemission measurements [7] and infrared reflectivity measurements [8] reports the bandwidth ranging from 0.2 to 1.2 eV.

Doping of alkali metal in the parent C₆₀ essentially expands the lattice and causes an increase in the lattice constant; the result is the enhanced density of states at the Fermi level N(ε _F ). An intermediate consequence is a fairly large electron-phonon interaction (λ ≡ N(ε _F )V)) depending upon whether inter- or intramolecular phonon is the mediating boson. The thermoelectric power S(T) is a powerful probe not only to understand the electronic structure, but also to shed light on the electron-phonon interaction. The mass enhancement effects can increase the thermoelectric power of a metal at low temperatures [9]. The mass enhancement is proportional to (1 + λ), and this correction is normally masked by large phonon-drag effect.

In contrast to conventional superconductors, the physical properties of organic superconductors directly reflect the electronic structure of the molecules they consist. Inabe et al. have first reported the thermoelectric power of single crystal K₃C₆₀ and Rb₃C₆₀ superconductors [10]. Nonlinear temperature dependences of S(T) with negative sign are documented above T_c for both K- and Rb-doped fullerides. The room-temperature value of S(T) is about −11 μV/K, indicating the metallic nature in the normal state with electron as sole carriers. The absolute value of S decreases linearly until it drops discontinuously to zero at T _c and at a broad hump around 70 K. The analysis of density of states in K₃C₆₀ reveals that low-frequency phonon modes are significant in normal state transport, and the nonlinear behavior in low-temperature regime (T < 70 K) is ascribed to phonon drag.

The measured thermoelectric power in K₄C₇₀ thin films also shows a negative S, indicating that charge carriers are electrons and that roughly linear temperature dependence is below 100 K. For higher temperatures T > 100 K, S(T) decreases slowly with a deviation from linearity [11]. Since the conduction band in K₄C₇₀ is half-filled, similar to that of K₃C₆₀, an estimate of bandwidth is about 0.5 to 0.6 eV, consistent with a value of approximately 0.6 eV in K₃C₆₀. Henceforth, the normal state of K₃C₆₀ is a metallic state with a bandwidth of approximately 0.6 eV; the electron-phonon interaction and electron-electron interaction are important for charge transport.

Later on, Suighara et al. have discussed the possibility of phonon-drag thermopower in doped fullerides [12]. It is concluded that a small but clear deviation from the linear relation at low temperatures is ascribed to the phonon-drag effect. The broad dip over 100 K and its small magnitude suggest that the anomaly is not related to the electron-intramolecular phonon interaction but to the electron-intermolecular phonon interaction. A visual comparison of data indicates that the temperature dependence of the thermopower of superconducting fullerenes is very similar to that of systems in which mass enhancement effect has been observable [13, 14].

Motivated by the available experimental data [10–12] and carrier diffusion term with mass enhancement [13], for thermoelectric power, we plan to seek the role of scattering of phonon with defects, grain boundaries, phonon and electrons for phonon-drag thermoelectric power, as well as the carrier diffusion contribution. This improves our understanding of the interplay of scattering processes between heat carriers themselves and between carriers and impurities for the explanation of the reported behavior of thermoelectric power S(T). Also, it is important to look for the relative magnitudes of these scattering processes, which lead to the anomalous behavior, and this is another motivation for the present investigation. The results we report here indeed shed some very important light on the nature of phonon and electron as carrier channel of thermoelectric power in Rb₃C₆₀.

We first describe the method of calculation, give further details of the results, and speculate on the reliability of our approach to the problem of S(T) in alkali-metal-doped fullerides. In ‘The model’ section, we give details about the scattering rates within the relaxation time approximation to estimate the phonon-drag thermoelectric power (S_ph^drag) incorporating the scattering of phonons with defects, electrons as carriers, grain boundaries, and phonon-phonon interactions. While obtaining numerical results, we place particular emphasis on the acoustic phonons as a source of S_ph^drag in the Hamiltonian model. Also, the role of carrier diffusion contribution up to room temperature and zero magnetic field for S(T) is investigated. We find that the S(T) behavior can be well accounted for through the use of the Debye model. In the ‘Results and discussion’ section, we give the numerical estimation of various scattering rates for the calculation of diffusion and phonon-drag processes and discuss the results obtained. In the ‘Conclusions’ section, we provide physical descriptions of the numerical results calculated herein.

The model

Let us begin with the estimation of carrier diffusion thermoelectric power. We use the well-known Mott formula within parabolic band approximation to estimate the contribution of electrons towards thermoelectric power. The low-temperature carrier diffusion thermoelectric power [10] is as follows

$$S_{\text{c}}^{\text{diff}.}\left(T\right)=-\frac{{\pi }^2{k}_B^{2}T}{3\left|e\right|}{\left[\frac{\partial \text{ln}\sigma \left(\epsilon \right)}{\partial \epsilon }\right]}_{\epsilon ={\epsilon }_F}\text{.}$$

(1)

with σ (ω) = ne²τ(ε)/m as the energy dependence of electrical conductivity in the relaxation time approximation. Here, n(m) is the density (mass) of carriers, and τ is the relaxation time. Moreover, this expression is true within parabolic band approximation, but not with relaxation time approximation alone. For the sake of simplicity, it is sufficient to neglect the energy dependence in τ (ε), taking τ (ε) = τ (ε _F ); for three-dimensional model, Equation 1 becomes the following

$$S_{\text{c}}^{\text{diff}.}\left(T\right)=-\frac{{\pi }^2{k}_B^{2}T}{6\left|e\right|{\epsilon }_F}\text{.}$$

(2)

Keeping in mind that τ = ℓ/v _F , the method point to the scattering of carriers by impurities is dominant for constant relaxation times. Such a procedure has found success in explaining previous measurements on high-temperature superconductors on a wide temperature range [15, 16].

As a next step, in order to assess the role of phonons for thermoelectric power, we develop a Hamiltonian model where the low-energy intermolecular vibrations (phonons) in Rb₃C₆₀ are described in the Debye model, and the electrons as charge carriers are treated in an isotropic Bardeen-Cooper-Schrieffer (BCS)-like model. The charge carriers are quasiparticles in a periodic crystal and hence have a well-defined dispersion relation ε _k . The alkali-metal-doped fullerides are impure crystals, where the excitations may not be quasiparticles at all, but for which the physical properties are qualitatively same.

The phonon inelastic scattering events are assumed to be independent, and scattering of phonon with various scattering sources is additive. The Debye model with acoustic phonon is valid for Rb₃C₆₀ as the temperature domain of interest lies well below the effective Debye temperature θ _D ≈ 185 K [17]. We shall use the isotropic BCS-like model with intermolecular acoustic phonon to derive qualitative results for thermoelectric power.

The Hamiltonian model is as follows [18]:

$$\begin{array}{l}H={\displaystyle \sum _p{\epsilon }_p{a}_p^{+}{a}_p+{\displaystyle \sum _q{\omega }_q{b}_q^{+}{b}_q}}+{\displaystyle \sum _{p_1,p_2}\varphi }(p_1,p_2)a_{p_1}^{+}{a}_{p_2}\\ \begin{array}{}\end{array}+D_p{\displaystyle \sum _{p,q}q}{\left[\frac{\hslash }{2\rho {\omega }_q}\right]}^{1/2}{a}_{p+q}^{+}{a}_p(b_p+b_{-q}^{+})\\ \begin{array}{}\end{array}+\frac{R}{2n}{\displaystyle \sum _{q_1,q_2}{e}^{i\left(q_1+q_2\right)R_i}}{\left[\frac{\hslash {\omega }_{q_1}\hslash {\omega }_{q_2}}{4}\right]}^{1/2}(b_{q_1}-b_{-q_1}^{+})\\ \times (b_{q_2}-b_{-q_2}^{+})+H_{p-p}\text{.}\end{array}$$

(3)

The initial two terms are carrier (electron) and phonon excitations; the third and fourth terms represent carrier-impurity and carrier-phonon interactions, respectively. The fifth term is phonon-impurity interaction, and the last term stands for the phonon-phonon interaction. The symbols that appeared in Equation 3 are as follows: ε _p is the carrier free energy, a (a⁺) and b (b⁺) are the creation (annihilation) operators for phonon and electron, φ is coupling parameter of electron and impurity potential, D _p is deformation-potential constant, ρ is ionic mass density, ω _q is acoustic phonon frequency of a wave vector q, R is relative ionic-mass difference ((M″ − M) / M″), M (M″) symbolizes for C₆₀ (Rb), n is number of cells, and R _i stands for the position of defects due to substitutions.

To estimate the phonon-drag thermoelectric power, we shall use the Kubo formula following the Hamiltonian model [19]. It has contributions from both the phonons and the carriers. In the continuum approximation, the lattice part is as follows [20]:

$$S_{\text{ph}}^{\text{drag}}\left(T\right)=-\frac{k_B}{\left|e\right|}{\left[\frac{T}{{\theta }_D}\right]}^3{\displaystyle {\int }_0^{{\omega }_D}d\omega }{\left(\beta \omega \right)}^{4}A\left(\omega \right){\left(\beta \omega \right)}^4\frac{e^{\mathit{\beta \omega }}}{{\left(e^{\mathit{\beta \omega }}-1\right)}^2}\text{.}$$

(4)

The Boltzman constant is k _B ; e is the charge of carriers, ω _D is the Debye frequency, and β = ћ/k _B T.

The relaxation time is inhibited in A(ω) and is proportional to the imaginary part of the self-energy Σ. The relaxation time ratio can be calculated to the lowest order of the various interactions in the weak interaction case. The phonon-drag thermoelectric power relaxation time ratio A (ω) is as follows:

$$\begin{array}{l}A\left(\omega \right)={\left[1/{\tau }_{ph-d}+1/{\tau }_{ph-gb}+1/{\tau }_{ph-ph}\right]}^{-1}\\ \times \left[1/{\tau }_{ph-d}+1/{\tau }_{ph-gb}+1/{\tau }_{ph-ph}+1/{\tau }_{ph-c}\right]\text{,}\end{array}$$

(5)

The relaxation times are expressed as follows:

$${\tau }_{ph-d}^{-1}\left(\omega \right)=D_{\mathit{phd}}/k_B^3{\omega }^4{\hslash }^3\text{.}$$

(6)

The various relaxation times are defined in terms of transport coefficients as in the following:

$${\tau }_{ph-d}^{-1}\left(\omega \right)=D_{\mathit{phd}}/k_B^3{\omega }^4{\hslash }^3\text{,}$$

(7)

$${\tau }_{ph-gb}^{-1}\left(\omega \right)=D_{\mathit{phgb}}{v}_s/L\text{,}$$

(8)

$${\tau }_{ph-ph}^{-1}\left(\omega \right)=D_{\mathit{phph}}{\left(T\omega \hslash /k_B\right)}^3\text{,}$$

(9)

and

$${\tau }_{ph-c}^{-1}\left(\omega \right)=D_{\mathit{phe}}\omega n_F\left(\mathrm{\Delta }\right)\text{,}$$

(10)

where v _s is the velocity of sound, L is the crystal dimension, n _F is the Fermi-Dirac distribution function, and Δ is the energy gap parameter. The notations τ_ph-d, τ_ph-gb, τ_ph-ph, and τ_ph-c are the phonon scattering relaxation time due to substitutional defects, grain boundaries, phonon-phonon interaction, and phonon-carrier interaction, respectively. We note that to this order, Mathiessen's rule holds: the inverse of the total relaxation time is the sum of the various contributions for the different scattering channels.

The transport coefficients appearing in Equations 7 to 10 are defined as

$$D_{\text{phd}}=\left[\frac{3n_i{R}^2}{4{\theta }_D^3}\right]\text{,}$$

(11)

with n _i as the density of impurities or point defects, R is the relative ionic-mass difference, and θ _D the Debye temperature. The above essentially accounts for the density of real point defects in Rb₃C₆₀, such as impurities, vacancies, and interstitials, which should be fairly large in a weakly bound crystal such as orientationally ordered C₆₀ and increase in orientationally disordered Rb₃C₆₀. The potential exerted on electron as free carriers in the doped C₆₀ is due to the ionized cage of orientationally ordered C_60. The strength of the electron-phonon scattering in terms of deformation potential D _p , carrier mass m, ionic mass M, and the Fermi energy (ε _F ) is as follows:

$$D_{\text{phe}}=\frac{9\pi }{4}{\left[\frac{m}{3M}\right]}^{1/2}\frac{{D_p}^2}{{\epsilon }_F^2}\text{,}$$

(12)

Herein, the Thomas-Fermi approximation defines the electron-acoustic phonon coupling strength as D _p = −ε _F / 2.

The estimation and numerical computation of the carrier diffusion and phonon-drag contributions to the thermoelectric power by incorporating the effects of different scattering mechanisms are presented in the ‘Results and discussion’ section.

Results and discussion

We first estimate the carrier diffusion thermoelectric power (S_c^diff), which is given by the expressions (Equations 1 and 2). The Fermi energy ε _F in Rb₃C₆₀ is calculated as 0.23 eV, which is used for the computation of S_c^diff. The carrier-impurity contribution to the thermoelectric power S_c^diff is documented in Figure 1 as functions of temperature. It is evident from the plot that S_c^diff(T) increases linearly with increasing temperature. The numerically computed S_c^diff is subtracted from the experimental data [10] to obtain the difference (S_difference = S_experimental − S_c^diff). The difference S_difference is characterized as phonon-drag thermoelectric power and fitted within the relaxation time approximation.

Figure 1

Variation of carrier diffusion thermoelectric power with temperature compared with experimental data. Adapted from Inabe et al. [10] for Rb₃C₆₀ superconductors.

The thermoelectric power is a powerful probe to study the nature of carriers and scattering process between them in the phonon system with either the absence or presence of a magnetic field. For the actual calculation of the phonon-drag thermoelectric power in Rb₃C₆₀, more realistic values of some physical parameters derived from the experimental data follows. The Coulomb interactions among the adjacent ions in an ionic crystal such as Rb₃C₆₀ are expressed in terms of inverse-power overlap repulsion as in the following [21]:

$$\Phi \left(r\right)=-{\left(Ze\right)}^2\left[\frac{1}{r}-\frac{f}{r^s}\right]\text{,}$$

(13)

with f is being the repulsion force parameter between the ion cores.

The elastic force constant κ is conveniently derived from Φ(r) at the equilibrium interionic distance r ₀ as of the following:

$$\kappa ={\left(\frac{{\partial }^2\Phi }{\partial r^2}\right)}_{r_0}\text{,}$$

$$={\left(Ze\right)}^2\left[\frac{s-1}{r_0^3}\right]\text{,}$$

(14)

Here, s is the index number of the overlap repulsive potential. For Rb₃C₆₀, the acoustic mass M = (M (Rb) + M (C₆₀)) is 979.29 amu. The elastic force constant κ^* = 2κ for each directional oscillation mode to get the acoustic phonon frequency as follows:

$${\omega }_D=\sqrt{\frac{{\kappa }^{*}}{2M}}\text{,}$$

$$=\left(Ze\right)\sqrt{\frac{\left(s-1\right)}{2M}\frac{1}{r_0^3}}\text{.}$$

(15)

The acoustic phonon mode frequency is estimated following the ionic model. Here, we have used the value of effective ion charge Ze = 7e. The Debye temperature for Rb₃C₆₀ is obtained by taking s = 7 and the bond distance r₀ = 10.21 Å (a/√2) (a = 14.45 Å) [1], yielding κ = 6.36 × 10⁴ gm s⁻². For most ionic crystals, the index number of the repulsive potential has been reported to be s = 6 to 8 [21]. Such procedure yields the Debye temperature of about 95 K.

Deduced value of the Debye temperature is consistent with specific heat data [18], inelastic neutron scattering results [5], isotope effect studies [22], and thermoelectric power data [10, 12]. We do not claim the process to be rigorous, but a consistent agreement following spring ball lattice model with repulsive force between Rb cations and C₆₀ is obtained to evaluate acoustic phonon energy. We note that the present model has only one free parameter, i.e., the index number of the repulsive potential. Value of θ _D differs from technique to technique, and its value also vary from sample to sample with an average value and standard deviation of θ _D = θ _D ± 15 K.

In Rb₃C₆₀, the electron density n = (4 × 3/a³) is calculated from the lattice parameter a of 14.45 Å [1] to get n = 3.98 × 10²¹ cm⁻³. We have used the band structure value of mass as 3.6 m_e[9, 20]. The electron parameters such as Fermi velocity and Fermi wave vector are obtained as 1.55 × 10⁷ cm s⁻¹ and 0.486 Å⁻¹, respectively. The length of the sample is about 3 mm and v _s = 2.2 × 10⁵ cm s⁻¹. The above corresponds to the weak intermolecular interaction. Herein, we presume that Rb₃C₆₀ contains the defects, the phonons, and the BCS-like quasiparticles, interacting with one another as evident from the Hamiltonian model.

While estimating the temperature-dependent thermoelectric power of Rb₃C₆₀, we make use of the transport parameters which characterize the strengths of the phonon defects, phonon-grain boundary, phonon-phonon, and phonon-carrier scattering processes as D_phd = 3.1 × 10⁻⁹ K⁻³, D_phgb = 2.5 × 10⁻² K⁻⁶ s⁻¹, D_phph = 2.3 × 10⁻³ K⁻⁶ s⁻¹, and D_phe = 5.3 K⁻⁶ s⁻¹, respectively. These are material-dependent fitting parameters for phonon-drag thermoelectric power in the present model. It is instructive to mention that the electron-phonon interaction is limited to the coupling of intermolecular acoustic phonons. The phonon-drag term is not related to the intramolecular phonons because of an unusual high energy but is responsible for superconductivity in alkali-intercalated fullerides.

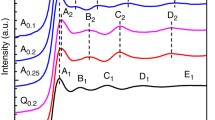
We first discuss the relative magnitudes of the various scattering mechanisms. A plot of various scattering phonon relaxation time as a function of ξ (ξ = ℏω / k _B T) in terms of frequency at T = 10, and 35 K from Equations 7 to 10 are shown in Figure 2. It can be seen that for low values of ξ, the phonon-electron scattering is higher, while for high values of ξ, it is the phonon-defect scattering that grows faster. However, at both small and large values of ξ, the phonon-phonon scattering process is weaker. As we go above T _c , i.e., with increase in temperature (see Figure 3 in the normal state at T = 50, 150, and 250 K), phonon-electron scattering improves and phonon-phonon scattering becomes weaker. In the superconducting state T < T _c , carriers are condensed in Cooper pair; the increase in temperature order parameter reduces the state, and concentration of free carriers increases the state. In particular, phonon-phonon umklapp scattering grows faster than phonon-electron scattering due to a large mobility of carriers in the normal state (T > T _c ). In this respect, for all temperatures above T _c , the phonon-impurity scattering saturates for higher ξ values.

Figure 2

Variation of phonon relaxation times as function of ξ for T = 10 and 35 K. For Rb₃C₆₀ superconductors.

Figure 3

Variation of phonon relaxation times as function of ξ for T = 50, 150, and 250 K. For Rb₃C₆₀ superconductors.

We now qualitatively discuss S_ph^drag in the presence of various scattering mechanisms (please see Figure 4). At low temperatures through T _c , the quasiparticle excitations condensed into the ground state, and they did not scatter the phonons. S_ph^drag, thus, increases exponentially with temperature in the vicinity of transition temperature, As various scatterings of phonons contribute to the reduction of thermal conductivity, it leads to the increase of thermoelectric power. The individual effect on thermoelectric power due to different scattering mechanisms is shown in Figure 4. The results show that the effect of scatterings on thermoelectric power is additive, and the final variation depends on the relative magnitude of different scattering processes available. It is worth to mention that S in the superconducting state is zero (T → 0 K) and that every nonzero value is an artifact due to the model.

Figure 4

Variation of phonon-drag thermoelectric power as function of temperature in presence of various phonon scattering mechanism. A comparison is being made with the data obtained from Figure 1 as S_experimental − S_c^diff for Rb₃C₆₀ superconductors.

To ascertain the physical significance of the density of impurities, we evaluate the transport coefficient D_phd that appeared in Equation 7. We estimate the product of density of impurities and square of relative ionic mass difference, n _i R² = 4.91 × 10⁻³, from the value of coefficient D_phd. Due to the fact that the transport parameter D_phd is determined by the magnitude of the phonon-impurity interaction, we are able to roughly estimate the density of impurity scatterers which may point to the fact that the quasiparticles in the metallic state are essentially localized. In this way, one can set a limit to the concentration of impurities if the impurities as scatterers are isotope in origin. Herein, we believe that the density of impurity is constant with respect to temperature. In passing, we refer to the work of Erwin and Pickett [23] who worked out the lowest-order variational solution for S (T). The energy dependence of scattering rate 1/τ is considered (1) the electron-phonon coupling strength λ(ε) = constant, leading to a free-hole-like thermoelectric power with positive slope and linear behavior and (2) λ(ε) = N(ε), resulting in an electron behavior up to ≈200 K and a slow transition to positive slope thereafter.

The S(T) behavior depends on the competition among the various scattering mechanisms for the heat carriers and balances between the electron and phonon competition. It is worth emphasizing that S(T) increases linearly above 70 K and is well reproduced from the present theoretical model; this phenomenon is attributed to the shortened phonon mean free path as compared to that at low temperatures. It may be seen that the slope change in S_ph^drag is much more pronounced than that in S_c^diff below 150 K. The reason for this change is due to the fact that the phonon-impurity scattering dominates and electron-impurity scattering is weaker. Below T _c , the opening of the superconducting gap rapidly reduces the phonon-electron scattering rate, and the competition in between phonon-impurity and phonon-electron scattering mechanism leads to faster change of slope in S_ph^drag.

The reason is further related to the fact that the mean free path of both electrons and phonons changes below T _c because the scattering of phonons on electrons is reduced when electrons condense in the superconducting state. Deduced results on temperature dependence of thermoelectric power of Rb₃C₆₀ from the present model are consistent qualitatively with the experimental data [10, 12]. Apart from the qualitative agreement at low temperature, the diffusive thermoelectric power improves on phonon-drag thermoelectric power at room temperature. The reason for this is the shorter mean free path of carriers in the normal state. However, below T _c , where the concentration of charge carrier is decreased by the formation of a Cooper pair and the phonon mean free path becomes longer, S_c^diff continues to play an important role even near room temperature.

It is customary to mention that a change in slope of S_ph^drag is obtained at about T ≈ θ _D , as in the normal metals [9]. Indeed, below T ≈ θ _D , the temperature dependence of the phonon-drag contribution is attributed to be a competitive process in which S(T) decreases on lowering temperature and slightly improves on increasing temperature which is attributed to phonon-phonon scattering. To an extent, a comparison of the computed data, a consistent fit with the reported data, was obtained by choosing a reasonable set of parameters. It is instructive to compare the observation with that of conventional superconductors where the thermally excited quasiparticles of the electron system are dominant carriers of heat; a similar situation is revealed in Rb₃C₆₀.

Conclusions

The thermoelectric power S(T) behavior is an instructive probe to reveal the lattice effects and carrier diffusion as well the interaction of these excitations with one another with impurities, grain boundaries, and defects. Among various transport probes, it brings information about available subsystems, and the measurements are fairly simple. The present study intends to contribute towards a thorough understanding of the scattering processes taking place in this fascinating material. The thermoelectric power behavior exhibits nearly T-linear dependence above T _c , a broad peak around 70 K, and further deviation from T-linear dependence above 200 K. In order to simulate the actual situation occurring in the temperature-dependent behavior of S(T) in Rb₃C₆₀, we considered two channels to S (T): carrier diffusion or S_c^diff using the Mott expression within parabolic band approximation and phonon drag or S_ph^drag.

S_ph^drag is discussed within the Debye-type relaxation rate approximation in terms of acoustic phonon frequency, a relaxation time τ, and the sound velocity. The total phonon relaxation rate is the sum of terms corresponding to independent scattering mechanism, like defects, grain boundaries, and phonons and holes as carriers dominating in different temperature intervals. We have made a careful analysis taking into account several different processes that could exist in this material whose interaction can yield the observed dependence.

The quasiparticles caused an increase of the carrier heat and a slow increase of the phonon contribution below T _c . The rapid increase in S(T) is attributed to the increase in phonon mean free path due to carrier condensation in the superconducting state limited by various impurity scattering mechanisms. The physical entities in the present scheme that characterize the strengths of the phonon-defect, phonon-electron, and phonon-phonon scattering lead to a result that successfully retrace the experimental curve.

The anomalous behavior around 70 K is attributed to electron-phonon interactions. The model calculations assume electrons as carriers using Mott expression within parabolic band approximation for thermoelectric power, and the negative behavior of S up to room temperature with a value of 18.9 μV/K is in good agreement with the room-temperature values in the range 10 to 20 μV/K. It is known that the satisfactory explanation of these peculiar properties is constrained as electron, and the lattice contributes to the thermoelectric power because both contributions are limited by various scattering mechanisms. Despite the limitations and use of material parameters for the estimation of transport coupling strengths, the present theoretical model on the thermoelectric power of the Rb₃C₆₀ consistently reveals the interesting behavior reported experimentally. The intermolecular acoustic phonons are solely responsible for the thermoelectric power behavior; however, electron-intramolecular phonon interaction is key for superconductivity.

Conclusively, thermoelectric power is one of those important transport entities which exhibit nonzero values in both the normal and superconducting state. Its behavior is determined by competition among the several operating scattering rates for the heat carriers and a balance between diffusive carrier, and phonon-drag contributions with acoustic phonons are effectively scattered by various scatterers for thermoelectric power. Moreover, the scatterings of quasiparticles are constrained by impurities as clearly evidenced in orientationally disordered Rb₃C₆₀. Despite the limitations, the present approach successfully describes the nearly T-linear dependence above T _c , a broad peak around 70 K, and further deviation from T-linear dependence above 200 K of thermoelectric power in Rb₃C₆₀. Although we have provided a simple phenomenological explanation of this effect, there is clearly a need for a good theoretical understanding of the transport.

Authors' information

DV is a professor in the School of Physics, Devi Ahilya University, Indore, India. NS is an assistant professor in the Department of Physics, Ranchi College, Ranchi University Ranchi.