Abstract
By constructing available upper and lower solutions and combining the Schauder's fixed point theorem with maximum principle, this paper establishes sufficient and necessary conditions to guarantee the existence of  as well as
as well as  positive solutions for a class of singular boundary value problems on time scales. The results significantly extend and improve many known results for both the continuous case and more general time scales. We illustrate our results by one example.
positive solutions for a class of singular boundary value problems on time scales. The results significantly extend and improve many known results for both the continuous case and more general time scales. We illustrate our results by one example.
Similar content being viewed by others
1. Introduction
Recently, there have been many papers working on the existence of positive solutions to boundary value problems for differential equations on time scales; see, for example, [1–22]. This has been mainly due to its unification of the theory of differential and difference equations. An introduction to this unification is given in [10, 14, 23, 24]. Now, this study is still a new area of fairly theoretical exploration in mathematics. However, it has led to several important applications, for example, in the study of insect population models, neural networks, heat transfer, and epidemic models; see, for example, [9, 10].
Motivated by works mentioned previously, we intend in this paper to establish sufficient and necessary conditions to guarantee the existence of positive solutions for the singular dynamic equation on time scales:

subject to one of the following boundary conditions:

or

where  is a time scale,
is a time scale,  , where
, where  is right dense and
is right dense and  is left dense. and
is left dense. and is continuous. Suppose further that
is continuous. Suppose further that  is nonincreasing with respect to
is nonincreasing with respect to  , and there exists a function
, and there exists a function  such that
such that

A necessary and sufficient condition for the existence of  as well as
as well as  positive solutions is given by constructing upper and lower solutions and with the maximum principle. The nonlinearity
positive solutions is given by constructing upper and lower solutions and with the maximum principle. The nonlinearity  may be singular at
may be singular at  and/or
and/or  . By singularity we mean that the functions
. By singularity we mean that the functions  in (1.1) is allowed to be unbounded at the points
in (1.1) is allowed to be unbounded at the points  and/or
and/or  A function
A function  is called a
is called a  (positive) solution of (1.1) if it satisfies (1.1) (
(positive) solution of (1.1) if it satisfies (1.1) ( ); if even
); if even  exist, we call it is a
exist, we call it is a  solution.
solution.
To the best of our knowledge, there is very few literature giving sufficient and necessary conditions to guarantee the existence of positive solutions for singular boundary value problem on time scales. So it is interesting and important to discuss these problems. Many difficulties occur when we deal with them. For example, basic tools from calculus such as Fermat's theorem, Rolle's theorem, and the intermediate value theorem may not necessarily hold. So we need to introduce some new tools and methods to investigate the existence of positive solutions for problem (1.1) with one of the above boundary conditions.
The time scale related notations adopted in this paper can be found, if not explained specifically, in almost all literature related to time scales. The readers who are unfamiliar with this area can consult, for example, [6, 11–13, 25, 26] for details.
The organization of this paper is as follows. In Section 2, we provide some necessary background. In Section 3, the main results of problem (1.1)-(1.2) will be stated and proved. In Section 4, the main results of problem (1.1)–(1.3) will be investigated. Finally, in Section 5, one example is also included to illustrate the main results.
2. Preliminaries
In this section we will introduce several definitions on time scales and give some lemmas which are useful in proving our main results.
Definition 2.1.
A time scale  is a nonempty closed subset of
is a nonempty closed subset of  .
.
Definition 2.2.
Define the forward (backward) jump operator  at
at  for
for  at
at  for
for  by
by

for all  . We assume throughout that
. We assume throughout that  has the topology that it inherits from the standard topology on
has the topology that it inherits from the standard topology on  and say
and say  is right scattered, left scattered, right dense and left dense if
is right scattered, left scattered, right dense and left dense if  and
and  , respectively. Finally, we introduce the sets
, respectively. Finally, we introduce the sets  and
and  which are derived from the time scale
which are derived from the time scale  as follows. If
as follows. If  has a left-scattered maximum
has a left-scattered maximum  , then
, then  , otherwise
, otherwise  . If
. If  has a right-scattered minimum
has a right-scattered minimum  , then
, then  , otherwise
, otherwise  .
.
Definition 2.3.
Fix  and let
and let  . Define
. Define  to be the number (if it exists) with the property that given
to be the number (if it exists) with the property that given  there is a neighborhood
there is a neighborhood  of
of  with
with

for all  , where
, where  denotes the (delta) derivative of
denotes the (delta) derivative of  with respect to the first variable, then
with respect to the first variable, then

implies

Definition 2.4.
Fix  and let
and let  . Define
. Define  to be the number (if it exists) with the property that given
to be the number (if it exists) with the property that given  there is a neighborhood
there is a neighborhood  of
of  with
with

for all  . Call
. Call  the (nabla) derivative of
the (nabla) derivative of  at the point
at the point  .
.
If  then
then  . If
. If  then
then  is the forward difference operator while
is the forward difference operator while  is the backward difference operator.
is the backward difference operator.
Definition 2.5.
A function  is called rd-continuous provided that it is continuous at all right-dense points of
is called rd-continuous provided that it is continuous at all right-dense points of  and its left-sided limit exists (finite) at left-dense points of
and its left-sided limit exists (finite) at left-dense points of  . We let
. We let  denote the set of rd-continuous functions
denote the set of rd-continuous functions  .
.
Definition 2.6.
A function  is called
is called  -continuous provided that it is continuous at all left-dense points of
-continuous provided that it is continuous at all left-dense points of  and its right-sided limit exists (finite) at right-dense points of
and its right-sided limit exists (finite) at right-dense points of  . We let
. We let  denote the set of
denote the set of  -continuous functions
-continuous functions  .
.
Definition 2.7.
A function  is called a delta-antiderivative of
is called a delta-antiderivative of  provided that
provided that  holds for all
holds for all  . In this case we define the delta integral of
. In this case we define the delta integral of  by
by

for all  .
.
Definition 2.8.
A function  is called a nabla-antiderivative of
is called a nabla-antiderivative of  provided that
provided that  holds for all
holds for all  . In this case we define the delta integral of
. In this case we define the delta integral of  by
by

for all  .
.
Throughout this paper, we assume that  is a closed subset of
is a closed subset of  with
with  .
.
Let  , equipped with the norm
, equipped with the norm

It is clear that  is a real Banach space with the norm.
is a real Banach space with the norm.
Lemma 2.9 (Maximum Principle).
Let  and
and  . If
. If  ,
,  , and
, and  Then
Then 
3. Existence of Positive Solution to (1.1)-(1.2)
In this section, by constructing upper and lower solutions and with the maximum principle Lemma 2.9, we impose the growth conditions on  which allow us to establish necessary and sufficient condition for the existence of (1.1)-(1.2).
which allow us to establish necessary and sufficient condition for the existence of (1.1)-(1.2).
We know that

is the Green's function of corresponding homogeneous BVP of (1.1)-(1.2).
We can prove that  has the following properties.
has the following properties.
Proposition 3.1.
For  , one has
, one has

To obtain positive solutions of problem (1.1)-(1.2), the following results of Lemma 3.2 are fundamental.
Lemma 3.2.
Assume that  holds. If
holds. If  and
and  exist and are finite, then one has
exist and are finite, then one has

Proof.
Without loss of generality, we suppose that there is only one right-scattered point  . Then we have
. Then we have

that is,

Similarly, we can prove

The proof is complete.
Theorem 3.3.
Suppose that  holds. Then problem (1.1)-(1.2) has a
holds. Then problem (1.1)-(1.2) has a  positive solution if and only if the following integral condition holds:
positive solution if and only if the following integral condition holds:

Proof.
-
(1)
Necessity
By (H), there exists  such that
such that  . Without loss of generality, we assume that
. Without loss of generality, we assume that  is nonincreasing on
is nonincreasing on  with
with  .
.
Suppose that  is a positive solution of problem (1.1)-(1.2), then
is a positive solution of problem (1.1)-(1.2), then

which implies that  is concave on
is concave on  . Combining this with the boundary conditions, we have
. Combining this with the boundary conditions, we have  Therefore
Therefore  . So by [10, Theorem 1.115], there exists
. So by [10, Theorem 1.115], there exists  satisfying
satisfying  or
or  . And
. And  for
for  ,
,  , for
, for  . Denote
. Denote  , then
, then  .
.
First we prove  .
.
By ( ), for any fixed
), for any fixed  , we have
, we have

It follows that

If  , then we have by (3.10)
, then we have by (3.10)

This means  , then
, then  , which is a contradiction with
, which is a contradiction with  being positive solution. Thus
being positive solution. Thus  , then
, then  .
.
Second, we prove  .
.
If  then
then

If  , then
, then  ,
,  , and
, and

It follows that

By (3.14) we have


Combining this with (3.10) we obtain

Similarly

Then we can obtain

-
(2)
Sufficiency
Let

Then

Let

then 
Let  then
then

So, we have

and  . Hence
. Hence  are lower and upper solutions of problem (1.1)-(1.2), respectively. Obviously
are lower and upper solutions of problem (1.1)-(1.2), respectively. Obviously  for
for  .
.
Now we prove that problem (1.1)-(1.2) has a positive solution  with
with 
Define a function

Then  is continuous. Consider BVP
is continuous. Consider BVP

Define mapping  by
by

Then problem (1.1)-(1.2) has a positive solution if and only if  has a fixed point
has a fixed point  with
with 
Obviously  is continuous. Let
is continuous. Let  By (3.7) and (3.16), for all
By (3.7) and (3.16), for all  , we have
, we have

Then  is bounded. By the continuity of
is bounded. By the continuity of  we can easily found that
we can easily found that  are equicontinuous. Thus
are equicontinuous. Thus  is completely continuous. By Schauder fixed point theorem we found that
is completely continuous. By Schauder fixed point theorem we found that  has at least one fixed point
has at least one fixed point  .
.
We prove  . If there exists
. If there exists  such that
such that

Let 

 then
then  for
for  Thus
Thus  . By (3.24) we know that
. By (3.24) we know that  And
And  . By Lemma 2.9 we have
. By Lemma 2.9 we have  , which is a contradiction. Then
, which is a contradiction. Then  . Similarly we can prove
. Similarly we can prove  . The proof is complete.
. The proof is complete.
Theorem 3.4.
Suppose that ( ) holds. Then problem (1.1)-(1.2) has a
) holds. Then problem (1.1)-(1.2) has a  positive solution if and only if the following integral condition holds:
positive solution if and only if the following integral condition holds:

Proof.
-
(1)
Necessity
Let  be a positive solution of problem (1.1)-(1.2). Then
be a positive solution of problem (1.1)-(1.2). Then  is decreasing on
is decreasing on  . Hence
. Hence  is integrable and
is integrable and

By simple computation and using [10, Theorem 1.119], we obtain  . So there exist
. So there exist  such that
such that  . By
. By  we obtain
we obtain

By

we have
-
(2)
Sufficiency
Let  , then
, then

Similar to Theorem 3.3, let  , there exists
, there exists  satisfying
satisfying  , and
, and

then  is integral and
is integral and  exist, hence
exist, hence  is a positive solution in
is a positive solution in  . The proof is complete.
. The proof is complete.
4. Existence of Positive Solution to (1.1)–(1.3)
Now we deal with problem (1.1)–(1.3). The method is just similar to what we have done in Section 3, so we omit the proof of main result of this section.
Let

be the Green's function of corresponding homogeneous BVP of (1.1)–(1.3).
We can prove that  has the following properties.
has the following properties.
Similar to (3.2), we have

Theorem 4.1.
Suppose that  holds, then problem (1.1)–(1.3) has a
holds, then problem (1.1)–(1.3) has a  positive solution if and only if the following integral condition holds:
positive solution if and only if the following integral condition holds:

Theorem 4.2.
Suppose that  holds, then problem (1.1)–(1.3) has a
holds, then problem (1.1)–(1.3) has a  positive solution if and only if the following integral condition holds:
positive solution if and only if the following integral condition holds:

5. Example
To illustrate how our main results can be used in practice we present an example.
Example 5.1.
We have

where  Select
Select  , then we have
, then we have  Moreover, we have
Moreover, we have

By Theorem 3.3, problem (5.1) has a positive solution in  .
.
Remark 5.2.
Example 5.1 implies that there is a large number of functions that satisfy the conditions of Theorem 3.3. In addition, the conditions of Theorem 3.3 are also easy to check.
References
Anderson DR: Eigenvalue intervals for a two-point boundary value problem on a measure chain. Journal of Computational and Applied Mathematics 2002,141(1-2):57-64. 10.1016/S0377-0427(01)00435-6
Avery RI, Anderson DR: Existence of three positive solutions to a second-order boundary value problem on a measure chain. Journal of Computational and Applied Mathematics 2002,141(1-2):65-73. 10.1016/S0377-0427(01)00436-8
Atici FM, Guseinov GSh: On Green's functions and positive solutions for boundary value problems on time scales. Journal of Computational and Applied Mathematics 2002,141(1-2):75-99. 10.1016/S0377-0427(01)00437-X
Anderson D, Avery R, Henderson J:Existence of solutions for a one dimensional
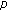 -Laplacian on time-scales. Journal of Difference Equations and Applications 2004,10(10):889-896. 10.1080/10236190410001731416
-Laplacian on time-scales. Journal of Difference Equations and Applications 2004,10(10):889-896. 10.1080/10236190410001731416Bohner M, Luo H: Singular second-order multipoint dynamic boundary value problems with mixed derivatives. Advances in Difference Equations 2006, 2006:-15.
Luo H, Ma Q: Positive solutions to a generalized second-order three-point boundary-value problem on time scales. Electronic Journal of Differential Equations 2005,2005(17):1-14.
Henderson J:Multiple solutions for 2
 th order Sturm-Liouville boundary value problems on a measure chain. Journal of Difference Equations and Applications 2000,6(4):417-429. 10.1080/10236190008808238
th order Sturm-Liouville boundary value problems on a measure chain. Journal of Difference Equations and Applications 2000,6(4):417-429. 10.1080/10236190008808238Henderson J, Peterson A, Tisdell CC: On the existence and uniqueness of solutions to boundary value problems on time scales. Advances in Difference Equations 2004,2004(2):93-109. 10.1155/S1687183904308071
Agarwal RP, Bohner M, Li W-T: Nonoscillation and Oscillation: Theory for Functional Differential Equations, Monographs and Textbooks in Pure and Applied Mathematics. Volume 267. Marcel Dekker, New York, NY, USA; 2004:viii+376.
Bohner M, Peterson A: Dynamic Equations on Time Scales. An Introduction with Applications. Birkhäuser, Boston, Mass, USA; 2001:x+358.
He Z:Double positive solutions of three-point boundary value problems for
 -Laplacian dynamic equations on time scales. Journal of Computational and Applied Mathematics 2005,182(2):304-315. 10.1016/j.cam.2004.12.012
-Laplacian dynamic equations on time scales. Journal of Computational and Applied Mathematics 2005,182(2):304-315. 10.1016/j.cam.2004.12.012He Z, Jiang X:Triple positive solutions of boundary value problems for
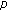 -Laplacian dynamic equations on time scales. Journal of Mathematical Analysis and Applications 2006,321(2):911-920. 10.1016/j.jmaa.2005.08.090
-Laplacian dynamic equations on time scales. Journal of Mathematical Analysis and Applications 2006,321(2):911-920. 10.1016/j.jmaa.2005.08.090Tian Y, Ge W: Existence and uniqueness results for nonlinear first-order three-point boundary value problems on time scales. Nonlinear Analysis: Theory, Methods & Applications 2008,69(9):2833-2842. 10.1016/j.na.2007.08.054
Lakshmikantham V, Sivasundaram S, Kaymakcalan B: Dynamic Systems on Measure Chains, Mathematics and Its Applications. Volume 370. Kluwer Academic Publishers, Dordrecht, The Netherlands; 1996:x+285.
Erbe L, Peterson A, Saker SH: Hille-Kneser-type criteria for second-order dynamic equations on time scales. Advances in Difference Equations 2006, 2006:-18.
Khan RA, Nieto JJ, Otero-Espinar V: Existence and approximation of solution of three-point boundary value problems on time scales. Journal of Difference Equations and Applications 2008,14(7):723-736. 10.1080/10236190701840906
Agarwal RP, Otero-Espinar V, Perera K, Vivero DR: Multiple positive solutions in the sense of distributions of singular BVPs on time scales and an application to Emden-Fowler equations. Advances in Difference Equations 2008, 2008:-13.
Wang D-B:Three positive solutions of three-point boundary value problems for
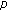 -Laplacian dynamic equations on time scales. Nonlinear Analysis: Theory, Methods & Applications 2008,68(8):2172-2180. 10.1016/j.na.2007.01.037
-Laplacian dynamic equations on time scales. Nonlinear Analysis: Theory, Methods & Applications 2008,68(8):2172-2180. 10.1016/j.na.2007.01.037Sun J-P: A new existence theorem for right focal boundary value problems on a measure chain. Applied Mathematics Letters 2005,18(1):41-47. 10.1016/j.aml.2003.04.008
Feng M, Zhang X, Ge W: Positive solutions for a class of boundary value problems on time scales. Computers & Mathematics with Applications 2007,54(4):467-475. 10.1016/j.camwa.2007.01.031
Feng M-Q, Li X-G, Ge W-G:Triple positive solutions of fourth-order four-point boundary value problems of
 -Laplacian dynamic equations on time scales. Advances in Difference Equations 2008, 2008:-9.
-Laplacian dynamic equations on time scales. Advances in Difference Equations 2008, 2008:-9.Feng M, Feng H, Zhang X, Ge W:Triple positive solutions for a class of
 -point dynamic equations on time scales with
-point dynamic equations on time scales with 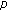 -Laplacian. Mathematical and Computer Modelling 2008,48(7-8):1213-1226. 10.1016/j.mcm.2007.12.016
-Laplacian. Mathematical and Computer Modelling 2008,48(7-8):1213-1226. 10.1016/j.mcm.2007.12.016Bohner M, Peterson A (Eds): Advances in Dynamic Equations on Time Scales. Birkhäuser, Boston, Mass, USA; 2003:xii+348.
Hilger S: Analysis on measure chains—a unified approach to continuous and discrete calculus. Results in Mathematics 1990,18(1-2):18-56.
Henderson J, Tisdell CC: Topological transversality and boundary value problems on time scales. Journal of Mathematical Analysis and Applications 2004,289(1):110-125. 10.1016/j.jmaa.2003.08.030
He Z:Existence of two solutions of
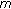 -point boundary value problem for second order dynamic equations on time scales. Journal of Mathematical Analysis and Applications 2004,296(1):97-109. 10.1016/j.jmaa.2004.03.051
-point boundary value problem for second order dynamic equations on time scales. Journal of Mathematical Analysis and Applications 2004,296(1):97-109. 10.1016/j.jmaa.2004.03.051
Acknowledgments
This work is sponsored by the National Natural Science Foundation of China (10671012, 10671023) and the Scientific Creative Platform Foundation of Beijing Municipal Commission of Education (PXM2008-014224-067420).
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 2.0 International License ( https://creativecommons.org/licenses/by/2.0 ), which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
About this article
Cite this article
Feng, M., Zhang, X., Li, X. et al. Necessary and Sufficient Conditions for the Existence of Positive Solution for Singular Boundary Value Problems on Time Scales. Adv Differ Equ 2009, 737461 (2009). https://doi.org/10.1155/2009/737461
Received:
Revised:
Accepted:
Published:
DOI: https://doi.org/10.1155/2009/737461



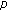 -Laplacian on time-scales. Journal of Difference Equations and Applications 2004,10(10):889-896. 10.1080/10236190410001731416
-Laplacian on time-scales. Journal of Difference Equations and Applications 2004,10(10):889-896. 10.1080/10236190410001731416 th order Sturm-Liouville boundary value problems on a measure chain. Journal of Difference Equations and Applications 2000,6(4):417-429. 10.1080/10236190008808238
th order Sturm-Liouville boundary value problems on a measure chain. Journal of Difference Equations and Applications 2000,6(4):417-429. 10.1080/10236190008808238 -Laplacian dynamic equations on time scales. Journal of Computational and Applied Mathematics 2005,182(2):304-315. 10.1016/j.cam.2004.12.012
-Laplacian dynamic equations on time scales. Journal of Computational and Applied Mathematics 2005,182(2):304-315. 10.1016/j.cam.2004.12.012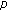 -Laplacian dynamic equations on time scales. Journal of Mathematical Analysis and Applications 2006,321(2):911-920. 10.1016/j.jmaa.2005.08.090
-Laplacian dynamic equations on time scales. Journal of Mathematical Analysis and Applications 2006,321(2):911-920. 10.1016/j.jmaa.2005.08.090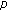 -Laplacian dynamic equations on time scales. Nonlinear Analysis: Theory, Methods & Applications 2008,68(8):2172-2180. 10.1016/j.na.2007.01.037
-Laplacian dynamic equations on time scales. Nonlinear Analysis: Theory, Methods & Applications 2008,68(8):2172-2180. 10.1016/j.na.2007.01.037 -Laplacian dynamic equations on time scales. Advances in Difference Equations 2008, 2008:-9.
-Laplacian dynamic equations on time scales. Advances in Difference Equations 2008, 2008:-9. -point dynamic equations on time scales with
-point dynamic equations on time scales with 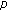 -Laplacian. Mathematical and Computer Modelling 2008,48(7-8):1213-1226. 10.1016/j.mcm.2007.12.016
-Laplacian. Mathematical and Computer Modelling 2008,48(7-8):1213-1226. 10.1016/j.mcm.2007.12.016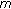 -point boundary value problem for second order dynamic equations on time scales. Journal of Mathematical Analysis and Applications 2004,296(1):97-109. 10.1016/j.jmaa.2004.03.051
-point boundary value problem for second order dynamic equations on time scales. Journal of Mathematical Analysis and Applications 2004,296(1):97-109. 10.1016/j.jmaa.2004.03.051