1 Introduction

Similar to Newton’s apple, under gravity an antimatter object falls down, not up. This is the new finding in a recent experiment at CERN [1]. Since we analyzed the fall of a neutral particle in the field of a thick plate (a slab) [2], we wish to analyze a similar problem for a charged test particle. It was shown that a chargeless mass, for specific Kasner exponents [3] to parameterize our plate geometry, falls Newtonian-like, namely, downward. Our analysis was for specific exponents which can easily be generalized to check the fall/levitation of a particle in any given geometry. The geometry of a plate, both thin [4] and thick, is described by the generic line element

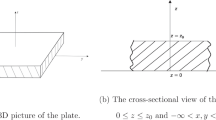
$$\begin{aligned} \text {d}s^{2}=f^{2k\left( k-1\right) }\left( \text {d}t^{2}-\text {d}z^{2}\right) -f^{2k} \text {d}x^{2}-f^{2\left( 1-k\right) }\text {d}y^{2} \end{aligned}$$
(1)

where the metric function \(f\left( z\right)\) depends only on z, and the parameter k relates to the Kasner exponents [3]. We note that our ordering of coordinates is \(x^{\mu }=\left( t,z,x,y\right)\). For \(k=\frac{1}{2}\), we have \(\left( x,y\right)\) symmetry with respect to the z-axis, whereas for \(k\ne \frac{1}{2}\), there is no such symmetry. The asymmetry naturally reflects in the stress-energy distribution of the plate. The reason that we consider z as the singled-out coordinate is to study the fall problem in the z-direction. Similar analysis can be conducted in spherical geometry in which the fall amounts to inward or outward motion relative to the central mass. The choice of \(f\left( z\right)\) in the present problem characterizes the nature of the plate. By virtue of the \(\pm z\) symmetry, we consider only the upper half, \(z>0\) part of the plate. To make our spacetime regular, free of singularity, we consider a particular plate confined in \(0<z\le z_{0}\), where \(z_{0}\) represents the thickness of the plate. In summary, the relevant spacetime is described by the pair of line elements [2]

$$\begin{aligned} \text {d}s^{2}=z^{2k\left( k-1\right) }\left( \text {d}t^{2}-\text {d}z^{2}\right) -z^{2k} \text {d}x^{2}-z^{2\left( 1-k\right) }\text {d}y^{2} \textit{,}\hspace{4.6cm} \left( \text {for } z>z_{0}\right) \end{aligned}$$
(2)

and

$$\begin{aligned} \text {d}s^{2}=\left( \cosh a_{0}z\right) ^{2k\left( k-1\right) }\left( \text {d}t^{2}-\text {d}z^{2}\right) -\left( \cosh a_{0}z\right) ^{2k}\text {d}x^{2}-\left( \cosh a_{0}z\right) ^{2\left( 1-k\right) }\text {d}y^{2} \textit{,}\hspace{0.5cm} \left( \text {for } 0< z\le z_{0}\right) \end{aligned}$$
(3)

representing the outer vacuum \(\left( z>z_{0}\right)\) and inner \(\left( 0\le z\le z_{0}\right)\) sourceful region, respectively. The constant \(a_{0}\) is introduced such that \(a_{0}^{2}\) is proportional to the energy density of the plate. As described in [2], the energy–momentum components of the thick plate are

$$\begin{aligned} T_{tt}&=a_{0}^{2}\left( \Theta \left( z\right) -\Theta \left( z-z_{0}\right) \right) \nonumber \\ T_{xx}&=-\left( k-1\right) ^{2}\left( \cosh a_{0}z\right) ^{2k\left( 2-k\right) }T_{tt}\\ T_{yy}&=-k^{2}\left( \cosh a_{0}z\right) ^{2\left( 1-k^{2}\right) }T_{tt}\nonumber \\ T_{zz}&=0\nonumber \end{aligned}$$
(4)

in which \(\Theta \left( z\right)\) stands for the step function.Footnote 1 In accordance with the definition \(T_{\mu }^{\nu }=\text {diag}\left( -\rho ,p_{x},p_{y},p_{z}\right)\), we obtain (for \(0<z<z_{0}\))

$$\begin{aligned} \rho&=-a_{0}^{2}\left( \cosh a_{0}z\right) ^{2k\left( 1-k\right) }\nonumber \\ p_{x}&=a_{0}^{2}\left( k-1\right) ^{2}\left( \cosh a_{0}z\right) ^{2k\left( 1-k\right) }\nonumber \\ p_{y}&=a_{0}^{2}k^{2}\left( \cosh a_{0}z\right) ^{2k\left( 1-k\right) } \\ p_{z}&=0\nonumber \end{aligned}$$
(5)

A test electric field can be obtained from the Maxwell equation \(\varvec{\nabla }_{\mu }F^{\mu \nu }=0\), where \(F_{\mu \nu }=\partial _{\mu }A_{\nu }-\partial _{\nu }A_{\mu }\) in the vacuum region \(z>z_{0}\). We obtain \(A_{\mu }=\left( E_{0}z^{2k\left( k-1\right) },0,0,0\right)\), in which \(E_{0}=\)const, in the background vacuum geometry. Similarly, a test magnetic field solution is obtained in the form \(A_{\mu }=\left( A_{t},A_{z},A_{x},A_{y}\right) =\left( 0,0, B_{0}z^{2k},0\right)\) with \(B_{0}=\)const. In the following section, we shall choose the particular exponent with \(2k\left( k-1\right) =1\), which amounts to \(k=\frac{1}{2}\left( 1\pm \sqrt{3}\right)\). This particular k gives us the simplest form for the test electric field, although the source is not isotropic.

2 Charged particle fall on the anisotropic plate

The motion of a test particle is described by the geodesic Lagrangian [5] (with \(2k\left( k-1\right) =1\))

$$\begin{aligned} \mathcal {L}=\frac{1}{2}z\left( \overset{\cdot }{t}^{2}-\overset{\cdot }{z}^{2}\right) -\frac{1}{2}z^{2k}\overset{\cdot }{x}^{2}-\frac{1}{2}z^{2\left( 1-k\right) }\overset{\cdot }{y}^{2}+qA_{\mu }\overset{\cdot }{x}^{\mu } \end{aligned}$$
(6)

in which q is the test particle charge, and \(A_{\mu }\) is the corresponding test potential found above. Also a ‘dot’ represents proper time derivative. We proceed now with the electric and magnetic solutions separately.

2.1 The acceleration in electric field

Fig. 1
figure 1

Acceleration plots \(a_{+}\) (for \(q>0\)) and \(a_{-}\) (for \(q<0\)) versus \(\alpha =qz\). Since we considered the half-space \(z>0\), the sign of \(\alpha\) is the sign of q. The starting point for \(\alpha\) from below is \(\alpha =qz_{0}\) since \(z>z_{0}\), and extends to \(z\xrightarrow {}\infty\) upward. The \(q<0\) particle attains \(\left( -\right)\) acceleration only for \(-1<\alpha <0\). For \(-2<\alpha <-1\), it has \(\left( +\right)\), decreasing acceleration with \(a_{-}=0\) at \(\alpha =-1\). It reaches to \(a_{-}=-\frac{1}{2}\) at \(\alpha =0\) corresponding to \(q=0\). Overall, the \(q<0\) particle has a bounded acceleration \(-\frac{1}{2}<a_{-}<\frac{1}{54}\) with a maximum at \(\alpha =-2\). On the other hand, the \(q>0\) particle starts at \(a_{+}=-\frac{1}{2}\) at \(\alpha =0\) (for \(q=0\)) and drops without bound until \(\alpha =1\). In the domain \(\alpha >1\), the \(q>0\) particle starts with an unbounded repulsive acceleration to drop to \(a_{+}=0\) with \(\alpha \xrightarrow {}\infty\) (or \(z\xrightarrow {}\infty\)). We note that consideration of the mirror-symmetric domain, i.e., for \(z<-z_{0}\), part of the space will change the roles of the particle and antiparticle accelerations

Full size image

We have \(A_{\mu }=\left( E_{0}z,0,0,0\right)\) to be substituted into (4), and for simplicity, we set \(\overset{\cdot }{x}=0=\overset{\cdot }{y}\), to study the motion only in z-direction. The metric condition for time-like geodesics \(\text {d}s^{2}=g_{tt}\text {d}t^{2}+g_{zz}\text {d}z^{2}\), becomes \(\overset{\cdot }{t}^{2}-\overset{\cdot }{z}^{2}=\frac{1}{z}\). A first integral of motion with \(E=\text{const}\) gives

$$\begin{aligned} \overset{\cdot }{t}=\frac{E}{z}-qE_{0} \end{aligned}$$
(7)

By using this together, with the geodesic condition and transforming the proper time derivative into the coordinate time derivative, we obtain

$$\begin{aligned} \frac{\text {d}^{2}z}{\text {d}t^{2}}&=-\frac{1}{2}\frac{1+\alpha }{\left( 1-\alpha \right) ^{3}} \\ (\alpha&=qz\textit{)}\nonumber \end{aligned}$$
(8)

Note that the unit of acceleration is the inverse length in the geometrical unit system. It is observed that acceleration depends on the sign of the charge q. (Recall that our choice for the plate was \(z>0\)). Figure 1 describes the acceleration for \(q>0\) (\(a_{+}\)) and \(q<0\) (\(a_{-}\)) for \(z_{0}<z<\infty\). At this point, we wish to comment also on the divergence of acceleration in (8), at \(\alpha =1\). The \(\alpha =qz=1\) is a singularity of acceleration \(\frac{\text {d}^{2}z}{\text {d}t^{2}}\), not a spacetime singularity. It can easily be shown that the proper acceleration \(\frac{\text {d}^{2}z}{\text {d}\tau ^{2}}\) is everywhere finite. The problem originates from the usage of the coordinate time t of the Newtonian theory which lacks a proper time. We can still make use of expression (8) by choosing \(\alpha <1\) or \(\alpha >1\) to avoid the divergence. Infinite planes are known to host spacetime singularities at a finite distance [6, 7]. For this reason, we have chosen a thick plate whose external region is free of singularity. Yet we encounter a trouble at \(\alpha =1\) which can be circumvented by tuning the test charge.

2.2 Acceleration of a charge in the test magnetic field \(B_{y}\ne {0}\)


In section I, we have obtained the test magnetic field solution of the Maxwell equation as \(A_{\mu }=\left( 0,0, B_{0}z^{2k},0\right)\), where \(A_{x}=B_{0}z^{2k}\), so that we have only \(B_{y}\ne {0}\). The test particle Lagrangian with unit mass is given by

$$\begin{aligned} \mathcal {L}=\frac{1}{2}z\left( \overset{\cdot }{t}^{2}-\overset{\cdot }{z}^{2}\right) -\frac{1}{2}z^{2k}\overset{\cdot }{x}^{2}-\frac{1}{2}z^{2\left( 1-k\right) }\overset{\cdot }{y}^{2}+qB_{0}z^{2k}\overset{\cdot }{x}\ \end{aligned}$$
(9)

in which the last term will generate the Lorentz force in the equation of motion. For convenience, we set \(\overset{\cdot }{y}=0\), to obtain the first integrals

$$\begin{aligned} z\overset{\cdot }{t} &=E=\text {const.} \nonumber \\ z^{2k}\left( \overset{\cdot }{x}-qB_{0}\right) &=\alpha _{0}=\text {const}. \end{aligned}$$
(10)

Together with the geodesic condition, we eliminate the proper time in favor of t and obtain

$$\begin{aligned} \left( \frac{\text {d}z}{\text {d}t}\right) ^{2}=1-\frac{z}{E^{2}}\left[ 1+z^{2k}\left( qB_{0}+\alpha _{0}z^{-2k}\right) ^{2}\right] \end{aligned}$$
(11)

Upon taking \(\frac{\text {d}}{\text {d}t}\) of both sides results in

$$\begin{aligned} \frac{\text {d}^{2}z}{\text {d}t^{2}}=-\frac{1}{2E^{2}}\big [1+\left( qB_{0}+\alpha _{0}z^{-2k}\right) \left[ \left( 2k+1\right) qB_{0}z^{2k}+\alpha _{0}\right] \big ] \end{aligned}$$
(12)

in which \(2k\left( k-1\right) =1\), is to be imposed.

Fig. 2
figure 2

Acceleration plots versus z for various constants \(qB_{0}k\). As we considered the half-space \(z>z_{0}>0\) with the specific choice of Kasner exponent, \(2k\left( k-1\right) =1\), we will not face a singularity for acceleration, and we will have two different accelerations which come from two solutions for \(k=\frac{1}{2}\left( 1\pm \sqrt{3}\right)\). In case of \(q=0\), acceleration will be \(-\frac{1}{2}\). However, despite both acceleration behaviors, for a charged particle in the magnetic field, acceleration is attractive irrespective of the sign of the particle charge

Full size image

This acceleration can be analyzed for various \(\alpha _{0}\) values; however, for technical reasons, we make the choice \(\alpha _{0}=0\). This particular choice reduces (12) to a simple form

$$\begin{aligned} \frac{\text {d}^{2}z}{\text {d}t^{2}}=-\frac{1}{2E^{2}}\big [1+2\left( qB_{0}k\right) ^{2}z^{2k}\big ] \end{aligned}$$
(13)

which informs about the sign of acceleration, irrespective of the sign of q. Figure 2 plots this acceleration for different parameters. Interestingly, the singularity that we faced in the electric field case in Eq. (8) does not arise in the magnetic field problem.

3 The isotropic plate (\(k=\frac{1}{2}\))

Our vacuum line element for \(z>z_{0}\) takes the form for \(k=\frac{1}{2}\)

$$\begin{aligned} \text {d}s^{2}=\frac{1}{\sqrt{z}}\left( \text {d}t^{2}-\text {d}z^{2}\right) -z\left( \text {d}x^2+\text {d}y^{2}\right) \end{aligned}$$
(14)

whereas for the inside (\(0<z<z_{0}\)), we have

$$\begin{aligned} \text {d}s^{2}=\left( \cosh {a_{0}z}\right) ^{-\frac{1}{2}}\left( \text {d}t^{2}-\text {d}z^{2}\right) -\left( \cosh {a_{0}z}\right) \left( \text {d}x^2+\text {d}y^{2}\right) \end{aligned}$$
(15)

The energy–momentum, \(T_{\mu \nu }=\left( -\rho ,p_{x},p_{y},p_{z}\right)\), has components

$$\begin{aligned} \rho&=-a_{0}^{2}\left( \cosh a_{0}z\right) ^{\frac{1}{2}}\nonumber \\ p_{x}&=p_{y}= \frac{1}{4}a_{0}^{2}\left( \cosh a_{0}z\right) ^{\frac{1}{2}}\\ p_{z}&=0\nonumber \end{aligned}$$
(16)

The energy conditions are violated. To check the weak energy condition (WEC), we see that \(\rho <0\) and \(\rho +p_{i}<0\), mean such a violation. To consider the test Maxwell solution for the electric field, we can easily check that \(A_{\mu }=\left( \frac{E_{1}}{\sqrt{z}},0,0,0\right)\) solves the Maxwell equation with \(E_{1}=\)const. Note that since \(z>z_{0}\), there is no divergence problem in the test potential. Our Lagrangian for the present problem is (with \(\overset{\cdot }{x}=\overset{\cdot }{y}=0\))

$$\begin{aligned} \mathcal {L}=\frac{1}{2\sqrt{z}}\left( \overset{\cdot }{t}^{2}-\overset{\cdot }{z}^{2}\right) +\frac{qE_{1}}{\sqrt{z}}\overset{\cdot }{t} \end{aligned}$$
(17)

which yields the first integral

$$\begin{aligned} \overset{\cdot }{t}\ {}&= C_{1}\sqrt{z}-qE_{1}\\ {(}C_{1}&=\text {const.)}\nonumber \end{aligned}$$
(18)

Combining this with the time-like geodesic condition gives

$$\begin{aligned} \left( \frac{\text {d}z}{\text {d}t}\right) ^{2}=1-\frac{\sqrt{z}}{\left( C_{1}\sqrt{z}-qE_{1}\right) ^{2}} \end{aligned}$$
(19)

which results upon differentiation in the acceleration

$$\begin{aligned} \frac{\text {d}^{2}z}{\text {d}t^{2}}=\frac{1}{4C_{1}^{2}z^{\frac{3}{2}}}\frac{\left( 1+\beta \right) }{\left( 1-\beta \right) ^{3}} \end{aligned}$$
(20)

for \(\beta =\frac{qE_{1}}{C_{1}\sqrt{z}}\). It is seen that choosing (\(E_{1}>0\)) and \(C_{1}>0\) provides, for \(-1<\beta <+1\), a positive acceleration, namely, levitation. For \(\beta <-1\) and \(\beta >+1\), we have (−) acceleration as in the Newton’s theory. The role of the sign of charge of the test particle is manifest in these results, and the singularity at \(\beta =1\) can be argued similar to the case of \(\alpha =1\) of Eq. (8). The interesting point about Eq. (20) is that for \(q=0\), the test particle falls ’up’ from the plate. This was the reason that we had to choose our exponent factor \(k\ne \frac{1}{2}\) in Ref. [2]. From a physics standpoint, it seems that the asymmetric plate creates extra stresses to render a downward fall possible.

4 Conclusion

We study charged test particles falling toward a plate of thickness \(z_{0}\). For \(z>z_{0}\), we have vacuum space and particles move in this region. Our analysis shows that downward fall is not guaranteed throughout the space \(z>z_{0}\). A similar result is valid also for uncharged particles covering the case of neutral matter/antimatter. We provide the fall only for specific Kasner-type parameter k. In the asymmetric plate case, different stresses/forces arise in the plate which become effective in providing the fall. The presence of opposite charges in the test particles yields much different accelerations. Namely, the fall is charge asymmetric: If a particle accelerates slowly during the fall, its antiparticle attains much different acceleration. Figure 1 depicts this fact. Our plate is chosen to be free of singularities which fails to satisfy the WEC. We note also that the choice (for \(k=\frac{1}{2}\)) of the metric function \(f\left( z\right) =1-|az|\) (\(a=\) const.) gives a domain wall [8,9,10] with distributional energy conditions satisfied in integral form and has similarity to a brane world model in higher dimensions [11]. Such a choice, however, involves a singular hypersurface at a finite distance. For a detailed analysis of planer symmetric geometry, one may consult [6, 7]. As a final remark, we speculate that any presence of a dipole coupling in the \(\left( \pm \right) z\) directions can split matter versus antimatter in the fall problem. Such an analysis, which is to justify the theoretical prediction of [1], remains to be seen.