Skip to main content
SpringerLink
Log in

Optimal solutions of Lie subalgebra, dynamical system, travelling wave solutions and conserved currents of (3+1)-dimensional generalized Zakharov–Kuznetsov equation type I

  • Regular Article
  • Published:
The European Physical Journal Plus Aims and scope Submit manuscript

Abstract

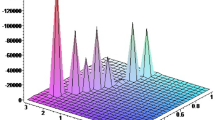
In this paper, we analytically examine a (3+1)-dimensional generalized Zakharov–Kuznetsov equation which contains the (3+1)-dimensional ZK as well as mKdV–ZK equations. We contemplate both the power-law and dual power-law of the equation. We subsequently utilize the Lie symmetries technique to reduce the partial differential equations to various ordinary differential equations via an optimal system of Lie subalgebras in one dimension. Furthermore, diverse travelling wave solutions are obtained. These solutions include various kinds of solitary wave solutions, periodic wave solutions as well as two families of unbounded exact solutions. In order to appreciate the somatic appearance of this model, we pictorially depict the motions of the secured results. Imposing the Helmholtz conditions, we gain the conserved vectors by engaging Noether’s theorem.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20
Fig. 21
Fig. 22

Similar content being viewed by others

References

  1. O.D. Adeyemo, T. Motsepa, C.M. Khalique, A study of the generalized nonlinear advection-diffusion equation arising in engineering sciences. Alex. Eng. J. 61, 185–194 (2022)

    Article  Google Scholar 

  2. C.M. Khalique, O.D. Adeyemo, A study of (3+1)-dimensional generalized Korteweg-de Vries-Zakharov-Kuznetsov equation via Lie symmetry approach. Results Phys. 18, 103197 (2020)

    Article  Google Scholar 

  3. A. Shafiq, C.M. Khalique, Lie group analysis of upper convected Maxwell fluid flow along stretching surface. Alex. Eng. J. 59, 2533–2541 (2020)

    Article  Google Scholar 

  4. N. Benoudina, Y. Zhang, C.M. Khalique, Lie symmetry analysis, optimal system, new solitary wave solutions and conservation laws of the Pavlov equation. Commun. Nonlinear Sci. Numer. Simulat. 94, 105560 (2021)

    Article  MathSciNet  MATH  Google Scholar 

  5. O.D. Adeyemo, C.M. Khalique, Dynamics of soliton waves of group-invariant solutions through optimal system of an extended KP-like equation in higher dimensions with applications in medical sciences and mathematical physics. J. Geom. Phys. 177, 104502 (2022)

    Article  MathSciNet  MATH  Google Scholar 

  6. C.M. Khalique, O.D. Adeyemo, K. Maefo, Symmetry solutions and conservation laws of a new generalized 2D Bogoyavlensky-Konopelchenko equation of plasma physics. AIMS Math. 7, 9767–9788 (2022)

    Article  MathSciNet  Google Scholar 

  7. M.J. Ablowitz, P.A. Clarkson, Solitons, Nonlinear Evolution Equations and Inverse Scattering (Cambridge University Press, Cambridge, 1991)

    Book  MATH  Google Scholar 

  8. C.H. Gu, Soliton Theory and Its Application (Zhejiang Science and Technology Press, Zhejiang, 1990)

    Google Scholar 

  9. Y. Zhou, M. Wang, Y. Wang, Periodic wave solutions to a coupled KdV equations with variable coefficients. Phys. Lett. A 308, 31–36 (2003)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  10. N.A. Kudryashov, N.B. Loguinova, Extended simplest equation method for nonlinear differential equations. Appl. Math. Comput. 205, 396–402 (2008)

    MathSciNet  MATH  Google Scholar 

  11. L.V. Ovsiannikov, Group Analysis of Differential Equations (Academic Press, New York, 1982)

    MATH  Google Scholar 

  12. L. Zhang, C.M. Khalique, Classification and bifurcation of a class of second-order ODEs and its application to nonlinear PDEs. Discrete and Continuous dynamical systems Series S 11(4), 777–790 (2018)

    MathSciNet  MATH  Google Scholar 

  13. M. Wang, X. Li, J. Zhang, The \( (G^{\prime }/G)-\) expansion method and travelling wave solutions for linear evolution equations in mathematical physics. Phys. Lett. A 24, 1257–1268 (2005)

    Google Scholar 

  14. N.A. Kudryashov, Simplest equation method to look for exact solutions of nonlinear differential equations. Chaos Solitons Fracts. 24, 1217–1231 (2005)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  15. V.B. Matveev, M.A. Salle, Darboux Transformations and Solitons (Springer, New York, 1991)

    Book  MATH  Google Scholar 

  16. Y. Chen, Z. Yan, New exact solutions of (2+1)-dimensional Gardner equation via the new sine-Gordon equation expansion method. Chaos Solitons Fracts. 26, 399–406 (2005)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  17. R. Hirota, The Direct Method in Soliton Theory (Cambridge University Press, Cambridge, 2004)

    Book  MATH  Google Scholar 

  18. V.E. Zakharov, E.A. Kuznetsov, On three-dimensional solitons. Zhurnal Eksp. Teoret. Fiz. 66, 594–597 (1974)

    ADS  Google Scholar 

  19. H.L. Zhen, B. Tian, H. Zhong, Y. Jiang, Dynamic behaviors and soliton solutions of the modified Zakharov-Kuznetsov equation in the electrical transmission line. Comput. Math. Appl. 68, 579–588 (2014)

    Article  MATH  Google Scholar 

  20. A.R. Seadawy, Stability analysis for two-dimensional ion-acoustic waves in quantum plasmas. Phys. Plasmas 21, 052107 (2014)

    Article  ADS  Google Scholar 

  21. B.K. Shivamoggi, Nonlinear ion-acoustic waves in a magnetized plasma and the Zakharov-Kuznetsov equation. J. Plasma Phys. 41, 83–88 (1989)

    Article  ADS  Google Scholar 

  22. T. Nawaz, A. Yıldırım, S.T. Mohyud-Din, Analytical solutions Zakharov-Kuznetsov equations. Adv. Powder Technol. 24, 252–256 (2013)

    Article  Google Scholar 

  23. L.D. Moleleki, B. Muatjetjeja, A.R. Adem, Solutions and conservation laws of a (3+1)-dimensional Zakharov-Kuznetsov equation. Nonlinear Dyn. 87, 2187–2192 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  24. S. Kumar, D. Kumar, Solitary wave solutions of (3+1)-dimensional extended Zakharov-Kuznetsov equation by Lie symmetry approach. Comput. Math. Appl. 77, 2096–2113 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  25. G. Magalakwe, C.M. Khalique, Conservation laws for a (3+1)-dimensional extended Zakharov-Kuznetsov equation. AIP Conf. Proc. 2116, 190008 (2019). https://doi.org/10.1063/1.5114177

    Article  Google Scholar 

  26. M.H. Islam, K. Khan, M.A. Akbar, M.A. Salam, Exact traveling wave solutions of modified KdV-Zakharov-Kuznetsov equation and viscous Burgers equation. SPRINGERPLUS 3, 105 (2014)

    Article  Google Scholar 

  27. K.U.H. Tariq, A.R. Seadawy, Soliton solutions of (3+1)-dimensional Korteweg-de Vries Benjamin-Bona-Mahony, Kadomtsev-Petviashvili Benjamin-Bona-Mahony and modified Korteweg de Vries-Zakharov-Kuznetsov equations and their applications in water waves. J. King Saud Univ. Sci. 31, 8–13 (2019)

    Article  Google Scholar 

  28. A.R. Seadawy, Stability analysis solutions for nonlinear three-dimensional modified Korteweg-de Vries-Zakharov-Kuznetsov equation in a magnetized electron-positron plasma. Physica A 455, 44–51 (2016)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  29. D. Lu, A.R. Seadawy, M. Arshad, J. Wang, New solitary wave solutions of (3+1)-dimensional nonlinear extended Zakharov-Kuznetsov and modified KdV-Zakharov-Kuznetsov equations and their applications. Results Phys. 7, 899–909 (2017)

    Article  ADS  Google Scholar 

  30. S. Munro, E.J. Parkes, The derivation of a modified Zakharov-Kuznetsov equation and the stability of its solutions. J. Plasma Phys. 62, 305–317 (1999)

    Article  ADS  Google Scholar 

  31. H. Schamel, A modified Korteweg-de Vries equation for ion acoustic waves due to resonant electrons. J. Plasma Phys. 9, 377–387 (1973)

    Article  ADS  Google Scholar 

  32. Y. Liu, Q. Teng, W. Tai, J. Zhou, Z. Wang, Symmetry reductions of the (3+1)-dimensional modified Zakharov-Kuznetsov equation. Adv. Diff. Equ-NY 2019, 77 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  33. S. Munro, E.J. Parkes, Stability of solitary-wave solutions to a modified Zakharov-Kuznetsov equation. J. Plasma Phys. 64, 411–426 (2000)

    Article  ADS  Google Scholar 

  34. S. Munro, E.J. Parkes, The stability of obliquely-propagating solitary-wave solutions to a modified Zakharov-Kuznetsov equation. J. Plasma Phys. 70, 543–552 (2004)

    Article  ADS  Google Scholar 

  35. A.R. Seadawy, Three-dimensional nonlinear modified Zakharov-Kuznetsov equation of ion-acoustic waves in a magnetized plasma. Comput. Math. Appl. 71, 201–212 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  36. P.J. Olver, Applications of Lie Groups to Differential Equations, 2nd edn. (Springer-Verlag, Berlin, 1993)

    Book  MATH  Google Scholar 

  37. X. Hu, Y. Li, Y. Chen, A direct algorithm of one-dimensional optimal system for the group invariant solutions. J. Math. Phys. 56, 053504 (2015)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  38. P.J. Olver, Applications of Lie Groups to Differential Equations (Springer Science & Business Media, Berlin, 2000)

    MATH  Google Scholar 

  39. L. Zhang, C.M. Khalique, Exact solitary wave and periodic wave solutions of the Kaup-Kuperschmidt equation. J. Appl. Anal. Comp. 5(3), 485–495 (2015)

    MathSciNet  MATH  Google Scholar 

  40. I.S. Gradshteyn, I.M. Ryzhik, Table of Integrals, Series, and Products, 7th edn. (Academic Press, New York, NY, USA, 2007)

    MATH  Google Scholar 

  41. N.I. Akhiezer, Elements of The Theory of Elliptic Functions (American Mathematical Soc Providence, Rhode Island, USA, 1990)

    Book  MATH  Google Scholar 

  42. J. Smoller, Shock Waves and Reaction-Diffusion Equations, vol. 258 (Springer Science & Business Media, Berlin, Germany, 2012)

    MATH  Google Scholar 

  43. C.R. Nappi, E. Witten, Wess-Zumino-Witten model based on a nonsemisimple group. Phys. Rev. Lett. 71, 3751 (1993)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  44. G.S. Adkins, C.R. Nappi, E. Witten, Static properties of nucleons in the Skyrme model. Nucl. Phys. B. 228, 552–566 (1983)

    Article  ADS  Google Scholar 

  45. E. Noether, Invariante variationsprobleme. Nachr. v. d. Ges. d. Wiss. zu Göttingen 2, 235–257 (1918)

    MATH  Google Scholar 

  46. S.C. Anco, Generalization of Noether’s Theorem in modern Form to Nonvariational Partial Differential Equations, in Recent Progress and Modern Challenges in Applied Mathematics, Modeling and Computational Science, ed. by R. Melnik, R. Makarov, J. Beglair. Fields Institute Communications, vol. 79 (Springer, New York, 2017)

    Google Scholar 

  47. W. Sarlet, Comment on ‘conservation laws of higher order nonlinear PDEs and the variational conservation laws in the class with mixed derivatives’. J. Phys. A: Math. Theor. 43, 458001 (2010)

    Article  ADS  MATH  Google Scholar 

Download references

Acknowledgements

The authors appreciate the support of North-West University. This work is partially kept up by the National Nature Science Foundation of China No.11672270.

Author information

Authors and Affiliations

  1. Department of Mathematical Sciences, International Institute for Symmetry Analysis and Mathematical Modelling, North-West University, Mafikeng Campus, Private Bag X 2046, Mmabatho, 2735, Republic of South Africa

    Oke Davies Adeyemo & Chaudry Masood Khalique

  2. College of Mathematics and Systems Science, Shandong University of Science and Technology, Qingdao, Shandong, 266590, People’s Republic of China

    Lijun Zhang

Authors
  1. Oke Davies Adeyemo
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Lijun Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Chaudry Masood Khalique
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Chaudry Masood Khalique.

Rights and permissions

Springer Nature or its licensor holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Adeyemo, O.D., Zhang, L. & Khalique, C.M. Optimal solutions of Lie subalgebra, dynamical system, travelling wave solutions and conserved currents of (3+1)-dimensional generalized Zakharov–Kuznetsov equation type I. Eur. Phys. J. Plus 137, 954 (2022). https://doi.org/10.1140/epjp/s13360-022-03100-z

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1140/epjp/s13360-022-03100-z

Search

Navigation