1 Introduction

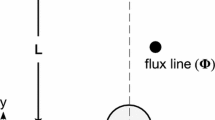
The bouncing ball is a very useful example to learn the differences and the analogies between the classical and the quantum world. In classical physics, we can observe a ball while it is bouncing many times between the two extremals \(x=0\) and \(x=h\) of its one-dimensional path, embedded in the Earth gravitational field that is approximated by a constant acceleration of gravity g. When we study the corresponding quantum system, this kind of visualization immediately disappears because, according to the Copenhagen interpretation of quantum mechanics, we cannot follow the trajectory of a quantum particle, but we can only predict the probability to find it, with a measurement, in a determined position between the two extremals. The quantum bouncing ball is a frequently studied problem in quantum physics, and in this case the solution of the Schroedinger equation is proportional to an Airy function [1,2,3]. Examining the oscillating behaviour of the probability density (Fig.  1), we can perform an ideal experiment in which a thousand particles fall one by one from a height \(x= h\) in a spatial interval \(\Delta z\) (Fig. 2). We will observe a number of alternating bands in which we could find or not find the balls, but we will not be able to describe the mechanism that has constructed the alternating strips and we will not see the balls bouncing in a continuous manner from an extremal to another. Even worse will be the picture coming from the original de Broglie–Bohm [4,5,6,7,8,9] interpretation of quantum mechanics, in which the trajectory of the particle in principle exists, but, in our particular case of a real wavefunction, the velocity of the particle is vanishing and the ball would be static in one of the coloured strips of Fig. 2, without bouncing at all.

In this paper we want to suggest a possible interpretation of the motion of the quantum particle, generalizing the expression of the probability current derived in all the textbooks from the Schroedinger equation. We will start with a parallelism between classical and quantum probability in Sect. 2, while in Sect. 3 we will define the corresponding classical and quantum velocity and we will generalize the expression of the probability current. Finally, in the last section we will discuss the obtained results.

Fig. 1
figure 1

Probability density (pink line) and classical probability (green line) for the case of a bouncing proton studied in ref. [10] (equation (32) and Fig. 1)

Full size image
Fig. 2
figure 2

The result of an ideal experiment with particles bouncing between \(x=0\) and a height \(x=h\) in a spatial interval \(\Delta z\). It is possible to observe a number of alternating bands in which the balls may or may not be found. We plot also the same probability density of Fig. 1 with a red line

Full size image

2 Classical and quantum probability density

We consider a particle of mass m that moves along a one dimensional path between two extremals. The motion is periodic with a classical period \(\tau = \sqrt{8h/g}\) (bounce period). In classical physics, the probability that the point particle can be found in the region between x and \(x+dx\) is proportional to the time the body spends in that region

$$\begin{aligned} Q(x)dx=Q(x)vdt=\frac{2dt}{\tau }. \end{aligned}$$
(1)

Hence, the classical probability density is related to the magnitude of the classical velocity field of the particle by the formula

$$\begin{aligned} Q(x)=\frac{2}{\tau v_{c}}. \end{aligned}$$
(2)

in the non-relativistic approximation. Otherwise, one can consider \(v_{c}\) as the derivative with respect to the proper time. It is well known that

$$\begin{aligned} v_{c}(x)=\sqrt{\frac{2\left( E-V(x) \right) }{m}} \end{aligned}$$
(3)

where E and V are the total energy and the potential in which the particle is embedded, respectively, so that

$$\begin{aligned} Q(x)=\frac{1}{\tau }\sqrt{\frac{2m}{E-V(x)}}. \end{aligned}$$
(4)

On the other side, the one-dimensional Schrödinger equation for stationary states is

$$\begin{aligned} -\frac{\hslash ^{2}}{2m}\frac{\partial ^{2}\psi }{\partial x^{2}}=\left( E-V\right) \psi \end{aligned}$$
(5)

and it can be rewritten in terms of Q(x) in this way

$$\begin{aligned} \frac{\partial ^{2}\psi }{\partial x^{2}}=-\left( \frac{2m}{\hslash \tau } \right) ^{2}\frac{1}{Q^{2}(x)}\psi . \end{aligned}$$
(6)

In quantum mechanics the role of Q(x) is played by the square modulus of the wave function \(\left| \psi \right| ^{2}.\) In a previous paper we have examined the case of a bouncing ball moving between \(x=0\) and \(x=h\) under the acceleration of gravity g [10]. The corresponding Schroedinger equation is (5) with a potential \(V = mgx\). Using the results and the notations contained in that paper, it is easy to show that a sort of average (that we will define later) of the quantum probability density \(\left| \psi \right| ^{2}\) is equal to the classical probability density Q(x) at least in a ‘semiclassical’ approximation. In fact, if we choose the variable

$$\begin{aligned} y=k\left( x-h\right) = k\left( x-\frac{g \tau ^2}{8}\right) \end{aligned}$$
(7)

(where \(k^3 = 2m^2 g/\hbar ^2\)) that can be expressed in terms of the height h or also of the classical bounce period \(\tau \), from the solution of the Schrödinger equation we obtained [10]

$$\begin{aligned} \left| \psi \right| ^{2}=\pi \sqrt{\frac{k}{h}}\left[ A_{i}(y)\right] ^{2} \end{aligned}$$
(8)

where \(A_{i}(y)\) is the Airy function. In the stationary phase approximation

$$\begin{aligned} \left[ A_{i}(y)\right] ^{2}\simeq \frac{\cos ^{2}\left( \frac{2}{3} \left| y\right| ^{3/2}-\frac{\pi }{4}\right) }{\pi \sqrt{y}} \end{aligned}$$
(9)

and considering that

$$\begin{aligned} Q(y)=\frac{1}{2\sqrt{y}}\sqrt{\frac{k}{h}}, \end{aligned}$$
(10)

we have

$$\begin{aligned} \left| \psi \right| ^{2}\approx 2Q(y)\cos ^{2}\left( \frac{2}{3} \left| y\right| ^{3/2}-\frac{\pi }{4}\right) \end{aligned}$$
(11)

In this expression \(I(y) = 2Q(y)\) represents the upper envelope of the oscillating function touching all its maximal values (the lower envelope being the x-axis). If we take, for each y, the half of I(y), we obtain a new function passing for all the points at half height (amplitude) of the original \(\vert \psi \vert ^{2} \). In general, we can define on a generic oscillating function f(y), an operation \(<< f^2 >>\) that calculates this kind of average Half Height Function (HHF)

$$\begin{aligned} HHF =<< [f(y)]^2>>=\frac{I(y)}{2}. \end{aligned}$$
(12)

that in our particular case gives

$$\begin{aligned}<<\left| \psi \right| ^{2}>>=Q(y). \end{aligned}$$
(13)

This result can be generalized from the particular case of the bouncing ball to a more general periodic motion in several ways [11, 12]. We can use, for example, the WKB approximation [13] and given

$$\begin{aligned} K(x)=\frac{\sqrt{2m\left( E-V(x)\right) }}{\hslash }=\frac{mv_{c}}{\hslash }= \frac{2 m }{\hslash \tau Q(x) }, \end{aligned}$$
(14)

the approximate solution to the Schrödinger equation can be written

$$\begin{aligned} \psi (x)=\frac{D}{\sqrt{K(x)}}\cos \left[ \int \limits _{0}^{x}dz K(z)+\varphi \right] \end{aligned}$$
(15)

(where D and \(\varphi \) are constants) such that

$$\begin{aligned}<<\left| \psi \right| ^{2}>>=\frac{D^{2}}{2 K(x)} \end{aligned}$$
(16)

proportional to Q(x). In the case of a bouncing ball, the WKB wave function becomes

$$\begin{aligned} \psi (x)=\frac{D}{\sqrt{k^{3/2} (h-x)^{1/2}}}\cos \left[ \frac{2}{3} (kh)^{3/2} [1 - (1- x/h)^{3/2}]+\varphi \right] \end{aligned}$$
(17)

and the details about this argument can be found in Problem 119 of ref. [3]. Therefore, the bouncing ball can be treated as a particular case of applications of WKB method. It is interesting to discuss what happens in the two classical turning points at \(x =0\) and \(x = h\), where the WKB approximation fails. The problem can be cured using Langer’s boundary conditions. This means to replace ‘the differential equation which has the WKB wavefunctions as exact solutions by another equation that agrees with the Schroedinger equation in the vicinity of the turning point and agrees with the WKB equation elsewhere’[3].

3 Classical and quantum velocity

Now, if in the transition from the classical to the quantum world, we do not change the definition of probability to find a particle in a region between x and \(x+dx\), we are induced to preserve the relation (2) even in the quantum regime and to write it this way:

$$\begin{aligned} \left| \psi \right| ^{2}=\frac{2}{\tau v_{q}} \end{aligned}$$
(18)

knowing that we will recover the classical formula (2) when we perform the average of the quantum functions because \(<<\left| \psi \right| ^{2}>>\rightarrow Q(x)\) and \(<<v_{q}>>\rightarrow v_{c}\). So, it seems that we are proposing a new definition of velocity in quantum mechanics

$$\begin{aligned} v_{q}=\frac{2}{\tau \left| \psi \right| ^{2}} \end{aligned}$$
(19)

different from the standard one

$$\begin{aligned} \overrightarrow{v}=\frac{\overrightarrow{J}}{\rho }=\frac{\hslash }{2im} \frac{\psi ^{*}\overrightarrow{\nabla }\psi -\psi \overrightarrow{\nabla }\psi ^{*}}{\psi \psi ^{*}} \end{aligned}$$
(20)

that can be found in many textbooks derived from the continuity equation

$$\begin{aligned} \frac{\partial \rho }{\partial t}+\overrightarrow{\nabla } \cdot \overrightarrow{J} =0 \end{aligned}$$
(21)

in which, for stationary states, \(\frac{\partial \rho }{\partial t} = 0\).

In the Copenhagen interpretation the concept of quantum velocity is not equal to the classical one, as emphasized by Griffiths [14] ‘Nothing we have seen so far would enable us to calculate the velocity of a particle. It’s not even clear what velocity means in quantum mechanics. If the particle doesn’t have a determinate position (prior to measurement), neither does it have a well-defined velocity’. Griffiths [14] concludes writing that ‘for our present purposes it will suffice to postulate that the expectation value of the velocity is equal to the time derivative of the expectation value of position’.

On the contrary, in the de Broglie–Bohm interpretation of quantum mechanics [4,5,6,7,8,9] trajectories have been worked out for a number of interesting cases and there is a well-defined concept of velocity given by equation (20) in which putting

$$\begin{aligned} \psi =R e^{\frac{i}{\hslash }S} \end{aligned}$$
(22)

one can obtain the so-called guiding formula

$$\begin{aligned} \overrightarrow{v}=\frac{\overrightarrow{\nabla }S}{m} \end{aligned}$$
(23)

but Bohm and Hiley [15] admit: ‘However for stationary states with real wave function we obtain \(S=const\) and \(\overrightarrow{p} = \overrightarrow{\nabla }S=0\)’.

‘Thus, for example, an electron in the s-state of the hydrogen atom is standing still somewhere in the region where the wave function is appreciable. This feature has been regarded as unsatisfactory by many physicists including Einstein’.

We do not intend to list all the efforts done by many authors to improve the original de Broglie–Bohm proposal and to solve all the related problems. We only want to underline a different point of view. If we repeat the calculations leading to the continuity equation, multiplying the Schrödinger equation for \(\psi ^{*}\) and its complex conjugate for \(\psi \) and subtract one equation from the other, we obtain:

$$\begin{aligned} i\hslash \left( \psi ^{*}\frac{\partial \psi }{\partial t}+\psi \frac{\partial \psi ^{*}}{\partial t}\right) =-\frac{\hslash ^2}{2m}\left( \psi ^{*}\frac{\partial ^2 \psi }{\partial x^2}-\psi \frac{\partial ^2 \psi ^{*}}{\partial x^2}\right) \end{aligned}$$
(24)

and then

$$\begin{aligned} i\hslash \frac{\partial (\psi ^{*}\psi )}{\partial t}=-\frac{\hslash ^2}{2m}\frac{\partial }{\partial x}\left( \psi ^{*}\frac{\partial \psi }{\partial x}-\psi \frac{\partial \psi ^{*}}{\partial x}\right) \end{aligned}$$
(25)

that leads to

$$\begin{aligned} \frac{\partial (\psi ^{*}\psi )}{\partial t}+\frac{\hslash }{2im}\frac{\partial }{\partial x}\left( \psi ^{*}\frac{\partial \psi }{\partial x}-\psi \frac{\partial \psi ^{*}}{\partial x}\right) =0 \end{aligned}$$
(26)

that is the continuity equation (21) written in one spatial dimension.

We argue that, going from the equation (24) to the equation (25), we can safely add an arbitrary function depending only on time in the parenthesis on the right-hand side of the equation (25). It means that in three dimensions we can write the probability current as

$$\begin{aligned} \overrightarrow{J}=\frac{\hslash }{2im}\left[ \psi ^{*}\overrightarrow{ \nabla }\psi -\psi \overrightarrow{\nabla }\psi ^{*}+\overrightarrow{F} \right] \end{aligned}$$
(27)

because we have the freedom to add an arbitrary vector \( \overrightarrow{F}(t)= (F_x, F_y, F_z)\). This vector must be imaginary in order to obtain a real velocity field. The consequence is that, in the case of the real wave function \(\left( \psi =\psi ^{*}\right) \), the probability current is no longer vanishing, solving the problem underlined by Bohm and Hiley.

In the one-dimensional case of the bouncing ball

$$\begin{aligned} \overrightarrow{v}_{q}=\frac{\hslash F_x }{2im\left| \psi \right| ^{2}}\hat{x} \end{aligned}$$
(28)

and we have

$$\begin{aligned} F_x = f(t) \frac{4im}{\hslash \tau } \end{aligned}$$
(29)

where \(f(t) = 1\) for \(0< t < \tau /2\) and \(f(t) = -1\) for \(\tau /2<t < \tau \).

Therefore, our equation (19) can be explained simply generalizing the standard expression of the probability current \(\overrightarrow{J}.\) Of course, it would be useful to determine the properties of the vector \( \overrightarrow{F}\) in a more general case, but this task goes beyond the aim of this paper.

In order to avoid any problems, one can choose to consider this velocity field only when the WKB approximation works, i.e. not in the neighbourhood of the turning points. Actually, we observe that the WKB solution for the bouncing ball (17) at the turning points has a good behaviour if we apply our definition of velocity (19). In fact the WKB wave function diverges to infinity in \(x = h\) in agreement with a classical velocity \(v =0\) and converges to a finite value at \(x =0\) that, choosing suitable arbitrary constants in (17), can be made coincident with the classical value \(v =\sqrt{2gh}\). But this behaviour is different with respect to the exact solution of Schroedinger equation (8), because we had required as boundary conditions \(\psi (0) = 0\) and \(\psi (\infty ) = 0\), hence if we insist to consider the velocity field near the turning points too, we must change the boundary conditions used to obtain the exact solution of the Schroedinger equation.

4 Conclusions

The definition of velocity of a quantum particle is well known in the de Broglie–Bohm interpretation of quantum mechanics [4,5,6,7,8,9, 15]. If we analyse the problem of the bouncing ball in this framework, we would obtain a not bouncing particle that may be found in a static equilibrium in some areas of the space between \(x =0\) and \(x=h\) and not found in other areas alternatively (Fig. 2). This way, the description of the motion appears very strange. We have proposed a correction to the probability current that allows to have a non-vanishing velocity of the quantum particle even when the wavefunction is real. The description of the motion of a bouncing ball in the quantum regime becomes possible relating the velocity to the square modulus of the wave function. In the values of x, where the wavefunction is vanishing, the quantum velocity tends to infinity in the non-relativistic approximation, but it is not a problem in a relativistic framework if we consider \(v_{c}\) as a derivative of the displacement with respect to the proper time. Hence the quantum particle has a well-defined trajectory, it moves along its one-dimensional vertical path going up and down between the two extremals and we have a bigger probability to find it in the coloured strips (Fig. 2), because in the white ones the ball has a great velocity and spends there only a little bit of time. But we can dare to go further and to help our visualization of the motion using an analogy similar (but not equal) to the one created by Miller [16] to explain the effects of Higgs field. A Prime Minister enters in a room full of people invited to a cocktail party. ‘As she moves she attracts the people she comes close to’ (her inertial mass increases and her velocity decreases), then ‘the ones she has left return to their even spacing’ (her inertial mass decreases and the velocity increases) and this process goes on while she crosses all the room. In our case the velocity of the particle decreases when the \(\vert \psi \vert ^2\) field has a maximum and increases when there is a minimum.