Abstract
Polarization of dispersive and dissipative dielectric media with smoothed-out inhomogeneities is studied with the goal to clarify the question of renormalizability of electromagnetic stress–energy tensor. The stress tensor is computed with the Lifshitz approach to van der Waals forces in the non-retarded limit, which accounts for dominant effects at the distances from the interface shorter than the absorption wavelength. After the substraction of the leading free space ultraviolet divergencies, there still remain two types of divergencies. First, contributions diverging in the sharp interface case become finite once it is smoothed out. Second, new subleading ultraviolet cut-off-dependent contributions appear due to the interface internal structure. The Hadamard expansion, based on the heat kernel method, is applied to systematically single out both finite and subleading contributions and to demonstrate incomplete renormalizability of the Lifshitz theory. The above approach also allows us to reveal the purely quantum mechanical nature of surface tension, which consists of finite cut-off-independent as well as cut-off-dependent contributions. The deduced theory of surface tension and its calculations for real dielectric media are favourably compared to the available experimental data. The problem of surface tension proves to be of a distinguished limit type because the sharp interface formulation loses the critical information about the internal structure of an interface. The general theory offered here is illustrated with an exactly solvable model representing a smooth transition between two different dielectric media, which relies upon a solution of the Schrödinger equation with the Scarf potential.







Similar content being viewed by others
Notes
Because in the time domain the relation between the displacement vector \({\varvec{D}}\) and the electric field vector \({\varvec{E}}\) is nonlocal, \({\varvec{D}}(t,{\varvec{x}}) = {\varvec{E}}(t,{\varvec{x}}) + \int _{0}^{\infty }{f(\tau ) {\varvec{E}}(t-\tau ,{\varvec{x}}) \, \mathrm {d}\tau }\), while in the frequency-domain it becomes \(\widehat{{\varvec{D}}}(\omega ;{\varvec{x}}) = \varepsilon (\omega ) \widehat{{\varvec{E}}}(\omega ;{\varvec{x}}), \ \varepsilon (\omega ) = 1 + \int _{0}^{\infty }{f(\tau ) \, e^{\mathrm {i}\omega \tau } \, \mathrm {d}\tau }\).
The sign convention [57, 58] used here is dictated by the need to regularize the Feynman path integral via an analytic continuation from real t to complex t with negative imaginary part. However, the opposite sign convention is often used [54], which also transforms the Lorentzian into the Euclidean metric.
Consider deformation of a small volume with fixed number of molecules as an example.
The power \(-12\) was chosen by Lennard-Jones for convenience: in reality, the actual potential may have a mixed polynomial-exponential form, cf. [67] and references therein. For example, in the case of helium atoms the repulsive interaction between them is caused by a depletion in electron density in the overlap region that descreens the nuclei from each other resulting in internuclear repulsion. In general, while the \(r^{-12}\) term is often attributed to the Pauli exclusion principle for fermions due to anti-symmetry of their wave functions resulting in an exchange interaction between identical particles (which also applies to bosons)—this interaction is different from Coulomb electrostatic repulsion and, in fact, stronger on shorter distances and ultimately responsible for the stability of matter [68, 69]—it could also originate from the Heisenberg principle of uncertainty [70].
Physically, this absorption of electrostriction to the renormalized isotropic pressure follows from the chemical potential [14, 62], which must be constant for media in equilibrium. One may think of the electrostriction stress as analogous to the gravity in the ocean compensated by the mechanical stresses in water: if it is balanced in the r-direction, then due to isotropy it must be balanced in the \(\theta \)-direction as well. Notably, the \(\theta \)-dependence of the electrostriction stress leads to non-uniform compression of the matter which is stronger near the interface. However, due to isotropy, electrostriction does not contribute to ST (Sect. 4).
Because from now on we will work mostly with the Fourier transforms of all quantities, we implicitly assume that they depend on the Matsubara frequencies \(\zeta _n\) and q, and, for simplicity, we omit these arguments in functions. Thus, in these notations we have \(\varepsilon (\mathrm {i}\zeta ;{\varvec{x}})\equiv \varepsilon ({\varvec{x}})\), \({\widehat{G}}(\zeta ;{\varvec{x}},{\varvec{x'}})\equiv {\widehat{G}}({\varvec{x}},{\varvec{x'}})\), etc. Since \(\varepsilon \) is already dimensionless, we use the same letter for the functions \(\varepsilon =\varepsilon ({\overline{z}})=\varepsilon (\mathrm {i}\zeta ;{w}{\overline{z}})\).
Recall that the pressure is the force per unit area of the wall should it be bounding the medium, while normal stress is the force per unit area acting on the medium itself.
In the case of the Scarf model, \(\gamma ^{ \text{(div) }}\) can be calculated explicitly:
$$\begin{aligned} \gamma ^{ \text{(div) }}={23\over 6 (4\pi )^{3/2} \beta \lambda _{0}w}\sum _n \frac{(\ln \varepsilon _{\infty })^2}{(\ln \varepsilon _{\infty })^2+4\pi ^2}\frac{1+\sqrt{\varepsilon _{\infty }}}{\sqrt{\varepsilon _{\infty }}}. \end{aligned}$$First, this leads to the Keesom effect [82] contributing to vdW forces due to attraction of permanent dipoles with moments \(\mathrm {p}\)—in the case of water the ratio of the Keesom to London energies of interaction \(\varphi _{K}/\varphi _{L} = 4 \mathrm {p}^{4}/(9 h_{{ \text{ B }}} T \alpha _{w}^{2} \omega _{a}) \approx 6.32\), where \(\alpha _{w}\) is the water molecular polarizability; most importantly, the hydrogen bond energy \(3.82 \cdot 10^{-20} \, \mathrm {J}\) per bond is comparable to that of the latent heat of evaporation per molecule \(6.78 \cdot 10^{-20} \, \mathrm {J}\) and much larger than \(k_{{ \text{ B }}} T \approx 4.14 \cdot 10^{-21} \, \mathrm {J}\) thus indeed being capable of affecting the value of ST. Second, the water molecule polarity may give rise to a local structure in the bulk and potentially at the interface thus, in particular, invalidating assumptions behind the isotropy of the dielectric permittivity upon which the Lifshitz theory is based—if one thinks of water molecules as permanent dipoles, they may orient themselves along the interface thus increasing ST as opposed to, say, surfactants, which, when added to water, stick their polar heads in water and thus orient themselves perpendicularly to the interface, thus decreasing ST due to repulsion between themselves.
This is typical for non-closed theories, e.g. the Navier–Stokes equations require the empirically measured viscosity coefficient.
References
I.Z. Fisher, Statistical Theory of Liquids (University of Chicago Press, Chicago, 1964)
R. Zallen, The Physics of Amorphous Solids (Wiley, New York, 2004)
J.P. Hansen, I.R. McDonald, Theory of Simple Liquids (Academic Press, Boca Raton, 2006)
J. Israelachvili, Intermolecular and Surface Forces (Academic Press, Boca Raton, 2011)
B.V. Derjaguin, M.M. Kusakov, The properties of thin layers of liquids. Proc. Acad. Sci. USSR Chem. Ser. 5, 741 (1936)
H.B.G. Casimir, D. Polder, The influence of retardation on the London–van der Waals forces. Phys. Rev. 73, 360 (1948)
H.B.G. Casimir, On the attraction between two perfectly conducting planes. Proc. K. Ned. Akad. Wet. 51, 793 (1948)
B.V. Derjaguin, A.S. Titijevskaia, I.I. Abricossova, A.D. Malkina, Investigations of the forces of interaction of surfaces in different media and their application to the problem of colloid stability. Disc. Faraday Soc. 18, 24 (1954)
B.V. Deryaguin, I.I. Abrikosova, E.M. Lifshitz, Direct measurement of molecular attraction between solids separated by a narrow gap. Q. Rev. 10, 295 (1956)
D. Tabor, R.H.S. Winterton, Direct measurement of normal and retarded van der Waals forces. Nature (London) 219, 1120 (1968)
B. Derjaguin, Untersuchungen über die Reibung und Adhäsion. IV. Kolloid Z. 69, 155 (1934)
H.C. Hamaker, The London—van der Waals attraction between spherical particles. Physica 4, 1058 (1937)
E.M. Lifshitz, The theory of molecular attractive forces between solids. Sov. Phys. JETP 2, 73 (1956)
I.E. Dzyaloshinskii, E.M. Lifshitz, L.P. Pitaevskii, General theory of Van der Waals’ forces. Sov. Phys. Uspekhi 4, 153 (1961)
M.J. Sparnaay, Measurement of attractive forces between flat plates. Physica 24, 751 (1958)
S.K. Lamoreaux, Demonstration of the Casimir force in the 0.6 to 6 \(\mu {{\rm m}}\) range. Phys. Rev. Lett. 78, 5 (1997)
R. Decca, V.A.D. López, Casimir force in micro and nano electro mechanical systems, in Casimir Physics, ed. by D. Dalvit, P. Milonni, D. Roberts, F. da Rosa (Springer, New York, 2011), pp. 287–309
K.A. Milton, S.A. Fulling, P. Parashar, P. Kalauni, T. Murphy, Stress tensor for a scalar field in a spatially varying background potential: Divergences, “renormalization,” anomalies, and Casimir forces. Phys. Rev. D 93, 085017 (2016)
L.S. Brown, G.J. Maclay, Vacuum stress between conducting plates: an image solution. Phys. Rev. 184, 1272 (1969)
D. Deutsch, P. Candelas, Boundary effects in quantum field theory. Phys. Rev. D 20, 3063 (1979)
T.G. Philbin, C. Xiong, U. Leonhardt, Casimir stress in an inhomogeneous medium. Ann. Phys. (N.Y.) 325, 579 (2010)
C. Xiong, T.W. Kelsey, S.A. Linton, U. Leonhardt, Casimir forces for inhomogeneous planar media. J. Phys: Conf. Ser. 410, 012165 (2013)
W.M.R. Simpson, S.A.R. Horsley, U. Leonhardt, Divergence of Casimir stress in inhomogeneous media. Phys. Rev. A 87, 043806 (2013)
K.A. Milton, Local and global Casimir energies: divergences, renormalization, and the coupling to gravity, in Casimir Physics, ed. by D. Dalvit, P. Milonni, D. Roberts, F. da Rosa (Springer, New York, 2011), pp. 39–95
M. Bordag, D. Hennigt, D. Robaschik, Vacuum energy in quantum field theory with external potentials concentrated on planes, 1. Phys. A: Math. Gen. 25, 4483 (1992)
P. Candelas, Vacuum energy in the presence of dielectric and conducting surfaces. Ann. Phys. 143, 241 (1982)
A.A. Actor, Scalar quantum fields confined by rectangular boundaries. Fortschr. Phys. 43, 141 (1995)
S.A. Fulling, T.E. Settlemyre, K.A. Milton, Renormalization for a scalar field in an external scalar potential. Symmetry 10, 54 (2018)
P. Parashar, K.A. Milton, Y. Li, H. Day, X. Guo, S.A. Fulling et al., Quantum electromagnetic stress tensor in an inhomogeneous medium. Phys. Rev. D 97, 125009 (2018)
D.V. Vassilevich, Heat kernel expansion: user’s manual. Phys. Rep. 388, 279 (2003)
M. Bordag, K. Kirsten, D.V. Vassilevich, Path-integral quantization of electrodynamics in dielectric media. J. Phys. A: Math. Gen. 31, 2381 (1998)
S.K. Blau, M. Visser, A. Wipf, Zeta functions and the Casimir energy. Nucl. Phys. B 310, 163 (1988)
G. ’t Hooft, M.J.G. Veltman, Regularization and renormalization of gauge fields. Nucl. Phys. B 44, 189 (1972)
F. Bao, J.S. Evans, M. Fang, S. He, Inhomogeneity-related cutoff dependence of the Casimir energy and stress. Phys. Rev. A 93, 013824 (2016)
Y. Li, K.A. Milton, X. Guo, H.L. Dodge, G. Kennedy, S.A. Fulling, Casimir forces in inhomogeneous media: Renormalization and the principle of virtual work. Phys. Rev. D 99, 125004 (2019)
S.A. Fulling, K.A. Milton, M. Bordag, Vacuum energy density and pressure near boundaries. Int. J. Mod. Phys. A 25, 2364 (2010)
S.A. Fulling, K.A. Milton, J. Wagner, Energy density and pressure in power-wall models. Int. J. Mod. Phys. A 27, 1260009 (2012)
K. Symanzik, Schrödinger representation and Casimir effect in renormalizable quantum field theory. Nucl. Phys. B 190, 1 (1981)
D.V. Vassilevich, Divergences in the vacuum energy for frequency-dependent interactions. Phys. Rev. D 79, 065016 (2009)
K.A. Milton, Hard and soft walls. Phys. Rev. D 84, 065028 (2011)
J.D. Bouas, S.A. Fulling, F.D. Mera, K. Thapa, C.S. Trendafilova, J. Wagner, Investigating the spectral geometry of a soft wall. Proc. Symp. Pure Math. 84, 139 (2012)
S.W. Murray, C.M. Whisler, S.A. Fulling, J. Wagner, H.B. Carter, D. Lujan et al., Vacuum energy density and pressure near a soft wall. Phys. Rev. D 93, 105010 (2016)
N. Graham, R.L. Jaffe, H. Weigel, Casimir effects in renormalizable quantum field theories. Int. J. Mod. Phys. A 17, 846 (2002)
N. Graham, R.L. Jaffe, V. Khemani, M. Quandt, M. Scandurra, H. Weigel, Calculating vacuum energies in renormalizable quantum field theories: a new approach to the Casimir problem. Nucl. Phys. B 645, 49 (2002)
N. Graham, R.L. Jaffe, V. Khemani, M. Quandt, M. Scandurra, H. Weigel, Casimir energies in light of quantum field theory. Phys. Lett. B 572, 196 (2003)
N. Graham, R. Jaffe, V. Khemani, M. Quandt, O. Schroeder, H. Weigel, The Dirichlet Casimir problem. Nucl. Phys. B 677, 379 (2004)
K.A. Milton, Casimir energies and pressures for delta-function potentials. J. Phys. A 37, 6391 (2004)
J.S. Høye, I. Brevik, Casimir force and its relation to surface tension. Phys. Rev. A 95, 052127 (2017)
T.-P. Hack, V. Moretti, On the stress-energy tensor of quantum fields in curved spacetimes-comparison of different regularization schemes and symmetry of the Hadamard/Seeley-DeWitt coefficients. J. Phys. A: Math. Theor. 45, 374019 (2012)
S. Hollands, R.M. Wald, Conservation of the stress tensor in perturbative interacting quantum field theory in curved spacetimes. Rev. Math. Phys. 17, 227 (2005)
B.S. DeWitt, Dynamical Theory of Groups and Fields (Gordon & Breach, New York, 1965)
C.W. Misner, K.S. Thorne, J.A. Wheeler, Gravitation (Freeman, San Francisco, 1973)
Y.S. Barash, V.L. Ginzburg, Electromagnetic fluctuations in matter and molecular (Van-der-Waals) forces between them. Sov. Phys. Uspekhi 18, 305 (1975)
E.M. Lifshitz, L.P. Pitaevskii, Statistical Physics, Part 2: Theory of the Condensed State (Pergamon, New York, 1980)
D. Fursaev, D. Vassilevich, Operators, Geometry, and Quanta (Springer, New York, 2011)
J. Schwinger, J.L.L. Deraad, K.A. Milton, Casimir effect in dielectrics. Ann. Phys. (N.Y.) 115, 1 (1978)
R.M. Wald, On the Euclidean approach to quantum field theory in curved spacetime. Commun. Math. Phys. 70, 221 (1979)
S.A. Fulling, S.N.M. Ruijsenaars, Temperature, periodicity and horizons. Phys. Rep. 152, 135 (1987)
Y.S. Barash, V.L. Ginzburg, Some problems in the theory of van der Waals forces. Sov. Phys. Uspekhi 27, 467 (1984)
J.A. Stratton, Electromagnetic Theory (Wiley, New York, 2007)
J. Schwinger, On gauge invariance and Vacuuwn polarization. Phys. Rev. 82, 664 (1951)
L.D. Landau, L.P. Pitaevskii, E.M. Lifshitz, Electrodynamics of Continuous Media (Butterworth-Heinemann, Oxford, 1984)
G.W. Gibbons, S.W. Hawking, Action integrals and partition functions in quantum gravity. Phys. Rev. D 15, 2752 (1977)
P.B. Gilkey, Invariance Theory, the Heat Equation and the Atyah-Singer Index Theorem (Chemical Rubber Company Press, Boca Raton, 1994)
K.M. Apfeldorf, H.E. Camblong, C.R. Ordóñezc, Field redefinition invariance in Quantum Field Theory. Mod. Phys. Lett. A 16, 103 (2001)
F. Ravndal, Problems with the Casimir vacuum energy, in Problems with Vacuum Energy, 8 (2000). arXiv:hep-ph/0009208
J.A. Rackers, J.W. Ponder, Classical Pauli repulsion: an anisotropic, atomic multipole model. J. Chem. Phys. 150, 084104 (2019)
F.J. Dyson, A. Lenard, Stability of matter. I. J. Math. Phys. 8, 423 (1967)
A. Lenard, F.J. Dyson, Stability of matter. II. J. Math. Phys. 9, 698 (1968)
G. Volovik, The Universe in a Helium Droplet, vol. 117 (Clarendon Press, Oxford, 2006)
K.G. Wilson, The renormalization group and critical phenomena. Rev. Mod. Phys. 55, 583 (1983)
J. Mahanty, B.W. Ninham, Dispersion Forces (Academic Press, Boca Raton, 1976)
J.D. Jackson, Classical Electrodynamics (Wiley, New York, 1998)
S.L. Adler, Qunatum theory of the dielectric constant in real solids. Phys. Rev. 126, 413 (1962)
M. Bordag, K. Kirsten, Heat kernel coefficients and divergencies of the Casimir energy for the dispersive sphere. Int. J. Mod. Phys. A 17, 813 (2002)
V. Moretti, One loop stress tensor renormalization in curved background: the relation between zeta function and point splitting approaches, and an improved point splitting procedure. J. Math. Phys. 40, 3843 (1999)
F. Scarf, New soluble energy band problem. Phys. Rev. 112, 1137 (1958)
J. Dereziński, M. Wrochna, Exactly solvable Schrödinger operators. Ann. Henri Poincaré 12, 397 (2011)
M. Abramowitz, I.A. Stegun, Handbook of Mathematical Functions: With Formulas, Graphs, and Mathematical Tables (Dover, New York, 1965)
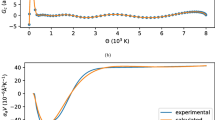
J.M. Douillard, Experimental approach of the relation between surface tension and interfacial thickness of simple liquids. J. Colloid Interface Sci. 337, 307 (2009)
K. Penanen, M. Fukuto, R.K. Heilmann, I.F. Silvera, P.S. Pershan, \(^{4}\)He liquid-vapor interface below \(1 \, {{\rm K}}\) studied using x-ray reflectivity. Phys. Rev. B 62, 9621 (2000)
W.H. Keesom, The second virial coefficient for rigid spherical molecules whose mutual attraction is equivalent to that of a quadruplet placed at its center. Proc. R. Nether. Acad. Arts Sci. 18, 636 (1915)
G. Luo, S. Malkova, S.V. Pingali, D.G. Schultz, B. Lin, M. Meron et al., Structure of the interface between two polar liquids: Nitrobenzene and water. J. Phys. Chem. Lett. B 110, 4527 (2006)
R.S. Taylor, L.X. Dang, B.C. Garrett, Molecular dynamics simulations of the liquid/vapor interface of SPC/E water. J. Phys. Chem. 100, 11720 (1996)
D. Beaglehole, Ellipsometric study of the surface of simple liquids. Physica B 100, 163 (1980)
E.S. Wu, W.W. Webb, The liquid-vapor interface of Sulfur-Hexafluoride near the critical-point. J. Phys. C1(33), 149 (1972)
F. Buff, R. Lovett, F. Stillinger, Interfacial density profile for fluids in the critical region. Phys. Rev. Lett. 15, 621 (1965)
J. Pressing, J.E. Mayer, Surface tension and interfacial density. J. Chem. Phys. 59, 2711 (1973)
D. Beysens, M. Robert, Thickness of fluid interfaces near the critical point from optical reflectivity measurements. J. Chem. Phys. 87, 3056 (1987)
J.D. van der Waals, Thermodynamische Theorie der Kapillarität unter Voraussetzung stetiger Dichteänderung. Z. Phys. Chemie 13, 657 (1894)
J.W. Cahn, J.E. Hilliard, Free energy of a nonuniform system. I. Interfacial free energy. J. Chem. Phys. 28, 258 (1958)
B. Lautrup, Physics of Continuous Matter: Exotic and Everyday Phenomena in the Macroscopic World (CRC Press, Boca Raton, 2011)
J.E. Lennard-Jones, B.M. Dent, The change in lattice spacing at a crystal boundary. Proc. R. Soc. Lond. A 121, 246 (1928)
G. Jaffé, A statistical theory of liquids. I. Phys. Rev. 62, 463 (1942)
J.G. Kirkwood, F.P. Buff, The statistical mechanical theory of surface tension. J. Chem. Phys. 17, 338 (1949)
P.D. Shoemaker, G.W. Paul, L.E. Marc de Chazal, Surface tension of simple liquids from the radial distribution function. J. Chem. Phys. 52, 491 (1970)
M.V. Berry, R.F. Durrans, R. Evans, The calculation of surface tension for simple liquids. J. Phys. A: Gen. Phys. 5, 166 (1972)
J. Walton, D.J. Tildesley, J.S. Rowlinson, J.R. Henderson, The pressure tensor at the planar surface of a liquid. Mol. Phys. Int. J. Interface Between Chem. Phys. 48, 1357 (1983)
R. Laghaei, A.E. Nasrabad, B.C. Eu, Statistical-mechanical theory of rheology: Lennard-Jones fluids. J. Chem. Phys. 123, 234507 (2005)
T. Yamaguchi, Stress-structure coupling and nonlinear rheology of Lennard-Jones liquid. J. Chem. Phys. 148, 243507 (2018)
A. Jabbarzadeh, J.D. Atkinson, R.I. Tanner, Rheological properties of thin liquid films by molecular dynamics simulations. J. Non-Newtonian Fluid Mech. 69, 169 (1997)
A. Trokhymchuk, J. Alejandre, Computer simulations of liquid/vapor interface in lennard-jones fluids: Some questions and answers. J. Chem. Phys. 111, 8510 (1999)
J. Baacke, G. Krüsemann, Perturbative analysis of the divergent contributions to the Casimir energy. Z. Phys. C Particles Fields 30, 413 (1986)
I.H. Brevik, B. Jensen, K. Milton, Comment on Casimir energy for spherical boundaries. Phys. Rev. D 64, 088701 (2001)
C. Lutken, F. Ravndal, Energy level shifts in atoms between metallic planes. Phys. Rev. A 31, 2082 (1985)
R. Shankar, Effective field theory in condensed matter physics, in Conceptual Foundations of Quantum Field Theory, ed. by T.Y. Cao (Cambridge University Press, Cambridge, 1999), pp. 47–55
P.A. Lagerstrom, Matched Asymptotic Expansions (Springer, New York, 1988)
K.A. Milton, Julian Schwinger and the Casimir effect: the reality of zero point energy. Conf. Proc. C 9809142, 20 (1999)
L. Bergström, Hamaker constants of inorganic materials. Adv. Colloid Interface Sci. 70, 125 (1997)
E. Orowan, Surface energy and surface tension in solids and liquids. Proc. R. Soc. Lond. A 316, 473 (1970)
S.M. Christensen, Vacuum expectation value of the stress tensor in an arbitrary curved background: the covariant point-separation method. Phys. Rev. D 14, 2490 (1976)
Acknowledgements
This work was partially supported by the Natural Sciences and Engineering Research Council of Canada (NSERC). A.Z. also acknowledges financial support by the Killam Trust.
Author information
Authors and Affiliations
Corresponding author
Heat kernel calculations
Heat kernel calculations
Let us define the new field
and the corresponding Green’s function
where \(\upphi ({\varvec{x}})\) is the operator corresponding to the field \(\phi ({\varvec{x}})\). Then, the inner product for this new field takes the covariant form \(\int \mathrm {d}{\varvec{x}}\, \varPhi _1 \varPhi _2=\int \mathrm {d}{\varvec{x}}\,\varepsilon ^{-3/2}\, \phi _1 \phi _2=\int \mathrm {d}{\varvec{x}}\sqrt{\mathrm {g}}\, \phi _1 \phi _2\). Equation (3) transforms to
Taking into account that
the new operator \({\mathbb {O}}\) becomes
Note that
and the covariant Laplacian in metric \(\mathrm {g}_{ij}\) reads
Therefore, we obtain the covariant form for the operator in (80)
which is Hermitian with the covariant measure \(\sqrt{\mathrm {g}}\). The potential \({\mathbb {V}}\) can also be obtained following the computations in [31, 64], which require the knowledge of the Christoffel symbols
and the connection
Heat kernel corresponding to operator \({\widehat{O}}\) is \({\widehat{K}}(s|{\varvec{x}},{\varvec{x'}})=\mathrm {g}^{1/4}({\varvec{x}})\mathrm {g}^{1/4}({\varvec{x}}'){\mathbb {K}}(s|{\varvec{x}},{\varvec{x'}})\), where
here s is the proper time, \(\sigma ({\varvec{x}},{\varvec{x}}')\) a world function defined as one half of the square of geodesic distance between points in the metric \(\mathrm {g}_{ij}\) and \(\varDelta ^{1/2}({\varvec{x}},{\varvec{x}}') \equiv \mathrm {g}^{-1/2}({\varvec{x}}) \mathfrak {D}({\varvec{x}},{\varvec{x}}') \mathrm {g}^{-1/2}({\varvec{x}}')\), where \(\mathfrak {D}({\varvec{x}},{\varvec{x}}') = -\det \left( -\sigma _{;\mu \nu '}\right) \) is the van Vleck–Morette determinant. For the computation of the heat kernel of this operator, we need to know \(\varDelta ^{1/2}({\varvec{x}},{\varvec{x}}')\), \(a_0({\varvec{x}},{\varvec{x}}')\), and \(a_1({\varvec{x}},{\varvec{x}}')\) and their derivatives. In particular they include the curvature terms:
here we used the relations
The regularized Green’s function (79), in the original notation, assumes the form
where \(\lambda _{0}\) is the cut-off parameter, and can be expanded as
Derivation of the regularized stress tensor then reduces to the following steps:
-
i)
Compute \(\varDelta ^{1/2}({\varvec{x}},{\varvec{x}}')\), \(a_0({\varvec{x}},{\varvec{x}}')\), and \(a_1({\varvec{x}},{\varvec{x}}')\);
-
ii)
Compute the second partial derivative of the Green’s function \(\partial _{{\varvec{x}}}\partial _{{\varvec{x}}'}{\widehat{G}}_{\lambda _{0}}(\zeta ;{\varvec{x}},{\varvec{x'}})\);
-
iii)
Take the limit \({\varvec{x}}={\varvec{x'}}\);
-
iv)
Integrate over the proper time s and then expand the result in the cut-off \(\lambda _{0}\);
-
v)
Compute \({\widehat{\sigma }}_{ij}(\zeta ;{\varvec{x}})\) by substituting the result to (11).
For the quantities in the limit of coincident points, we introduce notation \([\dots ]\equiv (\dots )|_{{\varvec{x}}={\varvec{x'}}}\). The expressions that we need to compute divergent parts (33) of the Green’s function \({\widehat{G}}_{\lambda _{0}}(\zeta ;{\varvec{x}},{\varvec{x'}})\) are [111]
Rights and permissions
About this article
Cite this article
Zelnikov, A., Krechetnikov, R. Scarf for Lifshitz. Eur. Phys. J. Plus 136, 755 (2021). https://doi.org/10.1140/epjp/s13360-021-01714-3
Received:
Accepted:
Published:
DOI: https://doi.org/10.1140/epjp/s13360-021-01714-3