Skip to main content
SpringerLink
Log in

Integrable nonlinear triplet lattice system with the combined inter-mode couplings

  • Regular Article
  • Published:
The European Physical Journal Plus Aims and scope Submit manuscript

Abstract

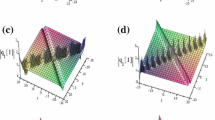
In this article we introduce the nonlinear model of three dynamical subsystems coupled both in their kinetic and potential parts. Due to the quasi-one-dimensional spatial structure of its underlying lattice, the model grasps several degrees of freedom capable to imitate the dynamical behavior of long macromolecules both natural and synthesized origins. The model admits a clear Hamiltonian formulation with the standard form of fundamental Poisson brackets and it demonstrates the complex-conjugate symmetry between two subsystems of a Toda type. The integrability of system equations is supported by their zero-curvature representation based upon the auxiliary linear problem with the relevant spectral operator of third order. In view of these facts, we have developed an appropriate twofold Darboux–Bäcklund dressing technique capable to generate the nontrivial crop solution embracing all three coupled subsystems. The explicit crop solution to the nonlinear model is found to be of a pronounced pulson character.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. L.A. Takhtadzhyan, L.D. Faddeyev, Gamil’tonov Podkhod v Teorii Solitonov (Nauka, Moskva, 1986)

    Google Scholar 

  2. L.D. Faddeev, L.A. Takhtajan, Hamiltonian Methods in the Theory of Solitons (Springer, Berlin, 1987)

    Book  Google Scholar 

  3. M.J. Ablowitz, B. Prinari, A.D. Trubatch, Discrete and Continuous Nonlinear Schrödinger Systems (Cambridge University Press, New York, 2004)

    MATH  Google Scholar 

  4. T. Tsuchida, Integrable discretizations of derivative nonlinear Schrödinger equations. J. Phys. A Math. Gen. 35(36), 7827–7847 (2002)

    Article  ADS  Google Scholar 

  5. O.O. Vakhnenko, Nonlinear integrable systems containing the canonical subsystems of distinct physical origins. Phys. Lett. A 384(3), 126081 (2020)

    Article  MathSciNet  Google Scholar 

  6. P.J. Caudrey, Differential and Discrete Spectral Problems and Their Inverses, vol. 97, North-Holland Mathematics Studies (Elsevier, Amsterdam, 1984), pp. 221–232

    MATH  Google Scholar 

  7. O.O. Vakhnenko, Inverse scattering transform for the nonlinear Schrödinger system on a zigzag-runged ladder lattice. J. Math. Phys. 51(10), 103518 (2010)

    Article  ADS  MathSciNet  Google Scholar 

  8. V.B. Matveev, Darboux transformation and the explicit solutions of differential–difference and difference–difference evolution equations. I. Lett. Math. Phys. 3(3), 217–222 (1979)

    Article  ADS  MathSciNet  Google Scholar 

  9. V.B. Matveev, M.A. Salle, Differential–difference evolution equations II. (Darboux transformation for the Toda lattice). Lett. Math. Phys. 3(5), 425–429 (1979)

    Article  ADS  MathSciNet  Google Scholar 

  10. O.O. Vakhnenko, Nonlinear integrable system of coherently coupled excitations on an intercalated ladder lattice. Eur. Phys. J. Plus 133(6), 243 (2018)

    Article  Google Scholar 

  11. V.S. Gerdjikov, On the integrability of Ablowitz–Ladik models with local and nonlocal reductions. J. Phys. Conf. Ser. 1205, 012015 (2019)

    Article  Google Scholar 

  12. O.O. Vakhnenko, Integrable nonlinear Schrödinger system on a triangular-lattice ribbon. J. Phys. Soc. Japan 84(1), 014003 (2015)

    Article  ADS  Google Scholar 

  13. B. Prinari, Discrete solitons of the focusing Ablowitz–Ladik equation with nonzero boundary conditions via inverse scattering. J. Math. Phys. 57(8), 083510 (2016)

    Article  ADS  MathSciNet  Google Scholar 

  14. G. Biondini, Q. Wang, Discrete and continuous coupled nonlinear integrable systems via the dressing method. Stud. Appl. Math. 142(2), 139–161 (2019)

    Article  MathSciNet  Google Scholar 

  15. H.-T. Wang, X.-Y. Wen, Soliton elastic interactions and dynamical analysis of a reduced integrable nonlinear Schrödinger system on a triangular-lattice ribbon. Nonlinear Dyn. 100(2), 1571–1587 (2020)

    Article  Google Scholar 

  16. P.L. Christiansen, A.V. Zolotaryuk, A.V. Savin, Solitons in an isolated helix chain. Phys. Rev. E 56(1), 877–888 (1997)

    Article  ADS  MathSciNet  Google Scholar 

  17. S.F. Mingaleev, Y.B. Gaididei, F.G. Mertens, Solitons in anharmonic chains with ultra-long-range interatomic interactions. Phys. Rev. E 61(2), R1044–R1047 (2000)

    Article  ADS  Google Scholar 

  18. V.F. Nesterenko, Dynamics of Heterogeneous Materials (Springer, New York, 2001)

    Book  Google Scholar 

  19. M. Salerno, F.K. Abdullaev, Symmetry breaking of localized discrete matter waves induced by spin–orbit coupling. Phys. Lett. A 379(37), 2252–2256 (2015)

    Article  ADS  Google Scholar 

  20. Y. Zolotaryuk, I.O. Starodub, Fluxon mobility in an array of asymmetric superconducting quantum interference devices. Phys. Rev. E 91(1), 013202 (2015)

    Article  ADS  Google Scholar 

  21. R. Driben, V.V. Konotop, T. Meier, A.V. Yulin, Bloch oscillations sustained by nonlinearity. Sci. Rep. 7, 3194 (2017)

    Article  ADS  Google Scholar 

  22. L.J. Maczewsky, K. Wang, A.A. Dovgiy, A.E. Miroshnichenko, A. Moroz, M. Ehrhardt, M. Heinrich, D.N. Christodoulides, A. Szameit, A.A. Sukhorukov, Synthesizing multi-dimensional excitation dynamics and localization transition in one-dimensional lattices. Nat. Photon. 14(2), 76–81 (2020)

    Article  ADS  Google Scholar 

  23. O.O. Vakhnenko, Three component nonlinear dynamical system generated by the new third-order discrete spectral problem. J. Phys. A Math. Gen. 36(20), 5405–5430 (2003)

    Article  ADS  MathSciNet  Google Scholar 

  24. O.O. Vakhnenko, A discrete nonlinear model of three coupled dynamical fields integrable by the Caudrey method. Ukr. J. Phys. 48(7), 653–666 (2003)

    MathSciNet  Google Scholar 

  25. V.I. Inozemtsev, The finite Toda lattices. Commun. Math. Phys. 121(4), 629–638 (1989)

    Article  ADS  MathSciNet  Google Scholar 

  26. O.O. Vakhnenko, Semidiscrete integrable nonlinear systems generated by the new fourth-order spectral operator. Local conservation laws. J. Nonlinear Math. Phys. 18(3), 401–414 (2011)

    Article  ADS  MathSciNet  Google Scholar 

  27. A.R. Chowdhury, G. Mahato, A Darboux–Bäcklund transformation associated with a discrete nonlinear Schrödinger equation. Lett. Math. Phys. 7(4), 313–317 (1983)

    Article  ADS  MathSciNet  Google Scholar 

  28. D.V. Laptev, M.M. Bogdan, Nonlinear periodic waves solutions of the nonlinear self-dual network equations. J. Math. Phys. 5(4), 042903 (2014)

    Article  ADS  MathSciNet  Google Scholar 

  29. M.M. Bogdan, D.V. Laptev, Exact description of the discrete breathers and solitons interaction in the nonlinear transmission lines. J. Phys. Soc. Japan 83(6), 064007 (2014)

    Article  ADS  Google Scholar 

Download references

Acknowledgements

The work has been supported by the National Academy of Sciences of Ukraine within the Project No 0120U100855 (Properties of low-dimensional functional materials at nano-scales). The author acknowledges the constructive criticism of the Referee having encouraged considerable amendments of the manuscript especially in Sects. 4, 10 and 11.

Author information

Authors and Affiliations

  1. Department for Theory of Nonlinear Processes in Condensed Matter, Bogolyubov Institute for Theoretical Physics, The National Academy of Sciences of Ukraine, 14-B Metrologichna Street, Kyïv, 03143, Ukraine

    Oleksiy O. Vakhnenko

Authors
  1. Oleksiy O. Vakhnenko
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Oleksiy O. Vakhnenko.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Vakhnenko, O.O. Integrable nonlinear triplet lattice system with the combined inter-mode couplings. Eur. Phys. J. Plus 135, 769 (2020). https://doi.org/10.1140/epjp/s13360-020-00794-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1140/epjp/s13360-020-00794-x

Search

Navigation