Phonon-mediated quantum discord in dark solitons

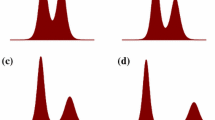
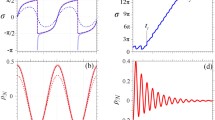
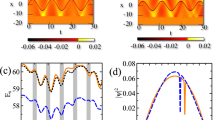
Abstract

We investigate the quantum correlation dynamics in dark-soliton qubits with a special attention to quantum discord. Recently, dark-soliton qubit exhibiting appreciably long lifetime is proved to be an excellent candidate for information processing. Depending on the precise distance between the dark-soliton qubits, the decay rate of Dicke symmetric and antisymmetric state is suppressed or enhanced. With the Renyi-2 entropy, we derive a simple analytical expression for the quantum discord and explore the generation and decay of correlation for different initial states. We believe that the present work could pave the stage for a new generation of quantum discord based purely on matter-wave phononics.

Appendices

Appendix A: Entangled state elements

The linear entropy $S_2(\rho _B)$ for an entangled state of Eq. (29) is given by:

$$\begin{aligned} S_2\left( \rho _B \right)= & {} \frac{2 \mathrm{e}^{ - 2\gamma t}}{\left( \gamma ^2 - \Gamma ^2 \right) ^2} \left[ 2\alpha \left( \gamma ^2 - \Gamma ^2 \right) ^2\left\{ 1-\delta \mathrm{e}^{ \gamma t} \right. \right. \\&+\left. \left. 2\alpha \delta \mathrm{e}^{ -\gamma t} \right\} \right. - \left. 2\alpha ^2 \left\{ \delta ^2+\left( \gamma ^2 - \Gamma ^2 \right) ^2 \mathrm{e}^{ -2\gamma t} \right\} \right] . \end{aligned}$$

The elements of the matrix L after simplification can be written as:

$$\begin{aligned} L_{11}= & {} \frac{2\alpha \gamma \Gamma Z - \alpha \left( {\gamma ^2} + {\Gamma ^2} \right) \sinh \left( {\Gamma t} \right) + \left( {\gamma ^2} - {\Gamma ^2} \right) \sqrt{\alpha \left( {1 - \alpha } \right) } }{{\sqrt{\alpha \left( {\left( {{\gamma ^2} - {\Gamma ^2}} \right) \left( {{\mathrm{e}^{\gamma t}} - \alpha {\mathrm{e}^{ - \gamma t}}} \right) - \alpha \delta } \right) \left( {\delta + \left( {{\gamma ^2} - {\Gamma ^2}} \right) {\mathrm{e}^{ - \gamma t}}} \right) } }}, \nonumber \\ L_{22}= & {} \frac{{2\alpha \gamma \Gamma Z - \alpha \left( {{\gamma ^2} + {\Gamma ^2}} \right) \sinh \left( {\Gamma t} \right) - \left( {{\gamma ^2} - {\Gamma ^2}} \right) \sqrt{\alpha \left( {1 - \alpha } \right) } }}{{\sqrt{\alpha \left( {\left( {{\gamma ^2} - {\Gamma ^2}} \right) \left( {{\mathrm{e}^{\gamma t}} - \alpha {\mathrm{e}^{ - \gamma t}}} \right) - \alpha \delta } \right) \left( {\delta + \left( {{\gamma ^2} - {\Gamma ^2}} \right) {\mathrm{e}^{ - \gamma t}}} \right) } }}, \nonumber \\ L_{33}= & {} \frac{{{{\left( {{\gamma ^2} - {\Gamma ^2}} \right) }^2}\left( {1 - \alpha {\mathrm{e}^{ - 2\gamma t}}} \right) - \left( {{\gamma ^2} - {\Gamma ^2}} \right) 2\alpha \delta {\mathrm{e}^{ - 2\gamma t}} - \alpha {\delta ^2}}}{{\left( {\left( {{\gamma ^2} - {\Gamma ^2}} \right) \left( {{\mathrm{e}^{\gamma t}} - \alpha {\mathrm{e}^{ - \gamma t}}} \right) - \alpha \delta } \right) \left( {\delta + \left( {{\gamma ^2} - {\Gamma ^2}} \right) {\mathrm{e}^{ - \gamma t}}} \right) }}. \end{aligned}$$

(A1)

In this case, the quantum discord takes the form:

$$\begin{aligned} \mathcal {Q}\left( {{t}} \right)&= \frac{{ - 2\alpha {\mathrm{e}^{ - \gamma t}}}}{{\left( {{\gamma ^2} - {\Gamma ^2}} \right) }}\left( {\delta + \left( {{\gamma ^2} - {\Gamma ^2}} \right) {\mathrm{e}^{ - \gamma t}}} \right) {\log _2}\left( {\frac{{\alpha {\mathrm{e}^{ - \gamma t}}}}{{\left( {{\gamma ^2} - {\Gamma ^2}} \right) }}\left( {\delta + \left( {{\gamma ^2} - {\Gamma ^2}} \right) {\mathrm{e}^{ - \gamma t}}} \right) } \right) \nonumber \\&\quad + \sum \limits _{i = 1}^4 {{\zeta _i}} {\log _2}\left( {{\zeta _i}} \right) - \frac{{2{\mathrm{e}^{ - \gamma t}}}}{{\left( {{\gamma ^2} - {\Gamma ^2}} \right) }}\left( {\left( {{\gamma ^2} - {\Gamma ^2}} \right) \left( {{\mathrm{e}^{\gamma t}} - \alpha {e^{ - \gamma t}}} \right) - \alpha \delta } \right) \nonumber \\&\quad \times {\log _2}\left( {\frac{{{\mathrm{e}^{ - \gamma t}}}}{{\left( {{\gamma ^2} - {\Gamma ^2}} \right) }} \left( {\left( {{\gamma ^2} - {\Gamma ^2}} \right) \left( {{e^{\gamma t}} - \alpha {\mathrm{e}^{ - \gamma t}}} \right) - \alpha \delta } \right) } \right) \nonumber \\&\quad - S_2\left( \rho _B \right) \max \left\{ {{L_{11}}^2,{L_{22}}^2,{L_{33}}^2} \right\} , \end{aligned}$$

(A2)

where the eigenvalues ${\zeta _{i}}$ of the density matrix ${{\rho _{AB}}}$ are:

$$\begin{aligned} \zeta _{1,2}= & {} \frac{{{\mathrm{e}^{ - \gamma t}}}}{{2\left( {{\gamma ^2} - {\Gamma ^2}} \right) }}\nonumber \\&\left[ \left( {{\gamma ^2} - {\Gamma ^2}} \right) {\mathrm{e}^{\gamma t}} - 2\alpha \delta \pm \sqrt{{{\left( {\left( {{\gamma ^2} - {\Gamma ^2}} \right) \left( {2\alpha {\mathrm{e}^{ - \gamma t}} - {\mathrm{e}^{\gamma t}}} \right) + 2\alpha \delta } \right) }^2} + 4\alpha \left( {1 - \alpha } \right) {{\left( {{\gamma ^2} - {\Gamma ^2}} \right) }^2}} \right] , \nonumber \\ {\zeta _3}= & {} \frac{{\left( {\gamma + \Gamma } \right) \alpha {\mathrm{e}^{ - \gamma t}}}}{{\left( {\gamma - \Gamma } \right) }}\left( {{\mathrm{e}^{ - \Gamma t}} - {\mathrm{e}^{ - \gamma t}}} \right) , \quad {\zeta _4} = \frac{{\left( {\gamma - \Gamma } \right) \alpha {\mathrm{e}^{ - \gamma t}}}}{{\left( {\gamma + \Gamma } \right) }}\left( {{\mathrm{e}^{\Gamma t}} - {\mathrm{e}^{ - \gamma t}}} \right) . \end{aligned}$$

(A3)

Appendix B: Mixed-state elements

The classical correlation for the initial mixed state is given by:

$$\begin{aligned} {\mathcal{C}_2}\left( {{t}} \right)= & {} \frac{{2{\mathrm{e}^{ - 2\gamma t}}}}{{9{{\left( {{\gamma ^2} - {\Gamma ^2}} \right) }^2}}}\left[ \begin{array}{l} 9{\left( {{\gamma ^2} - {\Gamma ^2}} \right) ^2}{\mathrm{e}^{2\gamma t}} - {\left( {\left( {{\gamma ^2} - {\Gamma ^2}} \right) \left( {\alpha {\mathrm{e}^{ - \gamma t}} + {\mathrm{e}^{ - \Gamma t}}} \right) + \alpha \delta } \right) ^2}\\ - {\left( {\left( {{\gamma ^2} - {\Gamma ^2}} \right) \left( {3{\mathrm{e}^{\gamma t}} - \alpha {\mathrm{e}^{ - \gamma t}} - {\mathrm{e}^{ - \Gamma t}}} \right) - \alpha \delta } \right) ^2} \end{array} \right] \nonumber \\&\times \max \left\{ {{L_{11}}^2,{L_{33}}^2} \right\} , \end{aligned}$$

(B1)

with

$$\begin{aligned} {L_{11}}= & {} {L_{22}} = \frac{{2\alpha \gamma \Gamma Z - \alpha \left( {{\gamma ^2} + {\Gamma ^2}} \right) \sinh \left( {\Gamma t} \right) + \left( {{\gamma ^2} - {\Gamma ^2}} \right) {\mathrm{e}^{ - \Gamma t}}}}{{\sqrt{\left( {\left( {{\gamma ^2} - {\Gamma ^2}} \right) \left( {\alpha {\mathrm{e}^{ - \gamma t}} + {\mathrm{e}^{ - \Gamma t}}} \right) + \alpha \delta } \right) \left( {\left( {{\gamma ^2} - {\Gamma ^2}} \right) \left( {3{\mathrm{e}^{\gamma t}} - \alpha {\mathrm{e}^{ - \gamma t}} - {\mathrm{e}^{ - \Gamma t}}} \right) - \alpha \delta } \right) } }}, \nonumber \\ {L_{33}}= & {} \frac{{\alpha {{\left( {{\gamma ^2} - {\Gamma ^2}} \right) }^2}\left( {3{\mathrm{e}^{\gamma t}} - \alpha {\mathrm{e}^{ - \gamma t}} - 2{e^{ - \Gamma t}}} \right) - 2{\alpha ^2}\left( {{\gamma ^2} - {\Gamma ^2}} \right) \delta - \left( {\left( {{\gamma ^2} - {\Gamma ^2}} \right) {\mathrm{e}^{ - \Gamma t}} + \alpha \delta } \right) }}{{\left( {\left( {{\gamma ^2} - {\Gamma ^2}} \right) \left( {\alpha {\mathrm{e}^{ - \gamma t}} + {\mathrm{e}^{ - \Gamma t}}} \right) + \alpha \delta } \right) \left( {\left( {{\gamma ^2} - {\Gamma ^2}} \right) \left( {3{\mathrm{e}^{\gamma t}} - \alpha {\mathrm{e}^{ - \gamma t}} - {\mathrm{e}^{ - \Gamma t}}} \right) - \alpha \delta } \right) }}.\nonumber \\ \end{aligned}$$

(B2)

The quantum discord for this state can be written as:

$$\begin{aligned} \mathcal{Q}\left( {{t}} \right)&= - \frac{{2{\mathrm{e}^{ - \gamma t}}}}{{3\left( {{\gamma ^2} - {\Gamma ^2}} \right) }}\left( {\left( {{\gamma ^2} - {\Gamma ^2}} \right) \left( {\alpha {\mathrm{e}^{ - \gamma t}} + {\mathrm{e}^{ - \Gamma t}}} \right) + \alpha \delta } \right) \nonumber \\&\quad \times {\log _2} \left[ {\frac{{{e^{ - \gamma t}}}}{{3\left( {{\gamma ^2} - {\Gamma ^2}} \right) }}\left( {\left( {{\gamma ^2} - {\Gamma ^2}} \right) \left( {\alpha {\mathrm{e}^{ - \gamma t}} + {\mathrm{e}^{ - \Gamma t}}} \right) + \alpha \delta } \right) } \right] \nonumber \\&\quad - \frac{{2{\mathrm{e}^{ - \gamma t}}}}{{3\left( {{\gamma ^2} - {\Gamma ^2}} \right) }}\left( {\left( {{\gamma ^2} - {\Gamma ^2}} \right) \left( {3{\mathrm{e}^{\gamma t}} - \alpha {\mathrm{e}^{ - \gamma t}} - {\mathrm{e}^{ - \Gamma t}}} \right) - \alpha \delta } \right) \nonumber \\&\quad \times {\log _2} \left[ {\frac{{2{\mathrm{e}^{ - \gamma t}}}}{{3\left( {{\gamma ^2} - {\Gamma ^2}} \right) }}\left( {\left( {{\gamma ^2} - {\Gamma ^2}} \right) \left( {3{\mathrm{e}^{\gamma t}} - \alpha {\mathrm{e}^{ - \gamma t}} - {\mathrm{e}^{ - \Gamma t}}} \right) - \alpha \delta } \right) } \right] \nonumber \\&\quad + \sum \limits _{i = 1}^4 {{\zeta _i}} {\log _2}\left( {{\zeta _i}} \right) - {\mathcal{C}_2}\left( {{ t}} \right) , \end{aligned}$$

(B3)

with the eigenvalues ${\zeta _{i}}$ as:

$$\begin{aligned} {\zeta _1}= & {} \frac{\alpha }{3}{\mathrm{e}^{ - 2\gamma t}}, \quad {\zeta _2} = \frac{{{\mathrm{e}^{ - \gamma t}}}}{{3\left( {{\gamma ^2} - {\Gamma ^2}} \right) }}\left( {\left( {{\gamma ^2} - {\Gamma ^2}} \right) \left( {3{\mathrm{e}^{\gamma t}} - \alpha {\mathrm{e}^{ - \gamma t}} - 2{e^{ - \Gamma t}}} \right) - 2\alpha \delta } \right) , \nonumber \\ {\zeta _3}= & {} \frac{{{\mathrm{e}^{ - \gamma t}}}}{{3\left( {{\gamma ^2} - {\Gamma ^2}} \right) }}\left( {2\left( {{\gamma ^2} - {\Gamma ^2}} \right) {\mathrm{e}^{ - \Gamma t}} + \alpha \delta + 2\gamma \alpha \Gamma Z - \alpha \left( {{\gamma ^2} + {\Gamma ^2}} \right) \sinh \left( {\Gamma t} \right) } \right) , \nonumber \\ {\zeta _4}= & {} \frac{{\alpha {\mathrm{e}^{ - \gamma t}}}}{{3\left( {{\gamma ^2} - {\Gamma ^2}} \right) }}\left( {\delta - 2\gamma \Gamma Z + \left( {{\gamma ^2} + {\Gamma ^2}} \right) \sinh \left( {\Gamma t} \right) } \right) . \end{aligned}$$

(B4)