Implications of vanishing complexity condition in $f({\textbf{R}})$ theory

Abstract

This paper introduces the concept of complexity for a static spherical spacetime and extends it to the modified $f({\textbf{R}})$ framework. The formulation of the corresponding field equations is then carried out to describe the anisotropic interior. The spherical mass function is defined in both geometric as well as matter terms. Utilizing the orthogonal splitting of the curvature tensor, specific scalars are developed, with one of them denoted as ${\mathcal {Y}}_{TF},$ identified as the complexity factor for the considered fluid setup. In addressing the system of field equations admitting some extra degrees of freedom, the complexity-free condition is introduced. In conjunction with this condition, three other constraints are applied, leading to the development of different models. We also provide a graphical representation of the resulting solutions, using specific parametric values. From this analysis, we conclude that our all three models exhibit the properties required for the existence of physically viable and stable structures for certain values of the model parameter.

1 Introduction

In modern cosmology, the widely accepted understanding is that the universe is currently undergoing accelerated expansion. This phenomenon has been detected from recent observations, including type Ia supernovae [1, 2], large-scale structures [3] and anisotropies in the cosmic microwave background (CMB) radiations [4, 5]. Such a puzzle of an accelerated expansion has prompted astrophysicists to implement one of the two primary approaches for exploration, either practicing the notion of dark energy, or extending the theory of general relativity $({\mathbb{G}\mathbb{R}}).$ In the first approach, the accelerated expansion is attributed to an enigmatic force termed ‘dark energy’, the precise nature of which still remains elusive. Various candidates for dynamical dark energy have been suggested to unreveal its mysterious nature, encompassing the cosmological constant, phantom [6, 7], tachyon [8] and Chaplygin gas models [9]. The dark energy equation of state, expressed as the ratio of pressure of the fluid distribution and its density, serves as a crucial descriptor for cosmic evolution and its ongoing rapid expansion.

The alternative approach involves modifying ${\mathbb{G}\mathbb{R}},$ giving rise to what is known as modified theories. Among these modifications, the $f({\textbf{R}})$ theory stands out as the simplest and most direct extension of ${\mathbb{G}\mathbb{R}}.$ In this theory, the curvature invariants (higher-order) are presented using different combinations of the Ricci scalar ${\textbf{R}}.$ Nojiri and Odintsov [10] developed a generalized formulation for rebuilding this modified gravity for the Friedmann–Lemaître–Robertson–Walker metric. Capozziello et al. [11] contributed to this field by examining the analytic solutions of different cosmic models within the $f({\textbf{R}})$ scenario. Nojiri and Odintsov [12] demonstrated this theory as a suitable candidate that can help in unifying inflationary era with the late-time accelerated phase of our universe. In a comprehensive review of $f({\textbf{R}})$ models, Felice and Tsujikawa [13] delved into various topics, encompassing dark energy, inflation, cosmological perturbations and spherical solutions in weak as well as strong gravity backgrounds. Said and Adami [14] proposed the generalization of an uncertainty principle for a black hole structure in the presence of charge. Further, Tripathy and Mishra [15] have elucidated the dynamics of anisotropic Bianchi type-I models, providing solutions to the corresponding equations of motion. Recently, Agrawal et al. [16] explored the same cosmological model in this modified gravity with a perfect fluid configuration and used different forms of the curvature scalar to discuss the profile of gravitational baryogenesis. Some other important works are [17,18,19].

The system of field equations within any theory of gravity serves to comprehensively depict a self-gravitating structure. The quest for solutions to these equations has become challenging, primarily due to the intricate involvement of higher-order derivatives of geometric quantities. Such solutions can be determined either analytically or numerically, the latter contingent upon the provision of initial or boundary conditions compatible with the specific scenario under consideration. However, to arrive at a solution, supplementary information concerning local physics is indispensable. For instance, recent investigations into compact objects reveal the potential existence of multiple factors that affect the isotropic/anisotropic nature of these structures [20,21,22]. Additionally, other physical components, such as inhomogeneous density, shear and dissipation flux, play pivotal roles in destabilizing the condition of pressure isotropy [23].

In this perspective, three independent components of the field equations exist for the anisotropic spherical structure, involving five unknowns (metric components and matter triplet). To obtain their solution, it is imperative to introduce two additional conditions, typically in the form of an empirical assumption involving a physical parameter or an equation of state [24, 25]. Notably, the utilization of a polytropic equation [26, 27] has been a common choice by various authors for exploring the physical characteristics of objects like white dwarfs [28]. This investigation has been extended to anisotropic systems within the framework of ${\mathbb{G}\mathbb{R}}$ [29,30,31,32,33]. In parallel, constraints have been imposed on the metric potentials, exemplified by the Karmarkar condition, where one function is arbitrarily picked to produce the complete solution [34,35,36,37,38,39,40]. Another alternative is the imposition of a conformally flat geometry, resulting in the disappearance of the Weyl tensor [41]. A particular shape function has also been used to discuss the wormhole solutions [42]. The comprehensive analysis indicates that the structural evolution within a self-gravitating body can be portrayed by various contexts, each governed by distinct constraints.

The concept of complexity within celestial systems has emerged as an extremely relevant and intriguing topic in modern research. The impetus behind exploring this concept lies in the quest to comprehend the properties of compact objects, where gravitational forces interact with the collective particles’ dynamics. These systems encompass a broad spectrum, ranging from astronomical phenomena like star clusters and galaxies to cosmic structures such as the large-scale fluid distribution in the universe. Complexity in celestial systems arises from various factors, including non-linear dynamics, where a small alteration in reigning parameters can lead to considerable differences in structural development, as well as emergent phenomena. The term complexity denotes the presence of several elements in the interior fluid distribution, contributing to its intricate nature. These elements may include density, pressure, heat flux, and more. Researchers have endeavored to formulate a comprehensive definition of complexity applicable across scientific fields. Initially, complexity was defined in terms of information and entropy within the system under consideration [43,44,45]. However, this definition faced challenges when applied to two distinct physical structures, namely an ideal gas and a perfect crystal, as their properties are inherently opposite, yet both systems exhibit no complexity.

A recently proposed and widely accepted definition of complexity comes from Herrera [46]. In his work, he defined this in relation with the physical parameters such as inhomogeneous energy density and pressure anisotropy. Drawing inspiration from Bel’s orthogonal decomposition of the Riemann tensor [47, 48], Herrera determined some scalars. Notably, he identified that the two above-mentioned factors are encapsulated in a single scalar, denoted as ${\mathcal {Y}}_{TF},$ and labeled it as the complexity factor. This concept is built on the premise that the complexity within a compact object diminishes in two scenarios: when the interior configuration is both isotropic and homogeneous, or when the effects of anisotropy in pressure and inhomogeneity in energy density are mutually canceled out. This phenomenon has been extended to explore the evolution patterns of dynamical and axially symmetric geometries through the lens of the complexity factor [49, 50] along with a detailed analysis for a spherical structure admitting static geometry [51,52,53].

Sharif and his co-researchers extended this description to charged spherical systems in ${\mathbb{G}\mathbb{R}}$ and modified minimally coupled framework, demonstrating that ${\mathcal {Y}}_{TF}$ serves as a complexity factor regardless of these frameworks [54,55,56]. Abbas and Nazar [57] explored some new solutions in the context of $f({\textbf{R}})$ theory, analyzing the impact of modified correction terms on the complexity and spherical mass function. They further discussed various compact stars using the complexity-free constraint and the Krori–Barua ansatz in a fluid-geometry coupled scenario, yielding satisfactory results [58]. In a study conducted by Manzoor and Shahid [59], the variation in the evolution of a compact object $4U1820-30$ has been investigated using the Starobinsky model. They revealed that the ratio between the density of dark and usual matter is greater than 1 for certain parametric values. This work has been generalized in modified scenarios from which noteworthy results were obtained [60,61,62,63,64,65,66,67].

This study expands upon the previously developed anisotropic models [68] in the context of $f({\textbf{R}})$ theory. The structure of this paper is organized as follows. In Sect. 2, we present the anisotropic ${\mathbb {EMT}}$ and formulate the field equations for the spherical interior distribution. We also determine the junction conditions at the spherical junction through matching the interior spacetime with the suitable exterior geometry. Section 3 introduces the structure scalars, among which ${\mathcal {Y}}_{TF}$ is selected as the complexity factor for the current scenario. A concise summary of essential conditions for a physically realistic model is outlined in Sect. 4. Furthermore, Sect. 5 presents three distinct models, accompanied by their graphical interpretation. Finally, our results are summarized in the last section.

2 $f({\textbf{R}})$ field equations and static sphere

The substitution of a generic functional of the curvature scalar in place of ${\textbf{R}}$ in the Einstein–Hilbert action provides the following form

(1)

where is the Lagrangian density that determines the role of fluid content on the self-gravitating geometry. Varying the action (1) with respect to the metric tensor, we are left with the general field equations in this modified theory as

$$\begin{aligned} {\textbf{G}}_{\delta \zeta }=8\pi {\textbf{T}}_{\delta \zeta }^{(total)}. \end{aligned}$$

(2)

Here, we define the Einstein tensor as ${\textbf{G}}_{\delta \zeta }={\textbf{R}}_{\delta \zeta }- \frac{1}{2}{\textbf{R}}g_{\delta \zeta },$ characterizing the spacetime geometry and ${\textbf{T}}_{\delta \zeta }^{(total)}$ being the total ${\textbf{EMT}}.$ The latter quantity can be classified as

$$\begin{aligned} {\textbf{T}}_{\delta \zeta }^{(total)}=\frac{1}{f_{{\textbf{R}}}} {\textbf{T}}_{\delta \zeta }^{(m)}+{\textbf{T}}_{\delta \zeta }^{(modified)}, \end{aligned}$$

(3)

where ${\textbf{T}}_{\delta \zeta }^{(m)}$ denotes the usual fluid distribution in the interior of a compact object and further shall be discussed latter. However, the term ${\textbf{T}}_{\delta \zeta }^{(modified)}$ is appeared in the above equation due to modifying the action. This entity is expressed by

$$\begin{aligned} {\textbf{T}}_{\delta \zeta }^{(modified)}{} & {} =\frac{1}{8\pi f_{{\textbf{R}}}}\nonumber \\{} & {} \quad \bigg [\frac{{\textbf{R}}g_{\delta \zeta }}{2} \bigg (\frac{f}{{\textbf{R}}}-f_{{\textbf{R}}}\bigg )+(\nabla _{\delta }\nabla _{\zeta }-g_{\delta \zeta }\Box )f_{{\textbf{R}}}\bigg ], \nonumber \\ \end{aligned}$$

(4)

where $f_{{\textbf{R}}}=\frac{\partial f({\textbf{R}})}{\partial {\textbf{R}}}.$ Some other mathematical quantities must be defined here to calculate the field equations. They are

• $\nabla _\delta$ indicates the covariant derivative,

• $\Box \equiv \frac{1}{\sqrt{-g}}\partial _\delta \big (\sqrt{-g}g^{\delta \zeta }\partial _{\zeta }\big )$ symbolizes the d’Alembert operator.

Combining Eqs. (2)–(4), we get the general field equations given by

$$\begin{aligned} {\textbf{G}}_{\delta \zeta }{} & {} =\frac{1}{f_{{\textbf{R}}}} \bigg [8\pi {\textbf{T}}_{\delta \zeta }^{(m)}+\frac{{\textbf{R}}g_{\delta \zeta }}{2} \bigg (\frac{f}{{\textbf{R}}}-f_{{\textbf{R}}}\bigg ) \nonumber \\{} & {} \quad +(\nabla _{\delta }\nabla _{\zeta }-g_{\delta \zeta }\Box )f_{{\textbf{R}}}\bigg ]. \end{aligned}$$

(5)

Since the trace of the Einstein tensor is null, we get after multiplying $g^{\delta \zeta }$ on both sides of the above equation as

$$\begin{aligned} 3\nabla ^{\delta }\nabla _{\delta }f_{\textbf{R}}+2{\textbf{R}}f_{\textbf{R}} -8\pi {\textbf{T}}^{(m)}-2f=0. \end{aligned}$$

It is interesting to note that the nature of the total ${\textbf{EMT}}$ in this theory remains conserved as same as in the ${\mathbb{G}\mathbb{R}}$ case.

We adopt a spherical geometry whose interior is distinguished by the outer region through the hypersurface $\Sigma .$ The metric representing the inner distribution is given by

$$\begin{aligned} ds^2=e^{\eta _1} dt^2-e^{\eta _2} dr^2-r^2\left( d\theta ^2+\sin ^2\theta d\phi ^2\right) , \end{aligned}$$

(6)

where $\eta _1=\eta _1(r)$ and $\eta _2=\eta _2(r).$ Since we are aimed at investigating the complexity within a self-gravitating system, the anisotropic fluid content is assumed and expressed by the following ${\textbf{EMT}}$

$$\begin{aligned} {\textbf{T}}_{\delta \zeta }^{(m)}=(\rho +p_t){\mathcal {K}}_{\delta }{\mathcal {K}}_{\zeta }-p_t g_{\delta \zeta }+\left( p_r-p_t\right) {\mathcal {W}}_\delta {\mathcal {W}}_\zeta , \end{aligned}$$

(7)

where $\rho$ being the energy density, $p_r$ is the radial and $p_t$ is the tangential component of the fluid pressure, ${\mathcal {K}}_{\delta }$ denotes the four-velocity and ${\mathcal {W}}_{\delta }$ indicates the four-vector. The expressions for the last two quantities become in terms of the metric (6) as

$$\begin{aligned} {\mathcal {K}}^\delta =(e^{\frac{-\eta _1}{2}},0,0,0), \quad {\mathcal {W}}^\delta =(0,e^{\frac{-\eta _2}{2}},0,0), \end{aligned}$$

(8)

following some particular relations such as ${\mathcal {W}}^\delta {\mathcal {K}}_{\delta }=0,~{\mathcal {K}}^\delta {\mathcal {K}}_{\delta }=1$ and ${\mathcal {W}}^\delta {\mathcal {W}}_{\delta }=-1.$ Combining Eqs. (5)–(7) yield non-zero components of the field equations as

$$\begin{aligned} 8\pi \rho&=f_{\textbf{R}}\bigg \{e^{-\eta _2}\bigg (\frac{\eta _2'}{r} -\frac{1}{r^2}\bigg )+\frac{1}{r^2}\bigg \} -\frac{1}{2}\big (f-{\textbf{R}}f_{\textbf{R}}\big )\nonumber \\&\quad -e^{-\eta _2} \bigg (f_{\textbf{R}}''+\frac{2f_{\textbf{R}}'}{r}-\frac{\eta _2'f_{\textbf{R}}'}{2}\bigg ), \end{aligned}$$

(9)

$$\begin{aligned} 8\pi {p_r}&=f_{\textbf{R}}\bigg \{e^{-\eta _2}\bigg (\frac{1}{r^2} +\frac{\eta _1'}{r}\bigg )-\frac{1}{r^2}\bigg \}+\frac{1}{2}\big (f-{\textbf{R}}f_{\textbf{R}}\big )\nonumber \\&\quad +e^{-\eta _2}\bigg (\frac{2f_{\textbf{R}}'}{r}+\frac{\eta _1'f_{\textbf{R}}'}{2}\bigg ), \end{aligned}$$

(10)

$$\begin{aligned} 8\pi {p_t}&=\frac{f_{\textbf{R}}e^{-\eta _2}}{4}\bigg (\eta _1'^2-\eta _2'\eta _1'+2\eta _1''-\frac{2\eta _2'}{r} +\frac{2\eta _1'}{r}\bigg )\nonumber \\&\quad +\frac{1}{2}\big (f-{\textbf{R}}f_{\textbf{R}}\big )\nonumber \\&\quad +e^{-\eta _2}\bigg (f_{\textbf{R}}''+\frac{2f_{\textbf{R}}'}{r}- \frac{\eta _2'f_{\textbf{R}}'}{2}+\frac{\eta _1'f_{\textbf{R}}'}{2}\bigg ), \end{aligned}$$

(11)

where $'$ means $\frac{\partial }{\partial r}.$ Moreover, from the conservation equation $\nabla _\delta {\textbf{T}}^{(m)\delta \zeta }=0,$ we get the following expression that is valid in Jordan frame (see [69] for a detailed explanation) as

$$\begin{aligned} \frac{dp_r}{dr}+\frac{\eta _1'}{2}\big (\rho +p_r\big )+\frac{2\Pi }{r}=0, \end{aligned}$$

(12)

where $\Pi =p_r-p_t$ is the anisotropic factor. Equation (12) is referred to the generalized Tolman–Oppenheimer–Volkoff $({{\textbf{TOV}}})$ for the anisotropic matter setup [70].

In order to make our findings expressive, we must choose a modified model among ones suggested in the literature. Many early universe inflation models rely on scalar fields present in supergravity and superstring theories. However, Starobinsky proposed a different inflation model [71]. This scenario, unlike models like ‘old inflation’ [72, 73], avoids the graceful exit problem. Additionally, it predicts the spectra of gravitational waves which are almost scale-invariant and also observes anisotropy in the temperature, found to be align with CMB observations [74, 75]. The Starobinsky model is defined as

$$\begin{aligned} f({\textbf{R}})={\textbf{R}}+\alpha {\textbf{R}}^2, \end{aligned}$$

(13)

where $\alpha$ being a positive constant and $f_{\textbf{RR}} > 0.$ The second term of the above model involving second-order Ricci scalar well explains the cosmic exponentially expanding phase. After a comprehensive analysis of this model in the context of compact stars, researchers deduced that the physically relevant results can be obtained only for $\alpha \in (0,6)$ [76].

Defining the mass function of a static sphere using Misner–Sharp notion, we express as follows

$$\begin{aligned} m(r)=\frac{r}{2}\big (1-e^{-\eta _2}\big ), \end{aligned}$$

(14)

whose alternative expression in the form of modified energy density (9) is

$$\begin{aligned} m(r)=4\pi \int _0^r\frac{\rho \bar{r}^2}{f_{{\textbf{R}}}}d\bar{r}. \end{aligned}$$

(15)

The term $\eta _1'$ used in Eq. (12) can be determined by joining (10) and (14). This takes the form

$$\begin{aligned} \eta _1'&=\frac{1}{r\big (r-2m\big )}\bigg [2\bigg (m+\frac{4\pi p_rr^3}{f_{\textbf{R}}}\bigg )-\frac{r^3}{f_{\textbf{R}}}\nonumber \\&\quad \times \bigg \{\frac{1}{2}\big (f-{\textbf{R}}f_{\textbf{R}}\big ) +e^{-\eta _2}\bigg (\frac{2f_{\textbf{R}}'}{r}+\frac{\eta _1'f_{\textbf{R}}'}{2} \bigg )\bigg \}\bigg ]. \end{aligned}$$

(16)

Incorporating the above value in Eq. (12) leads to

$$\begin{aligned}&\frac{dp_r}{dr}{+}\frac{1}{2}\big (\rho +p_r\big ) \bigg [\frac{2}{r\big (r-2m\big )}\bigg (m{+}\frac{4\pi p_rr^3}{f_{\textbf{R}}}\bigg ) {-}\frac{r^3}{rf_{\textbf{R}}\big (r{-}2m\big )}\nonumber \\&\qquad \times \bigg \{\frac{1}{2}\big (f-{\textbf{R}}f_{\textbf{R}}\big ) +e^{-\eta _2}\bigg (\frac{2f_{\textbf{R}}'}{r} + \frac{\eta _1'f_{\textbf{R}}'}{2}\bigg )\bigg \}\bigg ]+\frac{2\Pi }{r}=0. \end{aligned}$$

(17)

The junction conditions, which involve smoothly matching the interior and exterior metrics at the surface boundary, can be formulated to determine a complete solution which helps in understanding structural evolution in a well manner. For the outer geometry, we consider the Schwarzschild metric given by

$$\begin{aligned} ds^2= & {} \bigg (1-\frac{2{\mathcal {M}}}{r}\bigg )dt^2-\bigg (1-\frac{2{\mathcal {M}}}{r} \bigg )^{-1}dr^2\nonumber \\{} & {} -r^2\big (d\theta ^2+\sin ^2\theta d\phi ^2\big ). \end{aligned}$$

(18)

Smooth matching of these metrics could not be possible without taking the following relations into account at $r=r_\Sigma ={\mathcal {R}}.$ They are obtained from the two fundamental forms of junction conditions given by

$$\begin{aligned} e^{\eta _1}~{_=^\Sigma }~1-\frac{2{\mathcal {M}}}{{\mathcal {R}}}~{_=^\Sigma }~e^{-\eta _2}, \quad p_r~{_=^\Sigma }~0. \end{aligned}$$

(19)

The first two of the above equations just equate the temporal/radial metric components of both outer and inner spacetimes, while the last one guarantees no radial pressure at the boundary.

3 Structure scalars

The notion of complexity in self-gravitating systems has become a focal point in astrophysics. Numerous definitions of complexity exist in the literature, and one proposal suggests that a structure with an isotropic and homogeneous distribution should have zero complexity. This implies that a complexity factor essentially quantifies the relationship between inhomogeneous energy density and pressure anisotropy. Herrera [46] introduced a definition based on these factors by orthogonally splitting the Riemann tensor [47, 48] and selecting one of them as the complexity factor. This section provides a brief overview of the procedure for obtaining the complexity factor, which is linked to above-mentioned physical parameters.

The following equation splits the Riemann tensor ${\textbf{R}}^{\zeta \varphi }_{\delta \vartheta }$ given by

$$\begin{aligned} {\textbf{R}}^{\zeta \varphi }_{\delta \vartheta }={\textbf{C}}^{\zeta \varphi }_{\delta \vartheta }+16\pi {\textbf{T}}^{[\zeta }_{[\delta }\delta ^{\varphi ]}_{\vartheta ]}+8\pi {\textbf{T}}\left( \frac{1}{3}\delta ^{\zeta }_{[\delta }\delta ^{\varphi }_{\vartheta ]} -\delta ^{[\zeta }_{[\delta }\delta ^{\varphi ]}_{\vartheta ]}\right) , \end{aligned}$$

(20)

where ${\textbf{C}}^{\zeta \varphi }_{\delta \vartheta }$ symbolizes the Weyl tensor. Further, we define the two tensors as follows

$$\begin{aligned} {\mathcal {Y}}_{\zeta \delta }= & {} {\textbf{R}}_{\zeta \varphi \delta \vartheta } {\mathcal {K}}^{\varphi }{\mathcal {K}}^{\vartheta }, \end{aligned}$$

(21)

$$\begin{aligned} {\mathcal {X}}_{\zeta \delta }= & {} ^{*} {\textbf{R}}^{*}_{\zeta \varphi \delta \vartheta } {\mathcal {K}}^{\varphi }{\mathcal {K}}^{\vartheta } =\frac{1}{2}\eta ^{\omega \sigma }_{\zeta \varphi } {\textbf{R}}^{*}_{\omega \sigma \delta \vartheta } {\mathcal {K}}^{\varphi }{\mathcal {K}}^{\vartheta }, \end{aligned}$$

(22)

where $\eta ^{\omega \sigma }_{\zeta \varphi }$ being the Levi-Civita symbol and ${\textbf{R}}^{*}_{\zeta \varphi \delta \vartheta } =\frac{1}{2}\eta _{\omega \sigma \delta \vartheta }{\textbf{R}}^{\omega \sigma }_{\zeta \varphi }.$ Equations (21) and (22) can also be expressed in an alternate form as

$$\begin{aligned} {\mathcal {Y}}_{\zeta \delta }= & {} \frac{1}{3}\big \{h_{\zeta \delta } {\mathcal {Y}}_{T}+\big (3{\mathcal {W}}_{\zeta }{\mathcal {W}}_{\delta } -h_{\zeta \delta }\big ){\mathcal {Y}}_{TF}\big \}, \end{aligned}$$

(23)

$$\begin{aligned} {\mathcal {X}}_{\zeta \delta }= & {} \frac{1}{3} \big \{h_{\zeta \delta }{\mathcal {X}}_{T}+\big (3{\mathcal {W}}_{\zeta }{\mathcal {W}}_{\delta } -h_{\zeta \delta }\big ){\mathcal {X}}_{TF}\big \}. \end{aligned}$$

(24)

Here, $h_{\zeta \delta }={\mathcal {K}}_{\zeta }{\mathcal {K}}_{\delta }+g_{\zeta \delta }$ is named as the projection tensor. Performing some straightforward but lengthy calculations with Eqs. (20)–(24), we get four scalars as

$$\begin{aligned}{} & {} {\mathcal {X}}_{T}=8\pi \rho , \end{aligned}$$

(25)

$$\begin{aligned}{} & {} {\mathcal {X}}_{TF}=-{\textbf{E}}+4\pi \Pi , \end{aligned}$$

(26)

$$\begin{aligned}{} & {} {\mathcal {Y}}_{T}=4\pi \big (\rho +3p_r-2\Pi \big ), \end{aligned}$$

(27)

$$\begin{aligned}{} & {} {\mathcal {Y}}_{TF}={\textbf{E}}+4\pi \Pi . \end{aligned}$$

(28)

All physical factors in the above scalars are known except ${\textbf{E}},$ indicating the electric part of the Weyl tensor and given by

$$\begin{aligned} {\textbf{E}}{=}{-}\frac{e^{{-}\eta _2}}{4}\left[ \eta _1''{+} \frac{\eta _1'^2-\eta _2'\eta _1'}{2}{-}\frac{\eta _1'-\eta _2'}{r}{+}\frac{2(1{-}e^{\eta _2})}{r^2}\right] . \nonumber \\ \end{aligned}$$

(29)

We observe these scalar functions are to be linked with physical characteristics of a celestial structure, encompassing homogeneous/inhomogeneous density and anisotropy. Equations (25)–(28) contribute in understanding the evolutionary profile of a self-gravitating structure in the following way.

• ${\mathcal {X}}_{T}$ explains how the homogeneous energy density affects the fluid distribution.

• ${\mathcal {X}}_{TF}$ determines the inhomogeneity in the density.

• the local anisotropy is controlled by the factor ${\mathcal {Y}}_{T}.$

• ${\mathcal {Y}}_{TF}$ plays the role of both ${\mathcal {X}}_{TF}$ and ${\mathcal {Y}}_{T}.$

Our objective is to select the complexity factor from the above four scalars. The form of ${\mathcal {Y}}_{TF}$ in Eq. (28) demonstrates that this factor alone incorporates all the necessary parameters, expressed as

$$\begin{aligned} {\mathcal {Y}}_{TF}&=4\pi \Pi \left( 1+\frac{1}{f_{{\textbf{R}}}}\right) -\frac{4\pi }{r^3}\int _0^r\frac{\bar{r}^3}{f_{{\textbf{R}}}} \left( \rho '-\frac{\rho {f}_{{\textbf{R}}}'}{f_{{\textbf{R}}}}\right) d\bar{r}\nonumber \\&\quad -\frac{1}{2f_{{\textbf{R}}}}\left\{ \frac{1}{2}\left( f -{\textbf{R}}f_{{\textbf{R}}}\right) +\frac{2f_{{\textbf{R}}}'}{re^{\eta _2}}\right\} . \end{aligned}$$

(30)

It must be noted that this factor becomes null when assuming an isotropic and homogeneous matter content. Further, the formula for Tolman mass has been recently proposed, helping in providing an estimate of the total mass energy of a structure without guaranteeing its localization [77]. We now express this mass function within the modified $f({\textbf{R}})$ theory as follows

$$\begin{aligned} m_T=4\pi \int _0^r\frac{\bar{r}^2e^{(\eta _1+\eta _2)/2}}{f_{{\textbf{R}}}} ({\textbf{T}}^0_0-{\textbf{T}}^1_1-2{\textbf{T}}^2_2)d\bar{r}, \end{aligned}$$

(31)

that becomes after some necessary calculations as

$$\begin{aligned} m_T&=(m_T)_\Sigma \bigg (\frac{r}{{\mathcal {R}}}\bigg )^3\nonumber \\&\quad -r^3\int _r^{\mathcal {R}}e^{(\eta _1+\eta _2)/2} \bigg [\frac{4\pi }{r^4}\int _0^r\frac{\bar{r}^3}{f_{{\textbf{R}}}} \left( \rho '-\frac{\rho {f}_{{\textbf{R}}}'}{f_{{\textbf{R}}}}\right) d\bar{r}\nonumber \\&\quad -\frac{8\pi \Pi }{\bar{r}f_{{\textbf{R}}}} +\frac{1}{2\bar{r}f_{{\textbf{R}}}}\left\{ \frac{1}{2} \left( f-{\textbf{R}}f_{{\textbf{R}}}\right) +\frac{2f_{{\textbf{R}}}'}{\bar{r}e^{\eta _2}}\right\} + \frac{1}{\bar{r}^2f_{{\textbf{R}}}}\nonumber \\&\quad \times \left\{ \frac{1}{2}\left( f-{\textbf{R}}f_{{\textbf{R}}}\right) +\frac{f_{{\textbf{R}}}''}{2e^{\eta _2}}-\frac{\eta _2'f_{{\textbf{R}}}'}{4e^{\eta _2}}\nonumber \right. \\&\left. \quad +\frac{3\eta _1'f_{{\textbf{R}}}'}{4e^{\eta _2}}+ \frac{2'f_{{\textbf{R}}}'}{\bar{r}e^{\eta _2}}\right\} \bigg ]d\bar{r}. \end{aligned}$$

(32)

This highlights the significant implications arising from the second integral, delineating the influence of fluid parameters on the Tolman mass. Equation (32) further takes the form when combining with the scalar (30) as follows

$$\begin{aligned} m_T&=(m_T)_\Sigma \bigg (\frac{r}{{\mathcal {R}}}\bigg )^3+r^3 \int _r^{\mathcal {R}}\frac{e^{(\eta _1+\eta _2)/2}}{\bar{r}}\nonumber \\&\quad \times \bigg [\frac{{\mathcal {Y}}_{TF}}{f_{{\textbf{R}}}} -{\textbf{E}}\left( 1-\frac{1}{f_{{\textbf{R}}}}\right) \nonumber \\&\quad +\frac{1}{2f_{{\textbf{R}}}e^{\eta _2}}\left( f_{{\textbf{R}}}'' -\frac{\eta _2'f_{{\textbf{R}}}'}{2}\right) -\frac{1}{\bar{r}f_{{\textbf{R}}}}\nonumber \\&\quad \times \left\{ \frac{1}{2}\left( f-{\textbf{R}}f_{{\textbf{R}}}\right) +\frac{f_{{\textbf{R}}}''}{2e^{\eta _2}}-\frac{\eta _2'f_{{\textbf{R}}}'}{4e^{\eta _2}}\nonumber \right. \\&\left. \quad +\frac{3\eta _1'f_{{\textbf{R}}}'}{4e^{\eta _2}}+ \frac{2'f_{{\textbf{R}}}'}{\bar{r}e^{\eta _2}}\right\} \bigg ]d\bar{r}, \end{aligned}$$

(33)

which relates the complexity factor with the Tolman mass.

It is crucial to emphasize that the system with null complexity is not solely characterized by an isotropic and homogeneous configuration. In fact, Eq. (30) also explains such a structure $({\mathcal {Y}}_{TF}=0)$ under the following constraint

$$\begin{aligned} \Pi= & {} \frac{f_{{\textbf{R}}}}{r^3(f_{{\textbf{R}}}+1)} \int _0^r\frac{\bar{r}^3}{f_{{\textbf{R}}}} \left( \rho '-\frac{\rho {f}_{{\textbf{R}}}'}{f_{{\textbf{R}}}}\right) d\bar{r}\nonumber \\{} & {} +\frac{1}{8\pi (f_{\mathbf {{\textbf{R}}}}+1)}\left\{ \frac{1}{2} \left( f-{\textbf{R}}f_{{\textbf{R}}}\right) +\frac{2f_{{\textbf{R}}}'}{re^{\eta _2}}\right\} , \end{aligned}$$

(34)

implying the existence of a large number of solutions that satisfy it [68]. Since this condition is same as a non-local equation of state [78], it proves to be very advantageous in formulating solutions to the equations of motion (2). The possibility of fulfilling this condition in a real spherically symmetric configuration is an intriguing avenue for further discussion. We intend to delve into this aspect by considering scenarios where the anisotropic ${\mathbb {EMT}}$ can arise naturally, such as in the presence of exotic matter distributions or strong gravitational fields near compact objects like neutron stars or black holes. Exploring such scenarios can shed light on the applicability and consequences of the condition (34) in realistic astrophysical interiors. Therefore, as defined in [43], nullifying this may correspond to very different systems.

4 Physical conditions for compact models

Various proposals have been discussed in the literature to address the field equations illustrating physically relevant compact bodies. Nevertheless, if such solutions fall short of meeting acceptability criteria, they cease to be relevant for modeling real compact stars. Numerous conditions in this context have been suggested and adhered to by various researchers [79, 80]. Some of these conditions are outlined below.

• The radial/temporal metric functions should exhibit finiteness, free of singularities and remain positive everywhere in a self-gravitating fluid interior.

• The matter variables, including energy density and pressure, must reach their maximum at the center $(r=0),$ exhibiting positively finite trend across the entire domain. Additionally, their first-order derivatives needed to be null at $r=0$ and follows negative profile towards the boundary.

• In a compact body, the particles are arranged in a certain manner. This arrangement tells how much these particles are closed to each other, ultimately defining the compactness of that system. One can also define it as the mass to radius ratio which necessarily be lower than the proposed limit for spherical fluid distribution given by [81, 82]

  $$\begin{aligned} \frac{2{\mathcal {M}}}{{\mathcal {R}}} < \frac{8}{9}. \end{aligned}$$

  (35)

• Bearing an ordinary matter by the interior of a celestial body can be guaranteed through the satisfaction of some constraints. They are referred to the energy conditions which are in fact linear combinations of fluid parameters in the ${\mathbb {EMT}}.$ In the fluid setup under consideration, these constraints take the following form

  $$\begin{aligned}{} & {} \rho +p_t \ge 0, \quad \rho +p_{r} \ge 0,\nonumber \\{} & {} \rho -p_{t}\ge 0, \quad \rho -p_{r} \ge 0,\nonumber \\{} & {} \rho \ge 0, \quad \rho +p_{r}+2p_{t} \ge 0. \end{aligned}$$

  (36)

  Among all the above conditions, the dominant energy bounds (i.e., $\rho -p_{r} \ge 0$ and $\rho -p_{t} \ge 0$) are crucial, demanding the energy density to be greater than both radial/tangential pressures everywhere.

• The gravitational redshift can be defined in the interior fluid configuration as $z=e^{-\eta _1/2}-1.$ Given that this factor depends solely on $g_{tt}$ metric potential, it is imperative for it to decrease outwards. Additionally, its value must not exceed 5.211 at $\Sigma$ for the model to be considered acceptable [81].

• To assess the system’s stability just deviated from the hydrostatic equilibrium has garnered significant attention in modern research. Herrera et al. [83, 84] introduced the concept of cracking, occurring within the fluid when the total force in the radial direction switches its sign at a specific point due to some external disturbances. The avoidance of cracking relies on the satisfaction of the following inequality

  $$\begin{aligned} -1 \le v_{st}^{2}-v_{sr}^{2} \le 0, \end{aligned}$$

  (37)

  where $v_{st}^{2}=\frac{dp_{t}}{d\rho }$ being the tangential and $v_{sr}^{2}=\frac{dp_{r}}{d\rho }$ indicates the radial sound speed.

5 Formation of different models

Various models have been proposed by different researchers to derive solutions to the field equations. For instance, Herrera [46] presented such solutions by incorporating two constraints: the Gokhroo–Mehra ansatz, and the polytropic equation, along with the condition of a vanishing complexity factor. We formulate three solutions by taking different conditions among all of them and examine their physical properties in the subsequent subsections.

5.1 Vanishing complexity condition with null radial pressure

There are five unknowns $(\rho ,p_r,p_t,\eta _1,\eta _2)$ in the field equations (9)–(11), requiring additional constraints for obtaining a unique solution. To simplify the system, we impose the constraints $p_r=0$ and ${\mathcal {Y}}_{TF}=0,$ representing a spherical solution with only tangential pressure, as suggested by Florides [85]. The former condition yields from Eq. (10) as

$$\begin{aligned}&2 \eta _2 ' \big [4 e^{\eta _2 } \big \{-4 \alpha +\alpha \big (r^2-1\big ) r \eta _1 '\nonumber \\&\qquad +(8 \alpha -1) r^2\big \}+\alpha \big \{r \big (\eta _1 ' \big (4 \big (r^2-3\big )\nonumber \\&\qquad +r \eta _1 ' \big (r \eta _1 '+4\big )\big )+4 r \big (r \eta _1 '+2\big ) \eta _1 ''\big )-48\big \}\big ]\nonumber \\&\qquad -8 e^{\eta _2 } \big [\alpha \big (r^2-1\big ) \big \{2 r \eta _1 ''\nonumber \\&\qquad +\eta _1 ' \big (r \eta _1 '+4\big )\big \}+r \big (4 \alpha r^2-1\big )\big ]\nonumber \\&\qquad +8 r \big \{2 \alpha \big (r^2-2\big )+1\big \} e^{2 \eta _2 }+\alpha \big [r^3 \eta _1 '^4\nonumber \\&\qquad +4 r \big \{\eta _1 '' \big (r^2 \eta _1 ''+4 r^2-12\big )+4 \big (r^2+2\big )\nonumber \\&\qquad +16 \eta _2 ''-8 r \eta _1 ^{(3)}\big \}+4 r \eta _1 '^2 \big \{r^2 \nonumber \\&\qquad \times \big (\eta _2 ''-\eta _1 ''\big )+2 \big (r^2+5\big )\big \}+8 r^2 \eta _1 '^3\nonumber \\&\qquad +8 \eta _1 ' \big \{4 \big (r^2 \big (\eta _2 ''-\eta _1 ''\big )+r^2+3\big )\nonumber \\&\qquad -r^3\eta _1 ^{(3)}\big \}\big ]-\alpha r \eta _2 '^2 \big (r \eta _1 '+4\big ) \big (3 r \eta _1 '+4\big )=0, \end{aligned}$$

(38)

and the complexity-free constraint takes the form after combining with Eqs. (9), (11) and (34) as

$$\begin{aligned}&3\alpha \big [16 \big \{-\eta _1 ' \big (r^2 \eta _2 ''-2 r^2 \eta _1 ''+4\big )\nonumber \\&\qquad -\eta _2 ' \big (3 r^2 \eta _1 ''+r^2 \eta _1 '^2+4 r^2+4 r \eta _1 '-4\big )+2 r \nonumber \\&\qquad \times \big (r \eta _1 ^{(3)}-2 \eta _2 ''+2 \eta _1 ''\big )\nonumber \\&\qquad +r \eta _2 '^2 \big (r \eta _1 '+4\big )\big \}-r \big \{2 r \big (\eta _1 ''-2 e^{\eta _2 }+2\big )-\eta _2 ' \big (r \eta _1 '+4\big )\nonumber \\&\qquad +r\eta _1'^2+4\eta _1'\big \}^2\big ]\big [\pi \alpha r^{-1} e^{-\eta _2 } \big \{2 r \big (\eta _1 ''-2 e^{\eta _2 }+2\big )\nonumber \\&\qquad -\eta _2 ' \big (r \eta _1 '+4\big )+r \eta _1 '^2+4 \eta _1 '\big \}\nonumber \\&\qquad +2\pi \big ]^{-1}-\frac{3r}{\pi }\big [2 \eta _1 '^2 \big \{16 \alpha +6 \alpha r^2 \eta _2 ''\nonumber \\&\qquad -2 \alpha r^2 \big (2 e^{\eta _2 }+1\big ) \eta _1 ''-8 \alpha r^2 e^{\eta _2 }+4 \alpha r^2 e^{2 \eta _2 }\nonumber \\&\qquad +4 \alpha r^2-r^2 e^{2 \eta _2 }-8 \alpha e^{\eta _2 }\big \}\nonumber \\&\qquad -\alpha \eta _2 '^2 \big \{4 \big (4 \big (3 r^2+e^{\eta _2 }-2\big )+11 r^2 \eta _1 ''\big )+\big (2 e^{\eta _2 }+15\big ) \nonumber \\&\qquad \times r^2\eta _1 '^2+12 r \big (e^{\eta _2 }+6\big ) \eta _1 '\big \}\nonumber \\&\qquad +2 \eta _2 ' \big \{\eta _1 ' \big (4 \alpha r^2 \big (e^{\eta _2 }+6\big ) \eta _1''\nonumber \\&\qquad -32 \alpha -14 \alpha r^2 \eta _2 ''+4 \alpha r^2 \nonumber \\&\qquad +8\alpha r^2 e^{\eta _2 }-4 \alpha r^2 e^{2 \eta _2 }+r^2 e^{2 \eta _2 }+16 \alpha e^{\eta _2 }\big )\nonumber \\&\qquad +\alpha r^2 \big (2 e^{\eta _2 }+1\big ) \eta _1 '^3+2 r \big (8 \alpha -28 \alpha \eta _2 '' \nonumber \\&\qquad +2\alpha \big (3 e^{\eta _2 }+14\big ) \eta _1 ''+12 \alpha e^{\eta _2 }-4 \alpha e^{2 \eta _2 }\nonumber \\&\qquad +12 \alpha r \eta _1 ^{(3)}+e^{2 \eta _2 }\big )+4 \alpha r \big (3 e^{\eta _2 }+2\big ) \eta _1 '^2\big \}\nonumber \\&\qquad -\alpha r^2 \big (2 e^{\eta _2 }-1\big ) \eta _1 '^4+12 \alpha r \eta _2 '^3 \big (r \eta _1 '+4\big )\nonumber \\&\qquad +4 r \big \{4 \alpha \big (2 \eta _2 ^{(3)}+r \big (\big (e^{\eta _2 }-1\big )^2-\eta _1 ^{(4)}\big )\nonumber \\&\qquad -4\eta _1 ^{(3)}\big )+8 \alpha r \eta _2 '' \big (\eta _1 ''+1\big )-\alpha r \big (2 e^{\eta _2 }+3\big ) \eta _1 ''^2\nonumber \\&\qquad +r \big (4 \alpha -8 \alpha e^{\eta _2 }+(4 \alpha -1) e^{2 \eta _2 }\big ) \nonumber \\&\qquad \times \eta _1 ''\big \}+4 r \eta _1 ' \big \{8 \alpha +2 \alpha r \eta _2 ^{(3)}+16 \alpha \eta _2 ''\nonumber \\&\qquad -2 \alpha \big (3 e^{\eta _2 }+4\big ) \eta _1 ''-6 \alpha r \eta _1 ^{(3)}-12 \alpha e^{\eta _2 } \nonumber \\&\qquad -e^{2\eta _2 }+4 \alpha e^{2 \eta _2 }\big \}-4 \alpha r \big (3 e^{\eta _2 }-2\big ) \eta _1 '^3\big ]\nonumber \\&\qquad -4e^{\eta _2}r\big [4 \alpha r \eta _1 '^2 \big (2 r^2 e^{\eta _2 }-2 r^2 \eta _2 ''-r^2 \eta _1 '' \nonumber \\&\qquad -2 r^2+4 e^{\eta _2 }-12\big )-\alpha r^3 \eta _1 '^4-12 \alpha r^2 \eta _2 '^3 \big (r \eta _1 '+4\big )\nonumber \\&\qquad +\alpha r \eta _2 '^2 \big (44 r^2 \eta _1 ''+11 r^2 \eta _1 '^2\nonumber \\&\qquad +48 r^2+48 r \eta _1 '-64\big )-4 r \big \{4 \big (8 \alpha -2 \alpha r^2 e^{\eta _2 }\nonumber \\&\qquad +\alpha r^2 e^{2 \eta _2 }-\alpha r^2 \eta _1 ^{(4)}+\alpha r^2-12 \alpha e^{\eta _2 } \nonumber \\&\qquad +2 \alpha r \eta _2 ^{(3)}+4 \alpha e^{2 \eta _2 }-4 \alpha r \eta _1 ^{(3)}+2 e^{\eta _2 }\nonumber \\&\qquad -e^{2 \eta _2 }\big )+8 \alpha r^2 \eta _2 '' \big (\eta _1 ''+1\big )-4 \alpha \big (\big (r^2+2\big ) e^{\eta _2 } \nonumber \\&\qquad -r^2-4\big ) \eta _1 ''-3 \alpha r^2 \eta _1 ''^2\big \}-8 \alpha r^2 \eta _1 '^3\nonumber \\&\qquad +8 \alpha \eta _1 ' \big (2 r^3 \eta _1 ^{(3)}-6 r^2 \eta _2 ''+4 r^2 e^{\eta _2 }-r^3 \eta _2 ^{(3)}\nonumber \\&\qquad +2 r^2 \eta _1 ''-4 r^2+8 e^{\eta _2 }-16\big )-2 \eta _2 ' \big \{\alpha \big (-r^3\big ) \eta _1 '^3\nonumber \\&\qquad +2 \alpha r \eta _1 ' \big (9 r^2 \eta _1 ''-2 r^2-7 r^2 \eta _2 ''\nonumber \\&\qquad +2 r^2 e^{\eta _2 }+4 e^{\eta _2 }-28\big )-4 \alpha r^2 \eta _1 '^2 \nonumber \\&\qquad +4 \big (-16 \alpha +6 \alpha r^3 \eta _1 ^{(3)}-14 \alpha r^2 \eta _2 ''+8 \alpha r^2 e^{\eta _2 }\nonumber \\&\qquad +12 \alpha r^2 \eta _1 ''-r^2 e^{\eta _2 }+8 \alpha e^{\eta _2 }\big )\big \}\big ]\nonumber \\&\qquad \times \big [2 r \big \{2 \alpha +(1-2 \alpha ) e^{\eta _2 }+\alpha \eta _1 ''\big \}-\alpha \eta _2 ' \big (r \eta _1 '+4\big )\nonumber \\&\qquad +\alpha r \eta _1 '^2+4 \alpha \eta _1 '\big ]^{-1}=0. \end{aligned}$$

(39)

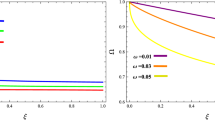
Since the higher-order curvature term in the modified model makes the above two equations up to forth-order in terms of the metric potentials, obtaining their analytical solution is not possible. Therefore, we perform the numerical integration on Eqs. (38) and (39) by choosing some admissible initial conditions and determine both $g_{rr}$ and $g_{tt}$ functions. We check the profile of resulting $e^{\eta _1}$ and $e^{-\eta _2}$ in Fig. 1 and find them exhibiting positive and non-singular trend everywhere. Furthermore, their values at the center are consistent with the expected results, as $e^{\eta _1(0)}=c_1$ (say $>0$) and $e^{-\eta _2(0)}=1.$ A point where both these functions meet with each other is referred to as the radius or surface boundary of a star. In the case under discussion, we retrieve its different values along with the compactness factor for distinct choices of the model parameter as

• For $\alpha =0.2,$ we obtain ${\mathcal {R}}=0.23$ and the compactness becomes

  $$\begin{aligned}{} & {} e^{\eta _1(0.23)}=e^{-\eta _2(0.23)}\approx 0.88=1-\frac{2{\mathcal {M}}}{{\mathcal {R}}}\\{} & {} \quad \Rightarrow \quad \frac{2{\mathcal {M}}}{{\mathcal {R}}} \approx 0.12 < \frac{8}{9}. \end{aligned}$$

• For $\alpha =0.3,$ we obtain ${\mathcal {R}}=0.3$ and the compactness becomes

  $$\begin{aligned}{} & {} e^{\eta _1(0.3)}=e^{-\eta _2(0.3)}\approx 0.83=1-\frac{2{\mathcal {M}}}{{\mathcal {R}}}\\{} & {} \quad \Rightarrow \quad \frac{2{\mathcal {M}}}{{\mathcal {R}}} \approx 0.17 < \frac{8}{9}. \end{aligned}$$

• For $\alpha =0.4,$ we obtain ${\mathcal {R}}=0.31$ and the compactness becomes

  $$\begin{aligned}{} & {} e^{\eta _1(0.31)}=e^{-\eta _2(0.31)}\approx 0.8=1-\frac{2{\mathcal {M}}}{{\mathcal {R}}}\\{} & {} \quad \Rightarrow \quad \frac{2{\mathcal {M}}}{{\mathcal {R}}} \approx 0.2 < \frac{8}{9}. \end{aligned}$$

Fig. 1

Metric functions $e^{\eta _1}$ (red) and $e^{-\eta _2}$ (blue) for $\alpha =0.2$ (upper left), 0.3 (upper right) and 0.4 (lower) corresponding to Model I

In Fig. 2, we explore the trend of the energy density, exhibiting a maximum at $r=0$ and decreasing towards the boundary surface. Additionally, the interior configuration influenced by $f({\textbf{R}})$ corrections becomes less dense in comparison with that of ${\mathbb{G}\mathbb{R}}$ [68]. As the system with no radial pressure is associated with Florides’ solution, stability of the system under consideration can be maintained only if the tangential pressure exhibits a positively increasing trend with increasing radius. The right plot of the same figure illustrates the behavior of $p_t,$ which aligns with the needful result. Moreover, the anisotropy is viewed to be just opposite to the tangential pressure as in this case we have $\Pi =-p_t$ (lower plot).

The energy conditions, depicted in Fig. 3, manifest an acceptable behavior, affirming the viability of the first resulting solution. In Fig. 4 (left), the gravitational redshift is illustrated, showcasing a decrease with increasing r,  with its surface value calculated as $z(0.23)\approx 0.065,~z(0.3)\approx 0.096,~z(0.31)\approx 0.115$ corresponding to $\alpha =0.2,~0.3,~0.4,$ respectively. This value is significantly lower than its observationally found upper limit, i.e., $z({{\mathcal {R}}})=5.211.$ We also examine stability criterion in Fig. 4 (right), revealing that cracking is not occurred in the developed model anywhere, and hence, maintains stability.

Fig. 2

Matter variables and anisotropy for $\alpha =0.2$ (red), 0.3 (blue) and 0.4 (black) corresponding to Model I

Fig. 3

Energy bounds for $\alpha =0.2$ (red), 0.3 (blue) and 0.4 (black) corresponding to Model I

Fig. 4

Redshift and cracking for $\alpha =0.2$ (red), 0.3 (blue) and 0.4 (black) corresponding to Model I

5.2 Polytrope admitting vanishing complexity

The polytrope associated with the anisotropic matter distribution plays a significant role in modern research. Various authors have discussed and investigated the polytropic solutions in different gravitational proposals [31,32,33]. In order to get a solution to the field equations (9)–(11), we consider a polytropic equation of state coupled with the vanishing complexity factor. Although a brief discussion of a polytropic model can be seen in [46], we comprehensively elaborate the corresponding solution through pictorial analysis. We introduce the above two conditions mathematically to proceed as

$$\begin{aligned} p_r={\mathcal {J}}\rho ^{\eta _3}={\mathcal {J}}\rho ^{1+\frac{1}{{\mathcal {X}}}}, \quad {\mathcal {Y}}_{TF}=0, \end{aligned}$$

(40)

where

• ${\mathcal {J}}$ symbolizes the polytropic constant,

• ${\mathcal {X}}$ indicates the polytropic index,

• $\eta _3$ is the polytropic exponent.

The two constraints given in Eq. (40) produce highly non-linear equations in $\eta _1$ and $\eta _2$ as

$$\begin{aligned}&-4 \alpha e^{-2 \eta _2 } \big (r \eta _1 '+4\big ) \big \{\eta _1 ' \big (r^2 \eta _2 ''-2 r^2 \eta _1 ''+4\big )\nonumber \\&\qquad +\eta _2 ' \big (3 r^2 \eta _1 ''+r^2 \eta _1 '^2+4 r^2+4 r \eta _1 '\nonumber \\&\qquad -4\big )-2 r \big (r \eta _1 ^{(3)}-2 \eta _2 ''+2 \eta _1 ''\big )-r \eta _2 '^2 \big (r \eta _1 '+4\big )\big \}\nonumber \\&\qquad +\alpha r e^{-2 \eta _2 } \big \{2 r \big (\eta _1 ''+2\big )-2 e^{\eta _2 }\nonumber \\&\qquad -\eta _2 ' \big (r \eta _1 '+4\big )+r \eta _1 '^2+4 \eta _1 '\big \}^2\nonumber \\&\qquad +8 e^{-2 \eta _2 } \big (-r \eta _2 '+e^{\eta _2 }+1\big ) \big \{\alpha \eta _2 ' \big (r \eta _1 '+4\big )+r \nonumber \\&\qquad \times \big ((4 \alpha +1) e^{\eta _2 }-4 \alpha -2 \alpha \eta _1 ''\big )\nonumber \\&\qquad -\alpha r\eta _1 '^2-4 \alpha \eta _1 '\big \}+{\mathcal {J}} 8^{\frac{2}{{\mathcal {X}}}} \pi ^{-\frac{1}{{\mathcal {X}}}} r^{\frac{3}{{\mathcal {X}}}}\big (e^{-2\eta _2}\big )^{1+\frac{1}{{\mathcal {X}}}}\nonumber \\&\qquad \times \big [\alpha r^3 \eta _1 '^4-4 \alpha r \eta _1 '^2 \big \{2 r^2 \eta _2 ''+2 \big (r^2+1\big ) \big (e^{\eta _2 }-1\big )\nonumber \\&\qquad -r^2 \eta _1 ''\big \}-12 \alpha r^2 \eta _2 '^3 \big (r \eta _1 '+4\big )\nonumber \\&\qquad +\alpha r \eta _2 '^2 \big (44 r^2 \eta _1 ''+13 r^2 \eta _1 '^2+48 r^2+64 r \eta _1 '-32\big )\nonumber \\&\qquad +4 r \big \{2 \big (2 \alpha r^2 e^{2 \eta _2 } -4 \alpha r^2 e^{\eta _2 }\nonumber \\&\qquad -4 \alpha +2 \alpha r^2 \eta _1 ^{(4)}+2 \alpha r^2-4 \alpha r \eta _2 ^{(3)}+4 \alpha e^{2 \eta _2 }\nonumber \\&\qquad +8 \alpha r \eta _1 ^{(3)}+e^{\eta _2}+e^{2 \eta _2 }\big )-8 \alpha r^2 \eta _2 ''\nonumber \\&\qquad \times \big (\eta _1 ''+1\big )-4 \alpha \big (\big (r^2+1\big ) e^{\eta _2 }-r^2+1\big ) \eta _1 ''+5 \alpha r^2 \eta _1 ''^2\big \}\nonumber \\&\qquad +8 \alpha r^2 \eta _1 '^3-8 \alpha \eta _1 ' \big (r^3 \eta _2 ^{(3)}\nonumber \\&\qquad -2 r^3 \eta _1 ^{(3)}+6 r^2 \eta _2 ''+4 r^2 e^{\eta _2 }-6 r^2 \eta _1 ''\nonumber \\&\qquad -4 r^2+4 e^{\eta _2 }+4\big )+2 \eta _2 ' \big \{2 \alpha r \eta _1 ' \big (7 r^2 \eta _2 ''\nonumber \\&\qquad +2 r^2 e^{\eta _2 }-11 r^2 \eta _1 ''-2 r^2+2 e^{\eta _2 }+6\big )-\alpha r^3 \eta _1 '^3\nonumber \\&\qquad -12 \alpha r^2 \eta _1 '^2-4 \big (6 \alpha r^3 \eta _1 ^{(3)}-4 \alpha \nonumber \\&\qquad -14 \alpha r^2 \eta _2 ''+16 \alpha r^2 \eta _1 ''+8 \alpha r^2\nonumber \\&\qquad +r^2 e^{\eta _2 }-4 \alpha e^{\eta _2 }\big )\big \}\big ]^{1+\frac{1}{{\mathcal {X}}}}=0 \end{aligned}$$

(41)

Fig. 5

Metric functions $e^{\eta _1}$ (red) and $e^{-\eta _2}$ (blue) for $\alpha =0.2$ (upper left), 0.3 (upper right) and 0.4 (lower) corresponding to Model II

$$\begin{aligned}&3\alpha \big [16 \big \{2 r \big (r \eta _1 ^{(3)}-2 \eta _2 ''+2 \eta _1 ''\big )\nonumber \\&\qquad -\eta _1 ' \big (r^2 \eta _2 ''-2 r^2 \eta _1 ''+4\big )\nonumber \\&\qquad -\eta _2 ' \big (3 r^2 \eta _1 ''+r^2 \eta _1 '^2-4 \nonumber \\&\qquad +4 r^2+4 r \eta _1 '\big )+r \eta _2 '^2 \big (r \eta _1 '+4\big )\big \}\nonumber \\&\qquad +r \big \{2 r \big (\eta _1 ''-2 e^{\eta _2 }+2\big )-\eta _2 ' \big (r \eta _1 '+4\big )+r \eta _1 '^2 \nonumber \\&\qquad +4 \eta _1 '\big \}^2\big ]\big [\pi \alpha r^{-r} e^{-\eta _2 } \big \{\eta _2 ' \big (r \eta _1 '+4\big )\nonumber \\&\qquad +2 r \big (2 e^{\eta _2 }-\eta _1 ''-2\big )-r \eta _1 '^2-4 \eta _1 '\big \}+2 \pi \big ]^{-1} \nonumber \\&\qquad +\frac{3}{\pi }\big [4 \alpha r \eta _1 '^2 \big \{r^2 \eta _2 ''+2 \big (r^2 e^{\eta _2 }-r^2+e^{\eta _2 }+1\big )\nonumber \\&\qquad -3 r^2 \eta _1 ''\big \}-\alpha r^3 \eta _1 '^4-4 r \big \{2 \big (e^{\eta _2 }\big (1 \nonumber \\&\qquad -4 \alpha r^2\big )+\big (2 \alpha \big (r^2+2\big )+1\big ) e^{2 \eta _2 }+2 \alpha \big (r^2-2\big )\nonumber \\&\qquad +4 \alpha r \eta _1 ^{(3)}\big )-4 \alpha \big (\big (r^2+1\big ) e^{\eta _2 } \nonumber \\&\qquad -r^2-3\big ) \eta _1 ''+\alpha r^2 \eta _1 ''^2-16 \alpha \eta _2 ''\big \}\nonumber \\&\qquad -\alpha r \eta _2 '^2 \big (5 r^2 \eta _1 '^2+32 r \eta _1 '+48\big )-8 \alpha r^2 \eta _1 '^3+8 \alpha \nonumber \\&\qquad \times \eta _1 ' \big (4 r^2 \eta _2 ''-r^3 \eta _1 ^{(3)}+4 r^2 e^{\eta _2 }-8 r^2 \eta _1 ''\nonumber \\&\qquad -4 r^2+4 e^{\eta _2 }+12\big )+\eta _2 ' \big \{6 \alpha r^3 \eta _1 '^3+8 \big (e^{\eta _2 }\nonumber \\&\qquad \times \big (r^2-4 \alpha \big ) +6 \alpha r^2 \eta _1 ''+4 \alpha \big (2 r^2-3\big )\big )\nonumber \\&\qquad +40 \alpha r^2 \eta _1 '^2-8 \alpha r \eta _1 ' \big (\big (r^2+1\big ) e^{\eta _2 }-5 \nonumber \\&\qquad -2 r^2 \eta _1 ''-3 r^2\big )\big \}\big ]+4re^{\eta _2}\big [4 \alpha r \eta _1 '^2 \big \{2 r^2 \eta _2 ''\nonumber \\ \end{aligned}$$

$$\begin{aligned}&\qquad +2 \big (r^2-2\big ) \big (e^{\eta _2 }-1\big )-r^2 \eta _1 ''\big \}+12 \alpha r^2 \nonumber \\&\qquad \times \eta _2 '^3 \big (r \eta _1 '+4\big )-\alpha r^3 \eta _1 '^4\nonumber \\&\qquad -\alpha r \eta _2 '^2 \big (44 r^2 \eta _1 ''+13 r^2 \eta _1 '^2+48 r^2+64 r \eta _1 '-32\big )-4 r \nonumber \\&\qquad \times \big \{4\big (\alpha r^2 e^{2 \eta _2 }-8 \alpha -2 \alpha r^2 e^{\eta _2 }+\alpha r^2 \eta _1 ^{(4)}+\alpha r^2\nonumber \\&\qquad -2 \alpha r \eta _2 ^{(3)}+12 \alpha e^{\eta _2 }-4 \alpha e^{2 \eta _2 } \nonumber \\&\qquad +4 \alpha r \eta _1 ^{(3)}+2 e^{\eta _2 }-e^{2 \eta _2 }\big )-8 \alpha r^2 \eta _2 '' \big (\eta _1 ''+1\big )\nonumber \\&\qquad -4 \alpha \big (\big (r^2-2\big ) e^{\eta _2 }-r^2+4\big ) \eta _1 ''+5 \alpha \nonumber \\&\qquad \times r^2 \eta _1 ''^2\big \}-8 \alpha r^2 \eta _1 '^3+8 \alpha \eta _1 ' \big (r^3 \eta _2 ^{(3)}\nonumber \\&\qquad -2 r^3 \eta _1 ^{(3)}+6 r^2 \eta _2 ''+4 r^2 e^{\eta _2 }-6 r^2 \eta _1 ''-4 r^2 \nonumber \\&\qquad -8 e^{\eta _2 }+16\big )+2 \eta _2 ' \big \{\alpha r^3 \eta _1 '^3\nonumber \\&\qquad -2 \alpha r \eta _1 ' \big (7 r^2 \eta _2 ''+2 r^2 e^{\eta _2 }-11 r^2 \eta _1 ''-2 r^2-4 e^{\eta _2 }\nonumber \\&\qquad +12\big )+12 \alpha r^2 \eta _1 '^2+4 \big (6 \alpha r^3 \eta _1 ^{(3)}-16 \alpha \nonumber \\&\qquad -14 \alpha r^2 \eta _2 ''+16 \alpha r^2 \eta _1 ''+8 \alpha r^2+r^2 e^{\eta _2 }\nonumber \\&\qquad +8 \alpha e^{\eta _2 }\big )\big \}\big ]\big [\alpha \eta _2 ' \big (r \eta _1 '+4\big )+2 r \big \{(2 \alpha +1) e^{\eta _2 }\nonumber \\&\qquad -2 \alpha -\alpha \eta _1 ''\big \}-\alpha r\eta _1 '^2-4 \alpha \eta _1'\big ]^{-1}\nonumber \\&\qquad +\frac{3r}{\pi }\big [\alpha \eta _2 '^2 \big \{r^2 \big (2 e^{\eta _2 }+17\big ) \eta _1 '^2\nonumber \\&\qquad +16 \big (3 r^2+e^{\eta _2 }\big )+44 r^2 \eta _1 ''+4 r \big (3 e^{\eta _2 }+22\big ) \eta _1 '\big \} \nonumber \\&\qquad -2 \eta _1 '^2 \big \{6 \alpha r^2 \eta _2 ''-2 \alpha r^2 \big (2 e^{\eta _2 }+3\big ) \eta _1 ''\nonumber \\&\qquad +4 \alpha r^2 e^{2 \eta _2 }-4 \alpha r^2+r^2 e^{2 \eta _2 }-8 \alpha e^{\eta _2 }\big \}+2\nonumber \\&\qquad \times \eta _2 ' \big \{\eta _1 ' \big (14 \alpha r^2 \eta _2 ''-4 \alpha r^2 \big (e^{\eta _2 }+7\big ) \eta _1 ''\nonumber \\&\qquad +4 \alpha r^2 e^{2 \eta _2 }-12 \alpha r^2+r^2 e^{2 \eta _2 }-16 \alpha e^{\eta _2 }\big )\nonumber \\&\qquad +\alpha r^2 \big (-\big (2 e^{\eta _2 }+3\big )\big ) \eta _1 '^3+2 r \big (28 \alpha \eta _2 ''-24 \alpha \nonumber \\&\qquad -6 \alpha \big (e^{\eta _2 }+6\big ) \eta _1 ''+4 \alpha e^{\eta _2 }+4 \alpha e^{2 \eta _2 }\nonumber \\&\qquad -12 \alpha r \eta _1 ^{(3)}+e^{2 \eta _2 }\big )-12 \alpha r \big (e^{\eta _2 }+2\big ) \eta _1 '^2\big \}\nonumber \\&\qquad +\alpha r^2 \big (2 e^{\eta _2 }+1\big ) \eta _1 '^4-12 \alpha r \eta _2 '^3 \big (r \eta _1 '+4\big ) \nonumber \\&\qquad -4 r \big \{-4 \alpha \big (r \big (\big (e^{\eta _2 }-1\big )^2+\eta _1 ^{(4)}\big )\nonumber \\&\qquad -2 \eta _2 ^{(3)}+4 \eta _1 ^{(3)}\big )+8 \alpha r \eta _2 '' \big (\eta _1 ''+1\big )-\alpha r \big (2 e^{\eta _2 }\nonumber \\&\qquad +5\big ) \eta _1 ''^2+r \big ((4 \alpha +1) e^{2 \eta _2 }-4 \alpha \big ) \eta _1 ''\big \}\nonumber \\&\qquad -4 r \eta _1 ' \big \{2 \alpha r \eta _2 ^{(3)}-8 \alpha +16 \alpha \eta _2 ''-2 \alpha \big (3 e^{\eta _2 }\nonumber \\&\qquad +8\big ) \eta _1 ''+4 \alpha e^{\eta _2 }+4 \alpha e^{2 \eta _2 }-6 \alpha r \eta _1 ^{(3)}\nonumber \\&\qquad +e^{2 \eta _2 }\big \}+4 \alpha r \big (3 e^{\eta _2 }+2\big ) \eta _1 '^3\big ]. \end{aligned}$$

(42)

We fix ${\mathcal {J}}=0.9$ and ${\mathcal {X}}=0.05$ to calculate $\eta _{1}$ and $\eta _{2}$ by solving Eqs. (41) and (42). Since these equations are solved numerically, we plot the graphical behavior of both resulting metric functions in Fig. 5 (showing an acceptable profile) rather than obtaining their expressions. Furthermore, it is found that $e^{\eta _1(0)}=c_2$ (a positive constant) and $e^{-\eta _2(0)}=1.$ The surface boundary along with the compactness factor for different values of $\alpha$ are

Fig. 6

Matter variables and anisotropy for $\alpha =0.2$ (red), 0.3 (blue) and 0.4 (black) corresponding to Model II

• For $\alpha =0.2,$ we obtain ${\mathcal {R}}=0.53$ and the compactness becomes

  $$\begin{aligned}{} & {} e^{\eta _1(0.53)}=e^{-\eta _2(0.53)}\approx 0.73=1-\frac{2{\mathcal {M}}}{{\mathcal {R}}}\\{} & {} \quad \Rightarrow \quad \frac{2{\mathcal {M}}}{{\mathcal {R}}} \approx 0.27 < \frac{8}{9}. \end{aligned}$$

• For $\alpha =0.3,$ we obtain ${\mathcal {R}}=0.54$ and the compactness becomes

  $$\begin{aligned}{} & {} e^{\eta _1(0.54)}=e^{-\eta _2(0.54)}\approx 0.6=1-\frac{2{\mathcal {M}}}{{\mathcal {R}}}\\{} & {} \quad \Rightarrow \quad \frac{2{\mathcal {M}}}{{\mathcal {R}}} \approx 0.4 < \frac{8}{9}. \end{aligned}$$

• For $\alpha =0.4,$ we obtain ${\mathcal {R}}=0.55$ and the compactness becomes

  $$\begin{aligned}{} & {} e^{\eta _1(0.55)}=e^{-\eta _2(0.55)}\approx 0.5=1-\frac{2{\mathcal {M}}}{{\mathcal {R}}}\\{} & {} \quad \Rightarrow \quad \frac{2{\mathcal {M}}}{{\mathcal {R}}} \approx 0.5 < \frac{8}{9}. \end{aligned}$$

Fig. 7

Energy bounds for $\alpha =0.2$ (red), 0.3 (blue) and 0.4 (black) corresponding to Model II

Figure 6 depicts the trend of the energy density and radial/tangential pressures in relation with the radial coordinate for chosen parametric values. They are found to attain their maximum at the core and minimum at the boundary followed by a monotonically decreasing behavior. For every choice of $\alpha ,$ the radial pressure disappears at its respective interface (upper left plot). We interpret the plots of all the energy conditions whose satisfaction is seen in Fig. 7, except $\rho -p_t$ for $\alpha =0.4.$ Hence, they characterize a physically viable solution only for $\alpha =0.2$ and 0.3.

We present the gravitational redshift corresponding to this model in the left plot of Fig. 8, following decreasing trend when r increases. For $\alpha =0.2,~0.3$ and 0.4,  this factor takes the values $z(0.53)=0.379,~z(0.54)=0.275$ and $z(0.55)=0.415,$ respectively. The stable region is also investigated in the same figure from which we find that the cracking occurs only for $\alpha =0.4.$ Hence, the system is not stable for this value. However, the other two values provide physically stable interiors, unlike the results obtained in ${\mathbb{G}\mathbb{R}}$ [68].

Fig. 8

Redshift and cracking for $\alpha =0.2$ (red), 0.3 (blue) and 0.4 (black) corresponding to Model II

A more suited approach for solving differential equations is to transform them into dimensionless form. To achieve this, we introduce new variables that offer the original variables as dimensionless quantities. Let us propose these variables as

$$\begin{aligned}&\rho _c=\frac{p_{rc}}{\delta }, \quad r=\frac{\zeta }{{\mathcal {Z}}}, \quad {\mathcal {Z}}^2=\frac{4\pi \rho _c}{\delta ({\mathcal {X}}+1)}, \end{aligned}$$

(43)

$$\begin{aligned}&\Upsilon ^{{\mathcal {X}}}=\frac{\rho }{\rho _c}, \quad \nu (\zeta )=\frac{{\mathcal {Z}}^3m(r)}{4\pi \rho _c}, \end{aligned}$$

(44)

4 where $p_{rc}$ and $\rho _{c}$ are the central radial pressure and central energy density, respectively. At $r={\mathcal {R}}$ (or $\zeta (r)=\zeta ({\mathcal {R}})$), we have $\Upsilon \big (\zeta ({\mathcal {R}})\big )=0.$ When combining the above variables with the mass function (15) and generalized ${\textbf{TOV}}$ equation (17), they take the following form

$$\begin{aligned}&\frac{d\nu }{d\zeta }=\frac{\Upsilon ^{{\mathcal {X}}}\zeta ^2}{f_{{\textbf{R}}}}, \end{aligned}$$

(45)

$$\begin{aligned}&\frac{2\Pi \Upsilon ^{-{\mathcal {X}}}\zeta {\mathcal {Z}}}{p_{rc} \big ({\mathcal {X}}+1\big )\big (1+\delta \Upsilon \big )} \bigg \{1-\frac{2({\mathcal {X}}+1)\delta \nu }{\zeta }\bigg \}+\frac{\zeta ^2}{1+\delta \Upsilon }\nonumber \\&\qquad \times \bigg \{1-\frac{2({\mathcal {X}}+1)\delta \nu }{\zeta }\bigg \} \frac{d\Upsilon }{d\zeta } +\frac{\delta \zeta ^3\Upsilon ^{{\mathcal {X}}+1}}{f_{{\textbf{R}}}}\nonumber \\&\qquad +\nu -\frac{\zeta ^3}{\delta {\mathcal {Z}}^2f_{{\textbf{R}}} ({\mathcal {X}}+1)}\nonumber \\&\qquad \times \left\{ \frac{1}{2}\left( f-{\textbf{R}}f_{{\textbf{R}}}\right) + \frac{e^{-\eta _2}{\mathcal {Z}}^2}{2}\frac{df_{{\textbf{R}}}}{d\zeta } \left( \frac{4}{\zeta }+\frac{de^{\eta _1}}{d\zeta }\right) \right\} =0. \end{aligned}$$

(46)

As there exist two differential equations represented by (45) and (46) involving the variables $\Upsilon ,~\nu$ and $\Pi ,$ one additional condition is required to determine them uniquely. To address this requirement, we opt for the condition ${\mathcal {Y}}_{TF}=0,$ which, when expressed in the variables (43) and (44), can be stated as follows

$$\begin{aligned}&\zeta (f_{{\textbf{R}}}+1)\frac{d\Pi }{d\zeta }+3\Pi (f_{{\textbf{R}}}+1)\nonumber \\&\qquad +\zeta \Pi f_{{\textbf{R}}}\frac{d}{d\zeta }\left( \frac{f_{{\textbf{R}}}+1}{f_{{\textbf{R}}}}\right) = \zeta \rho _c\Upsilon ^{{\mathcal {X}}-1}\nonumber \\&\qquad \times \left( {\mathcal {X}}\frac{d\Upsilon }{d\zeta }- \frac{1}{f_{{\textbf{R}}}}\frac{df_{{\textbf{R}}}}{d\zeta }\right) \nonumber \\&\qquad +\frac{3}{8\pi }\left\{ \frac{1}{2}\left( f-{\textbf{R}}f_{{\textbf{R}}} \right) +\frac{2{\mathcal {Z}}^2}{\xi e^{\eta _2}}\frac{df_{{\textbf{R}}}}{d\zeta }\right\} \nonumber \\&\qquad +\frac{{\zeta }f_{{\textbf{R}}}}{8\pi }\frac{d}{d\zeta } \left[ \frac{1}{f_{{\textbf{R}}}} \left\{ \frac{1}{2}\left( f-{\textbf{R}}f_{{\textbf{R}}}\right) +\frac{2{\mathcal {Z}}^2}{\zeta e^{\eta _2}}\frac{df_{{\textbf{R}}}}{d\zeta }\right\} \right] . \end{aligned}$$

(47)

Given that $\eta _1$ and $\eta _2$ are already known, the count of equations matches that of unknowns. Consequently, a unique solution is obtained through numerical integration, utilizing the initial conditions provided by

$$\begin{aligned} \Pi (\zeta )|_{\zeta =0}=0, \quad \Upsilon (\zeta )|_{\zeta =0}=1, \quad \nu (\zeta )|_{\zeta =0}=0. \end{aligned}$$

We set the constant $\delta =0.1$ and examine the graphical trend of the three aforementioned unknowns with respect to $\zeta ,$ while varying the values of ${\mathcal {X}}.$ In Fig. 9 (upper left), the behavior of the energy density is illustrated, reaching its maximum at $\zeta =0$ and decreasing outward, indicative of well-behaved polytropes. The corresponding mass displays a consistently increasing pattern, inversely related to ${\mathcal {X}}$ (shown in the upper right plot). The lower graph demonstrates anisotropy, diminishing at the core and exhibiting negative behavior towards the spherical surface for all opted values of ${\mathcal {X}}.$

Fig. 9

Variation of $\Upsilon ,~\nu$ and $\Pi$ for ${\mathcal {X}}=0.05$ (red), 0.06 (blue) and 0.07 (black) corresponding to Model II

5.3 Vanishing complexity condition with a non-local equation of state

Hernández and Núñez [78] proposed a particular equation connecting the radial pressure with the energy density and the integral term (which also appears in the definition of the complexity factor (30)), known as the non-local equation of state. Its expression alongside the complexity-free constraint are provided in the following

$$\begin{aligned} p_r=\rho -\frac{2}{r^3}\int _0^r\frac{\rho \bar{r}^2}{f_{{\textbf{R}}}}d\bar{r}+\frac{c_3}{2\pi r^3}, \quad {\mathcal {Y}}_{TF}=0, \end{aligned}$$

(48)

where $c_3$ being a constant. To avoid singularity at the core of a star, we impose this constant to be zero. The above (left) equation may be expressed by joining with the interior mass (15) as

$$\begin{aligned}&2 \alpha r \eta _1 '^2 \big \{3 r^2 \eta _2 ''+4 \big (r^2 e^{\eta _2 }-r^2 \eta _1 ''-r^2+e^{\eta _2 }\big )\big \}\nonumber \\&\qquad -\alpha r^3 \eta _1 '^4+6 \alpha r^2 \eta _2 '^3 \big (r \eta _1 '+4\big )\nonumber \\&\qquad -\alpha r \eta _2 '^2 \big (22 r^2 \eta _1 ''+9 r^2 \eta _1 '^2+24 r^2+48 r \eta _1 '+8\big )\nonumber \\&\qquad -4 r \big \{2 \big (2 \alpha r^2 e^{2 \eta _2 } -4 \alpha r^2 e^{\eta _2 }\nonumber \\&\qquad -4 \alpha +\alpha r^2 \eta _1 ^{(4)}+2 \alpha r^2-2 \alpha r \eta _2 ^{(3)}\nonumber \\&\qquad +4 \alpha e^{2 \eta _2 }+6 \alpha r \eta _1 ^{(3)}+2 e^{\eta _2 }\big )-4 \alpha \eta _2 '' \big (r^2 \eta _1 ''\nonumber \\&\qquad +r^2+2\big )-4 \alpha \big (r^2+1\big ) \big (e^{\eta _2 }-1\big ) \eta _1 ''\nonumber \\&\qquad +3 \alpha r^2 \eta _1 ''^2\big \}-8 \alpha r^2 \eta _1 '^3+4 \alpha \eta _1 ' \big (10 r^2 \eta _2 ''\nonumber \\&\qquad +r^3 \eta _2 ^{(3)}-3 r^3 \eta _1 ^{(3)}+8 r^2 e^{\eta _2 }-14 r^2 \eta _1 ''\nonumber \\&\qquad -8 r^2+8 e^{\eta _2 }+16\big )+\eta _2 ' \big \{4 \alpha r^3 \eta _1 '^3-2\nonumber \\&\qquad \times \alpha r \eta _1 ' \big (7 r^2 \eta _2 ''+4 r^2 e^{\eta _2 }-15 r^2 \eta _1 ''\nonumber \\&\qquad -8 r^2+4 e^{\eta _2 }-4\big )+32 \alpha r^2 \eta _1 '^2+8 \big (3 \alpha r^3 \eta _1 ^{(3)} \nonumber \\&\qquad -8 \alpha -7 \alpha r^2 \eta _2 ''+11 \alpha r^2 \eta _1 ''\nonumber \\&\qquad +8\alpha r^2+r^2 e^{\eta _2 }-4 \alpha e^{\eta _2 }\big )\big \}=0. \end{aligned}$$

(49)

However, ${\mathcal {Y}}_{TF}=0$ condition remains the same as defined in Eq. (42). Hence, both these equations are enough to obtain the solution to Eqs. (9)–(11). It should also be mentioned that Eqs. (42) and (49) are higher-order differential equations in metric components, thus we must solve it numerically as already perform in both previous models.

Figure 10 illustrates the non-singular, positive and finite profile of $e^{\eta _1}$ and $e^{-\eta _2},$ signifying physically acceptable potentials. Additionally, we establish $e^{\eta _1(0)}=c_4~(>0)$ and $e^{-\eta _2(0)}=1$ at the center, and they converge at a single point highlighted in the following

• For $\alpha =0.2,$ we obtain ${\mathcal {R}}=0.18$ and the compactness becomes

  $$\begin{aligned}{} & {} e^{\eta _1(0.18)}=e^{-\eta _2(0.18)}\approx 0.67=1-\frac{2{\mathcal {M}}}{{\mathcal {R}}}\\{} & {} \quad \Rightarrow \quad \frac{2{\mathcal {M}}}{{\mathcal {R}}} \approx 0.33 < \frac{8}{9}. \end{aligned}$$

• For $\alpha =0.3,$ we obtain ${\mathcal {R}}=0.52$ and the compactness becomes

  $$\begin{aligned}{} & {} e^{\eta _1(0.52)}=e^{-\eta _2(0.52)}\approx 0.72=1-\frac{2{\mathcal {M}}}{{\mathcal {R}}}\\{} & {} \quad \Rightarrow \quad \frac{2{\mathcal {M}}}{{\mathcal {R}}} \approx 0.28 < \frac{8}{9}. \end{aligned}$$

• For $\alpha =0.4,$ we obtain ${\mathcal {R}}=0.34$ and the compactness becomes

  $$\begin{aligned}{} & {} e^{\eta _1(0.34)}=e^{-\eta _2(0.34)}\approx 0.65=1-\frac{2{\mathcal {M}}}{{\mathcal {R}}}\\{} & {} \quad \Rightarrow \quad \frac{2{\mathcal {M}}}{{\mathcal {R}}} \approx 0.35 < \frac{8}{9}. \end{aligned}$$

Figure 11 depicts the acceptable nature of fluid triplet, exhibiting a positive maximum behavior at the core and subsequently decreasing outward. Notably, an outward trend of $p_t>p_r$ results in negative anisotropy, as evident in the lower right plot. Figure 12 illustrates the satisfaction of energy bounds, confirming the viability of the developed model containing ordinary matter. In Fig. 13 (left), the interior redshift is presented, decreasing with increasing r and reaching a value at the boundary as $z(0.18)=0.855,~z(0.52)=0.311$ and $z(0.34)=0.971$ for $\alpha =0.2,~0.3$ and 0.4,  respectively. The cracking condition, displayed in the right plot, takes negative values within the range $(-1,0)$ everywhere in the interior. Consequently, our developed solution for the constraints (34) and (48), is deemed stable, consistent with [68].

Fig. 10

Metric functions $e^{\eta _1}$ (red) and $e^{-\eta _2}$ (blue) for $\alpha =0.2$ (upper left), 0.3 (upper right) and 0.4 (lower) corresponding to Model III

Fig. 11

Matter variables and anisotropy for $\alpha =0.2$ (red), 0.3 (blue) and 0.4 (black) corresponding to Model III

Fig. 12

Energy bounds for $\alpha =0.2$ (red), 0.3 (blue) and 0.4 (black) corresponding to Model III

Fig. 13

Redshift and cracking for $\alpha =0.2$ (red), 0.3 (blue) and 0.4 (black) corresponding to Model III

6 Conclusions

The objective of this article is to propose various solutions to the Einstein field equations within the context of $f({\textbf{R}})$ theory. To achieve this goal, we have taken a static spherical interior metric into account, and determined both the modified field equations and the condition for a system to be in the hydrostatic equilibrium. Additionally, we have represented the spherical mass in terms of the geometric entity as well as the energy density. The exterior geometry has been defined by the Schwarzschild metric for calculating the compactness factor. Furthermore, we have orthogonally split the curvature tensor, leading to the derivation of four distinct scalars accompanying various physical quantities. It was observed that a factor denoted as ${\mathcal {Y}}_{TF}$ incorporates density inhomogeneity, anisotropy and modified corrections, prompting its adoption as the complexity factor for the considered fluid distribution, in line with Herrera’s proposed definition [46].

As the field equations (9)–(11) involve five unknowns, specifically, a fluid triplet and metric potentials, therefore, we have introduced certain constraints to facilitate the solvability of the system. The first constraint adopted is the complexity-free condition as expressed in Eq. (34). Additionally, we have implemented three constraints, namely, $p_r=0,$ a polytropic and a non-local equation of state, serving as the second condition, thereby resulting in different models. The geometric sector $(\eta _1,\eta _2)$ has been solved by integrating the corresponding equations in each scenario through numerical approach, along with specified feasible initial conditions. Subsequently, we have outlined several physical conditions such as the gravitational redshift, compactness and stability criteria, the fulfillment of which yields realistic self-gravitating models.

The matter sector, encompassing the energy density and pressure, associated with each solution, exhibits acceptable behavior, characterized by a maximum at $r=0$ and a subsequent decrease outward. Both the compactness and interior redshift have been observed to be within the acceptable limits. All solutions have met the viability criterion except one corresponding to a polytropic equation of state only for $\alpha =0.4,$ as evidenced by the satisfaction of energy conditions (Figs. 3, 7 and 12). Notably, the cracking condition is satisfied only by solutions corresponding to $p_r=0$ and a non-local equation of state for all the chosen values of the model parameter (Figs. 4 and 13). However, the second solution is stable only for $\alpha =0.2$ and 0.3 because we have observed the occurrence of cracking for $\alpha =0.4$ (Fig. 8). It is important to note that our derived solutions for the first and third models comply with those presented in [68]. However, the second and third models are found to deviate from the charged scenario [55].