1 Introduction

Among the large variety of gravity theories, only a few satisfy two essential criteria: (i) adherence to diffeomorphism invariance, and (ii) strict adherence to the strong equivalence principle. Notable examples include General Relativity, commonly referred to as GR, metric-affine gravity theories, and others (see [1,2,3] and references therein). In addition to the above, General Relativity has other attributes that confirm its prominent status as the favoured theory of gravity. The convincing detection of gravitational waves in 2015 stands out as a robust empirical support for the credibility of GR, as demonstrated by numerous studies [4, 5]. Moreover, GR has the versatility to incorporate the concept of dark energy, which underlies the accelerated expansion of the universe. In addition to its empirical support, the mathematical elegance of GR remains a highly valued aspect of the theory. Its ability to elegantly unify the concepts of spacetime and gravity is particularly appreciated for its simplicity and aesthetic appeal. However, despite of that, there are reasons to investigate alternative theories of gravity. From a theoretical point of view, GR manifests at least two strong problems: (i) the presence of singularities [6, 7], and (ii) the impossibility of renormalization (following standard processes of quantization) [8]. The observational evidence (i.e., the discovery of the dark sectors of the Universe) forces us to go beyond, reconsidering fundamental physics. In such a sense, any alternative self-consistent theory of gravity should connect both the ultraviolet and the infrared sectors, while preserving the main features of GR.

In this regard, there has been a recent growing interest in Extended Theories of Gravity (ETGs hereinafter) [2, 9, 10]. In particular, special attention has been devoted to f(R) [11, 12] and scalar-tensor theories [13], as quantum theories of gravity can be effectively described in the low-energy regime by them [14,15,16,17]. In addition, these theories have been consistently implemented in the cosmological context [18,19,20], as they naturally exhibit an inflationary behaviour [21,22,23,24] and lead to realistic dark energy [25,26,27,28].

On one hand, the gravitational action for f(R) theories of gravity takes the form (see [11, 12] and references therein):

$$\begin{aligned} S[g_{\mu \nu }]=\frac{1}{2 \kappa } \int d^{4} x \sqrt{-g} \ f(R) + S_{\text {matter}}, \end{aligned}$$
(1)

where f(R) is a real-valued function, \(\kappa \equiv 8 \pi G_0 c^{-4}\), \(G_0 \equiv 1\) is the gravitational constant, \(c\equiv 1\) is the speed of light in vacuum, and the rest of symbols have the usual meaning: \(g_{\mu \nu }\) is the metric field, g is the determinant of the metric field and R is the Ricci scalar.

Applying the variational metric formalism (which will be assumed along the rest of the manuscript), we get the corresponding equations of motion

$$\begin{aligned} F(R) R_{\mu \nu }-\frac{1}{2} f(R) g_{\mu \nu }+\big [g_{\mu \nu } \square -\nabla _{\mu } \nabla _{\nu }\big ] F(R)= 8 \pi T_{\mu \nu }, \end{aligned}$$
(2)

where \(F(R) \equiv \frac{d f}{d R} (R)\).

On the other hand, the general scalar-tensor gravitational action takes the form (see [13] and references therein)

$$\begin{aligned} \begin{aligned} S[g_{\mu \nu }, \phi ]&= \frac{1}{2\kappa } \int d^{4} x \sqrt{-g} \ \Bigg [ f(\phi ) R \ \\&\quad - \frac{\omega (\phi )}{2} g^{\alpha \beta } \nabla _\alpha \phi \nabla _\beta \phi -V(\phi ) \Bigg ] + S_{\text {matter}}, \end{aligned} \end{aligned}$$
(3)

where \(\phi \) is a scalar field and \(\omega (\phi ), V(\phi )\) are arbitrary functions representing a coupling function and a potential respectively. It’s worth noting that the connection of f(R) theories with scalar tensor theories, as discussed in the references [29,30,31], has allowed a reinterpretation of the equations of motion. This reinterpretation, in turn, has simplified the calculation of the Newtonian and post-Newtonian limits. Although it was initially suggested that such an equivalence might be questionable, the essential point is that if \(f'(R)\) is an invertible function and there is an inverse relation, denoted \(R(f')\), it becomes possible to construct the scalar field potential, denoted \(V (\phi )\). Consequently, this allows the representation of f(R) theories as scalar tensor theories of gravity. The most iconic example of these theories is the Brans–Dicke theory [33, 34], corresponding to \(f(\phi )\equiv \phi \), \(\omega (\phi ) \equiv \omega _0/\phi \) (with \(\omega _0\) a constant), and \(V(\phi ) \equiv 0\).

As shown in [32], particular f(R) and Brans–Dicke theories are formally related. Specifically, a given f(R) theory can be represented as an \(\omega = 0\) Brans–Dicke theory if \(f'(R)\) is invertible. The reciprocal identification requires the scalar-tensor equation of motion \(R = dV/d\phi \) to be invertible in order to obtain \(\phi (R)\) [32].

Thus, in light of previous comments, the purpose of this work is to expand this classification of ETGs by studying the equivalence between f(R) theories and scale-dependent gravity, a recent ETG that promotes the couplings of the classical gravitational action to scale-dependent quantities [35, 36], strongly inspired by asymptotically safe gravity.

This work is organized as follows: After this compact introduction, we summarize, in Sect. 2, the main idea behind scale-dependent gravity, whereas in Sect. 3 we show that every f(R) theory can be represented as a scale-dependent theory in two different ways: (i) at the level of the action and (ii) at the level of the field equations. Subsequently, in Sect. 4, we show two counterexamples in which scale-dependent solutions are not expressible as solutions of f(R) gravity, thus proving that f(R) formalism is strictly contained in the scale-dependent framework. After that, we exhibit some applications of the aforementioned relation between f(R) and scale-dependent gravity, in Sect. 5. In the same section, we also devote a few paragraphs to the study of light rings in light of the scale-dependent formalism, highlighting the relationship between such a concept and Gaussian curvature. Finally, in Sect. 6, we summarize our main findings.

2 A brief account of scale-dependent gravity

Scale-dependent gravity is an alternative approach to extend/find novel solutions commonly ignored in GR. In particular, we should reinforce that: (i) it introduces running constants, which justify why we find novel solutions, (ii) asymptotically safe gravity and scale-dependent gravity are consistent in the IR (IR instability) and (iii) a standard feature strongly inspired by particle physics is that quantum features can be parameterized as running couplings, as occurs, for instance in renormalization “improved” black hole solutions. However, our case does not require knowledge of the renormalization scale k in terms of \(x^\mu \), which is, of course, a natural advantage.

Let us start by considering the effective gravitational action, \(S[g_{\mu \nu }, k]\), for scale-dependent gravity which takes the form [36, 37]

$$\begin{aligned} S[g_{\mu \nu }, k] \equiv \int \textrm{d}^4 x \sqrt{-g} \Bigg [ \frac{1}{2 \kappa _k} \Bigl (R-2\Lambda _k \Bigl ) \Bigg ] + S_{\text {matter}}, \end{aligned}$$
(4)

where k is a scale-dependent field related to a renormalization scale, \(\kappa _k \equiv 8\pi G_k\) is the Einstein coupling, \(G_k\) and \(\Lambda _k\) refer to the scale-dependent gravitational and cosmological couplings, respectively, and the rest of the symbols have the usual meaning previously mentioned.

Taking variations with respect to the metric field, \(g_{\mu \nu }\), and the renormalization scale, k, we obtain: (i) the effective Einstein’s field equations and (ii) a consistent relation to close the system (conventionally referred to as a consistency equation). In this way, the effective equations of motion are [35,36,37,38,39]

$$\begin{aligned} G_{\mu \nu } + \Lambda _k g_{\mu \nu } + \Delta t_{\mu \nu } = 8 \pi G(x) T_{\mu \nu }. \end{aligned}$$
(5)

As is well-known, the tensor \(\Delta t_{\mu \nu }\) contains the scale-dependence of the gravitational coupling and it is defined as

$$\begin{aligned} \Delta t_{\mu \nu }&= G_k \big [ g_{\mu \nu } \square - \nabla _{\mu } \nabla _{\nu } \big ] G_k^{-1}. \end{aligned}$$
(6)

Be aware and notice that k(x), the auxiliary scalar field, is a real physical scale, usually identified as a momentum scale of any spacetime point, \(x^\mu \). Thus, the dependence of k on the physical coordinates is not unique. We will bypass the problem by considering that functions depending on k inherit a dependence on \(x^\mu \). In such a sense, we will consider that the functions \(\Lambda _k\) and \(G_k\) inherit the dependence on the spacetime coordinates from the spacetime dependence of the scale field, k(x). Thus, those couplings can be written as G(x) and \(\Lambda (x)\) [36, 40].

3 Scale-dependent representation of f(R) theories

In this section, we will consider the aforementioned formal relation between f(R) and scale-dependent gravities: (i) at the level of the action, and (ii) at the level of the field equations.

3.1 Analysis at the level of the action

Examining Eqs. (1) and (4) we see that the action of an f(R) theory can be represented by a scale-dependent theory by defining

$$\begin{aligned} \left\{ \begin{aligned}&G(x) \equiv \alpha (x),\\&\Lambda (x) \equiv \frac{1}{2} \bigg [ R(x) - \alpha (x) f(R(x)) \bigg ], \end{aligned} \right. \end{aligned}$$
(7)

where \(\alpha (x)\) is a sufficiently regular but arbitrary function.

Albeit it seems to be trivial, the reciprocal identification requires that the given scale-dependent theory fulfills

$$\begin{aligned} {\mathbb {F}}(x) \equiv \frac{R-2 \Lambda (x)}{G(x)} , \end{aligned}$$
(8)

which can be expressed as a function of the Ricci scalar, R,

$$\begin{aligned} {\mathbb {F}}(x) = {\mathbb {F}}(R(x)). \end{aligned}$$
(9)

In such a case, the scale-dependent gravitational action is equivalent to that of an f(R) theory with

$$\begin{aligned} f(R) \equiv {\mathbb {F}}(R). \end{aligned}$$
(10)

3.2 Analysis at the level of the field equations

Observe that representing a f(R) theory as a scale-dependent one alters the variation of the action with respect to the metric. This is because of the identification given by Eq. (7), where f(R(x)) acts a non-dynamical function, depending on the spacetime point.

This observation also applies to the reciprocal relation, as G and \(\Lambda \) are considered functions of the Ricci scalar, and not just non-dynamical functions. Therefore, an additional analysis at the level of the field equations is required.

A convenient reformulation of the f(R) equations of motion will allow a direct comparison with those of scale-dependent gravity. In particular, it is possible to restate the field equations for f(R) theories, Eq. (2) as

$$\begin{aligned} {\tilde{G}}_{\mu \nu } + \Delta {\tilde{t}}_{\mu \nu }&= 8 \pi F^{-1}(R) T_{\mu \nu } , \end{aligned}$$
(11)

where we have defined two auxiliary tensors defined as

$$\begin{aligned} {\tilde{G}}_{\mu \nu }&= R_{\mu \nu }-\frac{1}{2} \frac{f(R)}{F(R)} g_{\mu \nu } , \end{aligned}$$
(12)
$$\begin{aligned} \Delta {\tilde{t}}_{\mu \nu }&= F^{-1}(R) \bigg [ g_{\mu \nu } \square -\nabla _{\mu } \nabla _{\nu } \bigg ] F(R) , \end{aligned}$$
(13)

being (i) \({\tilde{G}}_{\mu \nu }\) an Einstein-like tensor, (ii) \(\Delta {\tilde{t}}_{\mu \nu }\) a tensor which accounts variation of F(R), and (iii) \(F(R) \equiv df(R)/dR\).

At this point, a straightforward comparison of the formal structure of Eqs. (11) and (5) shows that the equations of motion of an f(R) theory are equivalent to those of a scale-dependent theory. This can be seen to be true by taking the following identification,

$$\begin{aligned} \left\{ \begin{aligned}&G(x) \equiv F^{-1}(R(x)), \\&\Lambda (x) \equiv \frac{1}{2} \bigg [ R(x) - F^{-1}(R(x)) f(R(x)) \bigg ], \end{aligned} \right. \end{aligned}$$
(14)

In order to identify the equations of motion of a scale-dependent theory with those of an f(R) theory, notice that we should invert the relation given by Eq. (14). Thus, it is first required that G(x) and \( \Lambda (x)\) can be represented as a function of R, so,

$$\begin{aligned} \left\{ \begin{aligned}&G(x) = G(R(x)), \\&\Lambda (x) = \Lambda (R(x)). \end{aligned} \right. \end{aligned}$$
(15)

It is also mandatory, from Eq. (14), that

$$\begin{aligned} \frac{\textrm{d}}{\textrm{d} R}{\mathbb {F}}(R)=\frac{1}{G(R)}. \end{aligned}$$
(16)

Under these conditions, the equations of motion of such a scale-dependent theory are equivalent to those of an f(R) given by Eq. (10).

From the above discussion, we thus conclude one of the main points of this work: every f(R) theory can be represented as a scale-dependent theory. The reciprocal identification requires conditions, given by Eqs. (15) and (16), or just the weaker restriction, Eq. (9), if the identification is made at the level of the action.

These considerations allow us to include scale-dependent gravity into the already known classification of well-established ETGs [11], represented in Fig. 1. In this figure, the labels of each connection represent the assumptions needed to identify each formalism.

Finally, let us know that the problems of the appearance of singularities and renormalization depend on either a breakdown of the action or a change in the formulation of the action. Then, it is not a given fact that both f(R) and scale-dependent representations remain equivalent at this levelFootnote 1

Fig. 1
figure 1

Partial classification of some ETGs. See text for details

Full size image

4 Strict inclusion of f(R) gravity in the scale-dependent framework

In the previous section, we proved that every f(R) theory can be represented as a scale-dependent theory. At the same time, the reciprocal identification requires conditions, Eqs. (15) and (16), or just the weaker restriction, Eq. (9), at the level of the action. In this section we will show examples of scale-dependent gravity solutions not fulfilling the mentioned conditions, thus demonstrating that the f(R) formalism is strictly contained in the scale-dependent framework. In particular, we will focus on scale-dependent Schwarzschild–de Sitter and scale-dependent polytropic black holes.

4.1 Scale-dependent Schwarzschild–de Sitter black hole

The first example is the scale-dependent Schwarzschild–de Sitter black hole, originally obtained in [41] and revisited in [42]. Such a black hole solution is described by the line element

$$\begin{aligned} \textrm{d} s^{2}=-f(r) \textrm{d} t^{2}+f(r)^{-1} \mathrm {~d} r^{2}+r^{2}d \Omega ^2, \end{aligned}$$
(17)

where \(d \Omega ^2\) is the metric on the unit two-sphere and the lapse function is defined as

$$\begin{aligned} \begin{aligned} f(r)&= f_{0}(r) + \frac{1}{2} \epsilon \bigg [6 M-2 r +3 r \epsilon \left( r-4 M\right) \\&\quad + 2 r^{2} \epsilon \left( 1+6 M \epsilon \right) \ln \left( 1+\frac{1}{r \epsilon }\right) \bigg ]. \end{aligned} \end{aligned}$$
(18)

Here, \(f_0(r)\) corresponds to the classical Schwarzschild–de Sitter solution,

$$\begin{aligned} f_{0}(r)=1-\frac{2 M}{r}-\frac{1}{3} \Lambda _0 r^{2}, \end{aligned}$$
(19)

where \(M, \Lambda _0\) are constants associated with the classical mass and cosmological coupling, respectively.

The corresponding Newton’s coupling takes the form

$$\begin{aligned} G(r)=\frac{1}{1 + \epsilon r}, \end{aligned}$$
(20)

where \(\epsilon >0\) is a constant that acts as a running parameter measuring the deviation of the new solution with respect to its classical counterpart. In this way, for \(\epsilon \rightarrow 0\), the standard Schwarzschild–de Sitter solution is recovered.

To study if the above-mentioned solution can be represented as an f(R) solution, we will analyze the concrete form of the Ricci scalar, which is computed to be

$$\begin{aligned} \begin{aligned} R&= \frac{\epsilon }{r^2(1 + \epsilon r)^2}\bigg (6 r + 6 M(1 + 2 \epsilon r) \\&\quad \times (-1 + 6 \epsilon r ( 1 + \epsilon r)) + r^2 \epsilon (1 - 6 \epsilon r \big ( 4 + 3 \epsilon r)\big ) \\&\quad -12 r^2 \epsilon (1 + 6 M \epsilon ) ( 1 + \epsilon r)^2 \ln \left[ 1+\frac{1}{\epsilon r}\right] \bigg ) + 4 \Lambda _0. \end{aligned} \end{aligned}$$
(21)

At this point we should check both G(r) and R(r) in order to verify that G(r) in (20) is one-to-one, however, R(r) in (21) is not. In fact, by computing the first and second derivatives we have

$$\begin{aligned} \frac{\textrm{d} R}{\textrm{d} r} = -\frac{2 \epsilon \big (r^2 \epsilon (r \epsilon +3)+3r -6 M \big )}{r^3 (r \epsilon +1)^3}, \end{aligned}$$
(22)

which has one positive root \({\bar{r}}\) provided that \(M>0\). Besides,

$$\begin{aligned} \frac{\textrm{d}^2R}{\textrm{d}r^2}\Bigg |_{r={\bar{r}}} =-\frac{6 \epsilon ^4}{\root 3 \of { (6 M \epsilon +1)} \left( \root 3 \of { (6 M \epsilon +1)}-1 \right) ^3}<0, \end{aligned}$$
(23)

so \({\bar{r}}\) is a local maximum and R(r) fails to be injective.

It is thus deduced that, in such a case, the solution for the gravitational coupling can not be expressed as a function of the Ricci scalar. Therefore, following the exposed reasoning, this scale-dependent theory can not be identified (in general) with an f(R) theory at the field equations level.Footnote 2

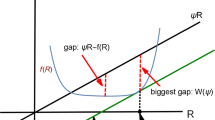
To confirm that the link between scale-dependent gravity and f(R) gravity is not possible to achieve, even at the level of the action, it is enough to find two points, \(r_1\) and \(r_2\), such that

$$\begin{aligned} {\mathbb {F}}(r_1)&\ne {\mathbb {F}}(r_2), \end{aligned}$$
(24)

but

$$\begin{aligned} R(r_1) = R(r_2) , \end{aligned}$$
(25)

as in that case \({\mathbb {F}}(r)\) could not be expressed as a function of the Ricci scalar. This behaviour can be explicitly shown in Fig. 2 for certain \(\{ M, \Lambda _0, \epsilon \}\).

Fig. 2
figure 2

R(r) and \( {\mathbb {F}}(r)\) for fixed parameters \( M = 1\), \(\epsilon = 1\), \(\Lambda _0 = 5\). See text for details

Full size image

4.2 Scale-dependent polytropic black hole

The second example will be the scale-dependent polytropic black hole, originally described in [43]. It also considers a spacetime endowed with a metric as Eq. (17), in the presence of a polytropic gas following the original paper [44, 45]. Thus, in the scale-dependent scenario, the solution has a scale-dependent gravitational coupling again of the form of Eq. (20), where

$$\begin{aligned} \begin{aligned} f(r)=&f_0(r) + 6 M r^2 \epsilon ^3 \ln \left[ 2 M \frac{r \epsilon +1}{r}\right] \\&+3 M \epsilon (1-2 r \epsilon ), \end{aligned} \end{aligned}$$
(26)

and \(f_0(r)\) corresponds to the classical polytropic black hole solution, given by

$$\begin{aligned} f_0\left( r, P_0\right) =-\frac{\Lambda _0 r^2}{3}-\frac{2 M}{r}. \end{aligned}$$
(27)

Again, to study if this scale-dependent solution can be represented as an f(R) solution, we analyze the explicit form of the Ricci invariant, which is computed to be

$$\begin{aligned} \begin{aligned} R =&\frac{2}{r^2} \left( \frac{3 M \epsilon \ (2 r \epsilon +1) \ (6 r \epsilon \ (r \epsilon +1)-1)}{(r \epsilon +1)^2}+1\right) \\&-72 M \epsilon ^3 \ln \left( 2 M \left( \frac{1}{r}+\epsilon \right) \right) + 4 \Lambda _0, \end{aligned} \end{aligned}$$
(28)

which again can be checked not to be injective in general as a function of r. Therefore, the argument of the previous example does apply.

Furthermore, even neglecting this argument and assuming that Eq. (15) is satisfied, it can be shown that condition (16) would not be met in general. Applying the chain rule, (16) is equivalent toFootnote 3

$$\begin{aligned} \frac{\textrm{d}{\mathbb {F}}}{\textrm{d} r} \left( \frac{\textrm{d}R}{\textrm{d}r} \right) ^{-1} = {G(r)}^{-1}. \end{aligned}$$
(29)

This relation applied to the scale-dependent polytropic black hole solution, results in

$$\begin{aligned} \frac{r \epsilon (r \epsilon +1)^3}{6 M \epsilon -2 (r \epsilon +1)^3} = 0, \end{aligned}$$
(30)

which is not true for \(\epsilon > 0\).

Starting from the action, we can also show that this scale-dependent solution can not (in general) be identified with an f(R) solution. Similarly to the previous example, this can be confirmed by observing that at certain points, \(r_1,r_2\), the curvature scalar, R(r), is not injective, but \({\mathbb {F}}(r)\) is, as Fig. 3 reveals.

Fig. 3
figure 3

R(r) and \( {\mathbb {F}}(r)\) for fixed parameters \( M = 0.5\), \(\epsilon = 1\), \(\Lambda _0 = 5\)

Full size image

Therefore, up to this point, the main message is that every f(R) theory can be represented as a scale-dependent theory but the reciprocal identification is not true in general. Thus, the f(R) formalism is strictly included in the scale-dependent framework.

5 Applications in spherically symmetric and static spacetimes

The scale-dependent representation of f(R) gravity already shown suggests an alternative physical reinterpretation of f(R) theories. Moreover, it allows for a novel approach to face gravitational problems in f(R) gravity which we proceed to exhibit now.

We consider henceforth spherically symmetric and static spacetimes described by the line element

$$\begin{aligned} ds^2 = -f(r)dt^2 + g(r)dr^2+r^2d\Omega . \end{aligned}$$
(31)

Employing a scale-dependent inspired strategy, detailed in Sect. 5.1, we will obtain a new geometric characterization of light rings in Sect. 5.2. In addition, we will introduce a compact derivation of the general solution in f(R) for \(fg = 1\), physically interpreted via its scale-dependent representation, in Sect. 5.3. Finally, we will present some previously unknown scale-dependent solutions, obtained from recognized f(R) solutions, in Sect. 5.4.

5.1 Scale-dependent inspired strategy

Following a straightforward argument pointed out in [77], the contraction of the Ricci tensor with radial null vectors vanishes iff fg is constant.

This remark has been applied in the scale-dependent framework to obtain the general solution for \(fg=1\) in vacuum [37, 40,41,42,43, 50,51,52,53,54,55,56,57,58,59,60,61,62,63,64,65,66,67,68,69,70,71,72,73,74,75,76]. By contracting the field equations (5) with radial null vectors \(l^{\mu }\), one arrives to the non-trivial differential equation

$$\begin{aligned} \Delta t_{\mu \nu } l^{\mu } l^{\nu } = 0. \end{aligned}$$
(32)

We proceed to extend this strategy to f(R) gravity, considering this time arbitrary null vectors, \(n^{\mu }\). In this way, we contract the vacuum field equations (11) with \(n^{\mu }\), obtaining

$$\begin{aligned} R_{\mu \nu } n^{\mu } n^{\nu } = -\Delta {\tilde{t}}_{\mu \nu } n^{\mu } n^{\nu }. \end{aligned}$$
(33)

Considering (31), the arbitrary null vector components are \((n^t, n^r, n^{\theta }, n^{\phi })\), satisfying

$$\begin{aligned} n^{t} = \sqrt{\frac{\bigl (n^{r}\bigl )^{2} g(r)+\bigl (n^{\theta }\bigl )^{2} r^{2} + \bigl (n^{\phi }\bigl )^{2} r^{2} \sin ^{2}\theta }{f(r)}}. \end{aligned}$$
(34)

Using this notation, the l. h. s and the r. h. s. of Eq. (33), take the form

$$\begin{aligned}{} & {} \begin{aligned} R_{\mu \nu } n^{\mu } n^{\nu }&= \left( {n^{r}} \right) ^{2} \frac{1}{r} \frac{d}{d r}\left( \ln f g \right) \\&\quad + \left[ {(n^{\theta })}^{2}+{(n^{\phi })}^{2} \sin ^{2} \theta \right] \frac{1}{4 r(f g)^{2}} \\&\quad \times \left( -r^{3} {f^{\prime }}^2 g + 4 r f^{2} g(g - 1) +2 r^{2} f^{2} g^{\prime } \right. \\&\quad \left. -\frac{1}{2} r^{3} \left( f^{2}\right) ^{\prime } g^{\prime }+2 r^{2} f \left( r f^{\prime }\right) ^{\prime } g \right) ,\\ \end{aligned} \end{aligned}$$
(35)
$$\begin{aligned}{} & {} \begin{aligned} \left( -\Delta {\tilde{t}}_{\mu \nu }\right) n^{\mu } n^{\nu }&= \left( {n^{r}} \right) ^{2}\left( \frac{F^{\prime \prime }}{F} -\frac{F^{\prime }}{2F} \frac{d}{d r}(\ln f g)\right) \\&\quad + \left[ {(n^{\theta })}^{2}+{(n^{\phi })}^{2} \sin ^{2} \theta \right] \\&\quad \times \left( \frac{r F^{\prime }\left( 2 f - r f^{\prime }\right) }{2 F f g}\right) .\\ \end{aligned} \end{aligned}$$
(36)

We have two natural ways to make use of this contracted equation: (i) by taking radial null vectors (\(n^{\theta } = 0 = n^{\phi }\)) and, (ii) by taking angular null vectors (\(n^{r} = 0\)).

Considering case (i) yields

$$\begin{aligned} F''(r) + a(r) F'(r) + b(r) F(r) = 0, \end{aligned}$$
(37)

where the functions a(r) and b(r) are auxiliary quantities defined as:

$$\begin{aligned} a(r)&= -\frac{1}{2} \frac{\textrm{d}}{\textrm{d}r}\ln \Bigl (f(r)g(r)\Bigl ), \end{aligned}$$
(38)
$$\begin{aligned} b(r)&= -\frac{1}{r} \frac{\textrm{d}}{\textrm{d}r}\ln \Bigl (f(r)g(r)\Bigl ). \end{aligned}$$
(39)

Regarding case (ii), let us introduce the Ricci curvatures corresponding to the \((\theta , \phi )\), (t, r) and \((r, \theta )\) sectors, denoted as \({ }^{(2)} R\), \( { }^{(2)} {\tilde{R}}\), and \({ }^{(2)} {\hat{R}}\), respectively, as

$$\begin{aligned}&{ }^{(2)}R = \frac{2}{r^2}, \end{aligned}$$
(40)
$$\begin{aligned}&{ }^{(2)} {\tilde{R}} = - \frac{f''}{fg} + \frac{f'g'}{2 f g^2} + \frac{\left( f' \right) ^2}{2 f^2 g}, \end{aligned}$$
(41)
$$\begin{aligned}&{ }^{(2)} {\hat{R}} = \frac{g'}{r g^2}. \end{aligned}$$
(42)

Using this notation, we obtain for case (ii) the relation

$$\begin{aligned} { }^{(2)} {\hat{R}} - { }^{(2)} {\tilde{R}}\ + \delta \ { }^{(2)} R&= 0, \end{aligned}$$
(43)

where we have defined the auxiliary function, \(\delta \), as follows

$$\begin{aligned} \delta&\equiv 1 - \frac{1}{2 fg}\Bigl (2f - rf'\Bigl ) \ \left( 1 + r \frac{\textrm{d}}{\textrm{d}r} \ln (F)\right) . \end{aligned}$$
(44)

This scale-dependent inspired procedure of contracting the field equations with null vectors, provides us with two equations: (37) and (43). Interestingly, for \(f g = 1\) these will allow us to obtain a new geometric characterization of light-rings and a compact derivation of the general solution. In the general case, with fg non-constant, the trace of Eq. (11), given by

$$\begin{aligned} F(R) R - 2 f(R) + 3 \square F(R) = 0, \end{aligned}$$
(45)

is required to close the system. That is, Eqs. (37), (43) and (45) are equivalent to the original field equations.Footnote 4

5.2 Geometric characterization of light-rings

The field equation (43) and its auxiliary function \(\delta \), given by Eq. (44), encode valuable information related to the appearance of circular null geodesics (or light rings), which will be studied employing the optical metric. Note that the geodesics of this metric are the light rays and, therefore, they can be characterized by the vanishing of their geodesic curvature, which can be computed in the hyperplane \(\theta = \pi /2\), as pointed out in [78]. In the following we will employ a standard approach based on the effective potential.

Let us consider a spacetime described by Eq. (31) with \(f g =1\). For a null geodesic and taking \(\theta =\pi /2\) due to the spherical symmetry restriction, the corresponding geodesic equation reads

$$\begin{aligned} \dot{r}^2=L^2\left( \frac{1}{b^2}-\frac{f(r)}{r^2} \right) , \end{aligned}$$
(46)

where \(\dot{r} \equiv \frac{dx}{d\lambda }\) (\(\lambda \) being an affine parameter), \(b = \frac{L}{E}\) is the impact parameter, \(E = f \dot{t}\) and \(L = r^2 {\dot{\phi }}\) being the photon’s conserved energy and angular momentum, respectively). The effective potential is given by

$$\begin{aligned} V(r)=\frac{f(r)}{r^2}. \end{aligned}$$
(47)

The circular null geodesics are obtained then when \(V'(r)=0\), which corresponds to \(2 f(r) -r f' (r) =0\).

With these considerations, Eq. (44) can be expressed as

$$\begin{aligned} \delta&= 1 - \frac{1}{2 r^3 fg} \ V'(r) \ \left( 1 + r \frac{\textrm{d}}{\textrm{d}r} \ln (F)\right) . \end{aligned}$$
(48)

Therefore, for f(R) theories such that

$$\begin{aligned} F(R(r))\ne \alpha r^{-1}, \end{aligned}$$
(49)

Equation (43) provides a new geometric characterization of light rings: for any gravitational theory satisfying Eq. (49), a circular curve is a light ring iff

$$\begin{aligned} { }^{(2)} {\hat{R}}+ { }^{(2)} R - { }^{(2)} {\tilde{R}}=0. \end{aligned}$$
(50)

At this point we would like to comment that the light ring condition previously mentioned (\(V'(r) = 0\)) is a purely kinematic effect; therefore, in principle, no field equations are involved within their definition. In this sense, it appears surprising that, within the aforementioned f(R) theories (which contain GR), the existence of light rings can be traced back to the very same field equations, given by Eq. (50).

Note that the light ring condition resembles somehow an equilibrium of curvatures between different spacetime sectors in a similar way that the event horizon can be defined through the corresponding equilibrium condition. Specifically, after decomposing the Riemann curvature into its Weyl and Ricci parts, it is possible to write both Eq. (50) and the horizon condition, given by \(g(r_{H})^{-1}=0\), using the so-called Newman–Penrose scalars (the interested reader can consult [47] for a Newman–Penrose formulation of the horizon condition related to horizon thermodynamics or [48] for a study of light rings in static and extremal black holes using the Newman–Penrose calculus). In the case of light rings, in order to interpret Eq. (50), here we will present only the final result of our rewriting without showing the whole calculations, which will reported elsewhere.

It turns out that the light ring condition can be equivalently written in any of the three following ways

$$\begin{aligned}{} & {} 2 f(r) - r f'(r) = 0 \end{aligned}$$
(51)
$$\begin{aligned}{} & {} { }^{(2)} {\hat{R}}+ { }^{(2)} R - { }^{(2)} {\tilde{R}}=0 \end{aligned}$$
(52)
$$\begin{aligned}{} & {} K -K^*-\left( \Psi _{2}+ 2 \Lambda \right) =0. \end{aligned}$$
(53)

At this point, some comments are in order. Let us consider a null tetrad, \(\{l,n,m,{{\bar{m}}}\}\). Then, we have: (i) For spherically symmetric spacetimes, K and \(K^*\) are given by \(K= \rho ^2 - \Psi _{2}+\Phi _{11}+ \Lambda \) and \(K^*=-\Psi _{2}-\Phi _{11}+\Lambda \), where \(\rho \) stands for the expansion of a null geodesic congruence, \(\Psi _{2}=C_{\alpha \beta \gamma \delta }l^{\alpha } m^{\beta } {{\bar{m}}}^{\gamma } n^{\delta }\) and \(\Phi _{11}=-\frac{1}{2}R_{\alpha \beta }l^{\alpha } n^{\beta }+ 3 \Lambda \), being \(C_{\alpha \beta \gamma \delta }\) and \(R_{\alpha \beta }\) the Weyl and Ricci tensor, respectively, and \(\Lambda = \frac{R}{24}\), where \(R= g^{\alpha \beta }R_{\alpha \beta }\). (ii) The asterisk (Sachs) operation interchanges the l, n and \({m,{{\bar{m}}}}\) sectors of the geometry. Interestingly, the four-dimensional curvatures K and \(K^*\) are, in essence, the Gaussian curvatures of the (t, r) and \((\theta ,\phi )\) sectors, respectively. (iii) The quantity \(\Psi _{2}+ 2 \Lambda \) is proportional to the surface gravity of a spacelike two-surface [46]. In particular, it can be shown [47] that it represents the Komar energy density associated with a particular spacelike surface. And (iv), from these observations we can conclude that the light ring condition is an equilibrium condition between very precise curvatures with a physically relevant meaning, which naturally appear in the Newman–Penrose formalism.

5.3 Scale-dependent representation of the vacuum f(R) solution for the case \(fg = 1\)

As a starting point, let us note that in the context of f(R) gravity, the general solution for \(fg=1\) in vacuum was reported in Ref. [49]. However, its interpretation remained unclear, as noted by the authors, who wrote “It is, however, unclear whether these solutions correspond to maximally symmetric (spatial) spaces or to some other type of spherically symmetric (but nonsingular) cases” [49].

In the following lines we will show that the aforementioned solution to f(R) gravity can be represented as the scale-dependent Schwarzschild–de Sitter spacetime (Sect. 4.1), thoroughly studied along the literature [41, 79, 80].

For \(fg=1\) Eqs. (37) and (43) are substantially simplified, and the trace equation, Eq. (45) is not longer necessary. The radial null vector equation, Eq. (37), reduces to

$$\begin{aligned} F''(r) = 0, \end{aligned}$$
(54)

so that

$$\begin{aligned} F(r) = \alpha _0 + \epsilon r, \end{aligned}$$
(55)

where \(\alpha _0\) and \(\epsilon \) are arbitrary constants. While \(\epsilon \) controls the deviations from GR, in the sense that \(f(R) \rightarrow \alpha _0 R\) as \(\epsilon \rightarrow 0\), assuming \(\alpha _0 = 1\) is then equivalent to considering the convention \(G_0 \equiv 1\).

With this assumption, the angular null vector equation, Eq.(43), results in

$$\begin{aligned} \begin{aligned}&\frac{1}{r^{2}} + f(r)\left( -\frac{1}{r^{2}} - \frac{\epsilon }{r(1+\epsilon r)}\right) \\&\quad + f^{\prime }(r) \frac{\epsilon }{2(1+\epsilon r)}+\frac{1}{2} f^{\prime \prime }(r)=0, \end{aligned} \end{aligned}$$
(56)

whose general solution is given by Eq. (18), which corresponds with the scale-dependent Schwarzschild–de Sitter black hole solution, as previously claimed.

Therefore, the general solution of vacuum f(R) gravity (for \(f g = 1\)) [49] can be represented as a black hole embedded in a de Sitter-like Universe, where both the Newton and cosmological couplings, G and \(\Lambda \), are functions which “run” only with the radial coordinate, r.

5.4 Novel scale-dependent solutions

The link we have established between f(R) and scale-dependent gravity can be also used in benefit of the latter. In fact, the scale-dependent Schwarzschild de Sitter black hole was originally obtained using a specific (and arbitrary) choice for the constants that define Newton’s coupling. In this sense, the function \(G(r)=\frac{1}{1+\epsilon r}\) is usually considered to recover the usual GR limit when \(\epsilon \rightarrow 0\). However, from the point of view of f(R) gravity, it has been shown [49] that both the Schwarzschild de Sitter and the de Sitter spacetimes are solutions of \(f(R)=R+\Lambda \pm \frac{2}{3\,M} \sqrt{-R-2 \Lambda }\) and \(f(R)=\pm 2\sqrt{R-2\Lambda }\), respectively. Interestingly, following our previous discussions, it can be seen that both of them are also solutions to scale-dependent gravity for \(G(r)=\frac{1}{1\mp \frac{1}{3\,M} r}\) and \(G(r)= \mp \frac{1}{r}\). Therefore, we conclude by noting that both the Schwarzschild de Sitter and the de Sitter spacetimes are solutions of certain models of scale-dependent gravity, where non-standard features of the Newton coupling appear.

6 Concluding remarks

The main result of this work is that every f(R) theory of gravity can be represented as a scale-dependent theory, both at the level of the action and at the level of the field equations. This inclusion is strict, i.e., not every scale-dependent theory can be represented in the f(R) formalism, as exemplified in Sect. 4 through the scale-dependent Schwarzschild de Sitter and polytropic black hole solutions.

Next, we have exhibited the potential of this relation to tackle gravitational problems in both theories in Sect. 5, focusing on spherically symmetric and static spacetimes.

In the f(R) formalism we employed a scale-dependent inspired strategy to analyze its field equations, which resulted in two equalities, Eqs. (37) and (43). These provide all the information needed to characterise the \(fg = 1\) case. Interestingly, the first equation provides a geometric characterization of light rings, as an equilibrium between particular scalar curvatures, which has been further analyzed using the Newman–Penrose formalism. At the same time, Eqs. (37) and (43) provide a compact derivation of the general f(R) solution for \(fg = 1\). Even more, in line with the main point of this paper, we have shown a physical interpretation of this solution through its scale-dependent representation, which is the scale-dependent Schwarzschild–de Sitter black hole.

Lastly, we exhibited how the scale-dependent gravity representation of the f(R) framework can be used in favour of the former. In particular, we showed that the Schwarzschild–de Sitter and the de Sitter spacetimes, which are solutions of f(R) gravity, are also solutions to scale-dependent gravity. These solutions, not previously reported in the literature, exhibit non-standard gravitational couplings.