Wave-packet effects: a solution for isospin anomalies in vector-meson decay

Abstract

There is a long-standing anomaly in the ratio of the decay width for $\psi (3770)\rightarrow D^0\overline{D^0}$ to that for $\psi (3770)\rightarrow D^+D^-$ at the level of $9.5\,\sigma$. A similar anomaly exists for the ratio of $\phi (1020)\rightarrow K_\text {L}^0K_\text {S}^0$ to $\phi (1020)\rightarrow K^+K^-$ at $2.1\,\sigma$. In this study, we reassess the anomaly through the lens of a Gaussian wave-packet formalism. Our comprehensive calculations include the localization of the overlap of the wave packets near the mass thresholds and the composite nature of the initial-state vector mesons. The results align within a $\sim 1 \sigma$ confidence level with the Particle Data Group’s central values for a physically reasonable value of the form-factor parameter, indicating a resolution to these anomalies. We also check the deviation of a wave-packet resonance from the Briet–Wigner shape and find that wide ranges of the wave-packet size are consistent with the experimental data.

1 Introduction

There is a long-standing anomaly (discrepancy between experimental and theoretical results) in the ratio of the decay width for $\psi (3770)\rightarrow D^0\overline{D^0}$ to that for $\psi (3770)\rightarrow D^+D^-$. A similar but weaker anomaly exists for the ratio of $\phi (1020)\rightarrow K_\text {L}^0K_\text {S}^0$ to $\phi (1020)\rightarrow K^+K^-$. On the other hand, the ratio of $\Upsilon (4S)\rightarrow B^+B^-$ to $\Upsilon (4S)\rightarrow B^0\overline{B^0}$ is consistent with the standard theoretical predictions.

At the quark level, these processes are¹

$$\begin{aligned} \phi \!\left( s{\overline{s}}\right)&\rightarrow K^+\!\left( u{\bar{s}}\right) K^-\!\left( s{\bar{u}}\right) ,\\ \psi \!\left( c{\overline{c}}\right)&\rightarrow D^+\!\left( c{\overline{d}}\right) D^-\!\left( d{\overline{c}}\right) ,\\ \Upsilon \!\left( b{\overline{b}}\right)&\rightarrow B^+\!\left( u{\overline{b}}\right) B^-\!\left( b{\bar{u}}\right) ,\\ \phi \!\left( s{\overline{s}}\right)&\rightarrow K^0\!\left( d{\bar{s}}\right) \overline{K^0}\!\left( s{\bar{d}}\right) \rightarrow K^0_\text {L}K^0_\text {S},\\ \psi \!\left( c{\overline{c}}\right)&\rightarrow D^0\!\left( c{\overline{u}}\right) \overline{D^0}\!\left( u{\overline{c}}\right) ,\\ \Upsilon \!\left( b{\overline{b}}\right)&\rightarrow B^0\!\left( d{\overline{b}}\right) \overline{B^0}\!\left( b{\overline{d}}\right) , \end{aligned}$$

which can be summarized as $V\!\left( Q{\overline{Q}}\right) \rightarrow P\!\left( Q{\overline{q}}\right) +{\overline{P}}\!\left( q{\overline{Q}}\right)$, where V and P are vector and pseudo-scalar mesons, respectively, and Q and q are heavy (s, c, b) and light (u, d) quarks, respectively. This ratio of decay widths is theoretically clean because most of the quantum chromodynamics (QCD) corrections cancel out between the numerator and the denominator. These decay processes are via strong interaction, and hence in the limit of exact isospin symmetry $u\leftrightarrow d$, the ratio becomes unity. The isospin violation makes a deviation from unity.

We name the ratio of the widths as²

$$\begin{aligned} R_\phi&:= {\Gamma \!\left( \phi \rightarrow K^+K^-\right) \over \Gamma \!\left( \phi \rightarrow K_\text {L}^0K_\text {S}^0\right) },\nonumber \\ R_\psi&:= {\Gamma \!\left( \psi \rightarrow D^+D^-\right) \over \Gamma \!\left( \psi \rightarrow D^0\overline{D^0}\right) },\nonumber \\ R_\Upsilon&:= {\Gamma \!\left( \Upsilon \rightarrow B^+B^-\right) \over \Gamma \!\left( \Upsilon \rightarrow B^0\overline{B^0}\right) }. \end{aligned}$$

(1)

The experimental results are combined by the Particle Data Group (PDG) [1]:³

$$\begin{aligned} R_\phi ^\text {PDG}&= 1.45\pm 0.03,\nonumber \\ R_\psi ^\text {PDG}&= 0.798\pm 0.010,\nonumber \\ R_\Upsilon ^\text {PDG}&= 1.058\pm 0.024. \end{aligned}$$

(2)

The theoretical prediction of the decay rates for $V\rightarrow P^+P^-$ and $V\rightarrow P^0\overline{P^0}$ is based on the plane-wave formalism so far. The tree-level result of the chiral perturbation theory reads

$$\begin{aligned} R_V^\text {plane} = {g_{V+}^2\over g_{V0}^2}\left( m_V^2-4m_{P^+}^2\over m_V^2-4m_{P^0}^2\right) ^{3/2}, \end{aligned}$$

(3)

where $g_{V+}$ ($g_{V0}$) is the coupling between V and $P^+P^-$ ($P^0\overline{P^0}$) and $m_V$, $m_{P^+}$, and $m_{P^0}$ are the masses of V, $P^+$, and $P^0$, respectively. Even if we assume an isospin-symmetric coupling $g_{V+}=g_{V0}$, the difference in the pseudo-scalar-meson masses $m_{P^+}\ne m_{P^0}$ results in a deviation in $R_V$ from unity: Putting the mass values in Ref. [1],⁴ we obtain

$$\begin{aligned} R_\phi ^\text {plane}&= {g_{\phi +}^2\over g_{\phi 0}^2}\left( 1.5156\pm 0.0033\right) ,\nonumber \\ R_\psi ^\text {plane}&= {g_{\psi +}^2\over g_{\psi 0}^2}\left( 0.6915\pm 0.0046\right) ,\nonumber \\ R_\Upsilon ^\text {plane}&= {g_{\Upsilon +}^2\over g_{\Upsilon 0}^2}\left( 1.047\pm 0.026\right) . \end{aligned}$$

(4)

Comparing Eqs. (2) and (4), we see that the isospin-symmetric limit for the coupling $g_{V+}=g_{V0}$ results in the anomaly at the level of $2.1\,\sigma$, $9.5\,\sigma$, and $0.32\,\sigma$ for $\phi$, $\psi$, and $\Upsilon$, respectively.

We briefly review the theoretical accounts for the anomaly within plane-wave formalism. For $R_\phi$, it turned out that radiative corrections make the anomaly more significant [2]: The standard quantum electrodynamics (QED) corrections make the theoretical prediction of the ratio 4 % larger, and isospin-breaking corrections to the ratio $g^2_{\phi +}/g^2_{\phi 0}$ further make it “some 2 %” [2] larger, leading to a larger anomaly of roughly $5.2\,\sigma$ assuming that the error is dominated by that in Eq. (2). In Ref. [3], the authors introduce a smeared decay rate that is a function of the energy difference between the initial and final plane-wave states; this smearing is by the Lorentzian distribution due to the inclusion of the width and by a phenomenological form factor put by hand to regularize an ultraviolet (UV) divergence; the anomaly for $\phi$ can be explained with a mass parameter $M\simeq 1.5\,\text {GeV}$ in the phenomenological form factor. In Ref. [4], the authors have estimated the effects of the electromagnetic structure of kaons and other model-dependent contributions to the radiative corrections, and the resultant corrections have turned out to be tiny. In Ref. [5], two (a Breit–Wigner and a nonrelativistic Lorentzian) types of averaged decay widths over the initial-state energy are introduced with two phenomenologically chosen energy intervals, 1.010–1.060 GeV and 1.000–1.100 GeV, to relax the anomaly.

For $R_\psi$, another type of averaged decay width is introduced in Ref. [6], and the resultant anomaly has become even more significant. There is no explanation for this $9.5\,\sigma$ anomaly so far.

The above smearing/averaging over the energy provides significant effects because the decay $V\rightarrow P{\overline{P}}$ is near the threshold $m_V\simeq 2m_P$. In situations near the threshold, it is desirable to treat the decay more rigorously by using wave packets for the initial and final states. Recall that the S-matrix in the plane-wave formalism contains the energy-momentum-conserving delta function and is theoretically ill-defined when computing the probability rather than the rate. A well-defined decay probability can be calculated only as a transition from a wave packet to a pair of wave packets. This is theoretically more reliable.

In the previous analyses [3,4,5], it has been assumed that the transition processes are described by the (plane-wave) rates alone.⁵ In this paper, we present an analysis based on the transition probability of the normalized states and wave packets, without the divergence of the delta function squared. Concretely, we compute the decay $V\rightarrow P{\overline{P}}$ in the Gaussian wave-packet formalism [9,10,11,12,13]; see also Refs. [14,15,16].⁶ In particular, we include a wave packet effect, called the in-time-boundary effect for the decay, by simply limiting the time integral of the decay interaction point to $t>T_\text {in}$ [10]. Here, $T_\text {in}$ is the time from which the interaction is switched on. This procedure is proven to provide approximate modeling of the full production process of V in the corresponding two-to-two wave-packet scattering, say, $e^+e^-\rightarrow V\rightarrow P{\overline{P}}$ [13]; see also Refs. [11, 12, 20,21,22,23,24] for related discussions.

The organization of this paper is as follows: In Sect. 2, we will introduce the minimum basics of calculating the (generalized) S-matrix that describes wave-packet-to-wave-packet transitions considering the initial state’s decaying nature when wave packets take the Gaussian form. In Sect. 3, we will review significant properties of the Gaussian wave-packet S-matrix. In Sect. 4, we will compare the theoretical predictions for the ratios of $R_\phi$, $R_\psi$, and $R_\Upsilon$ in the wave-packet and the plane-wave formalisms taking into account the form factor of the vector mesons. In Sect. 5, we will discuss the constraint from the resonant shape in the electron positron collider experiments for $\phi$ and $\psi$. In Sect. 6, we will provide a summary and further discussions. In Appendix A, we will review the form factor details for vector mesons used for our analysis. In Appendix B, we will provide the details on how to derive the total probability of $V \rightarrow P \overline{P}$ under nonrelativistic approximations. In Appendix C, a brief review on how to derive the plane-wave decay rate for $V \rightarrow P \overline{P}$ will be provided. In Appendix D, we will comment on a specific formal limit where the wave-packet decay rate coincides with the plane-wave decay rate. In Appendix E, we will briefly consider the isospin violation on the $\rho$ system.

2 Basics of Gaussian wave-packet formalism

For the near-threshold decay, the velocities in the final state are small, and the overlap of the wave packets becomes more significant in general. Therefore, it is important to take them into account.

Here, we spell out how to compute the probability for the $V\rightarrow P{\overline{P}}$ decay in the Gaussian wave-packet formalism. Throughout this paper, we work in the natural units $\hbar =c=1$. Readers who are more interested in analyses of experimental results rather than detailed theoretical formulation may skim through this section.

2.1 Wave-packet S-matrix

In the Gaussian wave-packet formalism, a transition from an initial wave-packet state $\left| \mathcal{W}\mathcal{P}_0 \right\rangle$ to a two-body final wave-packet state $\left| \mathcal{W}\mathcal{P}_1,\mathcal{W}\mathcal{P}_2 \right\rangle$ is characterized by the following generalized S-matrix [9]:

$$\begin{aligned} S_{\mathcal{W}\mathcal{P}_0 \rightarrow \mathcal{W}\mathcal{P}_1\mathcal{W}\mathcal{P}_2}&= \left\langle \mathcal{W}\mathcal{P}_1,\mathcal{W}\mathcal{P}_2 | \, {\widehat{U}}\!\left( T_{\textrm{out}}, T_{\textrm{in}}\right) | \mathcal{W}\mathcal{P}_0 \right\rangle , \end{aligned}$$

(5)

where ${{\widehat{U}}}$ describes the unitary time evolution from the initial time $T_{\textrm{in}}$ to the final time $T_{\textrm{out}}$

$$\begin{aligned} {\widehat{U}}\!\left( T_{\textrm{out}}, T_{\textrm{in}}\right) := \text {T}\, \text {exp}\!\left( -i \int _{T_{\textrm{in}}}^{T_{\textrm{out}}} \text {d}t \,\int \text {d}^3{\varvec{x}}\, \widehat{ \mathcal{H} }_\text {int}^\text {(I)}\!\left( t,{\varvec{x}}\right) \right) , \end{aligned}$$

(6)

in which $\text {T}$ denotes the time ordering, and $\widehat{ \mathcal{H} }_\text {int}^\text {(I)}$ is the interaction Hamiltonian density in the interaction picture. The local interaction point $\left( t,{\varvec{x}}\right)$ is integrated in the four-dimensional spacetime. It is noteworthy that the wave-packet states $\left| \mathcal{W}\mathcal{P}_0 \right\rangle$ and $\left| \mathcal{W}\mathcal{P}_1,\mathcal{W}\mathcal{P}_2 \right\rangle$ are normalizable, and hence the transition amplitude (5) is finite, unlike in the ordinary plane-wave formalism.⁷ Through the Dyson series expansion of ${\widehat{U}}\!\left( T_{\textrm{out}}, T_{\textrm{in}}\right)$, a perturbative S-matrix can be systematically constructed at any order of perturbation using Wick’s theorem, as in the plane-wave case [11]. Throughout this paper, the subscripts 0, 1, and 2 denote V, P, and ${\overline{P}}$, respectively.

A free Gaussian wave packet is characterized by a set of parameters $\left\{ m, \sigma , X^0, {\varvec{X}}, {\varvec{P}} \right\}$, where m is the mass; $\sigma$ is the width squared; and $X^0$ is a reference time at which the wave packet takes the Gaussian form with the central values of the peak position ${\varvec{X}}$ and momentum ${\varvec{P}}$.

Within the chiral perturbation theory, the effective interaction Hamiltonian density is

$$\begin{aligned} \widehat{\mathcal{H}}^\text {(I)}_\text {int,eff}&= i g_{V+} \mathcal{V}^\mu \left[ \mathcal{P}^+ \partial _\mu \mathcal{P}^- - \mathcal{P}^- \partial _\mu \mathcal{P}^+ \right] \nonumber \\&\quad + i g_{V0} \mathcal{V}^\mu \left[ \mathcal{P}^0 \partial _\mu \overline{\mathcal{P}^0} - \overline{\mathcal{P}^0} \partial _\mu \mathcal{P}^0 \right] , \end{aligned}$$

(7)

where $\mathcal V$, $\mathcal P^\pm$, $\mathcal{P}^0$, and $\overline{\mathcal{P}^0}$ are the fields representing the vector meson, the charged pseudo-scalar mesons, the neutral pseudo-scalar meson, and its antiparticle, respectively, and $g_{V+}$ and $g_{V0}$ are the vector-meson effective couplings to the charged pseudo-scalars and to the neutral pseudo-scalars, respectively. In this paper, we take the isospin-symmetric limit

$$\begin{aligned} g_{V+} = g_{V0} \quad (=:g_V), \end{aligned}$$

(8)

with which the effective coupling $g_\text {eff}$ takes the form in the momentum space

$$\begin{aligned} g_\text {eff}\!\left( \lambda _0,P_0,P_1,P_2\right) := g_V \, \varepsilon _\mu \!\left( P_0,\lambda _0\right) \left( P_1^\mu - P_2^\mu \right) , \end{aligned}$$

(9)

where $P_0$, $P_1$, and $P_2$ are the four-momenta of the vector meson V, the pseudo-scalar meson P, and its antiparticle ${\overline{P}}$, respectively, and $\varepsilon _\mu$ is the polarization vector of the vector meson with $\lambda _0$ being its helicity.⁸

In this paper, we investigate the transition from an off-shell initial state for V to an on-shell final state for $P\overline{P}$, having an off-shell energy ${\widetilde{E}}_0$ and on-shell ones $E_1$, $E_2$, respectively:⁹

$$\begin{aligned} \widetilde{E}_0&:= \sqrt{m_V^2 + {\varvec{P}}_0^2 -i \, \Gamma _V m_V} \nonumber \\&= \sqrt{E_0^2 -i \, \Gamma _V m_V} \simeq E_0 - i \frac{m_V}{2 E_0} \Gamma _V,\nonumber \\ E_1&:= \sqrt{m_P^2 + {\varvec{P}}_1^2 },\nonumber \\ E_2&:= \sqrt{m_P^2 + {\varvec{P}}_2^2 }, \end{aligned}$$

(10)

where $m_V$ and $m_P$ are the masses of V and P, respectively; $E_0:= \sqrt{m_V^2 + {\varvec{P}}_0^2}$ is the on-shell energy of V; and $\Gamma _V$ is the “decay width” of V, or more precisely, the imaginary part of its plane-wave propagator divided by $m_V$; see Ref. [13] for detailed discussion, and see also footnote 13. Throughout this paper, we take the narrow width approximation for $\Gamma _V$ as in Eq. (10).¹⁰ Here, the off-shell V should eventually be regarded as an intermediate state for a scattering process that includes the production of V, which necessarily introduces the in-time-boundary effect appearing below.

Their wave functions take the form

$$\begin{aligned} f_{V}\!\left( x\right)&:= N_V \left( \frac{\sigma _0}{\pi }\right) ^{3/4} \left( \frac{\pi }{\sigma _0}\right) ^{3/2}\nonumber \\ {}&\quad \times \frac{1}{\sqrt{2P^0_0} \left( 2\pi \right) ^{3/2}} e^{ i P_0 \cdot (x - X_0) - \frac{ \left( {\varvec{x}} - \varvec{\Xi }_0 \!\left( t\right) \right) ^2 }{2\sigma _0} } \Bigg |_{P_0^0 = \widetilde{E}_0}, \nonumber \\ f_P\!\left( x\right)&:= \left( \frac{\sigma _1}{\pi }\right) ^{3/4} \left( \frac{\pi }{\sigma _1}\right) ^{3/2}\nonumber \\ {}&\qquad \times \frac{1}{\sqrt{2P^0_1} \left( 2\pi \right) ^{3/2}} e^{ i P_1 \cdot (x - X_1) - \frac{ \left( {\varvec{x}} - \varvec{\Xi }_1 \!\left( t\right) \right) ^2 }{2\sigma _1} } \Bigg |_{P^0_1 = E_1}, \nonumber \\ f_{\overline{P}}\!\left( x\right)&:= \left( \frac{\sigma _2}{\pi }\right) ^{3/4} \left( \frac{\pi }{\sigma _2}\right) ^{3/2}\nonumber \\ {}&\quad \times \frac{1}{\sqrt{2P^0_2} \left( 2\pi \right) ^{3/2}} e^{ i P_2 \cdot (x - X_2) - \frac{ \left( {\varvec{x}} - \varvec{\Xi }_2 \!\left( t\right) \right) ^2 }{2\sigma _2} } \Bigg |_{P^0_2 = E_2}, \end{aligned}$$

(11)

where $N_V$ is a wave-function (field) renormalization factor for V due to its off-shellness ,¹¹ and

$$\begin{aligned} \varvec{\Xi }_A \!\left( t\right) := {\varvec{X}}_A + {\varvec{V}}_A \left( t - X^0_A\right) \qquad (A=0,1,2) \end{aligned}$$

(12)

describes the location of the center of the wave packet at a time t with the central velocity

$$\begin{aligned} {\varvec{V}}_A := \frac{ {\varvec{P}}_A }{ E_A }. \end{aligned}$$

(13)

Now it is straightforward to compute the S-matrix from Eq. (5) at the leading order in the Dyson series (6) with the effective Hamiltonian (7) from the wave functions (11) [11]:

$$\begin{aligned} S_{V \rightarrow P {\overline{P}}}&= i g_\text {eff}\!\left( \lambda _0,P_0,P_1,P_2\right) \nonumber \\&\quad \times \left( \prod _{A=0}^2 \frac{1}{\sqrt{2E_A}} \left( \frac{1}{\pi \sigma _A}\right) ^{3/4} \right) e^{ - \frac{\sigma _t}{2}(\delta \omega )^2 - \frac{\sigma _s}{2} (\delta {\varvec{P}})^2 - \frac{\mathcal{R}}{2} } \nonumber \\&\quad \times \int _{T_{\textrm{in}}}^{T_{\textrm{out}}} \text {d}t \, e^{ -\frac{1}{2\sigma _t} \left[ t - \left( {\mathfrak {T}} + i \sigma _t \delta \omega \right) \right] ^2 }\nonumber \\&\quad \times \int \text {d}^3 {\varvec{x}} \, e^{ -\frac{1}{2\sigma _s} \left[ {\varvec{x}} - \left( \overline{\varvec{{\mathfrak {X}}}} + \overline{{\varvec{V}}} t - i \sigma _s \delta {\varvec{P}} \right) \right] ^2 } \nonumber \\&\quad \times N_V \, e^{ - {\Gamma _V\over 2}\left( t - {T_0}\right) } \widetilde{F} \!\left( \left| {\varvec{V}}_1 - {\varvec{V}}_2\right| \right) , \end{aligned}$$

(14)

where the notation follows Eq. (27) of Ref. [11] (see also below for a short summary).¹² Differences from the previous calculation [11] are the following four points: First, the coupling is changed to $\kappa /\sqrt{2}\rightarrow g_\text {eff}$. Second, the “decay width” of V is included as the phenomenological factor $e^{ - {\Gamma _V\over 2}\left( t - {T_0}\right) }$,¹³ where $T_0:= X^0_0$ is the initial time from which V starts to exist.¹⁴ Third, $N_V$ in Eq. (11) is introduced. Fourth, we have included a phenomenological form factor $\widetilde{F}$ due to the composite nature of V:

$$\begin{aligned} \widetilde{F} \!\bigl (\left| {\varvec{V}}_1-{\varvec{V}}_2\right| \bigr ) := \frac{1}{1+\left( \frac{R_0 m_P\left| {\varvec{V}}_1-{\varvec{V}}_2\right| }{2}\right) ^2 }, \end{aligned}$$

(15)

where $R_0$ describes a typical length scale of the compositeness of V; see Appendix A. The normalization is such that $\widetilde{F}$ becomes unity for ${\varvec{V}}_1 = {\varvec{V}}_2$.

Fig. 1

Schematic figure for the finite wave-packet process (left) and the infinite plane-wave process (right), without taking into account the decay width $\Gamma _V$. In the left, we have shown the time of intersection ${\mathfrak {T}}$; the spatial and temporal sizes of the overlap $\sqrt{\sigma _s}$ and $\sqrt{\sigma _t}$; the center of wave packets $\varvec{\Xi }_A$ ($A=0,1,2$); and the initial and final times of the scattering $T_{\textrm{in}}$ and $T_{\textrm{out}}$. Also, the bulk $T_\text {in}\ll t\ll T_\text {out}$, in-time-boundary ($\left| t-T_\text {in}\right| \lesssim \sqrt{\sigma _t}$), and out-time-boundary ($\left| T_\text {out}-t\right| \lesssim \sqrt{\sigma _t}$) regions are shown. (This panel corresponds to the bulk-like case $\left| \mathfrak T-T_{\textrm{in}}\right| \gg \sqrt{\sigma _t}$; see Fig. 2.) In the right, the spatial overlap of the plane waves never decreases in time, and hence the interaction would be never switched off, and the scattering would be never completed; therefore the extra damping factor $e^{\mp \epsilon t\int \text {d}^3{\varvec{x}}\,\widehat{\mathcal{H}}_\text {int}^\text {(I)}\!\left( t,{\varvec{x}}\right) }$ with an infinitesimal $\epsilon >0$ is conventionally put by hand for the future and past infinite times $t\rightarrow \pm \infty$, which is depicted by the damping of the opacity of the orange region. This factor eventually results in the propagator $\propto \left( p^2+m^2-i\epsilon \right) ^{-1}$ in the conventional Feynman diagram calculation

Now we provide a brief introduction to other variables in the first two lines of (14) (see Sect. 3.1 of [11] for more details):

• $\sqrt{\sigma _s}$ is a typical spacial size of the region of interaction

  $$\begin{aligned} \sigma _s^{-1} := \sum _{A=0}^{2} \frac{1}{\sigma _A}. \end{aligned}$$

  (16)

• $\sqrt{\sigma _t}$ is a typical temporal size of the interaction region¹⁵

  $$\begin{aligned} \sigma _t := \frac{\sigma _s}{\Delta {\varvec{V}}^2}. \end{aligned}$$

  (17)

• ${\mathfrak {T}}$ is the time of intersection of the three wave packets,

  $$\begin{aligned} {\mathfrak {T}} := \sigma _t \frac{ \overline{{\varvec{V}}} \cdot \overline{\varvec{{\mathfrak {X}}}} - \overline{ {\varvec{V}} \cdot \varvec{{\mathfrak {X}}} } }{\sigma _s}, \end{aligned}$$

  (18)

  where

  $$\begin{aligned} \varvec{{\mathfrak {X}}}_A := {\varvec{\Xi }}_A\!\left( 0\right) \quad \left( \,= {\varvec{X}}_A - {\varvec{V}}_A X_A^0\ \right) \end{aligned}$$

  (19)

  is the location of the center of each wave packet at our reference time $t=0$. As mentioned above, each wave packet takes the Gaussian form centered at ${\varvec{X}}_A$ at its reference time $X_A^0$.

• $\mathcal{R}$ is called the overlap exponent, which provides the exponential suppression when wave packets are separated from each other:

  $$\begin{aligned} \mathcal{R} := \frac{\Delta \varvec{{\mathfrak {X}}}^2}{\sigma _s} - \frac{{\mathfrak {T}}^2}{\sigma _t}. \end{aligned}$$

  (20)

• We write the deviation of energy momentum from the conserved values (for their central values of wave packets) as

  $$\begin{aligned} \delta {\varvec{P}}&:= {\varvec{P}}_1 + {\varvec{P}}_2 - {\varvec{P}}_0, \nonumber \\ \delta E&:= E_1 + E_2 - E_0,&\delta \omega&:= \delta E - \overline{{\varvec{V}}} \cdot \delta {\varvec{P}}, \end{aligned}$$

  (21)

  where $\omega _A:= E_A - \overline{{\varvec{V}}} \cdot {\varvec{P}}_A$ is the “shifted energy” of each packet.

A schematic figure is shown in the left panel of Fig. 1, compared with the plane-wave counterpart in the right.

After the square completion of t and the analytic Gaussian integration over ${\varvec{x}}$ in (14), as made in [11], we represent the S-matrix as

$$\begin{aligned} S_{V \rightarrow P {\overline{P}}}&= ig_\text {eff} N_V\nonumber \\&\quad \times \left( \prod _{A=0}^2 \frac{1}{\sqrt{2E_A}} \left( \frac{1}{\pi \sigma _A}\right) ^{3/4} \right) e^{ - \frac{\sigma _t}{2}(\delta \omega )^2 - \frac{\sigma _s}{2} (\delta {\varvec{P}})^2 - \frac{\mathcal{R}}{2} }\nonumber \\&\quad \times \left( 2\pi \sigma _s\right) ^{3/2} \sqrt{2\pi \sigma _t} \, G({\mathfrak {T}}) \nonumber \\&\quad \times e^{ - {\Gamma _V\over 2}\left( {\mathfrak {T}} - {T_0} +i \sigma _t \delta \omega \right) + \frac{\Gamma _V^2\sigma _t}{8} }\, \widetilde{F} \!\left( \left| {\varvec{V}}_1 - {\varvec{V}}_2\right| \right) , \end{aligned}$$

(22)

where the window function $G({\mathfrak {T}})$ is defined as¹⁶

$$\begin{aligned} G({\mathfrak {T}})&:= \int _{T_{\textrm{in}}}^{T_{\textrm{out}}} \frac{\text {d}t}{\sqrt{2\pi \sigma _t}} e^{ -\frac{1}{2\sigma _t} \left[ t - \left( {\mathfrak {T}} - {\Gamma _V\sigma _t\over 2} + i \sigma _t \delta \omega \right) \right] ^2 } \nonumber \\&= \frac{1}{2} \left[ \text {erf}\left( \frac{{\mathfrak {T}} -T_{\textrm{in}}- {\Gamma _V\sigma _t\over 2} + i \sigma _t \delta \omega }{\sqrt{2\sigma _t}} \right) \right. \nonumber \\&\quad \left. - \text {erf}\left( \frac{{\mathfrak {T}} -T_{\textrm{out}}- {\Gamma _V\sigma _t\over 2} + i \sigma _t \delta \omega }{\sqrt{2\sigma _t}} \right) \right] , \end{aligned}$$

(23)

with

$$\begin{aligned} \text {erf}(z) := \frac{2}{\sqrt{\pi }} \int _0^z e^{-x^2} \text {d}x \end{aligned}$$

(24)

being the Gauss error function. The window function $G\!\left( {\mathfrak {T}}\right)$ becomes unity for $T_{\textrm{in}}\ll {\mathfrak {T}}\ll T_{\textrm{out}}$ and zero for ${\mathfrak {T}}\ll T_{\textrm{in}}$ and for $T_{\textrm{out}}\ll {\mathfrak {T}}$. For a given configuration of in and out states, which fixes the value of $\sigma _t$, the time regions $T_{\textrm{in}}\ll t\ll T_{\textrm{out}}$,¹⁷$\left| t-T_{\textrm{in}}\right| \lesssim \sqrt{\sigma _t}$, and $\left| t-T_{\textrm{out}}\right| \lesssim \sqrt{\sigma _t}$ are called the bulk, in-time-boundary, and out-time-boundary regions, respectively. In the phenomenological analysis below, we will neglect the out-time-boundary contributions as we will discuss.

2.2 Differential decay probability

From the S-matrix (22), the differential decay probability can be derived as

$$\begin{aligned}&\text {d}P_{V \rightarrow P \overline{P}} \nonumber \\&\quad = \frac{ \text {d}^3 {\varvec{X}}_1 \text {d}^3 {\varvec{P}}_1}{(2\pi )^3} \frac{ \text {d}^3 {\varvec{X}}_2 \text {d}^3 {\varvec{P}}_2}{(2\pi )^3} \left| S_{V \rightarrow P {\overline{P}}}\right| ^2 \nonumber \\&\quad = \overline{|g_\text {eff}|^2} N_V^2 \frac{1}{2 E_0} \frac{\text {d}^3 {\varvec{P}}_1}{(2\pi )^3 2E_1} \frac{\text {d}^3 {\varvec{P}}_2}{(2\pi )^3 2E_2} (2 \pi )^4\nonumber \\&\qquad \times \left( \sqrt{\frac{\sigma _t}{\pi }} e^{-\sigma _t\left( \delta \omega \right) ^2} \right) \left( \left( \frac{\sigma _s}{\pi }\right) ^{3/2} e^{-\sigma _s\left( \delta {\varvec{P}}\right) ^2} \right) \nonumber \\&\qquad \times \sqrt{ \frac{\sigma _t}{\pi ^5} \left( \frac{\sigma _s}{\sigma _0\sigma _1\sigma _2} \right) ^3 } \text {d}^3 {\varvec{X}}_1 \text {d}^3 {\varvec{X}}_2 e^{- {\mathcal {R}}} \, |G({\mathfrak {T}})|^2 \,\nonumber \\&\qquad \times e^{ - \Gamma _V\left( {\mathfrak {T}} - {T_0} \right) + \frac{\Gamma _V^2\sigma _t}{4} }\, \left| \widetilde{F}\!\left( \left| {\varvec{V}}_1 - {\varvec{V}}_2\right| \right) \right| ^2, \end{aligned}$$

(25)

where we have taken the average over the helicity $\lambda _0$, which results in the helicity-averaged effective coupling

$$\begin{aligned} \overline{|g_\text {eff}|^2}&:= \frac{g_V^2}{3} \sum _{\lambda _0} \bigl | \varepsilon _\mu (P_0,\lambda _0) \left( P_1^\mu - P_2^\mu \right) \bigr |^2\nonumber \\&= {\frac{g_V^2}{3}} ({\varvec{P}}_1 - {\varvec{P}}_2)^2. \end{aligned}$$

(26)

Here, the last equality further assumes the vanishing initial momentum ${\varvec{P}}_0=0$. We will compute the integrated decay probability under this assumption in Sect. 2.3 and in Appendix B.

Fig. 2

Schematic figures for two limiting cases $\left| {\mathfrak {T}}-T_{\textrm{in}}\right| \gg \sqrt{\sigma _t}$ (left) and $\sqrt{\sigma _t}\gg \Gamma _V^{-1}$ (right). The case $\delta \omega \gg \sigma _t^{-1/2}$ is hard to draw in the position space and is not shown here. The overlap region is determined by both the initial and final states as in Fig. 1

Hereafter, we assume both the following conditions:

$$\begin{aligned}&\frac{\left| {\mathfrak {T}} -T_{\textrm{in}}- {\Gamma _V\sigma _t\over 2} + i \sigma _t \delta \omega \right| }{\sqrt{2\sigma _t}} \gg 1, \nonumber \\ {}&\quad \frac{\left| {\mathfrak {T}} -T_{\textrm{out}}- {\Gamma _V\sigma _t\over 2} + i \sigma _t \delta \omega \right| }{\sqrt{2\sigma _t}} \gg 1. \end{aligned}$$

(27)

Physically, each of these conditions is satisfied when at least one of the following three conditions is met:

• $\left| {\mathfrak {T}}-T_{\textrm{in}}\right| \gg \sqrt{\sigma _t}$ (or $\left| {\mathfrak {T}}-T_{\textrm{out}}\right| \gg \sqrt{\sigma _t}$) when the interaction time ${\mathfrak {T}}$ is apart enough from $T_{\textrm{in}}$ (or $T_{\textrm{out}}$) compared to the temporal width of the overlap $\sqrt{\sigma _t}$, which typically corresponds to the “bulk-like” case (Fig. 2, left);

• $\sqrt{\sigma _t}\gg \Gamma _V^{-1}$ when the “mean lifetime through bulk effect” $\Gamma _V^{-1}$ is much shorter than the temporal width of the overlap region $\sqrt{\sigma _t}$, which typically corresponds to the so-to-say “decay within wave-packet overlap” case (Fig. 2, right);

• $\delta \omega \gg \sigma _t^{-1/2}$ when the deviation from the conservation of the shifted energy, $\delta \omega$, is much larger than the inverse of the temporal width of the overlap $1/\sqrt{\sigma _t}$, namely, the “violation of shifted energy” case.

This assumption (27) is made for simplicity, and there is no obstacle to using the full form (23) in the numerical computation in principle, but the result would remain the same approximately because this is satisfied anyway in the ordinary bulk-like case and when anything interesting happens around the (in-)time boundary.

Under the assumption (27), the following asymptotic form is obtained [11]:

$$\begin{aligned} G({\mathfrak {T}})&\simeq W({\mathfrak {T}}) \nonumber \\&\quad - {\frac{1}{2}} e^{ -\frac{ \left( {\mathfrak {T}} - T_{\textrm{in}}- {\Gamma _V\sigma _t\over 2}\right) ^2 }{2\sigma _t} + \frac{\sigma _t}{2}(\delta \omega )^2 -i \delta \omega \left( {\mathfrak {T}} -T_{\textrm{in}}- {\Gamma _V\sigma _t\over 2} \right) } \nonumber \\&\quad \times \sqrt{ \frac{2\sigma _t}{\pi } }\frac{1}{ {\mathfrak {T}} -T_{\textrm{in}}- {\Gamma _V\sigma _t\over 2} + i \sigma _t \delta \omega } \nonumber \\&\quad + {\frac{1}{2}} e^{ -\frac{ \left( {\mathfrak {T}} - {\Gamma _V\sigma _t\over 2} - T_{\textrm{out}}\right) ^2 }{2\sigma _t} + \frac{\sigma _t}{2}(\delta \omega )^2 -i \delta \omega \left( {\mathfrak {T}} - {\Gamma _V\sigma _t\over 2} -T_{\textrm{out}}\right) } \nonumber \\&\quad \times \sqrt{ \frac{2\sigma _t}{\pi } } \frac{1}{ {\mathfrak {T}} -T_{\textrm{out}}- {\Gamma _V\sigma _t\over 2} + i \sigma _t \delta \omega }, \end{aligned}$$

(28)

where we have defined the “bulk window function”

$$\begin{aligned} W({\mathfrak {T}})&:= \frac{1}{2} \left[ \text {sgn}\left( \frac{{\mathfrak {T}} -T_{\textrm{in}}- {\Gamma _V\sigma _t\over 2} + i \sigma _t \delta \omega }{\sqrt{2\sigma _t}} \right) \right. \nonumber \\&\quad \left. - \text {sgn}\left( \frac{{\mathfrak {T}} -T_{\textrm{out}}- {\Gamma _V\sigma _t\over 2} + i \sigma _t \delta \omega }{\sqrt{2\sigma _t}} \right) \right] , \end{aligned}$$

(29)

in which the sign function for a complex variable is

$$\begin{aligned} \text {sgn}(z)&:= {\left\{ \begin{array}{ll} +1 &{} \text {for } \Re z>0 \text { or } ( \Re z = 0 \text { and } \Im z >0 ), \\ -1 &{} \text {for } \Re z<0 \text { or } ( \Re z = 0 \text { and } \Im z <0 ), \\ 0 &{} \text {for } z=0. \end{array}\right. } \end{aligned}$$

(30)

Here and hereafter, $\Re$ and $\Im$ denote the real and imaginary parts, respectively. Equation (29) describes the ordinary “bulk contribution” for the quantum transition from the in to out states in the time period $[T_{\textrm{in}}, T_{\textrm{out}}]$. The second and third terms of Eq. (28) show the contributions near the in and out time boundaries $T_\text {in}$ and $T_\text {out}$, respectively. More explicitly,¹⁸

$$\begin{aligned} W({\mathfrak {T}})&= {\left\{ \begin{array}{ll} 1 &{} ( T_{\textrm{in}}+ {\Gamma _V\sigma _t\over 2}< {\mathfrak {T}} < T_{\textrm{out}}+ {\Gamma _V\sigma _t\over 2} ), \\ 0 &{} \text {otherwise}. \end{array}\right. } \end{aligned}$$

(31)

Because the contribution from the out-time-boundary ${\mathfrak {T}}\simeq T_\text {out}$ is suppressed by the extra dumping factor $e^{ -\Gamma _V\left( {\mathfrak {T}} - T_0\right) }$ in the differential probability (25),¹⁹ it is safe to neglect the out-time-boundary contribution, and we can take

$$\begin{aligned} T_{\textrm{out}}\rightarrow +\infty , \end{aligned}$$

(32)

with which the second line in Eq. (28) goes down to zero. With this limit, $\left| G({\mathfrak {T}})\right| ^2$ reads

$$\begin{aligned} \left| G({\mathfrak {T}})\right| ^2&\rightarrow \left[ \mathcal GG\right] _\text {bulk}({\mathfrak {T}}) + \left[ \mathcal GG\right] _\text {bdry}({\mathfrak {T}}) + \left[ \mathcal GG\right] _\text {intf}({\mathfrak {T}}), \end{aligned}$$

(33)

where

$$\begin{aligned}&\left[ \mathcal GG\right] _\text {bulk}({\mathfrak {T}}) := \bigl |{W({\mathfrak {T}})}\bigr |^2, \end{aligned}$$

(34)

$$\begin{aligned}&\left[ \mathcal GG\right] _\text {bdry}({\mathfrak {T}}) := \frac{1}{4} e^{ -\frac{ \left( {\mathfrak {T}} - T_{\textrm{in}}- {\Gamma _V\sigma _t\over 2}\right) ^2 }{\sigma _t} + {\sigma _t}(\delta \omega )^2 } \frac{2\sigma _t}{\pi }\nonumber \\&\times \frac{1}{ \left( {\mathfrak {T}} -T_{\textrm{in}}- {\Gamma _V\sigma _t\over 2} \right) ^2 + \left( \sigma _t \delta \omega \right) ^2 }, \end{aligned}$$

(35)

$$\begin{aligned}&\left[ \mathcal GG\right] _\text {intf}({\mathfrak {T}}) := -\frac{W\!\left( {\mathfrak {T}}\right) }{2} e^{ -\frac{ \left( {\mathfrak {T}} - T_{\textrm{in}}- {\Gamma _V\sigma _t\over 2}\right) ^2 }{2\sigma _t} + \frac{\sigma _t}{2}(\delta \omega )^2} \sqrt{ \frac{2\sigma _t}{\pi } } \nonumber \\&\quad \qquad \qquad \quad \times \left\{ \frac{ e^{ -i \delta \omega \left( {\mathfrak {T}} -T_{\textrm{in}}- {\Gamma _V\sigma _t\over 2} \right) } }{ {\mathfrak {T}} -T_{\textrm{in}}- {\Gamma _V\sigma _t\over 2} + i \sigma _t \delta \omega } \right. \nonumber \\ {}&\quad \quad \qquad \qquad \quad \left. + \frac{ e^{ +i \delta \omega \left( {\mathfrak {T}} -T_{\textrm{in}}- {\Gamma _V\sigma _t\over 2} \right) } }{ {\mathfrak {T}} -T_{\textrm{in}}- {\Gamma _V\sigma _t\over 2} - i \sigma _t \delta \omega } \right\} . \end{aligned}$$

(36)

The three functions $\left[ \mathcal GG\right] _\text {bulk}$, $\left[ \mathcal GG\right] _\text {bdry}$, and $\left[ \mathcal GG\right] _\text {intf}$ describe the square of the bulk term, the square of the in-time-boundary term, and the interference between the bulk and in-boundary terms, respectively.

With the approximation (27) and the limit (32), the differential probability (25) takes the simpler form and can be classified into the following three parts:

$$\begin{aligned} \text {d}P_{V \rightarrow P \overline{P}} = \text {d}P_{V \rightarrow P \overline{P}}^{\text {bulk}} + \text {d}P_{V \rightarrow P \overline{P}}^{\text {bdry}} + \text {d}P_{V \rightarrow P \overline{P}}^\text {intf}, \end{aligned}$$

(37)

with

$$\begin{aligned}&\text {d}P_{V \rightarrow P \overline{P}}^\text {``type''} := |g_\text {eff}|^2 N_V^2 \frac{1}{2 E_0} \frac{\text {d}^3 {\varvec{P}}_1}{(2\pi )^3 2E_1} \frac{\text {d}^3 {\varvec{P}}_2}{(2\pi )^3 2E_2} (2 \pi )^4\nonumber \\&\quad \times \left( \sqrt{\frac{\sigma _t}{\pi }} e^{-\sigma _t\left( \delta \omega \right) ^2} \right) \left( \left( \frac{\sigma _s}{\pi }\right) ^{3/2} e^{-\sigma _s\left( \delta {\varvec{P}}\right) ^2} \right) \nonumber \\&\quad \times \sqrt{ \frac{\sigma _t}{\pi ^5} \left( \frac{\sigma _s}{\sigma _0\sigma _1\sigma _2} \right) ^3 }\, \text {d}^3 {\varvec{X}}_1 \text {d}^3 {\varvec{X}}_2\, e^{- {\mathcal {R}}} \, \left[ \mathcal GG\right] _\text {``type''}\!\left( {\mathfrak {T}}\right) \, \nonumber \\&\quad \times e^{ - \Gamma _V\left( {\mathfrak {T}} - {T_0} \right) + \frac{\Gamma _V^2\sigma _t}{4} } \left| \widetilde{F}\!\bigl (\left| {\varvec{V}}_1 - {\varvec{V}}_2\right| \bigr ) \right| ^2, \end{aligned}$$

(38)

where the argument “type” discriminates the three types of contributions.

2.3 Integrated decay probability

To compare the theoretical predictions in the Gaussian wave-packet formalism with the experimental results in Eq. (2), we integrate the differential decay probability (37) over the whole position-momentum phase space of the final-state pseudo-scalar mesons, namely, over ${\varvec{X}}_1$, ${\varvec{X}}_2$, ${\varvec{P}}_1$, and ${\varvec{P}}_2$:

$$\begin{aligned} P_{V\rightarrow P{\overline{P}}}=P_{V\rightarrow P{\overline{P}}}^\text {bulk}+P_{V\rightarrow P{\overline{P}}}^\text {bdry}+P_{V\rightarrow P{\overline{P}}}^\text {intf}. \end{aligned}$$

(39)

We focus on the situation where these integrals can be performed analytically using the saddle-point approximation; see e.g. Ref. [11]. In the current setup, we can safely take nonrelativistic approximations in the kinematics of the system because the mass difference $m_V - 2 m_{P}$ is small.

Here, we only list the final form of the three types of contributions to the integrated decay probability: the bulk, boundary, and interference contributions. These calculations’ details are provided in Appendix B. For later convenience, we define a common dimensionless factor $\mathcal{C}_{V \rightarrow P \overline{P}}$ for all of $P_{V \rightarrow P\overline{P}}^\text {bulk}$, $P_{V \rightarrow P\overline{P}}^\text {bdry}$, and $P_{V \rightarrow P\overline{P}}^\text {intf}$ as

$$\begin{aligned} \mathcal{C}_{V \rightarrow P \overline{P}} := \frac{g^2_V m_P N_V^2 e^{ -\Gamma _V\left( T_{\textrm{in}}-{T_0}\right) } }{12 \pi m_V}. \end{aligned}$$

(40)

2.3.1 Bulk contribution

Integrating the bulk contribution in Eq. (37), we obtain

$$\begin{aligned} P_{V \rightarrow P\overline{P}}^\text {bulk}&\simeq {\mathcal{C}_{V \rightarrow P \overline{P}}\,m_P\over \Gamma _V} \left( \frac{\left( m_V-2m_P\right) ^2}{m_P^2} + {\Gamma _V^2\over 4m_P^2} \right) ^{3/4} \nonumber \\&\quad \times \frac{1}{2} \left[ 1 + \text {erf}\left( \frac{ m_P\sqrt{\sigma _P} {V_-^\text {B}} }{\sqrt{2}} \right) \right] \nonumber \\ {}&\quad \times {\frac{e^{-F^0_\text {bulk}}}{A_\text {bulk}^{3/2}} \left| \widetilde{F}\!\left( V_-^\text {B}\right) \right| ^2 }, \end{aligned}$$

(41)

where

$$\begin{aligned} V_-^\text {B}&:= 2 \left[ \frac{\left( m_V-2m_P\right) ^2}{m_P^2} + {\Gamma _V^2\over 4m_P^2} \right] ^{1/4}, \end{aligned}$$

(42)

$$\begin{aligned} F_\text {bulk}^0&:= m_P \sigma _P \left( -\left( m_V - 2m_P\right) \right. \nonumber \\ {}&\quad \left. + \sqrt{ \left( m_V - 2m_P\right) ^2+{\Gamma _V^2\over 4} } \right) , \end{aligned}$$

(43)

$$\begin{aligned} A_\text {bulk}&:= \frac{1}{2}+ \frac{ \left( m_V - 2m_P\right) }{2\sqrt{\left( m_V-2m_P\right) ^2+{\Gamma _V^2\over 4}}}. \end{aligned}$$

(44)

We note that the wave packet size of the decaying particle $\sigma _V$ drops out of this expression at this order of the saddle-point approximation.

Fig. 3

The P-factors (54) are shown for $\phi \rightarrow K^+ K^-$ and $\phi \rightarrow K^0 \overline{K^0}$ (in the top row), $\psi \rightarrow D^+ D^-$ and $\psi \rightarrow D^0 \overline{D^0}$ (in the middle row), and $\Upsilon \rightarrow B^+ B^-$ and $\Upsilon \rightarrow B^0 \overline{B^0}$ (in the bottom row), where we take a typical value $0.0015\,\text {MeV}^{-1}=\left( 0.67\,\text {GeV}\right) ^{-1}$ for $R_0$; see Appendix A. The wave-packet treatment breaks down when the wave-packet size of the decay product $\sqrt{\sigma _P}$ is smaller than the de Broglie wavelength of P, which is depicted by the hatched region

2.3.2 Boundary contribution

Integrating the boundary contribution in Eq. (37), we obtain

$$\begin{aligned} P_{V \rightarrow P\overline{P}}^\text {bdry}&\simeq \mathcal{C}_{V \rightarrow P \overline{P}} \frac{I_\text {bdry}}{2\pi }, \end{aligned}$$

(45)

where $I_\text {bdry}$ is written as an integration of a function of $V_-$ as

$$\begin{aligned} I_\text {bdry}&:= \int _0^\infty \text {d}V_- \widetilde{f}_\text {bdry}\!\left( V_-\right) , \end{aligned}$$

(46)

in which

$$\begin{aligned}&\widetilde{f}_\text {bdry}\!\left( V_-\right) = \frac{V_-^4}{\left( V_-^2 - 4\frac{m_V-2m_P}{m_P}\right) ^2 + \frac{4^2}{m_P^2} { {\Gamma _V^2\over 4} } } \left| \widetilde{F}\!\left( V_-\right) \right| ^2 \nonumber \\&\quad - \frac{ V_-^4 \left[ \left( V_-^2 - 4\frac{m_V-2m_P}{m_P}\right) ^2 -3 \frac{4\Gamma _V^2}{m_P^2}\right] }{ 2{ \frac{2 \sigma _P}{V_-^2} } \left( \frac{m_P}{4} \right) ^2 \left[ \left( V_-^2 - 4\frac{m_V-2m_P}{m_P}\right) ^2 + \frac{4\Gamma _V^2}{m_P^2} \right] ^3 } \left| \widetilde{F}\!\left( V_-\right) \right| ^2. \end{aligned}$$

(47)

The integral (46) will be evaluated numerically.

2.3.3 Interference contribution

Integrating the interference contribution in Eq. (37), we obtain

$$\begin{aligned}&P^\text {intf}_{V \rightarrow P\overline{P}} \sim \nonumber \\&\quad - \, \mathcal{C}_{V \rightarrow P \overline{P}} \frac{ m_P \sqrt{\sigma _P}}{2\sqrt{2} \sqrt{\pi }} \left( V_-^\text {I} \right) ^2 \frac{1}{2} \left[ 1 + \text {erf}\left( \frac{ m_P\sqrt{ \sigma _P} {V_-^\text {I}} }{2} \right) \right] \nonumber \\&\quad \times {\frac{e^{-F^0_\text {intf}}}{A_\text {intf}^{3/2}}}\, \left| \widetilde{F}\!\left( V^\text {I}_-\right) \right| ^2 \nonumber \\&\quad \times \frac{ \left( \Gamma _V^2 - \left( \widetilde{\delta \omega }\right) ^2 \right) \cos \!\left( 2 \Gamma _V\widetilde{\sigma _t} \widetilde{\delta \omega }\right) - 2 \Gamma _V \widetilde{\delta \omega } \sin \!\left( 2 \Gamma _V\widetilde{\sigma _t} \widetilde{\delta \omega }\right) }{\widetilde{\sigma _t}\left( \Gamma _V^2 + \left( \widetilde{\delta \omega }\right) ^2 \right) ^2}, \end{aligned}$$

(48)

where the definition of new parameters is

$$\begin{aligned}&V_-^\text {I} := \frac{ 2 \left[ \left( m_V-2m_P\right) ^2+{\Gamma _V^2\over 2}\right] ^{1/4} }{\sqrt{m_P }}, \end{aligned}$$

(49)

$$\begin{aligned}&{F_\text {intf}^0} \nonumber \\&:= \frac{ m_P \sigma _P }{2} \left[ -{\left( m_V - 2m_P\right) } + \sqrt{\left( m_V - 2m_P\right) ^2+{\Gamma _V^2\over 2}} \right] , \end{aligned}$$

(50)

$$\begin{aligned}&A_\text {intf} := \frac{1}{4} \left[ 3 + \frac{ m_V - 2m_P}{\sqrt{\left( m_V-2m_P\right) ^2+{\Gamma _V^2\over 2}}} \right] , \end{aligned}$$

(51)

$$\begin{aligned}&\widetilde{\sigma _t} := \frac{2 \sigma _P}{ \left( V_-^\text {I}\right) ^2 }, \end{aligned}$$

(52)

$$\begin{aligned}&\widetilde{\delta \omega } := \frac{1}{4} m_P \left( V_-^\text {I}\right) ^2 - \left( m_V - 2m_P\right) . \end{aligned}$$

(53)

The tilde denotes that the values are evaluated at the saddle-point for the interference contribution.

3 Magnitudes of three kinds of contributions

We have seen the magnitudes of the three kinds of contributions to the integrated probability from the bulk part $P_{V \rightarrow P\overline{P}}^\text {bulk}$ in Eq. (41), from the boundary part $P_{V \rightarrow P\overline{P}}^\text {bdry}$ in Eq. (45), and from the bulk boundary interference $P_{V \rightarrow P\overline{P}}^\text {intf}$ in Eq. (48). Hereafter, we call the following three ratios the P-factors:

$$\begin{aligned} {\mathscr {P}}_\text {bulk}&:= {P_{V \rightarrow P\overline{P}}^\text {bulk}\over \mathcal{C}_{V \rightarrow P\overline{P}}},\nonumber \\ {\mathscr {P}}_\text {bdry}&:= {P_{V \rightarrow P\overline{P}}^\text {bdry}\over \mathcal{C}_{V \rightarrow P\overline{P}}},\nonumber \\ {\mathscr {P}}_\text {intf}&:= {P_{V \rightarrow P\overline{P}}^\text {intf}\over \mathcal{C}_{V \rightarrow P\overline{P}}}, \end{aligned}$$

(54)

where the common $\mathcal{C}_{V \rightarrow P\overline{P}}$ given in Eq. (40) is factored out.

In Fig. 3, the P-factors (54) are shown for the following cases, with a typical value $0.0015\,\text {MeV}^{-1}=\left( 0.67\,\text {GeV}\right) ^{-1}$ for $R_0$ (see Appendix A):

• $\phi \rightarrow K^+ K^-$ and $\phi \rightarrow K^0 \overline{K^0}$ (first row),

• $\psi \rightarrow D^+ D^-$ and $\psi \rightarrow D^0 \overline{D^0}$ (second row),

• $\Upsilon \rightarrow B^+ B^-$ and $\Upsilon \rightarrow B^0 \overline{B^0}$ (third row).

We remind that all of $P_{V \rightarrow P\overline{P}}^\text {bulk}$, $P_{V \rightarrow P\overline{P}}^\text {bdry}$, and $P_{V \rightarrow P\overline{P}}^\text {intf}$ do not depend on $\sigma _V$ within the nonrelativistic saddle-point approximation; see Appendix B for details.

From Fig. 3, we can read the following properties:

• If the magnitude of $\sqrt{\sigma _P}$ is relatively low, the three kinds of the P-factors are of the same order.

• When $\sqrt{\sigma _P}$ is a certain magnitude, the bulk contribution is exponentially suppressed, while the boundary contribution takes almost the same value, where the interference part is negligible. For such a $\sqrt{\sigma _P}$ and other higher choices of it, the boundary part dominates.

• Physically, the wave-packet treatment of the decay product P breaks down when the wave-packet size $\sqrt{\sigma _P}$ is shorter than the de Broglie wavelength of P,

  $$\begin{aligned} \lambda _\text {de Broglie} := \frac{2\pi }{ m_P V_{-}^\text {B}}, \end{aligned}$$

  (55)

  where $V_{-}^\text {B}$ is the expectation value in the bulk part in Eq. (42). It is straightforward to estimate it for each decay process:

  $$\begin{aligned} \lambda _\text {de Broglie}|_{\phi \rightarrow K^+K^-}&= 0.025\,\text {MeV}^{-1} = {1\over 40\,\text {MeV}},\nonumber \\ \lambda _\text {de Broglie}|_{\phi \rightarrow K^0 \overline{K^0}}&= 0.029\,\text {MeV}^{-1} = {1\over 34\,\text {MeV}},&\nonumber \\ \lambda _\text {de Broglie}|_{\psi \rightarrow D^+D^-}&= 0.012\,\text {MeV}^{-1} = {1\over 83\,\text {MeV}},\nonumber \\ \lambda _\text {de Broglie}|_{\psi \rightarrow D^0 \overline{D^0}}&= 0.011\,\text {MeV}^{-1} = {1\over 91\,\text {MeV}},&\nonumber \\ \lambda _\text {de Broglie}|_{\Upsilon \rightarrow B^+B^-}&= 0.0090\,\text {MeV}^{-1} = {1\over 0.11\,\text {GeV}},\nonumber \\ \lambda _\text {de Broglie}|_{\Upsilon \rightarrow B^0 \overline{B^0}}&= 0.0091\,\text {MeV}^{-1} = {1\over 0.11\,\text {GeV}}. \end{aligned}$$

  (56)

  The theoretically excluded region $\sqrt{\sigma _P}<\lambda _\text {de Broglie}$ is depicted by the hatched region.

• The differences in the P-factors between the charged meson and neutral meson final states are sizable for higher-$\sqrt{\sigma _P}$ regions.

Fig. 4

The distributions of the P-factors (54) representing $\psi \rightarrow D^+ D^-$ are shown as functions of $\left( \sigma _D\right) ^{1/2}$ and $R_0$, where the bulk, boundary, and interference ones are shown by the orange, magenta, and green color, respectively

Fig. 5

We show the P-factors (54) for $\rho ^0 \rightarrow \pi ^+ \pi ^-$ (left panel) and the difference between the P-factors for $\rho ^0 \rightarrow \pi ^+ \pi ^-$ and $\rho ^+ \rightarrow \pi ^+ \pi ^0$ (right panel), with a typical value $0.0015\,\text {MeV}^{-1}=\left( 0.67\,\text {GeV}\right) ^{-1}$ for $R_0$; see Appendix A. We set the lowest $\sqrt{\sigma _\pi }$ near the bound from the de Broglie wavelength $\simeq 0.012\,\text {MeV}^{-1}$

To clarify the dependence on $R_0$, we prepare the surface plots for the bulk, boundary, and interference parts of the P-factors for $\Psi \rightarrow D^+ D^-$ as a typical example as Fig. 4. Here, the following properties are observed: (i) The magnitude of each part becomes larger for a smaller $\sqrt{\sigma _D}$ and a smaller $R_0$; (ii) In the entire domain of $\sqrt{\sigma _D}$ and $R_0$, the boundary part exceeds the bulk part in magnitude.

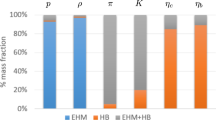
In Fig. 5, we have also plotted the P-factors for $\rho ^0 \rightarrow \pi ^+ \pi ^-$ and $\rho ^+ \rightarrow \pi ^+ \pi ^0$ by adopting the same formulas (41), (45), and (48) for a qualitative comparison between the decays with narrow phase spaces ($\phi$, $\psi$, and $\Upsilon$) and that with broad phase spaces ($\rho$), knowing that it is speculative whether we can still use the nonrelativistic approximation.²⁰ As expected, the difference between $\rho ^0 \rightarrow \pi ^+ \pi ^-$ and $\rho ^+ \rightarrow \pi ^+ \pi ^0$ is small since the magnitude of the isospin breaking is much smaller even for a smaller $\sqrt{\sigma _\pi }$.

4 Analysis of ratio of decay probabilities $R_V$

In this section, we discuss the ratio $R_V$ of decay probabilities for three vector mesons $\phi$, $\psi$, and $\Upsilon$ in the wave-packet formalism. We compare each with the PDG result and find an agreement around a reasonable value of $R_0$ of the form factor (for the compositeness of V). In particular, the 9.5 $\sigma$ discrepancy for $\psi$ is dramatically ameliorated. We find that the effect of the form factor is significant in both the wave-packet and plane-wave formalisms.

4.1 Wave-packet analysis

We estimate the wave-packet counterparts of the ratio of the decay rates defined in Eq. (1) for the three vector mesons as

$$\begin{aligned} R_\phi ^\text {WP}&:= {P_{\phi \rightarrow K^+K^-}\over P_{\phi \rightarrow K^0 \overline{K^0}}},\nonumber \\ R_\psi ^\text {WP}&:= {P_{\psi \rightarrow D^+D^-}\over P_{\psi \rightarrow D^0\overline{D^0}}},\nonumber \\ R_\Upsilon ^\text {WP}&:= {P_{\Upsilon \rightarrow B^+B^-}\over P_{\Upsilon \rightarrow B^0\overline{B^0}}}, \end{aligned}$$

(57)

where we ignored the tiny CP violation effect in $\phi \rightarrow K^0_\text {L}K^0_\text {S}$. We note that the ratio does not depend on the wave-function (field) renormalization factor $N_V$ (accounting for the off-shellness of the vector meson V), nor on the decay factor $e^{ -\Gamma _V\left( T_{\textrm{in}}- {T_0} \right) }$. Also, we take the isospin-symmetric limit in the couplings as introduced in Eq. (8), and the dependence on the coupling is dropped off. For further comparison, we also define a “bulk” ratio as

$$\begin{aligned} R_V^\text {bulk} :=&\ \frac{P^\text {bulk}_{V \rightarrow P^+ P^-}}{P^\text {bulk}_{V \rightarrow P^0 \overline{P^0}}}, \end{aligned}$$

(58)

which contains the wave-packet contribution only from the bulk part. Also, we introduce the ratio without interference as

$$\begin{aligned} R_V^\text {without interference} :=&\ \frac{P^\text {bulk}_{V \rightarrow P^+ P^-} + P^\text {bdry}_{V \rightarrow P^+ P^-}}{P^\text {bulk}_{V \rightarrow P^0 \overline{P^0}} + P^\text {bdry}_{V \rightarrow P^0 \overline{P^0}}}. \end{aligned}$$

(59)

Fig. 6

The ratio comparing the decay rates of $\phi \rightarrow K^+K^-$ to $\phi \rightarrow K^0\overline{K^0}$ is drawn as a function of $R_0$ for two fixed wave-packet sizes of the kaons of $\sqrt{\sigma _K} = 1\,\text {MeV}^{-1}$ (left panel) and $\sqrt{\sigma _K} = 0.1\,\text {MeV}^{-1}$ (right panel). The experimental result is provided by the PDG [1] [shown in Eq. (2)]

Fig. 7

The ratio comparing the decay rates of $\psi \rightarrow D^+D^-$ to $\psi \rightarrow D^0\overline{D^0}$ is drawn as a function of $R_0$ for two fixed wave-packet sizes of the D-mesons of $\sqrt{\sigma _D} = 1\,\text {MeV}^{-1}$ (left panel) and $\sqrt{\sigma _D} = 0.01\,\text {MeV}^{-1}$ (right panel). The experimental result is provided by the PDG [1] [shown in Eq. (2)]

Fig. 8

The ratio comparing the decay rates of $\Upsilon \rightarrow B^+B^-$ to $\Upsilon \rightarrow B^0\overline{B^0}$ is drawn as a function of $R_0$ for two fixed wave-packet sizes of the B-mesons of $\sqrt{\sigma _B} = 1\,\text {MeV}^{-1}$ (left panel) and $\sqrt{\sigma _B} = 0.01\,\text {MeV}^{-1}$ (right panel). The experimental result is provided by the PDG [1] [shown in Eq. (2)]

4.2 Wave-packet results

In Figs. 6, 7, and 8, we show the wave-packet results for the decay probability ratios of $\phi$, $\psi$, and $\Upsilon$, respectively. Several comments are in order.

• For the $\phi$ decay in Fig. 6, the full wave-packet result in the red solid line can fit the PDG result around the form-factor size $R_0\simeq 2\times 10^{-3}\,\text {MeV}^{-1}$ and $6\times 10^{-3}\,\text {MeV}^{-1}$ for the wave-packet size of the decay product ${\sqrt{\sigma _K}} = 1\,\text {MeV}^{-1}$ and $0.1\,\text {MeV}^{-1}$, respectively.

• For the $\psi$ decay in Fig. 7, the full wave-packet result in the red solid line can fit the PDG result around the form-factor size $R_0\simeq 2 \times 10^{-3}\,\text {MeV}^{-1}$ and $3 \times 10^{-3}\,\text {MeV}^{-1}$ for the wave-packet size of the decay product ${\sqrt{\sigma _D}}= 1\,\text {MeV}^{-1}$ and $0.01\,\text {MeV}^{-1}$, respectively.

• For the $\Upsilon$ decay in Fig. 8, the full wave-packet result in the red solid line can fit the PDG result around the form-factor size $R_0\simeq 2 \times 10^{-3}\,\text {MeV}^{-1}$ and $2 \times 10^{-3}\,\text {MeV}^{-1}$ for the wave-packet size of the decay product ${\sqrt{\sigma _B}}=1\,\text {MeV}^{-1}$ and $0.01\,\text {MeV}^{-1}$, respectively.

• As discussed in Fig. 3, if $\sqrt{\sigma _P}$ is sufficiently large, the bulk contribution becomes exponentially suppressed compared to the boundary one. In this regime, we may still formally evaluate the ratio between the (exponentially small) bulk contributions of $P^0$ and $P^-$ as

  $$\begin{aligned}&R_V^\text {bulk} \sim \frac{ e^{- F^0_\text {bulk}|_{\text {for }P^+} } }{ e^{- F^0_\text {bulk}|_{\text {for } P^0} } }\nonumber \\&\sim {\left\{ \begin{array}{ll} e^{12\,\text {MeV}^2\, \sigma _K} &{}\text {for } V = \phi ,\\ e^{- 1.0 \times 10^3\,\text {MeV}^2 \, \sigma _D} &{}\text {for } V = \psi , \\ e^{3.5 \times 10^2\,\text {MeV}^2\, \sigma _B} &{}\text {for } V = \Upsilon , \end{array}\right. } \end{aligned}$$

  (60)

  where the exponent is from Eq. (43). This ratio becomes either exponentially large or small due to the mass difference between $P^+$ and $P^0$, where the magnitude of the exponents is much greater than $\mathcal{O}\!\left( 1\right)$. For example, we obtain $\sigma _P\gtrsim 10\,\text {MeV}^{-2}$ and $100\,\text {MeV}^{-2}$ if we estimate $\sqrt{\sigma _P}$ to be larger than the smallest radius of an electron in atoms that interact with decay products of P, namely, the Bohr radius divided by a typical atomic number of the detector atoms, say, $a_\text {B}/Z\simeq 3\,\text {MeV}^{-1}$ and $10\,\text {MeV}^{-1}$ for lead and iron with $Z=82$ and 26, respectively. As introduced, the experimental results of $R_\phi$, $R_\psi$, and $R_\Upsilon$ are around unity, and they disagree with $R_V^\text {bulk}$, both for $\phi$ and $\psi$. So the $R_V^\text {bulk}$ curves are completely out of the depicted ranges of the left panels of Figs. 6, 7, and 8.

4.3 Plane-wave analysis

For a comparison with the wave-packet results, we also show results with the plane-wave decay rate $\Gamma ^\text {plane}$ (see Eq. (168) in Appendix C), taking into account the relativistic form factor (103). The resultant plane-wave ratio becomes

$$\begin{aligned} R_V^\text {plane}&:= \frac{\Gamma ^\text {plane}_{V \rightarrow P^+P^-}}{\Gamma ^\text {plane}_{V \rightarrow P^0 \overline{P^0}}} = R_V^\text {parton} \left| \frac{ \left( \frac{R_0 \left( m_V^2 - 4 m_{P^0}^2 \right) ^{1/2} }{2}\right) ^2 + 1 }{ \left( \frac{R_0 \left( m_V^2 - 4 m_{P^+}^2 \right) ^{1/2} }{2}\right) ^2 + 1 } \right| ^2, \end{aligned}$$

(61)

where the parton-level contribution to the ratio is

$$\begin{aligned} R_V^\text {parton} := \left( \frac{m_V^2 - 4 m_{P^+}^2}{m_V^2 - 4 m_{P^0}^2} \right) ^{3/2}, \end{aligned}$$

(62)

and the other factor is from the relativistic form factor (103) written in terms of the masses and $R_0$.

For another comparison, we will also show analyses using its nonrelativistic approximated form as

$$\begin{aligned} R_V^\text {plane}&\Rightarrow R_V^\text {plane, non-rel} \nonumber \\&:= \frac{ m_{P^+}^{1/2} \left( m_V - 2 m_{P^+} \right) ^{3/2} }{ m_{P^0}^{1/2} \left( m_V - 2 m_{P^0} \right) ^{3/2} } \left| \frac{ \left( \frac{R_0 m_{P^0} \left| {\varvec{V}}_1 - {\varvec{V}}_2\right| _{P^0} }{2}\right) ^2 + 1 }{ \left( \frac{R_0 m_{P^+} \left| {\varvec{V}}_1 - {\varvec{V}}_2\right| _{P^+} }{2}\right) ^2 + 1 } \right| ^2, \end{aligned}$$

(63)

with

$$\begin{aligned} \left| {\varvec{V}}_1 - {\varvec{V}}_2\right| _{P^+}&\approx \frac{ 2\left( m_V - 2m_{P^+}\right) ^{1/2} }{ m_{P^+}^{1/2} },\nonumber \\ \left| {\varvec{V}}_1 - {\varvec{V}}_2\right| _{P^0}&\approx \frac{ 2\left( m_V - 2m_{P^0}\right) ^{1/2} }{ m_{P^0}^{1/2} }, \end{aligned}$$

(64)

where “$\Rightarrow$” represents the operation of taking the nonrelativistic approximation, and $\approx$ denotes equality under the nonrelativistic approximation. The contributions from the form factor are not canceled out in $R_V^\text {plane}$.²¹ Note that the ratio (63) can be obtained from the wave-packet counterpart by taking the limits $\Gamma _V\rightarrow 0$ and $\sigma _P\rightarrow \infty$ in $\Gamma _VP_{V \rightarrow P\overline{P}}$; see Appendix D for details.

Fig. 9

The plane-wave ratio comparing the decay rates of $\phi \rightarrow K^+K^-$ to $\phi \rightarrow K^0\overline{K^0}$ is drawn as a function of $R_0$, where the captions “rel” and “Non-rel” mean the relativistic and nonrelativistic results shown in Eqs. (61) and (63), respectively. For comparison, we also show the wave-packet results for two fixed wave-packet sizes of the kaons of $\sqrt{\sigma _K} = 1\,\text {MeV}^{-1}$ (left panel) and $\sqrt{\sigma _K} = 0.1\,\text {MeV}^{-1}$ (right panel). In both panels, the plane-wave results are the same since they are independent of $\sigma _K$. The experimental result, shown in Eq. (2), is provided by the PDG [1]

Fig. 10

The plane-wave ratio comparing the decay rates of $\psi \rightarrow D^+D^-$ to $\psi \rightarrow D^0\overline{D^0}$ is drawn as a function of $R_0$. For comparison, we also show the wave-packet results for two fixed wave-packet sizes of the D-mesons of $\sqrt{\sigma _D} = 1\,\text {MeV}^{-1}$ (left panel) and $\sqrt{\sigma _D} = 0.01\,\text {MeV}^{-1}$ (right panel). The other conventions are the same as in Fig. 9

Fig. 11

The plane-wave ratio comparing the decay rates of $\Upsilon \rightarrow B^+B^-$ to $\Upsilon \rightarrow B^0\overline{B^0}$ is drawn as a function of $R_0$. For comparison, we also show the wave-packet results for two fixed wave-packet sizes of the B-mesons of $\sqrt{\sigma _B} = 1\,\text {MeV}^{-1}$ (left panel) and $\sqrt{\sigma _B} = 0.01\,\text {MeV}^{-1}$ (right panel). The other conventions are the same as in Fig. 9

4.4 Plane-wave results

We provide comments on the plane-wave results shown in Figs. 9, 10, and 11 below.

• For all of the vector mesons, $\phi$, $\psi$, and $\Upsilon$, the parton-level ratios (4) (under the isospin-symmetric limit for the couplings,²² without taking into account the form factor) are disfavored with the PDG’s central values at the level of $2.1\,\sigma$, $9.5\,\sigma$, and $0.32\,\sigma$, respectively.

• On the other hand, when the form factor effect is included, which is compulsory since the vector mesons are composite particles, we can see agreements with the PDG’s results. It suggests the importance of the form factor in addressing the ratio, where its effect is not fully canceled. Also, we can confirm that the nonrelativistic results approximate their relativistic counterparts well for the current system.

• We can find appropriate ranges of the form-factor parameter $R_0$, where theoretical predictions agree with the PDG’s results for both the full wave-packet and plane-wave curves. For each vector meson, the favored regions of $R_0$ for the wave packet and the plane wave are close to each other but different.

The calculation based on the plane wave works successfully, even though the presumption of free plane waves characterizing initial and final states is, at most, a viable approximation. The wave packet-based calculation provides a comprehensive approach, accounting for all aspects of the quantum nature inherent in the initial and final states, thereby enhancing its reliability. It would be important to precisely discuss the theoretically valid region of $R_0$, which depends on many details on the strong interaction. We leave this point for future research.

Note that we also briefly consider the isospin violation on the $\rho$ system, where the result is separately available in Appendix E since it might be out of our main interest.

5 Constraint from the shape of vector-meson resonances

In general, it is expected that the resonance shape of V is modified by the inclusion of the wave packet effects. That is, vector mesons, produced as resonances in electron positron colliders, are subjected to a shape-fitting process. This section addresses the constraint on the wave-packet size of pseudo-scalar mesons through the resonance shape of the process $e^-e^+ \rightarrow V \rightarrow P \overline{P}$. Sufficiently precise resonance data from experiments is available for $\phi$ and $\psi$, facilitating this objective. However, detailed resonance data for $\Upsilon$ is currently unavailable. Consequently, our focus is maintained on the instances of $\phi$ and $\psi$. Our analysis in this section is meant to be a brief consistency check, assuming the factorization of the production and decay processes of V in both the wave-packet and plane-wave formalisms, and hence is confined to data around the peak.

5.1 Invariant mass distribution of a decaying vector-meson wave packet

First, we summarize the invariant mass distribution of the decaying vector-meson when the Gaussian wave packet describes the decaying state.

We define a Lorentz-invariant mass squared $M^2$ for the pair of pseudo-scalars in the final state as

$$\begin{aligned} M^2&:= \left( E_1 + E_2\right) ^2 - \left( {{\varvec{P}}_1 + {\varvec{P}}_2} \right) ^2 \Rightarrow m_P^2 \left( 4 + V_-^2\right) , \end{aligned}$$

(65)

where $V_-$ is the magnitude of ${\varvec{V}}_-:={\varvec{V}}_1-{\varvec{V}}_2$ with ${\varvec{V}}_a={\varvec{P}}_a/E_a$ for $a=1,2$ [see Eq. (108) in Appendix B]. We will use

$$\begin{aligned} V_-^2 \approx \frac{1}{m_P^2} \left( M^2 - 4 m_P^2 \right) , \end{aligned}$$

(66)

which results in

$$\begin{aligned} V_-\text {d}V_-&\approx {M\text {d}M\over m_P^2} \simeq {m_V\text {d}M\over m_P^2}, \end{aligned}$$

(67)

where we have approximated that V decays at rest in the last step.

It is straightforward to derive the following forms after integrating Eq. (38) over the final state phase space, except for $V_-$, under the current nonrelativistic approximation, which is easily rewritten as the invariant mass distribution by use of Eq. (67):

$$\begin{aligned} \frac{\text {d}P_{V \rightarrow P \overline{P}}}{\text {d}M} = \frac{\text {d}P_{V \rightarrow P \overline{P}}^\text {bulk}}{\text {d}M} + \frac{\text {d}P_{V \rightarrow P \overline{P}}^\text {bdry}}{\text {d}M} + \frac{\text {d}P_{V \rightarrow P \overline{P}}^\text {intf}}{\text {d}M}, \end{aligned}$$

(68)

where

$$\begin{aligned}&\frac{\text {d}P_{V \rightarrow P \overline{P}}^\text {bulk}}{\text {d}M} \simeq \frac{2m_V}{m_P^2} \left( \frac{1}{16\sqrt{2\pi }} \mathcal{C}_{V \rightarrow P \overline{P}} \right) {m_P^2\sqrt{\sigma _P}\over \Gamma _V} V_-^2 \nonumber \\&\quad \qquad \qquad \qquad \times { \frac{ e^{ -F^0_\text {bulk} - \frac{m_P^2\sigma _P}{2}\left( V_- - V_-^\text {B}\right) ^2 } }{A_\text {bulk}^{3/2} } } \left| \widetilde{F}\!\left( V_-\right) \right| ^2, \end{aligned}$$

(69)

$$\begin{aligned}&\frac{\text {d}P_{V \rightarrow P \overline{P}}^\text {bdry}}{\text {d}M} \simeq \frac{2m_V}{m_P^2} \left( \frac{1}{4\pi } \mathcal{C}_{V \rightarrow P \overline{P}} \right) \frac{\widetilde{f}_\text {bdry}\!\left( V_-\right) }{V_-} \left| \widetilde{F}\!\left( V_-\right) \right| ^2, \end{aligned}$$

(70)

$$\begin{aligned}&\frac{\text {d}P_{V \rightarrow P \overline{P}}^\text {intf}}{\text {d}M} \simeq - \frac{2m_V}{m_P^2} \left( \frac{1}{8\sqrt{2} \pi } \mathcal{C}_{V \rightarrow P \overline{P}} \right) \left( \sigma _P m_P^2 \right) V_- \nonumber \\&\quad \times { \frac{e^{ -F^0_\text {intf} - \frac{1}{2} \left( \frac{m_P^2\sigma _P}{2}\right) \left( V_- - V_-^\text {I}\right) ^2 }}{A_\text {intf}^{3/2}} } \nonumber \\&\quad \times \left[ \frac{ \left( \Gamma _V^2 - \left( \delta \omega \right) ^2\right) \cos \!\left( 2 \Gamma _V\sigma _t\delta \omega \right) - 2 \Gamma _V\delta \omega \sin \!\left( 2 \Gamma _V\sigma _t\delta \omega \right) }{\left( \Gamma _V^2 + \left( {\delta \omega }\right) ^2 \right) ^2\sigma _t} \right] _{V_+ \rightarrow 0}\nonumber \\ {}&\quad \times \left| \widetilde{F}\!\left( V_-\right) \right| ^2. \end{aligned}$$

(71)

Here, we consider the distribution of M instead of $M^2$ due to the convenience of comparing the wave-packet shape with the nonrelativistic Breit–Wigner (BW) shape, which is the well-known resonant shape for the decaying plane wave with the decay rate $\Gamma _V$; see the next subsection.²³

We note that under the current setup $T_\text {out} \rightarrow \infty$, the factor $N_V^2$ in $\mathcal{C}_{V \rightarrow P \overline{P}}$ [recall Eq. (40)] can be determined by the normalization

$$\begin{aligned}&P^\text {bulk}_{V \rightarrow P \overline{P}} + P^\text {bdry}_{V \rightarrow P \overline{P}} + P^\text {intf}_{V \rightarrow P \overline{P}} \nonumber \\&\quad = \left( \text {the corresponding branching ratio}\right) \end{aligned}$$

(72)

that is obtained after integrating over M; see Eqs. (41), (45), and (48).

5.2 Breit–Wigner shape

For the plane-wave calculation, it is well-known that the nonrelativistic Breit–Wigner distribution nicely describes the shape of a narrow resonance:²⁴

$$\begin{aligned} f_\text {NR-BW}\!\left( E\right)&= \frac{\Gamma /\left( 2\pi \right) }{ \left( E-m_\text {res}\right) ^2 + {\Gamma }^2/4 }, \nonumber \\&\bigg ( \int _{-\infty }^{+\infty } \text {d}E \, f_\text {NR-BW}\!\left( E\right) = 1 \bigg ), \end{aligned}$$

(73)

where $m_\text {res}$, E, and $\Gamma$ are the resonant mass, the total energy in the center-of-the-mass frame, and the total width of an intermediate resonant particle, respectively. Note that

$$\begin{aligned} E = M. \end{aligned}$$

(74)

Since we used the nonrelativistic approximation, we are adopting the nonrelativistic Breit–Wigner shape (73) for comparison.

5.3 Method of analyzing the resonant shape

We assume the following factorization for the resonant production, where the cross section of the resonant production of V and its subsequent decay into P and $\overline{P}$, $\sigma _{e^-e^+ \rightarrow V \rightarrow P \overline{P}}$, can be factorized in the wave-packet (WP) and plane-wave (PW) formalisms, respectively, as

$$\begin{aligned} \sigma _{e^-e^+ \rightarrow V \rightarrow P \overline{P}}^\text {WP}\!\left( M\right)&= \mathcal{N}^\text {WP}_{e^-e^+ \rightarrow V} \frac{\text {d}P_{V \rightarrow P \overline{P}}}{\text {d}M}, \end{aligned}$$

(75)

$$\begin{aligned} \sigma _{e^-e^+ \rightarrow V \rightarrow P \overline{P}}^\text {PW-Parton}\!\left( M\right)&= \mathcal{N}^\text {PW-Parton}_{e^-e^+ \rightarrow V} f_\text {NR-BW}\!\left( M\right) , \end{aligned}$$

(76)

$$\begin{aligned} \sigma _{e^-e^+ \rightarrow V \rightarrow P \overline{P}}^\text {PW-FF}\!\left( M\right)&= \mathcal{N}^\text {PW-FF}_{e^-e^+ \rightarrow V} f_\text {NR-BW}\!\left( M\right) \nonumber \\ {}&\quad \times \left| \frac{1}{1+ {\frac{R_0^2 \left( M^2 - 4m_P^2\right) }{4}} } \right| ^2, \end{aligned}$$

(77)

where we consider the two cases for PW with and without the form factor (FF); the two cases are discriminated by the short-hand notations “PW-FF” and “PW-Parton.” For the form-factor part of (77), we used the relation in Eq. (66) to convert $V_-$ to M.

$\mathcal{N}^\text {WP}_{e^-e^+ \rightarrow V}$, $\mathcal{N}^\text {PW-Parton}_{e^-e^+ \rightarrow V}$, and $\mathcal{N}^\text {PW-FF}_{e^-e^+ \rightarrow V}$ possess the mass dimension of minus one and describe the factorized production part $e^-e^+ \rightarrow V$ via the $e^-e^+$ collision at the center-of-the-mass energy M. Here, we take these three factors to be independent of M since the primal structure of the resonance is in ${\text {d}P_{V \rightarrow P \overline{P}}}/{\text {d}M}$ or $f_\text {NR-BW}$, and we use only the data points near the peak of a resonance.²⁵ We will take $m_\text {res}$ and $\Gamma$ for $f_\text {NR-BW}\!\left( M\right)$ in Eq. (73) as $m_V$ and $\Gamma _V$, respectively; see also Eq. (74). The actual analysis for $e^-e^+ \rightarrow V \rightarrow P \overline{P}$ will be done in the following manner:

• We focus on the values of the experimentally given cross sections only around the resonant peak, namely, in $[m_V- \Gamma _V/2, m_V+ \Gamma _V/2]$ since the factorized forms in Eqs. (75), (76), and (77) may work only around the peak. Here, we will adopt the PDG values for $m_V$ and $\Gamma _V$ [1].

• In the analysis, we fix the values of $\Gamma _V$ and $m_P$ as confirmed by the PDG group [1], while we treat $m_V$ as an unfixed parameter and will determine it through our statistical fit. The isospin-symmetric coupling $g_V$ and the wave-function renormalization factor for V, $N_V$ are taken as unity since it can be absorbed into the factor $\mathcal{N}_{e^-e^+ \rightarrow V}$. Furthermore, for simplicity, we focus on $T_\text {in} = T_0$, where the exponential decay factor in $\mathcal{C}_{V \rightarrow P \overline{P}}$ in Eq. (40) does not work.

• Under the current scheme, $\sigma _{e^-e^+ \rightarrow V \rightarrow P \overline{P}}^\text {WP}$ has six parameters $\{ \mathcal{N}^\text {WP}_{e^-e^+ \rightarrow V},\, m_V, \, \Gamma _V, \, m_P, \, R_0, \, \sigma _P \}$, $\sigma _{e^-e^+ \rightarrow V \rightarrow P \overline{P}}^\text {PW-FF}$ has five parameters $\{ \mathcal{N}^\text {PW-FF}_{e^-e^+ \rightarrow V}, \, m_V, \, \Gamma _V, \, m_P,\, R_0 \}$, and $\sigma _{e^-e^+ \rightarrow V \rightarrow P \overline{P}}^\text {PW-Parton}$ has three parameters $\{ \mathcal{N}^\text {PW-Parton}_{e^-e^+ \rightarrow V}, \, m_V, \, \Gamma _V \}$. We will determine them through statistical analysis. We remind ourselves that the vector-meson wave-packet size $\sigma _V$ does not appear in $\sigma _{e^-e^+ \rightarrow V \rightarrow P \overline{P}}^\text {WP}$ under the saddle-point approximation.

5.4 Result of $\phi$

In Ref. [29], the latest result of the resonant shape of $\phi$ through $e^-e^+ \rightarrow \phi \rightarrow K^+ K^-$ measured with the CMD-3 detector in the center-of-mass energy range 1010–$1060\,\text {MeV}$ was reported, where the Born cross sections of $e^-e^+ \rightarrow \phi \rightarrow K^+ K^-$ around the resonance are available in Table I of [29]. According to our guideline, we adopt the seven data points from $1018.0\,\text {MeV}$ to $1021.3\,\text {MeV}$ and adopt the $\chi ^2$ functions:

$$\begin{aligned} \chi ^2_{\phi ,\, \text {WP}}&:= \sum _{i=1}^{7} \frac{\left( \sigma _i^\text {WP} - \sigma _i^\text {exp}\right) ^2}{\left( \delta \sigma _i^\text {exp}\right) ^2},&\nonumber \\ \chi ^2_{\phi ,\, \text {PW-Parton}}&:= \sum _{i=1}^{7} \frac{\left( \sigma _i^\text {PW-Parton} - \sigma _i^\text {exp}\right) ^2}{\left( \delta \sigma _i^\text {exp}\right) ^2},\nonumber \\ \chi ^2_{\phi ,\, \text {PW-FF}}&:= \sum _{i=1}^{7} \frac{\left( \sigma _i^\text {PW-FF} - \sigma _i^\text {exp}\right) ^2}{\left( \delta \sigma _i^\text {exp}\right) ^2}, \end{aligned}$$

(78)

where i discriminates the seven points of M where experimental data is available; $\sigma _i^\text {exp}$ and $\delta \sigma _i^\text {exp}$ are the central and error of the experimentally determined cross section at the point i, respectively. $\sigma _i^\text {WP}$, $\sigma _i^\text {PW-Parton}$, and $\sigma _i^\text {PW-FF}$ represent the theoretical values of the corresponding cross sections at the energy point identified by i.

Fig. 12

The fitted resonance distributions of $\phi$ in $e^-e^+ \rightarrow \phi \rightarrow K^+K^-$ are drawn for the two sets of the fixed parameters $\sqrt{\sigma _K} = 10\,\text {MeV}^{-1}$ and $R_0 = 0.0015 \, \text {MeV}^{-1}$ (left panel) and $\sqrt{\sigma _K} = 10\,\text {MeV}^{-1}$ and $R_0 = 0.01 \, \text {MeV}^{-1}$ (right panel), where the mass of $\phi$ and the normalization factor $\mathcal{N}_{e^-e^+ \rightarrow \phi }$ are determined through our statistical analysis based on the $\chi ^2$ function defined in Eq. (78). The best-fit parameters and the $\chi ^2$ functions for the left/right panels are shown in Eqs. (79) and (80)/and in Eqs. (81) and (82), respectively, for the wave-packet (WP) and plane-wave without/with form factor (BW/BW with FF)

As examples, we show the fitted distributions for the two sets of the fixed parameters $\sqrt{\sigma _K} = 10\,\text {MeV}^{-1}$ and $R_0 = 0.0015 \, \text {MeV}^{-1}$ for the left panel of Fig. 12,²⁶$\sqrt{\sigma _K} = {10}\,\text {MeV}^{-1}$ and $R_0 = 0.01 \, \text {MeV}^{-1}$ for the right panel of Fig. 12, where the two remaining parameters $\{ \mathcal{N}_{e^-e^+ \rightarrow \phi },\, m_\phi \}$ take the best-fit values, and the values of the $\chi ^2$ over the degrees of freedoms (DOFs), which is currently five, at the best-fit points are calculated as

• for $\sqrt{\sigma _K} = 10\,\text {MeV}^{-1}$ and $R_0 = 0.0015 \, \text {MeV}^{-1}$ (left panel of Fig. 12):

  $$\begin{aligned} \left. m_\phi ^\text {WP}\right| _\text {best fit}&= 1019.8 \, \text {MeV},\nonumber \\ \left. \mathcal{N}_{e^-e^+ \rightarrow \phi }^\text {WP}\right| _\text {best fit}&= 6.23 \times 10^{5} \,\text {MeV}^{-1}, \nonumber \\ \left. m_\phi ^\text {PW-Parton}\right| _\text {best fit}&= 1019.4 \, \text {MeV},\nonumber \\ \left. \mathcal{N}_{e^-e^+ \rightarrow \phi }^\text {PW-Parton}\right| _\text {best fit}&= 1.50 \times 10^{4} \,\text {MeV}^{-1}, \nonumber \\ \left. m_\phi ^\text {PW-FF}\right| _\text {best fit}&= 1019.4 \, \text {MeV},\nonumber \\ \left. \mathcal{N}_{e^-e^+ \rightarrow \phi }^\text {PW-FF}\right| _\text {best fit}&= 1.62 \times 10^{4} \,\text {MeV}^{-1}, \end{aligned}$$

  (79)
  $$\begin{aligned} \left. \frac{\chi ^2_{\phi ,\, \text {WP}}}{(\text {DOFs})}\right| _\text {best fit}&\simeq 5.5,\nonumber \\ \left. \frac{\chi ^2_{\phi ,\, \text {PW-Parton}}}{(\text {DOFs})}\right| _\text {best fit}&\simeq 6.3,\nonumber \\ \left. \frac{\chi ^2_{\phi ,\, \text {PW-FF}}}{(\text {DOFs})}\right| _\text {best fit}&\simeq 6.7, \end{aligned}$$

  (80)

• for $\sqrt{\sigma _K} = 10\,\text {MeV}^{-1}$ and $R_0 = 0.01 \, \text {MeV}^{-1}$ (right panel of Fig. 12),

  $$\begin{aligned} \left. m_\phi ^\text {WP}\right| _\text {best fit}&= 1019.9 \, \text {MeV},\nonumber \\ \left. \mathcal{N}_{e^-e^+ \rightarrow \phi }^\text {WP}\right| _\text {best fit}&= 3.94 \times 10^{6} \,\text {MeV}^{-1}, \nonumber \\ \left. m_\phi ^\text {PW-Parton}\right| _\text {best fit}&= 1019.4 \, \text {MeV},\nonumber \\ \left. \mathcal{N}_{e^-e^+ \rightarrow \phi }^\text {PW-Parton}\right| _\text {best fit}&= 1.51 \times 10^{4} \,\text {MeV}^{-1}, \nonumber \\ \left. m_\phi ^\text {PW-FF}\right| _\text {best fit}&= 1019.6 \, \text {MeV},\nonumber \\ \left. \mathcal{N}_{e^-e^+ \rightarrow \phi }^\text {PW-FF}\right| _\text {best fit}&= 1.03 \times 10^{5} \,\text {MeV}^{-1}, \end{aligned}$$

  (81)
  $$\begin{aligned} \left. \frac{\chi ^2_{\phi ,\, \text {WP}}}{(\text {DOFs})}\right| _\text {best fit}&\simeq 14,\nonumber \\ \left. \frac{\chi ^2_{\phi ,\, \text {PW-Parton}}}{(\text {DOFs})}\right| _\text {best fit}&\simeq 6.3,\nonumber \\ \left. \frac{\chi ^2_{\phi ,\, \text {PW-FF}}}{(\text {DOFs})}\right| _\text {best fit}&\simeq 14. \end{aligned}$$

  (82)

We comment on the difference between the plane-wave resonant shapes with and without the form factor. Without the factor, the shape obeys the Breit–Wigner distribution (73) and is symmetric under the reflection around the peak ($M = m_\phi$); while taking it into account, the resonant shape becomes asymmetric under the reflection around the peak. The magnitude of the asymmetry is governed by the part $R_0^2 \left( M^2 - 4 m_{K^+}^2\right)$ of the form factor. So for a greater $R_0$, a more significant asymmetry will be realized, as observed in Fig. 12.

Fig. 13

We plot the variable $\left. {\chi ^2_{\phi }}/{(\text {DOFs})}\right| _\text {min}$ defined in Eq. (83) to compare the significance of the wave-packet calculation with the plane-wave one for various $\sqrt{\sigma _K}$. Here, $R_0$ is fixed as $0.0015 \, \text {MeV}^{-1}$, and for each $\sqrt{\sigma _K}$, $m_\phi$ and $\mathcal{N}_{e^-e^+ \rightarrow \phi }$ are determined to (locally) minimize the corresponding $\chi ^2$ function in Eq. (78). The black curve and the blue dashed horizontal line describe the values in the wave-packet and plane-wave calculations, respectively, without form factor, where the latter is manifestly independent of $\sqrt{\sigma _K}$

Here, we comment on the origin of the “over-$5\sigma$” values of $\chi ^2/(\text {DOFs})$: this is because the resolution of the experimental results near the peak is very high, and the current simple scheme for $\sigma _{e^-e^+ \rightarrow V \rightarrow P\overline{P}}$ in Eqs. (75), (76), and (77) is not enough for discussing statistical significance precisely. On the other hand, however, we are able to discuss the relative significance between the wave-packet and plane-wave results. According to Eq. (80), the shape of the wave-packet resonant distribution is at least as good as that of the plane-wave resonant distribution at the focused parameter point, where we conclude that the wave-packet result at the first parameter point (for the left panel of Fig. 12) is consistent with the experiment. Note that at the first parameter point, $R_0$ is taken as a typical value in the current scheme of the form factor (see Appendix A), and a wave packet with a greater size looks similar to the plane wave.

We also see the significance of the wave-packet results over a broad range of $\sqrt{\sigma _K}$ under $R_0 = 0.0015 \, \text {MeV}^{-1}$. In Fig. 13, we plot the “minimized” $\chi ^2/(\text {DOFs})$ defined by

$$\begin{aligned} \left. \frac{\chi ^2_{\phi ,\, \text {WP/PW-Parton}}}{(\text {DOFs})}\right| _\text {min} := \min _{m_\phi ,\ \mathcal{N}_{e^-e^+ \rightarrow \phi }^\text {WP/PW-Parton}} \left[ \frac{\chi ^2_{\phi ,\, \text {WP/PW-Parton}}}{(\text {DOFs})} \right] , \end{aligned}$$

(83)

which measures the statistical significance for $\sqrt{\sigma _K}$. We do not consider the PW-FF case since no sizable difference is generated when $R_0 = 0.0015 \, \text {MeV}^{-1}$, as shown in the left panel of Fig. 12, and the form factor part does not depend on $\sqrt{\sigma _K}$. Under the simple guideline that a wave-packet result is at least as good as the ordinary plane-wave one, from Fig. 13, we can put the lower bound on $\sqrt{\sigma _K}$ as

$$\begin{aligned} \sqrt{\sigma _K} \gtrsim 3\,\text {MeV}^{-1} \end{aligned}$$

(84)

for $R_0 = 0.0015 \, \text {MeV}^{-1}$.

5.5 Result of $\psi$

The BaBar, Belle, BES, and CLEO experimental collaborations have provided recent experimental data of the $\psi$’s resonance produced by the $e^-e^+$ collision.

• We will adopt the results on [30] of the converting experimental results to the exclusive initial-state-radiation scattering cross section, $e^+ e^- \rightarrow 2D$, where the following experimental papers are taken into account by BaBar [31], by Belle [32], and by CLEO [33, 34]. “2D” means the inclusive final states of $D^+ D^-$ and $D^0 \overline{D^0}$.²⁷

• We also take account of the experimental results by BES of the inclusive hadron production cross section in [37, 38]. The original data is provided in the form,

  $$\begin{aligned} R(s) = \frac{\sigma ^0_\text {had}(s)}{\sigma ^0_{\mu ^+\mu ^-}(s)}, \end{aligned}$$

  (85)

  where $\sigma ^0_{\mu ^+\mu ^-}(s) = 4\pi \alpha ^2\!\left( 0\right) /3s$ is the lowest-order QED cross section for muon pair production at the total center-of-mass energy $E = \sqrt{s}$. $\alpha \!\left( 0\right) \simeq 1/137$ is the QED fine structure constant at the Thomson limit. $R_{uds(c)+\psi (3770)}$ and $R_{uds}$ are reported in [37] and in [38], respectively. Through the approximation $R_{\psi (3770)} \simeq R_{uds(c)+\psi (3770)} - R_{uds}$, we can recast the cross section of $e^+ e^- \rightarrow 2D$, as done in [39].

Since the final state is inclusive as 2D, we adopt the following factorized form for the production cross section:

$$\begin{aligned}&\sigma _{e^-e^+ \rightarrow \psi \rightarrow 2D}^\text {WP}\!\left( M\right) \nonumber \\&\quad = \mathcal{N}^\text {WP}_{e^-e^+ \rightarrow \psi } \left( \frac{\text {d}P_{\psi \rightarrow D^+ D^-}}{\text {d}M} + \frac{\text {d}P_{\psi \rightarrow D^0 \overline{D^0}}}{\text {d}M} \right) , \end{aligned}$$

(86)

$$\begin{aligned}&\sigma _{e^-e^+ \rightarrow \psi \rightarrow 2D}^\text {PW-Parton}\!\left( M\right) = \mathcal{N}^\text {PW-Parton}_{e^-e^+ \rightarrow \psi } \, f_\text {NR-BW}\!\left( M\right) , \end{aligned}$$

(87)

$$\begin{aligned}&\sigma _{e^-e^+ \rightarrow \psi \rightarrow 2D}^\text {PW-FF}\!\left( M\right) = \mathcal{N}^\text {PW-FF}_{e^-e^+ \rightarrow \psi } \, f_\text {NR-BW}\!\left( M\right) \nonumber \\&\quad \times \left[ \text {Br}_{\psi \rightarrow D^+D^-} \left| \frac{1}{1+ {\frac{R_0^2 \left( M^2 - 4m_{D^+}^2\right) }{4}} } \right| ^2 \right. \nonumber \\&\quad \left. + \text {Br}_{\psi \rightarrow D^0\overline{D^0}} \left| \frac{1}{1+ {\frac{R_0^2 \left( M^2 - 4m_{D^0}^2\right) }{4}} } \right| ^2 \right] , \end{aligned}$$

(88)

where $\text {Br}_{\psi \rightarrow D^+D^-} = 0.41$ and $\text {Br}_{\psi \rightarrow D^0\overline{D^0}} = 0.52$ are the corresponding branching ratios [1]. The $\chi ^2$ functions are defined as

$$\begin{aligned}&\chi ^2_{\psi ,\, \text {WP}} \nonumber \\&\quad := \sum _{\text {I: BaBar, Belle, BES, CLEO}} \sum _{i_\text {I}} \frac{\left( \sigma _{i_\text {I}}^\text {WP} - \sigma _{i_\text {I}}^\text {exp,I} \right) ^2}{\left( \delta \sigma _{i_\text {I}}^\text {exp,I} \right) ^2}, \nonumber \\&\chi ^2_{\psi ,\, \text {PW-Parton}} \nonumber \\&\quad := \sum _{\text {I: BaBar, Belle, BES, CLEO}} \sum _{i_\text {I}} \frac{\left( \sigma _{i_\text {I}}^\text {PW-Parton} - \sigma _{i_\text {I}}^\text {exp,I} \right) ^2}{\left( \delta \sigma _{i_\text {I}}^\text {exp,I} \right) ^2}, \nonumber \\&\chi ^2_{\psi ,\, \text {PW-FF}} \nonumber \\&\quad := \sum _{\text {I: BaBar, Belle, BES, CLEO}} \sum _{i_\text {I}} \frac{\left( \sigma _{i_\text {I}}^\text {PW-FF} - \sigma _{i_\text {I}}^\text {exp,I} \right) ^2}{\left( \delta \sigma _{i_\text {I}}^\text {exp,I} \right) ^2}, \end{aligned}$$

(89)

where $\sigma _{i_\text {I}}^\text {exp,I}$ and $\delta \sigma _{i_\text {I}}^\text {exp,I}$ are the central and error of the experimentally determined cross section at the point $i_\text {I}$ of the experiment $\text {I}$, respectively. $\sigma _{i_\text {I}}^\text {WP}$, $\sigma _{i_\text {I}}^\text {PW-Parton}$, and $\sigma _{i_\text {I}}^\text {PW-FF}$ represent the theoretical values of the corresponding cross sections at the energy point identified by ${i_\text {I}}$.

Fig. 14

The fitted resonance distributions of $\phi$ in $e^-e^+ \rightarrow \psi \rightarrow 2D$ are drawn for the fixed parameters $\sqrt{\sigma _D} = 1\,\text {MeV}^{-1}$ and $R_0 = 0.0015 \, \text {MeV}^{-1}$ (for the left panel), and $\sqrt{\sigma _D} = 1\,\text {MeV}^{-1}$ and $R_0 = 0.01 \, \text {MeV}^{-1}$ (for the right panel), where the mass of $\psi$ and the normalization factor $\mathcal{N}_{e^-e^+ \rightarrow \psi }$ are determined through our statistical analysis based on the $\chi ^2$ function defined in Eq. (89). The best-fit parameters and the $\chi ^2$ functions for the left/right panels are shown in Eqs. (90) and (91)/and in Eqs. (92) and (93), respectively. The other conventions are the same as those of Fig. 12

Here, we will see the two examples for the same wave-packet size $\sqrt{\sigma _D} = 1\,\text {MeV}^{-1}$ but two different values for the form-factor parameter $R_0$. In the left and right panels of Fig. 14, the fitted distributions about the parameters $\{ \mathcal{N}_{e^-e^+ \rightarrow \psi },\, m_\psi \}$ are shown for $R_0 = 0.0015\,\text {MeV}^{-1}$ and $R_0 = 0.01\,\text {MeV}^{-1}$, respectively, where the valid ranges of $\mathcal{N}_{e^-e^+ \rightarrow \psi }$ and $m_\psi$ are fixed as

• for $\sqrt{\sigma _D} = 1\,\text {MeV}^{-1}$ and $R_0 = 0.0015\,\text {MeV}^{-1}$ (left panel of Fig. 14),

  $$\begin{aligned} \left. m_\psi ^\text {WP}\right| _\text {best fit}&= 3770.4 \, \text {MeV},\nonumber \\ \left. \mathcal{N}_{e^-e^+ \rightarrow \psi }^\text {WP}\right| _\text {best fit}&= 1.02 \times 10^5 \,\text {MeV}^{-1}, \nonumber \\ \left. m_\psi ^\text {PW-Parton}\right| _\text {best fit}&= 3774.6 \, \text {MeV},\nonumber \\ \left. \mathcal{N}_{e^-e^+ \rightarrow \psi }^\text {PW-Parton}\right| _\text {best fit}&= 3.90 \times 10^2 \,\text {MeV}^{-1}, \nonumber \\ \left. m_\psi ^\text {PW-FF}\right| _\text {best fit}&= 3775.5 \, \text {MeV},\nonumber \\ \left. \mathcal{N}_{e^-e^+ \rightarrow \psi }^\text {PW-FF}\right| _\text {best fit}&= 5.77 \times 10^2 \,\text {MeV}^{-1}, \end{aligned}$$

  (90)
  $$\begin{aligned} \left. \frac{\chi ^2_{\phi ,\, \text {WP}}}{(\text {DOFs})}\right| _\text {best fit}&\simeq 0.92,\nonumber \\ \left. \frac{\chi ^2_{\phi ,\, \text {PW-Parton}}}{(\text {DOFs})}\right| _\text {best fit}&\simeq 0.91,\nonumber \\ \left. \frac{\chi ^2_{\phi ,\, \text {PW-FF}}}{(\text {DOFs})}\right| _\text {best fit}&\simeq 0.91. \end{aligned}$$

  (91)

• for $\sqrt{\sigma _D} = 1\,\text {MeV}^{-1}$ and $R_0 = 0.01\,\text {MeV}^{-1}$ (right panel of Fig. 14),

  $$\begin{aligned} \left. m_\psi ^\text {WP}\right| _\text {best fit}&= 3775.7 \, \text {MeV},\nonumber \\ \left. \mathcal{N}_{e^-e^+ \rightarrow \psi }^\text {WP}\right| _\text {best fit}&= 4.92 \times 10^6 \,\text {MeV}^{-1}, \nonumber \\ \left. m_\psi ^\text {PW-Parton}\right| _\text {best fit}&= 3774.6 \, \text {MeV},\nonumber \\ \left. \mathcal{N}_{e^-e^+ \rightarrow \psi }^\text {PW-Parton}\right| _\text {best fit}&= 3.90 \times 10^2 \,\text {MeV}^{-1}, \nonumber \\ \left. m_\psi ^\text {PW-FF}\right| _\text {best fit}&= 3780.0 \, \text {MeV},\nonumber \\ \left. \mathcal{N}_{e^-e^+ \rightarrow \psi }^\text {PW-FF}\right| _\text {best fit}&= 3.36 \times 10^4 \,\text {MeV}^{-1}, \end{aligned}$$

  (92)
  $$\begin{aligned} \left. \frac{\chi ^2_{\phi ,\, \text {WP}}}{(\text {DOFs})}\right| _\text {best fit}&\simeq 0.92,\nonumber \\ \left. \frac{\chi ^2_{\phi ,\, \text {PW-Parton}}}{(\text {DOFs})}\right| _\text {best fit}&\simeq 0.91,\nonumber \\ \left. \frac{\chi ^2_{\phi ,\, \text {PW-FF}}}{(\text {DOFs})}\right| _\text {best fit}&\simeq 0.92. \end{aligned}$$

  (93)

From Fig. 14, when $R_0$ is large as $\sim 10^{-2}\,\text {MeV}^{-1}$, the resonant distribution of the wave packet becomes identical with that of the plane-wave without taking into account the form factor. Meanwhile, when $R_0 \sim 10^{-3}\,\text {MeV}^{-1}$, where this size is favored with the agreement in $R_\psi$, we observe the deviation from the BW shape in the wave-packet distribution. Note that all three kinds of distributions agree with the experimental data for the larger and smaller $R_0$.

We comment on the large asymmetry observed in the right panel of Fig. 14, namely, the large deviation of “BW with FF” in the low M range. As mentioned in the previous subsection, the asymmetry under the reflection around the peak originates from the parts $R_0^2 \left( M^2 - 4 m_{D^+}^2\right)$ and $R_0^2 \left( M^2 - 4 m_{D^0}^2\right)$ of the form factors. The realized asymmetry in $R_0 = 0.01 \, \text {MeV}^{-1}$ becomes extensive when M is less than the range used for the statistical fit, so this case is considered to be disfavored even though the limited part near the resonant peak is fitted to the experimental results well.

Fig. 15

We plot the variable $\left. {\chi ^2_{\psi }}/{(\text {DOFs})}\right| _\text {min}$ defined in Eq. (94) to compare the significance of the wave-packet calculation with the plane-wave one for various $\sqrt{\sigma _D}$. Here, $R_0$ is fixed as $0.0015 \, \text {MeV}^{-1}$, and for each $\sqrt{\sigma _D}$, $m_\psi$ and $\mathcal{N}_{e^-e^+ \rightarrow \psi }$ are determined to (locally) minimize the corresponding $\chi ^2$ function in Eq. (89). The black curve and blue dashed horizontal line describe the values in the wave-packet and plane-wave calculations, where the latter is manifestly independent of $\sqrt{\sigma _D}$

To clarify the experimentally valid range for $\sqrt{\sigma _D}$, we see the curve of the “minimized” $\chi ^2/(\text {DOFs})$ defined by

$$\begin{aligned} \left. \frac{\chi ^2_{\psi ,\, \text {WP/PW-Parton}}}{(\text {DOFs})}\right| _\text {min} := \min _{m_\psi ,\ \mathcal{N}_{e^-e^+ \rightarrow \psi }^\text {WP/PW-Parton}} \left[ \frac{\chi ^2_{\psi ,\, \text {WP/PW-Parton}}}{(\text {DOFs})} \right] , \end{aligned}$$

(94)

for $R_0 = 0.0015\,\text {MeV}^{-1}$. Due to the same reason with the case of $\phi$ for $R_0 = 0.0015\,\text {MeV}^{-1}$, we skip to consider the PW-FF case. From Fig. 15, we recognize that the range for $\sqrt{\sigma _D}$ is consistent with the constraint

$$\begin{aligned} \left. \frac{\chi ^2_{\psi ,\, \text {WP/PW-Parton}}}{(\text {DOFs})}\right| _\text {min} < 2, \end{aligned}$$

even though the wave-packet shape does not exceed the BW shape in the goodness of fit. To summarize, within the current scheme for the production cross section, no significant bounds on $\sqrt{\sigma _D}$ are imposed. This is because, as recognized from Fig. 14, the experimental results still have sizable errors for the $\psi$’s resonant shape.

6 Summary and discussion

In this paper, we have discussed the long-standing anomaly in the ratio of the decay rates of the vector mesons $\phi$ and $\psi$, namely, $R_\phi = \Gamma \!\left( \phi \rightarrow K^+ K^-\right) /\Gamma \!\left( \phi \rightarrow K^0_\text {L} K^0_\text {S}\right)$ and $R_\psi = \Gamma \!\left( \psi \rightarrow D^+ D^-\right) /\Gamma \!\bigl (\psi \rightarrow D^0 \overline{D^0}\bigr )$, where the strong interaction causes the decay channels, and they measure isospin breakages. If we estimate their theoretical values in the plane-wave formalism without considering the effects originating from the composite nature of the initial-state vector mesons, they are disfavored with the PDG’s central values at the level of $2.1\,\sigma$ and $9.5\,\sigma$. In particular, there has been no explanation for the latter 9.5 $\sigma$ anomaly so far.

The decay channels that we focus on are near the mass thresholds, where the velocities in the final state are small, and hence the localization of the overlap of the wave packets is more significant. Here, we fully take into account such effects in the Gaussian wave-packet formalism. We carefully clarified the properties of one-to-two-body nonrelativistic quantum transitions between normalizable physical states described by Gaussian wave packets under the presence of the decaying nature of the initial state, which is a full-fledged calculation taking into account the essences that are missing in the plane-wave calculations.

The result shows agreement with the PDG’s combined results within a $\sim 1\,\sigma$ confidence level. We conclude that the long-standing anomalies in $R_\phi$ and $R_\psi$ are resolved.

In the calculation, the abovementioned compositeness has been described by the form factor. The agreement is achieved when we appropriately take the form factor parameter at around the physically reasonable value $R_0\sim \left( 500\,\text {MeV}\right) ^{-1}$.

We also analyzed and made a comment on the $b\overline{b}$-vector-meson counterpart $\Upsilon$, namely, $R_\Upsilon = \Gamma \!\left( \Upsilon \rightarrow B^+ B^-\right) /\Gamma \!\bigl (\Upsilon \rightarrow B^0 \overline{B^0}\bigr )$, where the plane-wave calculation without considering the abovementioned composite nature already agrees at the $0.32\,\sigma$ level with the corresponding PDG result due to the smallness of the mass difference between $B^\pm$ and $B^0$. The wave-packet result agrees well with the PDG result around the same value of $R_0$.

We mention that the same form factors can be formally multiplied on the ratio of the plane-wave decay rates in order to partially take into account the wave-packet effects, though the wave-packet approach is more comprehensive in describing quantum transitions. By doing so, around the same value of $R_0$, the plane-wave results can also be made to agree with the PDG ones.

In general, the shape of a wave-packet resonance deviates from the Briet–Wigner shape, where the magnitude of the deviation depends on the size of wave packets. For $\phi$ and $\psi$, experimental data is available, and we put constraints on the size. We found that when the size of the wave packets is small, the derivation from the Briet–Wigner shape tends to be sizable. Both for $\phi$ and $\psi$, wide ranges of the wave-packet size are consistent with the experimental data.

In the decay channels of the vector mesons, the nonrelativistic approximation works fine, which simplifies the integrations in the S-matrices and the final-state phase spaces in the wave-packet formalism. Many other quantum transitions in high-energy physics are relativistic, and it is worthwhile to establish the general method to perform such integrations without relying on the nonrelativistic approximation. Also, analyzing resonant productions precisely requires the full transition probabilities, including production parts. Doing more dedicated analyses on resonant shapes will be another important task.

Appendices

Appendix A: Vector-meson form factor

We focus on the following form for a nonrelativistic bound state $V\!\left( Q{\overline{Q}}\right)$ composed of a heavy constituent quark Q and its anti-particle $\overline{Q}$ at a certain time:

$$\begin{aligned} \left| V(Q\overline{Q}), {\varvec{P}} \right\rangle&:= {\sum _{s_1,s_2}} \int \text {d}^3{\varvec{x}}_1\, \text {d}^3{\varvec{x}}_2\, e^{i {\varvec{P}} \cdot \frac{{\varvec{x}}_1 + {\varvec{x}}_2}{2}} F_{s_1,s_2}({\varvec{x}}_1 - {\varvec{x}}_2)\nonumber \\&\quad \times Q^\dagger \!\left( {\varvec{x}}_1, s_1\right) \overline{Q}^\dagger \!\left( {\varvec{x}}_2, s_2\right) \left| 0 \right\rangle , \end{aligned}$$

(95)

where $F_{s_1,s_2}\!\left( {\varvec{x}}_1 - {\varvec{x}}_2\right)$ is a wave function for the bound state; $Q^\dagger \!\left( {\varvec{x}}_1, s_1\right)$ and $\overline{Q}^\dagger \!\left( {\varvec{x}}_2, s_2\right)$ are the Fourier transforms of the momentum-space creation operators of Q and ${\overline{Q}}$, respectively, with ${\varvec{x}}_{1,2}$ and $s_{1,2}$ being their positions and spins; and $\left| 0 \right\rangle$ is the vacuum; see e.g. Ref. [40].

We consider the following matrix element:

$$\begin{aligned}&\left\langle Q\left( {\varvec{p}}_1, s_1\right) \overline{Q}\left( {\varvec{p}}_2, s_2\right) | V(Q\overline{Q}), {\varvec{P}} \right\rangle \nonumber \\&\quad = \int \text {d}^3{\varvec{x}}_1\, \text {d}^3{\varvec{x}}_2\, e^{i {\varvec{P}} \cdot \frac{{\varvec{x}}_1 + {\varvec{x}}_2}{2}} F_{s_1,s_2}\!\left( {\varvec{x}}_1 - {\varvec{x}}_2\right) \left( \frac{1}{\sqrt{2\pi }}\right) ^6 \nonumber \\&\qquad \quad e^{- i {\varvec{p}}_1 \cdot {\varvec{x}}_1 -i {\varvec{x}}_2 \cdot {\varvec{p}}_2} \nonumber \\&\quad = \int \text {d}^3{\varvec{X}}\, \text {d}^3{\varvec{r}}\,e^{i \left( {\varvec{P}} - {\varvec{p}}_1 -{\varvec{p}}_2\right) \cdot {\varvec{X}} -i \left( \frac{{\varvec{p}}_1 - {\varvec{p}}_2}{2}\right) \cdot {\varvec{r}}} \frac{1}{\left( 2\pi \right) ^3} F_{s_1,s_2}\!\left( {\varvec{r}}\right) \nonumber \\&\quad = \delta ^{3}\!\left( {\varvec{P}} - {\varvec{p}}_1 - {\varvec{p}}_2\right) \underbrace{\int \text {d}^3{\varvec{r}}\, e^{ -i \left( \frac{{\varvec{p}}_1 - {\varvec{p}}_2}{2}\right) \cdot {\varvec{r}}} F_{s_1,s_2}\left( {\varvec{r}}\right) }_{=: \widetilde{F}_{s_1,s_2} \!\left( \frac{{\varvec{p}}_1 - {\varvec{p}}_2}{2}\right) },\nonumber \\ \end{aligned}$$

(96)

where ${\varvec{X}}:= ({\varvec{x}}_1 + {\varvec{x}}_2)/2$, ${\varvec{r}}:= {\varvec{x}}_1 - {\varvec{x}}_2$, and the normalization of the form factor $\widetilde{F}_{s_1,s_2} \!\left( \frac{{\varvec{p}}_1 - {\varvec{p}}_2}{2}\right)$ is irrelevant for our purpose.²⁸

Hereafter, we assume the separable form

$$\begin{aligned} \widetilde{F}_{s_1,s_2} \!\left( \frac{{\varvec{p}}_1 - {\varvec{p}}_2}{2}\right) = S_{s_1,s_2} \,\widetilde{F} \!\left( \frac{{\varvec{p}}_1 - {\varvec{p}}_2}{2}\right) \end{aligned}$$

(97)

for the heavy and nonrelativistic quarks $Q{\overline{Q}}$, which have negligible spin-orbital angular momentum interaction. Further, we drop the spin structure $S_{s_1,s_2}$, which will be canceled out in the ratio of the neutral to charged rates, and focus on the momentum part.²⁹

We adopt an approximate form of the wave function of the (s-wave) ground state under a Coulomb potential in the position space as

$$\begin{aligned} F\!\left( {\varvec{r}}\right) = \frac{N}{\sqrt{2\pi R_0}} \frac{e^{- \frac{r}{R_0}}}{r}, \end{aligned}$$

(98)

where $r:=\left| {\varvec{r}}\right|$, the parameter $R_0$ describes a typical length scale of the bound state discussed below, and N is the irrelevant normalization factor mentioned above. Its Fourier transform is

$$\begin{aligned} \widetilde{F}\!\left( {\varvec{p}}\right)&= N\int {\text {d}^3{\varvec{r}}\over \left( 2\pi \right) ^{3/2}}\,e^{-i {\varvec{p}} \cdot {\varvec{r}}} F\!\left( {\varvec{r}}\right) = {N\over \pi \sqrt{R_0}}{1\over {\varvec{p}}^2+{1\over R_0^2}}, \end{aligned}$$

(99)

where we used $\int _0^\infty \text {d}r \sin \!\left( pr\right) e^{- \frac{r}{R_0}} = \frac{p}{p^2 + \frac{1}{R_0^2}}$. In this paper, we choose $N= \pi /R_0^{3/2}$ such that ${\widetilde{F}}\!\left( {\varvec{0}}\right) =1$,

$$\begin{aligned} {\widetilde{F}}\!\left( {\varvec{p}}\right)&= {1\over R_0^2}{1\over {\varvec{p}}^2+{1\over R_0^2}}. \end{aligned}$$

(100)

This form is also introduced in Ref. [3] to cut off a UV divergence in the plane-wave computation. The treatment in Refs. [4, 5] is equivalent to taking this form factor to be unity.

Here, a vector meson $V\!\left( Q{\overline{Q}}\right)$ decays into two light pseudo-scalar mesons $P\!\left( Q{\overline{q}}\right)$ and ${\overline{P}}\!\left( q{\overline{Q}}\right)$. We approximate the mass and momentum for each pseudo-scalar P (${\overline{P}}$) by those of the constituent quark Q (${\overline{Q}}$): $m_P\simeq m_Q$ and ${\varvec{p}}_P\simeq {\varvec{p}}_1$ (${\varvec{p}}_{{\overline{P}}}\simeq {\varvec{p}}_2$), respectively. In this paper, we focus on the situation where the masses of the two pseudo-scalar mesons are almost the same (due to the approximated flavor isospin SU(2) symmetry), and the mass relation is near the decay threshold,

$$\begin{aligned} m_V \approx 2 \, m_P. \end{aligned}$$

(101)

Therefore, we can treat the process as a nonrelativistic one, and thus, we conclude that

$$\begin{aligned} {\varvec{p}}_1 - {\varvec{p}}_2 \approx m_P \left( {\varvec{V}}_1 - {\varvec{V}}_2\right) , \end{aligned}$$

(102)

thereby,

$$\begin{aligned} \widetilde{F} \!\left( \frac{{\varvec{p}}_1 - {\varvec{p}}_2}{2}\right)&= \frac{1}{\left( \frac{R_0 \left( {\varvec{p}}_1 - {\varvec{p}}_2\right) }{2}\right) ^2 + 1 } \end{aligned}$$

(103)

$$\begin{aligned}&\Rightarrow \frac{1}{\left( \frac{R_0 m_P \left( {\varvec{V}}_1 - {\varvec{V}}_2\right) }{2}\right) ^2 + 1 }, \end{aligned}$$

(104)

where ${\varvec{V}}_1$ and ${\varvec{V}}_2$ are the (nonrelativistic) velocities of P and ${\overline{P}}$, respectively.

Finally, we reach the spin-independent dimensionless function suitable for our purpose:

$$\begin{aligned} \widetilde{F}\!\left( \left| {\varvec{V}}_1 - {\varvec{V}}_2\right| \right) := \frac{1}{\left( \frac{R_0 m_P \left( {\varvec{V}}_1 - {\varvec{V}}_2\right) }{2}\right) ^2 + 1 }. \end{aligned}$$

(105)

This is the form factor shown in Eq. (15) for the matrix elements of the meson decays (with $m_V \approx 2 \, m_P$) in the rest system.

Now we estimate a typical value of the parameter $R_0$ in Eq. (98). The quarkonium potential can be approximated by a sum of the confining linear potential and the QCD Coulomb potential:

$$\begin{aligned} V(r) = \frac{r}{a_s^2} - \frac{\alpha _s}{r}, \end{aligned}$$

(106)

where $a_s$ is known as $a_s = 1.95\,\text {GeV}^{-1}$ [40], and $\alpha _s$ is the QCD fine structure constant. For the domain where $r\lesssim r_c:= a_s \sqrt{\alpha _s}\simeq 1.5\,\text {GeV}^{-1}$,³⁰ the wave function can be approximated by the Coulomb form (98). One can estimate r by equating the potential and kinetic energies, $V\!\left( r\right) \sim K_Q$, where $K_Q\sim m_V-2m_Q=\mathcal{O}(10)\,\text {MeV}$. Since $K_Q$ is much smaller than the typical energy scale $a_s^{-1}=0.5$ GeV of the potential, the typical $Q{\overline{Q}}$ distance can be estimated by equating two terms in the right-hand side of Eq. (106): $r\sim r_c$. The use of Coulomb wave function (98) is marginally justified, which suffices for our current consideration. See e.g. Ref. [42] for further refinement.

Finally, a typical value of the parameter in Eq. (98) is

$$\begin{aligned} R_0\sim r_c\simeq 1.5\,\text {GeV}^{-1}=0.0015\,\text {MeV}^{-1}={1\over 660\,\text {MeV}}. \end{aligned}$$

(107)

Appendix B: Details on calculations of $P_{V \rightarrow P \overline{P}}$

We present detailed computation to obtain the decay probability integrated over the final-state positions and momenta under the rest-frame assumption ${\varvec{P}}_0=0$.

1.1 B.1 Variables under nonrelativistic limit

As preparation, we show concrete forms of the kinetic variables and parameters, whose physical meaning is given in Sect. 2.1, under the nonrelativistic limit $\left| {\varvec{V}}_{1,2}\right| \ll 1$. At first, we define the “light-cone” variables for later convenience:

$$\begin{aligned} {\varvec{P}}_\pm&:= {\varvec{P}}_1 \pm {\varvec{P}}_2, \quad {\varvec{V}}_\pm := {\varvec{V}}_1 \pm {\varvec{V}}_2. \end{aligned}$$

(108)

The kinetic variables are

$$\begin{aligned}&{\varvec{P}}_0 = 0 \Rightarrow {\varvec{V}}_0 = 0, \quad {\varvec{P}}_1 \Rightarrow m_P {\varvec{V}}_1, \quad {\varvec{P}}_2 \Rightarrow m_P {\varvec{V}}_2, \nonumber \\&E_0 {=} m_V \Rightarrow m_V,\quad E_1 {\Rightarrow } m_P {+} \frac{m_P}{2} {\varvec{V}}_1^2, \quad E_2 {\Rightarrow } m_P {+} \frac{m_P}{2} {\varvec{V}}_2^2, \nonumber \end{aligned}$$

$$\begin{aligned} \overline{|g_\text {eff}|^2} = {\frac{g^2}{3}} ({\varvec{P}}_1 - {\varvec{P}}_2)^2&\Rightarrow {\frac{g^2}{3}} \left( m_P^2 {\varvec{V}}_-^2\right) , \end{aligned}$$

(109)

where the symbol $\Rightarrow$ represents the nonrelativistic approximation, which we will take later. We also have

$$\begin{aligned} \overline{{\varvec{V}}}&= \sigma _s \left( \frac{{\varvec{V}}_1}{\sigma _P} + \frac{{\varvec{V}}_2}{\sigma _P} \right) = \frac{\sigma _s}{\sigma _P} {\varvec{V}}_+, \end{aligned}$$

(110)

$$\begin{aligned} \overline{{\varvec{V}}^2}&= \sigma _s \left( \frac{{\varvec{V}}_1^2}{\sigma _P} + \frac{{\varvec{V}}_2^2}{\sigma _P} \right) = \frac{\sigma _s}{2\sigma _P} \left( {\varvec{V}}_+^2 + {\varvec{V}}_-^2 \right) , \end{aligned}$$

(111)

$$\begin{aligned} \delta {\varvec{P}}&= {\varvec{P}}_1 + {\varvec{P}}_2 \Rightarrow m_P {\varvec{V}}_+ , \end{aligned}$$

(112)

$$\begin{aligned} \delta E&= - m_V + E_1 + E_2 \Rightarrow - \left( m_V - 2m_P\right) \nonumber \\ {}&\quad + \frac{1}{2} m_P \left( {\varvec{V}}_1^2 + {\varvec{V}}_2^2 \right) \nonumber \\&\ = - \left( m_V - 2m_P\right) + \frac{1}{4} m_P \left( {\varvec{V}}_+^2 + {\varvec{V}}_-^2 \right) , \end{aligned}$$

(113)

$$\begin{aligned} \delta \omega&= \delta E - \overline{{\varvec{V}}} \cdot \delta {\varvec{P}} \Rightarrow \delta E - m_P \frac{\sigma _s}{\sigma _P} {\varvec{V}}_+^2, \end{aligned}$$

(114)

$$\begin{aligned} \sigma _s&= \frac{\sigma _V \sigma _P}{2\sigma _V + \sigma _P}, \end{aligned}$$

(115)

$$\begin{aligned} \sigma _t&= \frac{\sigma _s}{\overline{{\varvec{V}}^2} - \left( \overline{{\varvec{V}}}\right) ^2} = \frac{\sigma _P}{ \frac{1}{2} \left( {\varvec{V}}_+^2 + {\varvec{V}}_-^2\right) - \frac{\sigma _s}{\sigma _P} {\varvec{V}}_+^2 }. \end{aligned}$$

(116)

Also, we define the variables $V_+$ and $V_-$

$$\begin{aligned} V_+&:= \left| {\varvec{V}}_+\right| , \quad V_- := \left| {\varvec{V}}_-\right| . \end{aligned}$$

(117)

1.2 B.2 Bulk contribution

We compute the bulk contribution in Eq. (37). First, we perform the position integrals $\int _{-\infty }^{+\infty } \text {d}^3 {\varvec{X}}_1 \text {d}^3 {\varvec{X}}_2$. As in Ref. [11], we obtain

$$\begin{aligned} \text {d}^3 {\varvec{X}}_1 \text {d}^3 {\varvec{X}}_2&= \text {d}^5 y\, \text {d}y_0, \end{aligned}$$

(118)

$$\begin{aligned} \int \text {d}^5 y\, e^{ - {\mathcal {R}}}&= \sqrt{ \frac{\pi ^5}{\sigma _t} \left( \frac{\sigma _0\sigma _1\sigma _2}{\sigma _s} \right) ^3 } \frac{1}{ \sqrt{ \left( \delta {\varvec{V}}_1\right) ^2 + \left( \delta {\varvec{V}}_2\right) ^2 } }, \end{aligned}$$

(119)

$$\begin{aligned} {{\mathfrak {T}} - {\Gamma _V\sigma _t\over 2}}&= - \frac{y_0}{ \sqrt{\left( \delta {\varvec{V}}_1\right) ^2 + \left( \delta {\varvec{V}}_2\right) ^2} } + \cdots , \end{aligned}$$

(120)

$$\begin{aligned} \text {d}{\mathfrak {T}}&= - \frac{\text {d}y_0}{ \sqrt{\left( \delta {\varvec{V}}_1\right) ^2 + \left( \delta {\varvec{V}}_2\right) ^2} }, \end{aligned}$$

(121)

where $y_0$ becomes a flat direction under the (unphysical) no-decay limit $\Gamma _V \rightarrow 0$ (as considered in [11]), while the other five directions are not flat directions irrespective of $\Gamma _V$:

$$\begin{aligned}&\int \text {d}^3 {\varvec{X}}_1 \text {d}^3 {\varvec{X}}_2 e^{ - {\mathcal {R}} -\Gamma _V\left( {\mathfrak {T}}-T_0\right) + \frac{\Gamma _V^2\sigma _t}{4} } \bigl (W({\mathfrak {T}})\bigr )^2 \nonumber \\&\quad = \sqrt{ \frac{\pi ^5}{\sigma _t} \left( \frac{\sigma _0\sigma _1\sigma _2}{\sigma _s} \right) ^3 } \, \int _{ {T_{\textrm{in}}+ {\Gamma _V\sigma _t\over 2}} }^{ {T_{\textrm{out}}+ {\Gamma _V\sigma _t\over 2} } } \text {d}{\mathfrak {T}}\, e^{ -\Gamma _V\left( {\mathfrak {T}}-T_0\right) + \frac{\Gamma _V^2\sigma _t}{4} } \nonumber \\&\quad = \sqrt{ \frac{\pi ^5}{\sigma _t} \left( \frac{\sigma _0\sigma _1\sigma _2}{\sigma _s} \right) ^3 } \, \Gamma _V^{-1} e^{ -\Gamma _V\left( T_{\textrm{in}}-{T_0}\right) {- \frac{\Gamma _V^2\sigma _t}{4}} }, \end{aligned}$$

(122)

where we also used Eq. (31) and took the limit $T_{\textrm{out}}\rightarrow \infty$. The range of the integration is given by the bulk window function $W({\mathfrak {T}})$ in Eq. (31). After the position integrations, we obtain

$$\begin{aligned} \text {d}P^\text {bulk}_{V \rightarrow P \overline{P}}&= \left| g_\text {eff}\right| ^2 N_V^2 \Gamma _V^{-1} e^{ -\Gamma _V\left( T_{\textrm{in}}-{T_0}\right) {- \frac{\Gamma _V^2\sigma _t}{4}} } \frac{1}{2 E_0}\nonumber \\&\quad \times \frac{\text {d}^3 {\varvec{P}}_1}{(2\pi )^3 2E_1} \frac{\text {d}^3 {\varvec{P}}_2}{(2\pi )^3 2E_2} \nonumber \\&\quad \times \left( 2 \pi \right) ^4 \left( \sqrt{\frac{\sigma _t}{\pi }} \left( \frac{\sigma _s}{\pi }\right) ^{3/2} e^{-\sigma _t\left( \delta \omega \right) ^2 -\sigma _s\left( \delta {\varvec{P}}\right) ^2 } \right) \nonumber \\ {}&\quad \times \left| \widetilde{F}\!\left( V_-\right) \right| ^2, \end{aligned}$$

(123)

where the damping factors $\sqrt{\sigma _t\over \pi } \, e^{-\sigma _t\left( \delta \omega \right) ^2}$ and $\left( \sigma _s\over \pi \right) ^{3/2} e^{-\sigma _s\left( \delta {\varvec{P}}\right) ^2 }$ provide the approximate conservation for the mean energy and momentum, respectively.³¹ So far, the expression (123) does not assume ${\varvec{P}}_0=0$ nor the nonrelativistic approximation given in Sect. 1.

Next, we perform the momentum integrals under the saddle-point approximation with ${\varvec{P}}_0=0$ in the nonrelativistic approximation given in Sect. 1:

$$\begin{aligned} P^\text {bulk}_{V \rightarrow P \overline{P}}&\Rightarrow \left( N_V^2 \Gamma _V^{-1} e^{ -\Gamma _V\left( T_{\textrm{in}}-{T_0}\right) } \right) \frac{g^2}{3} \frac{1}{2 m_V} \frac{1}{(2\pi )^2 4 E_1 E_2} \nonumber \\&\quad \times \frac{m_P^6}{8} \left( \int _{0}^{\infty } 4 \pi V_+^2 \text {d}V_+ \right) \left( \int _0^\infty 4\pi V_-^2 \text {d}V_- \right) \nonumber \\&\quad \times \frac{1}{\pi ^2} \sigma _t^{1/2} \sigma _s^{3/2} \left( m_P^2 V_-^2\right) e^{ - F_\text {bulk}(V_+, V_-) } \left| \widetilde{F}\!\left( V_-\right) \right| ^2, \end{aligned}$$

(124)

where the factor $1/8 = 1/2^3$ is from the Jacobian, and the exponent is

$$\begin{aligned}&F_\text {bulk}(V_+, V_-) \nonumber \\ {}&\quad := \sigma _s\left( \delta {\varvec{P}}\right) ^2 + \sigma _t\left( \delta \omega \right) ^2 { + \frac{\Gamma _V^2\sigma _t}{4}}\nonumber \\&\quad = \sigma _s m_P^2 V_+^2 \nonumber \\&\qquad + \frac{2 \sigma _P}{ \left( 1 - \frac{2\sigma _s}{\sigma _P} \right) V_+^2 + V_-^2 } \Bigg \{ \left[ m_P \left( \frac{1}{4} - \frac{\sigma _s}{\sigma _P} \right) V_+^2 \right. \nonumber \\&\qquad \left. + \frac{1}{4} m_P V_-^2 - \left( m_V - 2m_P\right) \right] ^2 + { {\Gamma _V^2\over 4} } \Bigg \}. \end{aligned}$$

(125)

We see that

$$\begin{aligned} 1 - \frac{2\sigma _s}{\sigma _P} = \frac{1}{ 2 \sigma _V/\sigma _P + 1} > 0, \end{aligned}$$

(126)

which implies

$$\begin{aligned} \sigma _P - {2\sigma _s} > 0. \end{aligned}$$

(127)

Also, we see that

$$\begin{aligned} \sigma _P - 4\sigma _s = \frac{ \sigma _P \left( \sigma _P - 2\sigma _s \right) }{ \sigma _P + 2\sigma _s } > 0, \end{aligned}$$

(128)

which leads to

$$\begin{aligned} \frac{1}{4} - \frac{\sigma _s}{\sigma _P} = \frac{1}{4\sigma _P} \left( \sigma _P - 4 \sigma _s \right) > 0. \end{aligned}$$

(129)

Thereby,

$$\begin{aligned} e^{ - F_\text {bulk} }&\underbrace{\rightarrow }_{V_+ \rightarrow \infty } 0,&e^{ - F_\text {bulk} }&\underbrace{\rightarrow }_{V_- \rightarrow \infty } 0. \end{aligned}$$

(130)

The stationary point $(V_+^s, V_-^s)$ that satisfies

$$\begin{aligned} \frac{\partial F_\text {bulk} (V_+^s, V_-^s)}{\partial V_+} = 0 \quad \text {and} \quad \frac{\partial F_\text {bulk} (V_+^s, V_-^s)}{\partial V_-} = 0 \end{aligned}$$

(131)

is found to be

$$\begin{aligned} \left( V_+^s, V_-^s\right)&\in \left\{ \left( 0, \, \frac{ 2\left( \left( m_V-2m_P\right) ^2+{\Gamma _V^2\over 4}\right) ^{1/4} }{\sqrt{m_P}} \right) \right. , \nonumber \\ {}&\quad \left( 0, \, \frac{ -2\left( \left( m_V-2m_P\right) ^2+{\Gamma _V^2\over 4}\right) ^{1/4} }{\sqrt{m_P}} \right) , \nonumber \\&\quad \left( 0, \, \frac{ 2i\left( \left( m_V-2m_P\right) ^2+{\Gamma _V^2\over 4}\right) ^{1/4} }{\sqrt{m_P}} \right) ,\nonumber \\ {}&\quad \left( 0, \, \frac{ -2i\left( \left( m_V-2m_P\right) ^2+{\Gamma _V^2\over 4}\right) ^{1/4} }{\sqrt{m_P}} \right) , \nonumber \\&\quad \left( \frac{ 2\left( \left( m_V-2m_P\right) ^2+{\Gamma _V^2\over 4}\right) ^{1/4} }{\sqrt{m_P}} ,\, 0\right) , \nonumber \\ {}&\quad \left( \frac{ -2\left( \left( m_V-2m_P\right) ^2+{\Gamma _V^2\over 4}\right) ^{1/4} }{\sqrt{m_P}} ,\, 0\right) , \nonumber \\&\quad \left( \frac{ 2i\left( \left( m_V-2m_P\right) ^2+{\Gamma _V^2\over 4}\right) ^{1/4} }{\sqrt{m_P}} ,\, 0\right) , \nonumber \\ {}&\quad \left. \left( \frac{ -2i\left( \left( m_V-2m_P\right) ^2+{\Gamma _V^2\over 4}\right) ^{1/4} }{\sqrt{m_P}} ,\, 0\right) \right\} . \end{aligned}$$

(132)

Now we focus on the kinetic region ($V_+ \simeq 0$ and $V_- > 0$), and thus only the first one is relevant in our calculation:

$$\begin{aligned} \left( V_+^\text {B}, V_-^\text {B}\right)&:= \left( 0, \, \frac{ 2\left( \left( m_V-2m_P\right) ^2+{\Gamma _V^2\over 4}\right) ^{1/4} }{\sqrt{m_P}}\right. \nonumber \\&\left. = 2 \left[ \frac{\left( m_V-2m_P\right) ^2}{m_P^2} + {\Gamma _V^2\over 4m_P^2} \right] ^{1/4} \right) . \end{aligned}$$

(133)

Note that

$$\begin{aligned} {F_\text {bulk}^0}&:= F_\text {bulk}\left( V_+^\text {B}, V_-^\text {B}\right) = m_P \sigma _P\nonumber \\&\left( -\left( m_V - 2m_P\right) + \sqrt{ \left( m_V - 2m_P\right) ^2+{\Gamma _V^2\over 4} } \right) , \end{aligned}$$

(134)

which goes to zero in the (unphysical) limit $\Gamma _V\rightarrow 0$. Also, we can see

$$\begin{aligned} \left. \frac{\partial ^2 F_\text {bulk} }{\partial V_+^2}\right| _{\left( V_+^\text {B}, V_-^\text {B}\right) }&= 2 m_P^2 \sigma _s \nonumber \\ {}&\quad \times \left[ \frac{1}{2}+ \frac{m_V - 2m_P}{2\sqrt{\left( m_V-2m_P\right) ^2+{\Gamma _V^2\over 4}}} \right] \nonumber \\&=: 2 m_P^2 \sigma _s {A_\text {bulk}}\quad (>0) , \nonumber \\ \left. \frac{\partial ^2 F_\text {bulk} }{\partial V_-^2}\right| _{\left( V_+^\text {B}, V_-^\text {B}\right) }&= m_P^2 \sigma _P \quad (>0), \nonumber \\ \left. \frac{\partial ^2 F_\text {bulk} }{\partial V_+ \partial V_-}\right| _{\left( V_+^\text {B}, V_-^\text {B}\right) }&= 0, \end{aligned}$$

(135)

where $A_\text {bulk} \rightarrow 1$ under the (unphysical) limit $\Gamma _V \rightarrow 0$.

We perform the integrals under the saddle-point approximation (putting $E_1=E_2=m_P$ in the overall factor) as

$$\begin{aligned}&P^\text {bulk}_{V \rightarrow P\overline{P}} \simeq \frac{g_V^2}{3} \frac{1}{2 m_V} \frac{1}{(2\pi )^2 4m_P^2} \, \nonumber \\&\qquad \times \frac{m_P^6}{8} \left( \int _{0}^{\infty } 4 \pi V_+^2 \text {d}V_+ \right) \left( \int _0^\infty 4\pi \left( V_-^\text {B}\right) ^2 \text {d}V_- \right) \nonumber \\&\qquad \times \frac{1}{\pi ^2} \left[ \sigma _t^{1/2}\right] _{V_+ \rightarrow V_+^\text {B}, \, V_- \rightarrow {V_-^\text {B}}}\, \sigma _s^{3/2} \left[ m_P^2 \left( V_-^\text {B}\right) ^2\right] \nonumber \\&\qquad \times {e^{-F^0_\text {bulk}}} e^{ - \frac{1}{2} \left( 2 m_P^2 \sigma _s\right) {A_\text {bulk}} \left( V_+ - V_+^\text {B} \right) ^2 } e^{ - \frac{1}{2} \left( m_P^2 \sigma _P\right) \left( V_- - V_-^\text {B} \right) ^2 } \nonumber \\&\qquad \times \left( {N_V^2\over \Gamma _V} e^{ -\Gamma _V\left( T_{\textrm{in}}-{T_0}\right) } \right) \left| \widetilde{F}\!\left( V_-^\text {B}\right) \right| ^2 \nonumber \\&\quad = \frac{g^2 m_P^3 N_V^2 e^{ -\Gamma _V\left( T_{\textrm{in}}-{T_0}\right) } }{12 \pi m_V m_P^2}\, {m_P\over \Gamma _V} \left[ \frac{\left( m_V-2m_P\right) ^2}{m_P^2} + {\Gamma _V^2\over 4m_P^2} \right] ^{3/4} \nonumber \\&\qquad \times \frac{1}{2} \left[ 1 + \text {erf}\left( \frac{ m_P\sqrt{\sigma _P} {V_-^\text {B}} }{\sqrt{2}} \right) \right] \ {\frac{e^{-F^0_\text {bulk}}}{A_\text {bulk}^{3/2}}} \left| \widetilde{F}\!\left( V_-^\text {B}\right) \right| ^2, \nonumber \\ \end{aligned}$$

(136)

where we have used the formulas for $a > 0$,

$$\begin{aligned} \int _0^\infty 4 \pi \, r^2 e^{ - \frac{a}{2} r^2 } \text {d}r&= \frac{ 2\sqrt{2} \, \pi ^{3/2} }{ a^{3/2} }, \end{aligned}$$

(137)

$$\begin{aligned} \int _0^\infty e^{ - \frac{a}{2} \left( r - r_0 \right) ^2 } \text {d}r&= \sqrt{\frac{\pi }{2 a}} \left[ 1 + \text {erf}\left( \frac{\sqrt{a} r_0}{\sqrt{2}} \right) \right] , \end{aligned}$$

(138)

and the Taylor expansion around one of the stationary points $(V_+, V_-) = (V_+^s, V_-^s)$

$$\begin{aligned} F(V_+, V_-)&\simeq F(V_+^s, V_-^s) + \frac{1}{2} \nonumber \\&\quad \times \sum _{i,j = +, -} \frac{\partial ^2 F(V_+^s, V_-^s)}{\partial V_i\,\partial V_j} \left( V_i - V_i^s\right) \left( V_j - V_j^s\right) .\nonumber \\ \end{aligned}$$

(139)

1.3 B.3 Boundary contribution

We compute the boundary contribution in Eq. (37) as

$$\begin{aligned}&\int \text {d}^3{\varvec{X}}_1 \text {d}^3{\varvec{X}}_2 e^{ -\mathcal{R} - \Gamma _V\left( {\mathfrak {T}}-T_{\textrm{in}}\right) + \frac{\Gamma _V^2\sigma _t}{4} -\frac{ \left( {\mathfrak {T}} - T_{\textrm{in}}- {\Gamma _V\sigma _t\over 2}\right) ^2 }{\sigma _t} }\nonumber \\&\qquad \times \frac{2\sigma _t}{\pi } \frac{1}{ \left( {\mathfrak {T}} -T_{\textrm{in}}- {\Gamma _V\sigma _t\over 2} \right) ^2 + \left( \sigma _t \delta \omega \right) ^2 } \nonumber \\&\quad = \sqrt{ \frac{\pi ^5}{\sigma _t} \left( \frac{\sigma _0\sigma _1\sigma _2}{\sigma _s} \right) ^3 } e^{- \Gamma _V\left( T_{\textrm{in}}-T_0\right) } \frac{2\sigma _t}{\pi } \int _{-\infty }^{\infty } \text {d}{\mathfrak {T}}' e^{ - \frac{1}{\sigma _t} \left( {\mathfrak {T}}'\right) ^2} \nonumber \\&\qquad \times \frac{1}{ \left( {\mathfrak {T}}' - {\Gamma _V\sigma _t\over 2} \right) ^2 + \left( \sigma _t \delta \omega \right) ^2 }, \end{aligned}$$

(140)

where ${\mathfrak {T}}':={\mathfrak {T}}-T_{\textrm{in}}$, and the range of the integration is $(-\infty ,+\infty )$ since there exists no window function $W({\mathfrak {T}})$ for this boundary term other than the exponential factor.

Now we perform the Taylor expansion of ${\mathfrak {T}}'$ around the saddle-point ${\mathfrak {T}}'=0$ up to the second order:

$$\begin{aligned}&\frac{ e^{ - \frac{1}{\sigma _t} \left( {\mathfrak {T}}'\right) ^2} }{ \left( {\mathfrak {T}}' - {\Gamma _V\sigma _t\over 2} \right) ^2 + \left( \sigma _t \delta \omega \right) ^2 } \nonumber \\&\quad \simeq \left( \frac{1}{ \left( {\Gamma _V\sigma _t\over 2}\right) ^2 + \left( \sigma _t \delta \omega \right) ^2 } + \frac{ 2 \left( {\Gamma _V\sigma _t\over 2}\right) {\mathfrak {T}}' }{ \left( \left( {\Gamma _V\sigma _t\over 2}\right) ^2 + \left( \sigma _t \delta \omega \right) ^2\right) ^2 }\right. \nonumber \\&\qquad + \left. \frac{ \left( 3\left( {\Gamma _V\sigma _t\over 2}\right) ^2 - \left( \sigma _t \delta \omega \right) ^2 \right) \left( {\mathfrak {T}}'\right) ^2 }{ \left( \left( {\Gamma _V\sigma _t\over 2}\right) ^2 + \left( \sigma _t \delta \omega \right) ^2\right) ^3 } \right) e^{ - \frac{1}{\sigma _t} \left( {\mathfrak {T}}'\right) ^2}, \end{aligned}$$

(141)

to obtain

$$\begin{aligned}&\int _{-\infty }^{\infty } \text {d}{\mathfrak {T}}' \frac{ e^{ - \frac{1}{\sigma _t} \left( {\mathfrak {T}}'\right) ^2} }{ \left( {\mathfrak {T}}' - {\Gamma _V\sigma _t\over 2} \right) ^2 + \left( \sigma _t \delta \omega \right) ^2 } \nonumber \\&\quad \simeq \frac{\sqrt{ \pi \sigma _t }}{ \sigma _t^2 } \left( \frac{1}{ \left( {\Gamma _V\over 2}\right) ^2 + \left( \delta \omega \right) ^2 } + \frac{ \left( 3\left( {\Gamma _V\over 2}\right) ^2 - \left( \delta \omega \right) ^2 \right) }{ \left( \left( {\Gamma _V\over 2}\right) ^2 + \left( \delta \omega \right) ^2\right) ^3 2\sigma _t } \right) , \end{aligned}$$

(142)

which yields

$$\begin{aligned}&P^\text {bdry}_{V \rightarrow P\overline{P}} \simeq \left( N_V^2 e^{ -\Gamma _V\left( T_{\textrm{in}}-{T_0}\right) } \right) \frac{g_V^2}{3} \frac{1}{2 m_V} \frac{1}{(2\pi )^2 4 E_1 E_2} \nonumber \\&\quad \times \frac{m_P^6}{8} \left( \int _{0}^{\infty } 4 \pi V_+^2 \text {d}V_+ \right) \left( \int _0^\infty 4\pi \text {d}V_- \right) \nonumber \\&\quad \times \frac{1}{2\pi ^{5/2}} \sigma _s^{3/2}\,{m_P^2}\,e^{- \sigma _s m_P^2 V_+^2} \nonumber \\&\quad \times \left\{ \frac{V_-^4}{ \left( {\Gamma _V\over 2}\right) ^2 + \left( \delta \omega \right) ^2 } + \frac{ V_-^4 \left[ 3\left( {\Gamma _V\over 2}\right) ^2 - \left( \delta \omega \right) ^2 \right] }{ \left[ \left( {\Gamma _V\over 2}\right) ^2 + \left( \delta \omega \right) ^2\right] ^3 2\sigma _t } \right\} \nonumber \\ {}&\quad \times \left| \widetilde{F}\!\left( V_-\right) \right| ^2. \end{aligned}$$

(143)

There is no $V_-$ in the exponent, and we will perform the numerical computation for the $V_-$ integral. On the other hand, the saddle-point of $V_+$ is located at $V_+ = 0$. With it in mind, we approximate the polynomial part of the integrand by setting $V_+ = 0$ other than $V_+^2$:

$$\begin{aligned} P^\text {bdry}_{V \rightarrow P\overline{P}}&\simeq \left( N_V^2 e^{ -\Gamma _V\left( T_{\textrm{in}}-{T_0}\right) } \right) \frac{g_V^2}{3} \frac{1}{2 m_V} \frac{1}{(2\pi )^2 4 m_P^2} \nonumber \\&\quad \times \frac{m_P^6}{8} \int _{0}^{\infty } 4 \pi V_+^2 \text {d}V_+ \int _0^\infty 4\pi \text {d}V_- \nonumber \\&\quad \times \frac{1}{2\pi ^{5/2}} \sigma _s^{3/2} m_P^2 e^{- \sigma _s m_P^2 V_+^2} \left( \frac{m_P}{4}\right) ^{-2} \widetilde{f}_\text {bdry}\!\left( V_-\right) , \end{aligned}$$

(144)

where we define a dimensionless function

$$\begin{aligned}&\widetilde{f}_\text {bdry}\!\left( V_-\right) := \left( \frac{m_P}{4}\right) ^{2} \nonumber \\&\quad \times \left\{ \frac{V_-^4}{ \left( {\Gamma _V\over 2}\right) ^2 + \left( \delta \omega \right) ^2 } + \frac{ V_-^4 \left[ 3\left( {\Gamma _V\over 2}\right) ^2 - \left( \delta \omega \right) ^2 \right] }{ \left[ \left( {\Gamma _V\over 2}\right) ^2 + \left( \delta \omega \right) ^2\right] ^3 2\sigma _t } \right\} _{V_+ \rightarrow 0} \nonumber \\&\quad \times \left| \widetilde{F}\!\left( V_-\right) \right| ^2 \nonumber \\&\quad = \left\{ \frac{V_-^4}{\left( V_-^2 - 4\frac{m_V-2m_P}{m_P}\right) ^2 + \frac{4^2}{m_P^2} \left( {\Gamma _V^2\over 4} \right) }\right. \nonumber \\&\qquad - \left. \frac{ V_-^4 \left( \left[ V_-^2 - 4\frac{m_V-2m_P}{m_P}\right] ^2 -3 \frac{4\Gamma _V^2}{m_P^2}\right) }{ 2\left( \sigma _t|_{V_+\rightarrow 0}\right) \left( \frac{m_P}{4} \right) ^2 \left[ \left( V_-^2 - 4\frac{m_V-2m_P}{m_P}\right) ^2 + \frac{4\Gamma _V^2}{m_P^2} \right] ^3 } \right\} \nonumber \\&\quad \times \left| \widetilde{F}\!\left( V_-\right) \right| ^2, \end{aligned}$$

(145)

with

$$\begin{aligned} \sigma _t|_{V_+\rightarrow 0} := \frac{2 \sigma _P}{V_-^2}. \end{aligned}$$

(146)

Now we execute the $V_+$ integral using the formula (137)

$$\begin{aligned} P^\text {bdry}_{V \rightarrow P\overline{P}}&= {\frac{g^2 m_P^3 N_V^2 e^{ -\Gamma _V\left( T_{\textrm{in}}-{T_0}\right) } }{12 \pi m_V m_P^2}} \frac{I_\text {bdry}}{2\pi }, \end{aligned}$$

(147)

where the integral

$$\begin{aligned} I_\text {bdry}&:= \int _0^\infty \text {d}V_- \widetilde{f}_\text {bdry}\!\left( V_-\right) \end{aligned}$$

(148)

is convergent.

1.4 B.4 Interference contribution

We compute the interference contribution in Eq. (37). We focus on the part including the factor

$$\begin{aligned} \frac{ e^{ +i \delta \omega \left( {\mathfrak {T}} -T_{\textrm{in}}- {\Gamma _V\sigma _t\over 2} \right) } }{ {\mathfrak {T}} -T_{\textrm{in}}- {\Gamma _V\sigma _t\over 2} - i \sigma _t \delta \omega }, \end{aligned}$$

since the other part can be obtained by taking complex conjugation. At first, we perform the square completion of the ${\mathfrak {T}}$ part:

$$\begin{aligned} I_\text {intf}&:= \ \int \text {d}^3{\varvec{X}}_1 \text {d}^3{\varvec{X}}_2 \nonumber \\&\qquad \times \textrm{exp}\Bigg [{}{ -\mathcal{R} {-} \Gamma _V\left( {\mathfrak {T}} {-} {T_0} \right) + \frac{\Gamma _V^2\sigma _t}{4} {-}\frac{ \left( {\mathfrak {T}} {-} T_{\textrm{in}}{-} {\Gamma _V\sigma _t\over 2}\right) ^2 }{{2}\sigma _t} }\nonumber \\ {}&\quad \qquad {+i \delta \omega \left( {\mathfrak {T}} -T_{\textrm{in}}- {\Gamma _V\sigma _t\over 2} \right) }\Bigg ]\nonumber \\&\qquad \times \frac{1}{ {\mathfrak {T}} -T_{\textrm{in}}- {\Gamma _V\sigma _t\over 2} -i \sigma _t \delta \omega } W\!\left( {\mathfrak {T}}\right) \nonumber \\&\quad = \sqrt{ \frac{\pi ^5}{\sigma _t} \left( \frac{\sigma _0\sigma _1\sigma _2}{\sigma _s} \right) ^3 } \int _{T_{\textrm{in}}+{\Gamma _V\sigma _t\over 2}}^{T_{\textrm{out}}+{\Gamma _V\sigma _t\over 2}} \text {d}{\mathfrak {T}} \nonumber \\&\qquad \times \textrm{exp}\Bigg [{}{ - \frac{1}{2\sigma _t} \left( {\mathfrak {T}} - \left( T_{\textrm{in}}{-} {\Gamma _V\sigma _t\over 2} + i\sigma _t\delta \omega \right) \right) ^2 } \nonumber \\ {}&\quad \qquad {{-} \Gamma _V\left( T_{\textrm{in}}{-}T_0\right) {-} \frac{1}{2} \sigma _t\left( \delta \omega \right) ^2 {+} \frac{\Gamma _V^2\sigma _t}{4} {-} i \Gamma _V\sigma _t\delta \omega }\Bigg ] \nonumber \\&\qquad \times \frac{1}{ {\mathfrak {T}}-T_{\textrm{in}}- {\Gamma _V\sigma _t\over 2} -i \sigma _t \delta \omega } \nonumber \\&\quad \rightarrow \sqrt{ \frac{\pi ^5}{\sigma _t} \left( \frac{\sigma _0\sigma _1\sigma _2}{\sigma _s} \right) ^3 } \int _{T_{\textrm{in}}}^{\infty } \text {d}{\mathfrak {T}}' \nonumber \\&\qquad \times \textrm{exp}\Bigg [{}{ - \frac{1}{2\sigma _t} \left( {\mathfrak {T}}' - \left( T_{\textrm{in}}{-{\Gamma _V\sigma _t\over 2}} + i\sigma _t\delta \omega \right) \right) ^2 } \nonumber \\ {}&\quad \qquad {{-} \Gamma _V\left( T_{\textrm{in}}{-}T_0\right) {-} \frac{1}{2} \sigma _t\left( \delta \omega \right) ^2 {+} \frac{\Gamma _V^2\sigma _t}{4} {-} i \Gamma _V\sigma _t\delta \omega }\Bigg ] \nonumber \\&\qquad \times \frac{1}{ {\mathfrak {T}}' - T_{\textrm{in}}-i \sigma _t \delta \omega }, \end{aligned}$$

(149)

where in the last fine, we changed the variable to ${\mathfrak {T}}':= {\mathfrak {T}} - \Gamma _V\sigma _t/2$ and took the limit $T_{\textrm{out}}\rightarrow \infty$.

In order to use the analytic formula for $\sigma _t >0$ and $\alpha \in {\mathbb {C}}$,³²

$$\begin{aligned} \int _{T_{\textrm{in}}}^\infty \text {d}t \frac{1}{t - \alpha } e^{- \frac{1}{2\sigma _t} \left( t-\alpha \right) ^2} = -\frac{1}{2}\, {{\,\textrm{Ei}\,}}\!\left( - \frac{1}{2\sigma _t} \left( T_{\textrm{in}}-\alpha \right) ^2 \right) , \end{aligned}$$

(150)

where ${{\,\textrm{Ei}\,}}\!\left( z\right)$ is the exponential integral function defined by the principal value of

$$\begin{aligned} {{\,\textrm{Ei}\,}}\!\left( z\right) := - \int _{-z}^\infty \text {d}t \frac{e^{-t}}{t}, \end{aligned}$$

(151)

and we add an extra term in the denominator of the integrand of $I_\text {intf}$ such that

$$\begin{aligned} I_\text {intf}&\sim \sqrt{ \frac{\pi ^5}{\sigma _t} \left( \frac{\sigma _0\sigma _1\sigma _2}{\sigma _s} \right) ^3 } \int _{T_{\textrm{in}}}^{\infty } \text {d}{\mathfrak {T}}'\nonumber \\&\quad \times \textrm{exp}\Bigg [{}{ - \frac{1}{2\sigma _t} \left( {\mathfrak {T}}' - \left( T_{\textrm{in}}{-{\Gamma _V\sigma _t\over 2}} + i\sigma _t\delta \omega \right) \right) ^2 } \nonumber \\ {}&\quad {{-} \Gamma _V\left( T_{\textrm{in}}{-}T_0\right) {-} \frac{1}{2} \sigma _t\left( \delta \omega \right) ^2 {+} \frac{\Gamma _V^2\sigma _t}{4} {-} i \Gamma _V\sigma _t\delta \omega }\Bigg ] \nonumber \\&\quad \times \frac{1}{ {\mathfrak {T}}' - {\left( T_{\textrm{in}}- {\Gamma _V\sigma _t\over 2} + i\sigma _t\delta \omega \right) } }, \end{aligned}$$

(152)

which would underestimate the integral to some extent. Now we reach the analytic form

$$\begin{aligned} I_\text {intf}&\sim \sqrt{ \frac{\pi ^5}{\sigma _t} \left( \frac{\sigma _0\sigma _1\sigma _2}{\sigma _s} \right) ^3 } e^{ - \Gamma _V\left( T_{\textrm{in}}-T_0\right) - \frac{1}{2} \sigma _t\left( \delta \omega \right) ^2 + \frac{\Gamma _V^2\sigma _t}{4} - i \Gamma _V\sigma _t\delta \omega }\nonumber \\&\quad \times \left( -\frac{1}{2}{{\,\textrm{Ei}\,}}\!\left( -X' \right) \right) , \end{aligned}$$

(153)

with

$$\begin{aligned} X' := \frac{\sigma _t}{2} \left( \Gamma _V + i \delta \omega \right) ^2. \end{aligned}$$

(154)

Here, we will take the leading term of the expansion around infinity for $X'$:

$$\begin{aligned} - {{\,\textrm{Ei}\,}}\!\left( -X' \right) = e^{ - X' + \mathcal{O}\left( \frac{1}{{X'}^2} \right) } \left[ \frac{1}{X'} + \mathcal{O}\!\left( \frac{1}{{X'}^2} \right) \right] , \end{aligned}$$

(155)

which leads to

$$\begin{aligned} I_\text {intf} + \left( I_\text {intf}\right) ^*&\sim \frac{1}{2} \sqrt{ \frac{\pi ^5}{\sigma _t} \left( \frac{\sigma _0\sigma _1\sigma _2}{\sigma _s} \right) ^3 } e^{ - \Gamma _V\left( T_{\textrm{in}}-T_0\right) - \frac{\Gamma _V^2\sigma _t}{4} }\nonumber \\&\quad \times \left[ \frac{ e^{ -2i \Gamma _V\sigma _t \delta \omega } }{{\sigma _t\over 2}\left( \Gamma _V+i\delta \omega \right) ^2} + \frac{ e^{ 2i \Gamma _V\sigma _t \delta \omega } }{{\sigma _t\over 2}\left( \Gamma _V-i\delta \omega \right) ^2} \right] ,\nonumber \\ \end{aligned}$$

(156)

and

$$\begin{aligned}&dP^\text {intf}_{V \rightarrow P\overline{P}} \nonumber \\&\quad \sim - |g_\text {eff}|^2 N_V^2 \frac{1}{2 E_0} \frac{\text {d}^3 {\varvec{P}}_1}{(2\pi )^3 2E_1} \frac{\text {d}^3 {\varvec{P}}_2}{(2\pi )^3 2E_2} (2 \pi )^4\nonumber \\&\qquad \times \left( \sqrt{\frac{\sigma _t}{\pi }} e^{-\sigma _t\left( \delta \omega \right) ^2} \right) \nonumber \\&\qquad \times \left[ \left( \frac{\sigma _s}{\pi }\right) ^{3/2} e^{-\sigma _s\left( \delta {\varvec{P}}\right) ^2} \right] \nonumber \\&\qquad \times e^{ - \Gamma _V\left( T_{\textrm{in}}-T_0\right) - \frac{\Gamma _V^2\sigma _t}{4} + \frac{1}{2} \sigma _t\left( \delta \omega \right) ^2 } \times \sqrt{\frac{2\sigma _t}{\pi }} \nonumber \\&\qquad \times \frac{ \left( \Gamma _V^2 - \left( \delta \omega \right) ^2\right) \cos \!\left( 2 \Gamma _V\sigma _t\delta \omega \right) - 2 \Gamma _V\delta \omega \sin \!\left( 2 \Gamma _V\sigma _t\delta \omega \right) }{\sigma _t\left( \Gamma _V^2 + \left( \delta \omega \right) ^2 \right) ^2} \nonumber \\&\quad \times \left| \widetilde{F}\!\left( V_-\right) \right| ^2. \end{aligned}$$

(157)

We perform the momentum integrals in the nonrelativistic limit:

$$\begin{aligned}&P^\text {intf}_{V \rightarrow P\overline{P}} \Rightarrow - N_V^2 e^{ -\Gamma _V\left( T_{\textrm{in}}-{T_0}\right) } \frac{g_V^2}{3} \frac{1}{2 m_V} \frac{1}{(2\pi )^2 4 E_1 E_2} \nonumber \\&\quad \times \frac{m_P^6}{8} \left( \int _{0}^{\infty } 4 \pi V_+^2 \text {d}V_+ \right) \left( \int _0^\infty 4\pi V_-^2 \text {d}V_- \right) \nonumber \\&\quad \times \frac{1}{\pi ^2} \sigma _t^{1/2} \sigma _s^{3/2} \left( m_P^2 V_-^2\right) e^{ - F_\text {intf}(V_+, V_-) } \sqrt{\frac{2\sigma _t}{\pi }} \nonumber \\&\quad \times \frac{ \left( \Gamma _V^2 - \left( \delta \omega \right) ^2\right) \cos \!\left( 2 \Gamma _V\sigma _t\delta \omega \right) - 2 \Gamma _V\delta \omega \sin \!\left( 2 \Gamma _V\sigma _t\delta \omega \right) }{\sigma _t\left( \Gamma _V^2 + \left( \delta \omega \right) ^2 \right) ^2} \nonumber \\&\quad \times \left| \widetilde{F}\!\left( V_-\right) \right| ^2, \end{aligned}$$

(158)

where

$$\begin{aligned}&F_\text {intf}(V_+, V_-) :=\, \sigma _s\left( \delta {\varvec{P}}\right) ^2 + \frac{1}{2} \sigma _t\left( \delta \omega \right) ^2 + \frac{\Gamma _V^2\sigma _t}{4} \nonumber \\&\quad =\, \sigma _s m_P^2 V_+^2 \nonumber \\&\qquad + \underbrace{\frac{2 \sigma _P}{ \left( 1 - \frac{2\sigma _s}{\sigma _P} \right) V_+^2 + V_-^2 }}_{= \sigma _t} \Bigg \{ \frac{1}{2} \left[ m_P \left( \frac{1}{4} - \frac{\sigma _s}{\sigma _P} \right) V_+^2 \right. \nonumber \\&\qquad \left. + \frac{1}{4} m_P V_-^2 - \left( m_V - 2m_P\right) \right] ^2 + {\Gamma _V^2\over 4} \Bigg \}. \end{aligned}$$

(159)

This function is similar to $F_\text {bulk}$, but a factor of the half comes in front of $\sigma _t\left( \delta \omega \right) ^2$. As in the case of the bulk part, we can see

$$\begin{aligned} e^{ - F_\text {intf} } \underbrace{\rightarrow }_{V_+ \rightarrow \infty } 0, \quad e^{ - F_\text {intf} } \underbrace{\rightarrow }_{V_- \rightarrow \infty } 0. \end{aligned}$$

(160)

Stationary points $(V_+^s, V_-^s)$ are defined by

$$\begin{aligned} \frac{\partial F_\text {intf} (V_+^s, V_-^s)}{\partial V_+} = 0 \quad \text {and} \quad \frac{\partial F_\text {intf} (V_+^s, V_-^s)}{\partial V_-} = 0, \end{aligned}$$

(161)

and we find one stationary point in the kinetic region ($V_+ \simeq 0$ and $V_- > 0$),

$$\begin{aligned} \left( V_+^\text {I}, V_-^\text {I}\right)&:= \left( 0, \, \frac{ 2 \left[ \left( m_V-2m_P\right) ^2+{\Gamma _V^2\over 2}\right] ^{1/4} }{\sqrt{m_P}} \right) . \end{aligned}$$

(162)

Note that

$$\begin{aligned} {F_\text {intf}^0}&= F_\text {intf}\left( V_+^\text {I}, V_-^\text {I}\right) \nonumber \\&:= \frac{ m_P \sigma _P }{2} \left[ -\left( m_V - 2m_P\right) + \sqrt{ \left( m_V - 2m_P\right) ^2+{\Gamma _V^2 \over 2} } \right] , \end{aligned}$$

(163)

which takes a positive value when $\Gamma _V$ is finite, and goes to zero in the (unphysical) limit $\Gamma _V \rightarrow 0$. Also, we can see

$$\begin{aligned} \left. \frac{\partial ^2 F_\text {intf} }{\partial V_+^2}\right| _{\left( V_+^\text {I}, V_-^\text {I}\right) }&= \frac{1}{2} m_P^2 \sigma _s \left[ 3 + \frac{ m_V - 2m_P}{\sqrt{\left( m_V-2m_P\right) ^2+{\Gamma _V^2\over 2}}} \right] \nonumber \\&=: \left( \frac{1}{2} m_P^2 \sigma _s\right) 4{A_\text {intf}}, \nonumber \\ \left. \frac{\partial ^2 F_\text {intf} }{\partial V_-^2}\right| _{\left( V_+^\text {I}, V_-^\text {I}\right) }&= \frac{1}{2} m_P^2 \sigma _P, \nonumber \\ \left. \frac{\partial ^2 F_\text {intf} }{\partial V_+ \partial V_-}\right| _{\left( V_+^\text {I}, V_-^\text {I}\right) }&= 0, \end{aligned}$$

(164)

where $A_\text {intf} \rightarrow 1$ under the (unphysical) limit $\Gamma _V \rightarrow 0$.

Now we evaluate the nonrelativistic integral in Eq. (158). By use of Eqs. (137) and (138), we reach

$$\begin{aligned}&P^\text {intf}_{V \rightarrow P\overline{P}} \sim - \left( \frac{g_V^2 m_P^3 N_V^2 e^{ -\Gamma _V\left( T_{\textrm{in}}-{T_0}\right) } }{12 \pi m_V E_1 E_2}\right) \frac{ m_P \sqrt{\sigma _P}}{ 2\sqrt{2} \sqrt{\pi }} \left( V_-^\text {I} \right) ^2 \nonumber \\&\quad \times \frac{1}{2} \left[ 1 + \text {erf}\left( \frac{ m_P\sqrt{\sigma _P} {V_-^\text {I}} }{2} \right) \right] {\frac{e^{-F^0_\text {intf}}}{A_\text {intf}^{3/2}}}\, \left| \widetilde{F}\!\left( V^\text {I}_-\right) \right| ^2 \nonumber \\&\quad \times \left[ \frac{ \left( \Gamma _V^2 - \left( \widetilde{\delta \omega }\right) ^2 \right) \cos \!\left( 2 \Gamma _V\widetilde{\sigma _t} \widetilde{\delta \omega }\right) - 2 \Gamma _V \widetilde{\delta \omega } \sin \!\left( 2 \Gamma _V\widetilde{\sigma _t} \widetilde{\delta \omega }\right) }{\widetilde{\sigma _t}\left( \Gamma _V^2 + \left( \widetilde{\delta \omega }\right) ^2 \right) ^2} \right] , \end{aligned}$$

(165)

where the factor $\sqrt{\sigma _P}$ comes in the overall factor [instead of $\Gamma _V^{-1}$ compared with the bulk result in Eq. (136)], and we defined the parameters

$$\begin{aligned} \widetilde{\sigma _t}&:= \sigma _t \Big |_{V_+ \rightarrow V_+^\text {I},\, V_- \rightarrow V_-^\text {I}} = \left. \frac{2 \sigma _P}{ \left( 1 - \frac{2\sigma _s}{\sigma _P} \right) V_+^2 + V_-^2 } \right| _{V_+ \rightarrow V_+^\text {I},\, V_- \rightarrow V_-^\text {I}} \nonumber \\&= \frac{2 \sigma _P}{ \left( V_-^\text {I}\right) ^2 }, \end{aligned}$$

(166)

$$\begin{aligned} \widetilde{\delta \omega }&:= \delta \omega \Big |_{V_+ \rightarrow V_+^\text {I},\, V_- \rightarrow V_-^\text {I}} \nonumber \\&= \left. \left[ m_P \left( \frac{1}{4} - \frac{\sigma _s}{\sigma _P} \right) V_+^2 + \frac{1}{4} m_P V_-^2 - \left( m_V - 2m_P\right) \right] \right| _{V_+ \rightarrow V_+^\text {I},\, V_- \rightarrow V_-^\text {I}} \nonumber \\&= \frac{1}{4} m_P \left( V_-^\text {I}\right) ^2 - \left( m_V - 2m_P\right) . \end{aligned}$$

(167)

Appendix C: Plane-wave decay rate

From the effective Hamiltonian (7), under the use of the form factor (105), it is immediate to get the following form in the plane-wave formalism for the resting V:

$$\begin{aligned} \Gamma ^\text {plane}_{V \rightarrow P \overline{P}}&= \frac{2}{3} \left( \frac{{g_{VP}}^2}{4\pi } \right) \frac{ |{\varvec{k}}_{P}|^3 }{m_V^2} \left| \widetilde{F} \!\left( \frac{{\varvec{k}}_1 - {\varvec{k}}_2}{2}\right) \right| ^2 \nonumber \\&= \frac{{g_{VP}}^2}{48 \pi m_V^2} \left( m_V^2 - 4 m_{P}^2 \right) ^{3/2} \left| \frac{1}{\left( \frac{R_0 \left( {\varvec{k}}_1 - {\varvec{k}}_2\right) }{2}\right) ^2 + 1 } \right| ^2, \end{aligned}$$

(168)

where $g_{VP}$ represents $g_{V+}$ (for $P = P^+$) or $g_{V0}$ (for $P = P^0$), and the magnitude of the final-state momenta in the center of mass frame is given as

$$\begin{aligned} \left| {\varvec{k}}_P\right|&= \left| {\varvec{k}}_1\right| = \left| {\varvec{k}}_2\right| = \frac{1}{2} \left( m_V^2 - 4 m_{P}^2 \right) ^{1/2}. \end{aligned}$$

(169)

The form factor part does not take the nonrelativistic limit as in Eq. (105), and currently, ${\varvec{k}}_1 = - {\varvec{k}}_2$, and thus

$$\begin{aligned} \left| {\varvec{k}}_1 - {\varvec{k}}_2 \right| = 2 \left| {\varvec{k}}_1\right| = \left( m_V^2 - 4 m_{P}^2 \right) ^{1/2}. \end{aligned}$$

(170)

Note that under the nonrelativistic approximation taken in Eqs. (101) and (102), it is easy to obtain the approximated form as

$$\begin{aligned}&\Gamma ^\text {plane}_{V \rightarrow P \overline{P}} \Rightarrow \Gamma ^\text {plane,non-rel}_{V \rightarrow P \overline{P}} \nonumber \\&\quad := \frac{{g_{VP}}^2 m_P^2}{12 \pi m_V} \left( \frac{m_V - 2 m_P}{m_P} \right) ^{3/2} \left| \frac{1}{\left( \frac{R_0 m_P \left( {\varvec{V}}_1 - {\varvec{V}}_2\right) }{2}\right) ^2 + 1 } \right| ^2, \end{aligned}$$

(171)

$$\begin{aligned}&\left| {\varvec{V}}_1 - {\varvec{V}}_2\right| \approx \frac{ 2\left( m_V - 2m_P\right) ^{1/2} }{ \sqrt{m_P} }. \end{aligned}$$

(172)

Appendix D: Comparison with the plane-wave decay rate

As shown in Sect. 3, the boundary contribution dominates over the bulk and interference ones in all regions of the parameter space $R_0$ and $\sigma _P$. This is because of the fast decay of the vector meson V due to strong interactions. The fast decay suppresses the contribution from the bulk so that the contribution from the initial time boundary becomes more significant compared to the bulk one.

As mentioned above, the plane-wave decay width $\Gamma _V$ is dependent on other theory parameters such as $g_V$, $m_P$, and $m_V$. Therefore, it is meaningless to take an “on-shell” limit $\Gamma _V\rightarrow 0$ with other parameters being fixed. Nevertheless, one might pretend that one can take this unphysical limit, and then one extracts the plane-wave decay width (171) as

$$\begin{aligned} \Gamma _{V\rightarrow P{\overline{P}}}^\text {plane,non-rel}&= \lim _{\sigma _P\rightarrow \infty }\left( \lim _{\Gamma _V\rightarrow 0}\Gamma _VP_{V\rightarrow P{\overline{P}}}\right) \nonumber \\&= \lim _{\sigma _P\rightarrow \infty }\left( \lim _{\Gamma _V\rightarrow 0}\Gamma _VP_{V\rightarrow P{\overline{P}}}^\text {bulk}\right) , \end{aligned}$$

(173)

where the limit of large wave-packet size $\sigma _P\rightarrow \infty$ is taken after the limit $\Gamma _V\rightarrow 0$.³³ The second equality in Eq. (173) is derived as follows: Under the limit $\Gamma _V\rightarrow 0$, the two ratios $\Gamma _VP^\text {bdry}_{V \rightarrow P\overline{P}}$ and $\Gamma _VP^\text {intf}_{V \rightarrow P\overline{P}}$ approach zero since $P^\text {bdry}_{V \rightarrow P\overline{P}}$ and $P^\text {intf}_{V \rightarrow P\overline{P}}$ are not proportional to $\Gamma _V^{-1}$ as shown in Eqs. (45) and (48). When taking the above limits $\Gamma _V\rightarrow 0$ and $\sigma _P\rightarrow \infty$, the following is satisfied:

$$\begin{aligned} V_-^\text {B}&\rightarrow 2 \frac{\sqrt{m_V-2m_P}}{\sqrt{m_P}},&\text {erf}\left( \frac{ m_P\sqrt{\sigma _P} {V_-^\text {B}} }{\sqrt{2}} \right)&\rightarrow 1, \nonumber \\ F_\text {bulk}^0&\rightarrow 0,&A_\text {bulk}&\rightarrow 1, \end{aligned}$$

(174)

as is the physical requirement $N_V \rightarrow 1$ from $\Gamma _V\rightarrow 0$.

Fig. 16

Values of $R_\rho$ defined in Eq. (175) are depicted for $R_0 = 0.0015\,\text {MeV}^{-1}$ (left panel) and $R_0 = 0.01\,\text {MeV}^{-1}$ (right panel). The captions “Wave-Packet (full),” “Plane-Wave (rel),” and “Plane-Wave parton-level” correspond to $R_\rho ^\text {WP}$, $R_\rho ^\text {plane}$, and $R_\rho ^\text {parton}$, respectively. See the main text of this section for other details

In the actual setup, the (unphysical) $\Gamma _V\rightarrow 0$ limit is not good, and we see that the boundary contribution dominates over the bulk one for the parameters corresponding to the real experiments mainly because of the exponential suppression factor $e^{-F^0_\text {bulk}}$.

Appendix E: A brief comment on the isospin breaking of the $\rho$ system

We will make a brief comment on the isospin breaking of the $\rho$ system. Here, we define the following ratio:

$$\begin{aligned} R_\rho ^\text {WP}&:= {P_{\rho ^+ \rightarrow \pi ^+ \pi ^0}\over P_{\rho ^0 \rightarrow \pi ^+ \pi ^-}},\nonumber \\ R_\rho ^\text {plane}&:= \frac{\Gamma ^\text {plane}_{\rho ^+ \rightarrow \pi ^+ \pi ^0}}{\Gamma ^\text {plane}_{\rho ^0 \rightarrow \pi ^+ \pi ^-}},\nonumber \\ R_\rho ^\text {parton}&:= \frac{\left. \Gamma ^\text {plane}_{\rho ^+ \rightarrow \pi ^+ \pi ^0}\right| _\text {without form factor}}{\left. \Gamma ^\text {plane}_{\rho ^0 \rightarrow \pi ^+ \pi ^-}\right| _\text {without form factor}}, \end{aligned}$$

(175)

where we replace $m_P$ to $\left( m_{\pi ^+} + m_{\pi ^0}\right) /2$ for the calculations of $\rho ^+ \rightarrow \pi ^+ \pi ^0$. In Fig. 16, the $R_\rho$ in terms of the wave packet and the relativistic plane wave is depicted for $R_0 = 0.0015\,\text {MeV}^{-1}$ (left panel) and $R_0 = 0.01\,\text {MeV}^{-1}$ (right panel); see Sects. 2.3 and 4.3 for the details of the wave-packet decay probabilities and the plane-wave decay rates, respectively.

First, we mention the experimental inputs that we adopt. As official results reported by the PDG [1],

• Six digits are reported as typical mass scales of the broad resonant $\rho$ system, where their central values are located from $769.0\,\text {MeV}$ to $775.26\,\text {MeV}$.

• Also, six digits are shown as typical width scales of the $\rho$ system, where their central values are located from $147.4\,\text {MeV}$ to $151.5\,\text {MeV}$.

• The difference between $m_{\rho ^0}$ and $m_{\rho ^+}$ takes $m_{\rho ^0} - m_{\rho ^+} = -0.7 \pm 0.8\,\text {MeV}$.

• The difference between $\Gamma _{\rho ^0}$ and $\Gamma _{\rho ^+}$ takes $\Gamma _{\rho ^0} - \Gamma _{\rho ^+} = 0.3 \pm 1.3\,\text {MeV}$.

Since $R_\rho$ measures isospin-violating effects, it is thus insensitive to what is a typical mass scale of the system. So we simply take $m_{\rho ^0} = 770 \pm 1\,\text {MeV}$ and $\Gamma _{\rho ^0} = 147.4 \pm 0.8\,\text {MeV}$. Based on the mass scale, $m_{\rho ^+}$ is estimated by use of the above data on $m_{\rho ^0}$ and $m_{\rho ^0} - m_{\rho ^+}$ as

$$\begin{aligned} m_{\rho ^+}&= 770.7\,\text {MeV}\,\text {(central)},\\ m_{\rho ^+}&= 771.5\,\text {MeV}\,(+1\sigma ),\\ m_{\rho ^+}&= 772.3\,\text {MeV}\,(+2\sigma ), \end{aligned}$$

while the central value of $\Gamma _{\rho ^+}$ is similarly estimated as $147.7\,\text {MeV}$. As expected and as shown in Fig. 16, $R_\rho$ can depend on the mass difference notably.

Next, we discuss the numerical result shown in Fig. 16. As expected, the experimental result is located very near the unity since the $\rho$ vector mesons do not contain heavy quarks. The plane-wave theoretical predictions with and without the form-factor effect show similar results. The wave-packet predictions deviate from the unity several percent upward, showing fewer agreements. Nevertheless, this does not necessarily mean that wave-packet formalism works less effectively for the $\rho$ system than plane-wave formalism because the current wave-packet result is precise only for nonrelativistic systems (due to the usage of nonrelativistic approximations). Note that the decays $\rho ^+ \rightarrow \pi ^+ \pi ^0$ and $\rho ^0 \rightarrow \pi ^+ \pi ^-$ are fully relativistic due to the large difference between the total masses of the initial state and final state. Several percent of theoretical errors are expected from the fully relativistic wave-packet prediction, which is beyond the scope of this paper. The typical scale of the form factor under the current scheme $R_0 = 0.0015\,\text {MeV}$ works well compared with $R_0 = 0.01\,\text {MeV}$.

Also, we comment on the dependence on $R_0$ in $R_\rho$. As typically observed in the curves of “Plane-Wave (rel)” of Fig. 16, the form-factor part of $R_\rho$ is not sensitive to $R_0$ since the total mass differences between the initial and the final states take almost the same values in $\rho ^+ \rightarrow \pi ^+ \pi ^0$ and $\rho ^0 \rightarrow \pi ^+ \pi ^-$, and their final-state phase spaces are wide.