On flux integrals for generalized Melvin solution related to simple finite-dimensional Lie algebra

Abstract

A generalized Melvin solution for an arbitrary simple finite-dimensional Lie algebra $\mathcal G$ is considered. The solution contains a metric, n Abelian 2-forms and n scalar fields, where n is the rank of $\mathcal G$. It is governed by a set of n moduli functions $H_s(z)$ obeying n ordinary differential equations with certain boundary conditions imposed. It was conjectured earlier that these functions should be polynomials—the so-called fluxbrane polynomials. These polynomials depend upon integration constants $q_s$, $s = 1,\dots ,n$. In the case when the conjecture on the polynomial structure for the Lie algebra $\mathcal G$ is satisfied, it is proved that 2-form flux integrals $\Phi ^s$ over a proper 2d submanifold are finite and obey the relations $q_s \Phi ^s = 4 \pi n_s h_s$, where the $h_s > 0$ are certain constants (related to dilatonic coupling vectors) and the $n_s$ are powers of the polynomials, which are components of a twice dual Weyl vector in the basis of simple (co-)roots, $s = 1,\dots ,n$. The main relations of the paper are valid for a solution corresponding to a finite-dimensional semi-simple Lie algebra $\mathcal G$. Examples of polynomials and fluxes for the Lie algebras $A_1$, $A_2$, $A_3$, $C_2$, $G_2$ and $A_1 + A_1$ are presented.

1 Introduction

In this paper we start with a generalization of a Melvin solution [1], which was presented earlier in Ref. [2]. It appears in the model which contains a metric, n Abelian 2-forms and $l \ge n$ scalar fields. This solution is governed by a certain non-degenerate (quasi-Cartan) matrix $(A_{s s'})$, $s, s' = 1, \dots , n$. It is a special case of the so-called generalized fluxbrane solutions from Ref. [3]. For fluxbrane solutions see Refs. [4,5,6,7,8,9,10,11,12,13,14,15,16,17,18,19,20,21,22,23,24,25,26,27,28] and the references therein. The appearance of fluxbrane solutions was motivated by superstring/M theory.

The generalized fluxbrane solutions from Ref. [3] are governed by moduli functions, $H_s(z) > 0$, defined on the interval $(0, +\infty )$, where $z = \rho ^2$ and $\rho$ is a radial variable. These functions obey a set of n non-linear differential master equations governed by the matrix $(A_{s s'})$, equivalent to Toda-like equations, with the following boundary conditions imposed: $H_{s}(+ 0) = 1$, $s = 1,\ldots ,n$.

In this paper we assume that $(A_{s s'})$ is a Cartan matrix for some simple finite-dimensional Lie algebra $\mathcal G$ of rank n ($A_{ss} = 2$ for all s). According to a conjecture suggested in Ref. [3], the solutions to the master equations with the boundary conditions imposed are polynomials:

$$\begin{aligned} H_{s}(z) = 1 + \sum _{k = 1}^{n_s} P_s^{(k)} z^k, \end{aligned}$$

(1.1)

where the $P_s^{(k)}$ are constants. Here $P_s^{(n_s)} \ne 0$ and

$$\begin{aligned} n_s = 2 \sum _{s' =1}^{n} A^{s s'}, \end{aligned}$$

(1.2)

where we denote $(A^{s s'}) = (A_{s s'})^{-1}$. The integers $n_s$ are components of a twice dual Weyl vector in the basis of simple (co-)roots [29].

The set of fluxbrane polynomials $H_s$ defines a special solution to open Toda chain equations [30, 31] corresponding to a simple finite-dimensional Lie algebra $\mathcal G$ [32]. In Refs. [2, 33] a program (in Maple) for the calculation of these polynomials for the classical series of Lie algebras (A-, B-, C- and D-series) was suggested. It was pointed out in Ref. [3] that the conjecture on the polynomial structure of $H_{s}(z)$ is valid for Lie algebras of the A- and C-series. In Ref. [34] the conjecture from Ref. [3] was verified for the Lie algebra $E_6$ and certain duality relations for six $E_6$-polynomials were proved. In Sect. 2 we present the generalized Melvin solution from Ref. [2]. In Sect. 3 we deal with the generalized Melvin solution for an arbitrary simple finite-dimensional Lie algebra $\mathcal G$. Here we calculate 2-form flux integrals $\Phi ^s = \int _{M_{*}} F^s$, where $F^s$ are 2-forms and $M_{*}$ is a certain 2d submanifold. These integrals (fluxes) are finite when moduli functions are polynomials. In Sect. 3 we consider examples of fluxbrane polynomials and fluxes for the Lie algebras: $A_1$, $A_2$, $A_3$, $C_2$, $G_2$ and $A_1 + A_1$.

2 The solutions

We consider a model governed by the action

$$\begin{aligned} S= & {} \int d^Dx \sqrt{|g|} \biggl \{R[g]- h_{\alpha \beta }g^{MN}\partial _M\varphi ^{\alpha }\partial _N\varphi ^{\beta }\nonumber \\&-\frac{1}{2} \sum _{s =1}^{n}\exp [2\lambda _s(\varphi )](F^s)^2 \biggr \} \end{aligned}$$

(2.1)

where $g=g_{MN}(x)\mathrm{d}x^M\otimes \mathrm{d}x^N$ is a metric, $\varphi =(\varphi ^\alpha )\in {\mathbb R}^l$ is a set of scalar fields, $(h_{\alpha \beta })$ is a constant symmetric non-degenerate $l\times l$ matrix $(l\in {\mathbb N})$, $F^s = dA^s = \frac{1}{2} F^s_{M N} \mathrm{d}x^{M} \wedge \mathrm{d}x^{N}$ is a 2-form, $\lambda _s$ is a 1-form on ${\mathbb R}^l$: $\lambda _s(\varphi )=\lambda _{s \alpha }\varphi ^\alpha$, $s = 1,\ldots , n$; $\alpha =1,\dots ,l$. Here $(\lambda _{s \alpha })$, $s =1,\dots , n$, are dilatonic coupling vectors. In (2.1) we denote $|g| = |\det (g_{MN})|$, $(F^s)^2 = F^s_{M_1 M_{2}} F^s_{N_1 N_{2}} g^{M_1 N_1} g^{M_{2} N_{2}}$, $s = 1,\dots , n$.

Here we start with a family of exact solutions to field equations corresponding to the action (2.1) and depending on one variable $\rho$. The solutions are defined on the manifold

$$\begin{aligned} M = (0, + \infty ) \times M_1 \times M_2, \end{aligned}$$

(2.2)

where $M_1$ is a one-dimensional manifold (say $S^1$ or ${\mathbb R}$) and $M_2$ is a (D-2)-dimensional Ricci-flat manifold. The solution reads [2]

$$\begin{aligned} g= & {} \Bigl (\prod _{s = 1}^{n} H_s^{2 h_s /(D-2)} \Bigr ) \biggl \{ w \mathrm{d}\rho \otimes \mathrm{d}\rho \nonumber \\&+ \Bigl (\prod _{s = 1}^{n} H_s^{-2 h_s} \Bigr ) \rho ^2 \mathrm{d}\phi \otimes \mathrm{d}\phi + g^2 \biggr \}, \end{aligned}$$

(2.3)

$$\begin{aligned} \exp (\varphi ^\alpha )= & {} \prod _{s = 1}^{n} H_s^{h_s \lambda _{s}^\alpha }, \end{aligned}$$

(2.4)

$$\begin{aligned} F^s= & {} q_s \left( \prod _{s' = 1}^{n} H_{s'}^{- A_{s s'}} \right) \rho \mathrm{d}\rho \wedge \mathrm{d}\phi , \end{aligned}$$

(2.5)

$s = 1,\dots , n$; $\alpha = 1,\dots , l$, where $w = \pm 1$, $g^1 = \mathrm{d}\phi \otimes \mathrm{d}\phi$ is a metric on $M_1$ and $g^2$ is a Ricci-flat metric on $M_{2}$. Here $q_s \ne 0$ are integration constants, $q_s = - Q_s$ in the notations of Ref. [2], $s = 1,\dots , n$.

The functions $H_s(z) > 0$, $z = \rho ^2$, obey the master equations

$$\begin{aligned} \frac{\mathrm{d}}{\mathrm{d}z} \left( \frac{ z}{H_s} \frac{\mathrm{d}}{\mathrm{d}z} H_s \right) = P_s \prod _{s' = 1}^{n} H_{s'}^{- A_{s s'}}, \end{aligned}$$

(2.6)

with the following boundary conditions:

$$\begin{aligned} H_{s}(+ 0) = 1, \end{aligned}$$

(2.7)

where

$$\begin{aligned} P_s = \frac{1}{4} K_s q_s^2, \end{aligned}$$

(2.8)

$s = 1,\dots ,n$. The boundary condition (2.7) guarantees the absence of a conic singularity [in the metric (2.3)] for $\rho = +0$.

The parameters $h_s$ satisfy the relations

$$\begin{aligned} h_s = K_s^{-1}, \quad K_s = B_{s s} > 0, \end{aligned}$$

(2.9)

where

$$\begin{aligned} B_{ss'} \equiv 1 +\frac{1}{2-D}+ \lambda _{s \alpha } \lambda _{s' \beta } h^{\alpha \beta }, \end{aligned}$$

(2.10)

$s, s' = 1,\ldots , n$, with $(h^{\alpha \beta })=(h_{\alpha \beta })^{-1}$. In the relations above we denote $\lambda _{s}^{\alpha } = h^{\alpha \beta } \lambda _{s \beta }$ and

$$\begin{aligned} (A_{ss'}) = \left( 2 B_{s s'}/B_{s' s'} \right) . \end{aligned}$$

(2.11)

The latter is the so-called quasi-Cartan matrix.

We note that the constants $B_{s s'}$ and $K_s = B_{s s}$ have a certain mathematical sense. They are related to scalar products of certain vectors $U^s$ (brane vectors, or U-vectors), which belong to a certain linear space (“truncated target space”, for our problem it has dimension $l+2$), i.e. $B_{s s'} =(U^s,U^{s'})$ and $K_s = (U^s,U^{s})$ [35,36,37]. The scalar products of such a type are of physical significance, since they appear for various solutions with branes, e.g. black branes, S-branes, fluxbranes etc. Several physical parameters in multidimensional models with branes, e.g. the Hawking-like temperatures and the entropies of black holes and branes, PPN parameters, Hubble-like parameters, fluxes etc., contain such scalar products; see [36, 37] and Sect. 3 of this paper. The relation (2.11) defines generalized intersection rules for branes which were suggested in [35]. The constants $K_s$ are invariants of dimensional reduction. It is well known, see [37] and the references therein, that $K_s = 2$ for branes in numerous supergravity models, e.g. in dimensions $D = 10,11$.

It may be shown that if the matrix $(h_{\alpha \beta })$ has an Euclidean signature and $l \ge n$, and $(A_{ss'})$ is a Cartan matrix for a simple Lie algebra $\mathcal G$ of rank n, there exists a set of co-vectors $\lambda _1, \dots , \lambda _n$ obeying (2.11) (for $l = n$ see Remark 1 in the next section). Thus the solution is valid at least when $l \ge n$ and the matrix $(h_{\alpha \beta })$ is positive-definite.

The solution under consideration is a special case of the fluxbrane (for $w = +1$, $M_1 = S^1$) and S-brane ($w = -1$) solutions from [3] and [25], respectively.

If $w = +1$ and the (Ricci-flat) metric $g^2$ has a pseudo-Euclidean signature, we get a multidimensional generalization of Melvin’s solution [1].

In our notations Melvin’s solution (without scalar field) corresponds to $D = 4$, $n = 1$, $l =0$, $M_1 = S^1$ ($0< \phi < 2 \pi$), $M_2 = {\mathbb R}^2$, $g^2 = - \mathrm{d}t \otimes \mathrm{d}t + \mathrm{d}x \otimes \mathrm{d}x$ and $\mathcal{G} = A_1$.

For $w = -1$ and $g^2$ of Euclidean signature we obtain a cosmological solution with a horizon (as $\rho = + 0$) if $M_1 = {\mathbb R}$ ($- \infty< \phi < + \infty$).

3 Flux integrals for a simple finite-dimensional Lie algebra

Here we deal with the solution which corresponds to a simple finite-dimensional Lie algebra $\mathcal{G}$, i.e. the matrix $A = (A_{ss'})$ is coinciding with the Cartan matrix of this Lie algebra. We put also $n = l$, $w = + 1$ and $M_1 = S^1$, $h_{\alpha \beta } = \delta _{\alpha \beta }$ and denote $(\lambda _{s a }) = (\lambda _{s}^{a}) = \mathbf {\lambda }_{s}$, $s = 1, \dots , n$.

Due to (2.9)–(2.11) we get

$$\begin{aligned} K_s = \frac{D - 3}{D -2} + \mathbf {\lambda }_{s}^2, \end{aligned}$$

(3.1)

$h_s = K_s^{-1}$, and

$$\begin{aligned} \mathbf {\lambda }_{s} \mathbf {\lambda }_{l} = \frac{1}{2} K_l A_{sl} - \frac{D - 3}{D -2} \equiv \Gamma _{sl}, \end{aligned}$$

(3.2)

$s,l = 1, \dots , n$. [Equation (3.1) is a special case of (3.2)].

It follows from (2.9)–(2.11) that

$$\begin{aligned} \frac{h_i}{h_j} = \frac{K_{j}}{K_{i}} = \frac{B_{jj}}{B_{ii}} = \frac{B_{ji}}{B_{ii}} \frac{B_{jj}}{B_{ij}} = \frac{A_{ji}}{A_{ij}} \end{aligned}$$

(3.3)

for any $i \ne j$ obeying $A_{ij}, A_{ji} \ne 0$; $i,j = 1, \dots ,n$. It may be readily shown from (3.3) that the ratios $\frac{h_i}{h_j} = \frac{K_{j}}{K_{i}}$ are fixed numbers for any given Cartan matrix $({A_{ij}})$ of a simple (finite-dimensional) Lie algebra $\mathcal{G}$. (This follows from (3.3) and the connectedness of the Dynkin diagram of a simple Lie algebra.) The ratios (3.3) may be written as follows:

$$\begin{aligned} \frac{h_i}{h_j} = \frac{K_{j}}{K_{i}} = \frac{r_j }{r_i} \end{aligned}$$

(3.4)

$i \ne j$, where $r_i = (\alpha _{i}, \alpha _{i})$ is the length squared of a simple root $\alpha _{i}$ corresponding to the Lie algebra $\mathcal{G}$. Here we use the notations $A_{ij} = 2 (\alpha _{i}, \alpha _{j})/(\alpha _{j}, \alpha _{j})$; $i,j = 1, \dots ,n$. Equation (3.4) implies

$$\begin{aligned} K_{i} = \frac{1}{2} K r_i, \end{aligned}$$

(3.5)

$i = 1, \dots ,n$, where $K > 0$. (For simply laced (A, D, E) Lie algebras all $r_i$ are equal.)

Remark 1

For large enough K in (3.5) there exist vectors $\mathbf {\lambda }_s$ obeying (3.2) [and hence (3.1)]. Indeed, the matrix $(\Gamma _{sl})$ is positive-definite if $K > K_{*}$, where $K_{*}$ is some positive number. Hence there exists a matrix $\Lambda$, such that $\Lambda ^{T}\Lambda = \Gamma$. We put $(\Lambda _{as}) = (\lambda _{s}^a)$ and get the set of vectors obeying (3.2).

Now let us consider the oriented 2-dimensional manifold $M_{*} =(0, + \infty ) \times S^1$. The flux integrals

$$\begin{aligned} \Phi ^s= & {} \int _{M_{*}} F^s = \int _{0}^{+ \infty } \mathrm{d}\rho \int _{0}^{2 \pi } \mathrm{d}\phi \ \rho \mathcal{B}^s(\rho ^2) \nonumber \\= & {} 2 \pi \int _{0}^{+ \infty } \mathrm{d}\rho \ \rho \mathcal{B}^s(\rho ^2) , \end{aligned}$$

(3.6)

where

$$\begin{aligned} \mathcal{B}^s(\rho ^2) = q_s \prod _{l = 1}^{n} (H_{l}(\rho ^2))^{- A_{s l}}, \end{aligned}$$

(3.7)

are convergent for all s, if the conjecture for the Lie algebra $\mathcal{G}$ (on polynomial structure of moduli functions $H_s$) is obeyed for the Lie algebra $\mathcal{G}$ under consideration.

Indeed, due to the polynomial assumption (1.1) we have

$$\begin{aligned} H_s(\rho ^2) \sim C_s \rho ^{2n_s}, \quad C_s = P_s^{(n_s)}, \end{aligned}$$

(3.8)

as $\rho \rightarrow + \infty$; $s =1, \dots , n$. From (3.7), (3.8) and the equality $\sum _{1}^{n} A_{s l} n_l = 2$, following from (1.2), we get

$$\begin{aligned} \mathcal{B}^s(\rho ^2) \sim q_s C^s \rho ^{-4}, \quad C^s = \prod _{l = 1}^{n} C_l^{-A_{sl}}, \end{aligned}$$

(3.9)

and hence the integral (3.6) is convergent for any $s =1, \dots , n$.

By using the master equations (2.6) we obtain

$$\begin{aligned} \int _{0}^{+ \infty } \mathrm{d}\rho \rho \mathcal{B}^s(\rho ^2)= & {} q_s P_s^{-1} \frac{1}{2} \int _{0}^{+ \infty } \mathrm{d}z \frac{\mathrm{d}}{\mathrm{d}z} \left( \frac{ z}{H_s} \frac{\mathrm{d}}{\mathrm{d}z} H_s \right) \nonumber \\= & {} \frac{1}{2} q_s P_s^{-1} \lim _{z \rightarrow + \infty } \left( \frac{ z}{H_s} \frac{\mathrm{d}}{\mathrm{d}z} H_s \right) \nonumber \\= & {} \frac{1}{2} n_s q_s P_s^{-1}, \end{aligned}$$

(3.10)

which implies [see (2.8)]

$$\begin{aligned} \Phi ^s = 4 \pi n_s q_s^{-1} h_s, \end{aligned}$$

(3.11)

$s =1, \dots , n$.

Thus, any flux $\Phi ^s$ depends upon one integration constant $q_s \ne 0$, while the integrand form $F^s$ depends upon all constants: $q_1, \dots , q_n$.

We note that for $D =4$ and $g^2 = - \mathrm{d}t \otimes \mathrm{d}t + \mathrm{d}x \otimes d x$, $q_s$ is coinciding with the value of the x-component of the sth magnetic field on the axis of symmetry.

In the case of the Gibbons–Maeda dilatonic generalization of the Melvin solution, corresponding to $D = 4$, $n = l= 1$ and $\mathcal{G} = A_1$ [5], the flux from (3.11) ($s=1$) is in agreement with that considered in Ref. [26]. For Melvin’s case and some higher dimensional extensions (with $\mathcal{G} = A_1$) see also Ref. [14].

Due to (3.4) the ratios

$$\begin{aligned} \frac{q_i \Phi ^i}{q_j \Phi ^j} = \frac{n_i h_i}{n_j h_j} = \frac{n_i r_{j} }{n_j r_{i}} \end{aligned}$$

(3.12)

are fixed numbers depending upon the Cartan matrix $({A_{ij}})$ of a simple finite-dimensional Lie algebra $\mathcal{G}$.

Remark 2

The relation for flux integrals (3.11) is also valid when the matrix $(A_{ss'})$ is a Cartan matrix of a finite-dimensional semi-simple Lie algebra $\mathcal{G} = \mathcal{G}_1 \oplus \cdots \oplus \mathcal{G}_k$, where $\mathcal{G}_1, \dots , \mathcal{G}_k$ are simple Lie (sub)algebras. In this case the Cartan matrix $({A_{ij}})$ has a block-diagonal form, i.e. $({A_{ij}}) = \mathrm{diag} \left( \left( {A^{(1)}_{i_1 j_1}}\right) , \ldots , \left( {A^{(k)}_{i_k j_k}}\right) \right)$, where $\left( {A^{(a)}_{i_a j_a}}\right)$ is the Cartan matrix of the Lie algebra $\mathcal{G}_a$, $a = 1, \dots , k$. The set of polynomials in this case splits in a direct union of sets of polynomials corresponding to the Lie algebras $\mathcal{G}_1, \dots , \mathcal{G}_k$. Equations (3.4) and (3.12) are valid, when the indices i, j correspond to one ath block, $a = 1, \dots , k$. The quantities $q_i \Phi ^i$ and $q_j \Phi ^j$ corresponding to different blocks are independent. Equation (3.5) should be replaced by

$$\begin{aligned} K_{i_a} = \frac{1}{2} K^{(a)} r_{i_a}, \quad K^{(a)} > 0, \end{aligned}$$

(3.13)

for any index $i_a$ corresponding to the ath block; $a = 1, \dots , k$. The existence of dilatonic coupling vectors $\mathbf {\lambda }_s$ obeying (3.2) [(and (3.1)] just follows from the arguments of Remark 1, if we put all $K^{(a)} = K > 0$.

The manifold $M_{*} =(0, + \infty ) \times S^1$ is isomorphic to the manifold ${\mathbb R}^2_{*} = {\mathbb R}^2 \setminus \{ 0 \}$. The solution (2.3)–(2.5) may be understood (or rewritten by pull-backs) as defined on the manifold ${\mathbb R}^2_{*} \times M_2$, where the coordinates $\rho$, $\phi$ are understood as coordinates on ${\mathbb R}^2_{*}$. They are not globally defined. One should consider two charts with coordinates $\rho$, $\phi = \phi _1$ and $\rho$, $\phi = \phi _2$, where $\rho > 0$, $0< \phi _1 < 2 \pi$ and $- \pi< \phi _2 < \pi$. Here $\exp (i \phi _1 ) = \exp (i \phi _2)$. In both cases we have $x = \rho \cos \phi$ and $y = \rho \sin \phi$, where x, y are standard coordinates of ${\mathbb R}^2$. Using the identity $\rho \mathrm{d}\rho \wedge \mathrm{d}\phi = \mathrm{d}x \wedge \mathrm{d}y$ we get

$$\begin{aligned} F^s= q_s \prod _{s' = 1}^{n} (H_{s'}(x^2 + y^2))^{- A_{s s'}} \mathrm{d}x \wedge \mathrm{d}y, \end{aligned}$$

(3.14)

$s =1, \dots , n$. The 2-forms (3.14) are well defined on ${\mathbb R}^2$. Indeed, due to the conjecture from Ref. [3] any polynomial $H_{s}(z)$ is a smooth function on ${\mathbb R}= (- \infty , + \infty )$ which obeys $H_{s}(z) > 0$ for $z \in (- \varepsilon _s, + \infty )$, where $\varepsilon _s > 0$. This is valid due to the conjecture from Ref. [3] $H_{s}(z) > 0$ for $z > 0$ and $H_{s}(+0) = 1$. Thus, $\left( \prod _{s' = 1}^{n} \left( H_{s'}\left( x^2 + y^2\right) \right) ^{- A_{s s'}} \right)$ is a smooth function since it is a composition of two well-defined smooth functions $\left( \prod _{s' = 1}^{n} (H_{s'}(z))^{- A_{s s'}} \right)$ and $z = x^2 + y^2$.

Now we show that there exist 1-forms $A^s$ obeying $F^s = dA^s$ which are globally defined on ${\mathbb R}^2$. We start with the open submanifold ${\mathbb R}^2_{*}$. The 1-forms

$$\begin{aligned} A^s = \left( \int _{0}^{\rho } \mathrm{d}\bar{\rho } \bar{\rho } \mathcal{B}^s(\bar{\rho }^2) \right) \mathrm{d}\phi = \frac{1}{2} \left( \int _{0}^{\rho ^2} \mathrm{d}\bar{z} \mathcal{B}^s(\bar{z}) \right) \mathrm{d}\phi \end{aligned}$$

(3.15)

are well defined on ${\mathbb R}^2_{*}$ (here $\mathrm{d}\phi = (x^2 + y^2)^{-1} ( - y \mathrm{d}x + x \mathrm{d}y)$) and obey $F^s = dA^s$, $s = 1, \dots , n$. Using the master equation (2.6) we obtain

$$\begin{aligned} A^s= & {} \frac{q_s}{2 P_s} \left( \int _{0}^{\rho ^2} \mathrm{d}\bar{z} \frac{\mathrm{d}}{\mathrm{d}\bar{z}} \left( \frac{ \bar{z}}{H_s(\bar{z})} \frac{\mathrm{d}}{\mathrm{d}\bar{z}} H_s ( \bar{z}) \right) \right) \mathrm{d}\phi \nonumber \\= & {} \frac{2 h_s}{q_s} \frac{H^{'}_{s} (\rho ^2)}{H_s(\rho ^2)} \rho ^2 d \phi , \end{aligned}$$

(3.16)

$s = 1, \dots , n$. Here $H{'}_s = \frac{\mathrm{d}}{\mathrm{d}z} H_s$. Due to the relation $\rho ^2 \mathrm{d}\phi = - y \mathrm{d}x + x \mathrm{d}y$, we obtain

$$\begin{aligned} A^s = \frac{2 h_s}{q_s} \frac{H^{'}_{s} (x^2 + y^2)}{H_s(x^2 + y^2)} (- y \mathrm{d}x + x \mathrm{d}y), \end{aligned}$$

(3.17)

$s = 1, \dots , n$. The 1-forms (3.17) are well-defined smooth 1-forms on ${\mathbb R}^2$.

We note that in the case of the Gibbons–Maeda solution [5] corresponding to $D = 4$, $n = l= 1$ and $\mathcal{G} = A_1$ the gauge potential from (3.16) coincides (up to notations) with that considered in Ref. [7].

Now we verify our result (3.11) for flux integrals by using the relations for the 1-forms $A^s$. Let us consider a 2d oriented manifold (disk) $D_R = \{ (x,y): x^2 + y^2 \le R^2 \}$ with the boundary $\partial D_R = C_R = \{ (x,y): x^2 + y^2 = R^2 \}$. $C_R$ is a circle of radius R. It is an 1d oriented manifold with the orientation (inherited from that of $D_R$) obeying the relation $\int _{C_R} \mathrm{d}\phi = 2 \pi$. Using the Stokes–Cartan theorem we get

$$\begin{aligned} \int _{D_{R}} F^s= \int _{D_{R}} \mathrm{d} A^s= \int _{C_{R}} A^s = \frac{4 \pi h_s}{q_s} \frac{H^{'}_{s} (R^2)}{H_s(R^2)} R^2, \end{aligned}$$

(3.18)

$s = 1, \dots , n$. By using the asymptotic relation (3.8) we find

$$\begin{aligned} \lim _{R \rightarrow + \infty } \int _{D_{R}} F^s = \frac{4 \pi h_s n_s}{q_s}, \end{aligned}$$

(3.19)

$s = 1, \dots , n$, in agreement with (3.11).

Remark 3

We note (for completeness) that the metric and scalar fields for our solution with $w = +1$ and $l = n$ can be extended to the manifold ${\mathbb R}^2 \times M_2$. Indeed, in the coordinates x, y the metric (2.3) and scalar fields (2.4) read as follows:

$$\begin{aligned} g= & {} \Bigl (\prod _{s = 1}^{n} H_s^{2 h_s /(D-2)} \Bigr ) \biggl \{ \mathrm{d}x \otimes \mathrm{d}x + \mathrm{d}y \otimes \mathrm{d}y \nonumber \\&+ f (- y \mathrm{d}x + x \mathrm{d}y)^2 + g^2 \biggr \}, \end{aligned}$$

(3.20)

$$\begin{aligned} \varphi ^{a}= & {} \sum _{s = 1}^{n} h_s \lambda _{s}^{a} \ln H_s, \end{aligned}$$

(3.21)

$a =1, \dots , l$. Here $H_s = H_s(x^2 + y^2)$, $s = 1, \dots , n$, and $f = f(x^2 + y^2)$, where

$$\begin{aligned} f(z) = \left( \Bigl (\prod _{s = 1}^{n} (H_s(z))^{-2 h_s} \Bigr ) - 1 \right) z^{-1}, \end{aligned}$$

(3.22)

for $z \ne 0$ and $f(0)= \lim _{z \rightarrow 0} f(z)$ (the limit does exist). The function f(z) is smooth in the interval $(- \varepsilon , + \infty )$ for some $\varepsilon > 0$. Indeed, it is smooth in the interval $(0, + \infty )$ and holomorphic in the domain $\{z | 0< |z| < \varepsilon \}$ for a small enough $\varepsilon > 0$. Since the limit $\lim _{z \rightarrow 0} f(z)$ does exist the function f(z) is holomorphic in the disc $\{z | |z| < \varepsilon \}$ and hence it is smooth in the interval $(- \varepsilon , + \infty )$. This implies that the metric is smooth on the manifold ${\mathbb R}^2 \times M_2$. (See the text after Eq. (3.14).) The scalar fields are also smooth on ${\mathbb R}^2 \times M_2$.

4 Examples

Here we present fluxbrane polynomials corresponding to the Lie algebras $A_1$, $A_2$, $A_3$, $C_2$, $G_2$, $A_1 + A_1$ and related fluxes. Here as in [32] we use other parameters $p_s$ instead of $P_s$:

$$\begin{aligned} p_s = P_s/n_s, \end{aligned}$$

(4.1)

$s = 1, \ldots , n$.

$A_1$ -case. The simplest example occurs in the case of the Lie algebra $A_1 = sl(2)$. Here $n_1 = 1$. We get [3]

$$\begin{aligned} H_{1} = 1 + p_1 z \end{aligned}$$

(4.2)

and

$$\begin{aligned} \Phi ^1 = 4 \pi q_1^{-1} h_1, \end{aligned}$$

(4.3)

which is also valid for Melvin’s solution with $D = 4$ and $h_1 = 2$.

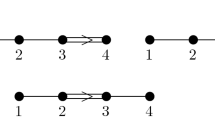
$A_2$ -case. For the Lie algebra $A_2 = sl(3)$ with the Cartan matrix

$$\begin{aligned} \left( A_{ss'}\right) = \left( \begin{array}{*{6}{c}} 2 &{}\quad -1\\ -1&{}\quad 2\\ \end{array} \right) \quad \end{aligned}$$

(4.4)

we have [3, 25, 32] $n_1 = n_2 =2$ and

$$\begin{aligned} H_{1}= & {} 1 + 2 p_1 z + p_1 p_2 z^{2}, \end{aligned}$$

(4.5)

$$\begin{aligned} H_{2}= & {} 1 + 2 p_2 z + p_1 p_2 z^{2}. \end{aligned}$$

(4.6)

We get in this case

$$\begin{aligned} (\Phi ^1, \Phi ^2) = 8 \pi h (q_1^{-1},q_2^{-1}), \end{aligned}$$

(4.7)

where $h_1 = h_2 = h$.

$A_3$ -case. The polynomials for the $A_3$-case read as follows [32, 33]:

$$\begin{aligned} H_{1}= & {} 1 + 3 p_1 z + 3 p_1 p_2 z^{2} + p_1 p_2 p_3 z^{3}, \end{aligned}$$

(4.8)

$$\begin{aligned} H_{2}= & {} 1 + 4 p_2 z + 3 \Bigl ( p_1 p_2 + p_2 p_3 \Bigr ) z^{2} \nonumber \\&+\,4 p_1 p_2 p_3 z^{3} + p_1 p_2^{2} p_3 z^{4}, \end{aligned}$$

(4.9)

$$\begin{aligned} H_{3}= & {} 1 + 3 p_3 z + 3 p_2 p_3 z^{2} + p_1 p_2 p_3 z^{3}. \end{aligned}$$

(4.10)

Here we have $(n_1, n_2, n_3) = (3,4,3)$ and

$$\begin{aligned} (\Phi ^1, \Phi ^2,\Phi ^3) = 4 \pi h (3q_1^{-1},4q_2^{-1}, 3q_3^{-1}) \end{aligned}$$

(4.11)

with $h_1 = h_2 = h_3 = h$.

$C_2$ -case. For the Lie algebra $C_2 = so(5)$ with the Cartan matrix

$$\begin{aligned} \left( A_{ss'}\right) = \left( \begin{array}{*{6}{c}} 2 &{} \quad -1\\ -2&{} \quad 2\\ \end{array} \right) \quad \end{aligned}$$

(4.12)

we get $n_1 = 3$ and $n_2 = 4$. For $C_2$-polynomials we obtain [25, 32]

$$\begin{aligned} H_1= & {} 1+ 3 p_1 z+ 3 p_1 p_2 z^2 + p_1^2 p_2 z^3, \end{aligned}$$

(4.13)

$$\begin{aligned} H_2= & {} 1+ 4 p_2 z+ 6 p_1 p_2 z^2 + 4 p_1^2 p_2 z^3 + p_1^2 p_2^2 z^4. \end{aligned}$$

(4.14)

In this case we find

$$\begin{aligned} (\Phi ^1, \Phi ^2) = 4 \pi (3 h_1 q_1^{-1}, 4 h_2 q_2^{-1}) \end{aligned}$$

(4.15)

where $h_1 = 2 h_2$.

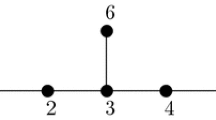
$G_2$ -case. For the Lie algebra $G_2$ with the Cartan matrix

$$\begin{aligned} \left( A_{ss'}\right) = \left( \begin{array}{*{6}{c}} 2 &{} \quad -1\\ -3&{} \quad 2\\ \end{array} \right) \quad \end{aligned}$$

(4.16)

we get $n_1 = 6$ and $n_2 = 10$. In this case the fluxbrane polynomials read [25, 32]

$$\begin{aligned} H_{1}= & {} 1+ 6 p_1 z+ 15 p_1 p_2 z^2 + 20 p_1^2 p_2 z^3 \nonumber \\&+\,15 p_1^3 p_2 z^4 + 6 p_1^3 p_2^2 z^5 + p_1^4 p_2^2 z^6 , \end{aligned}$$

(4.17)

$$\begin{aligned} H_2= & {} 1+ 10 p_2 z + 45 p_1 p_2 z^2 + 120 p_1^2 p_2 z^3\nonumber \\&+\, p_1^2 p_2( 135 p_1 + 75 p_2) z^4 \nonumber \\&+\,252 p_1^3 p_2^2 z^5 +\,p_1^3 p_2^2 \biggl (75 p_1 + 135 p_2 \biggr )z^6 \nonumber \\&+\,120 p_1^4 p_2^3 z^7\nonumber \\&+\,45 p_1^5 p_2^3 z^8 + 10 p_1^6 p_2^3 z^9 + p_1^{6} p_2^{4} z^{10}. \end{aligned}$$

(4.18)

We are led to the relations

$$\begin{aligned} (\Phi ^1, \Phi ^2) = 4 \pi (6 h_1 q_1^{-1}, 10 h_2 q_2^{-1}) \end{aligned}$$

(4.19)

where $h_1 = 3 h_2$.

$(A_1 + A_1)$ -case. For the semi-simple Lie algebra $A_1 + A_1$ we obtain $n_1 = n_2 = 1$,

$$\begin{aligned} H_{1} = 1 + p_1 z, \quad H_{2} = 1 + p_2 z, \end{aligned}$$

(4.20)

and

$$\begin{aligned} (\Phi ^1, \Phi ^2) = 4 \pi (q_1^{-1} h_1, q_2^{-1} h_2), \end{aligned}$$

(4.21)

where $h_1$ and $h_2$ are independent, as well as the quantities $q_1 \Phi ^1$ and $q_2 \Phi ^2$.

5 Conclusions

Here we have considered a multidimensional generalization of Melvin’s solution corresponding to a simple finite-dimensional Lie algebra $\mathcal{G}$. We have assumed that the solution is governed by a set of n fluxbrane polynomials $H_s(z)$, $s =1,\dots ,n$. These polynomials define special solutions to open Toda chain equations corresponding to the Lie algebra $\mathcal{G}$.

The polynomials $H_s(z)$ depend also upon parameters $q_s$, which are coinciding for $D =4$ (up to a sign) with the values of colored magnetic fields on the axis of symmetry.

We have calculated 2d flux integrals $\Phi ^s = \int F^s$, $s =1, \dots , n$. Any flux $\Phi ^s$ depends only upon one parameter $q_s$, while the integrand $F^s$ depends upon all parameters $q_1, \dots , q_n$. The relation for flux integrals (3.11) is also valid when the matrix $(A_{ss'})$ is a Cartan matrix of a finite-dimensional semi-simple Lie algebra $\mathcal G$.

Here we have considered examples of polynomials and fluxes for the Lie algebras $A_1$, $A_2$, $A_3$, $C_2$, $G_2$ and $A_1 + A_1$. The approach of this paper will be used for a calculation of certain flux integrals for forms $F^s$ of arbitrary ranks corresponding to certain fluxbrane solutions (of electric type by p-brane notation or magnetic type by fluxbrane classification¹) governed by fluxbrane polynomials [38].

An open problem is to find the fluxes for the solutions which are related to infinite-dimensional Lorentzian Kac–Moody algebras, e.g. hyperbolic ones [39, 40]. In this case one should deal with phantom scalar fields in the model (2.1) and non-polynomial solutions to Eqs. (2.6). Another possibility is to study the convergence of flux integrals for non-polynomial solutions for moduli functions corresponding to non-Cartan matrices $(A_{ss'})$ (e.g. for the model with two 2-forms from Ref. [41]).