A circulating particle current and energy currents in a circular tube with a temperature difference

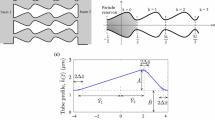
Abstract

Network effects in a circulating particle current and energy currents are discussed in the system consisting of hard disks confined in a circular tube with a temperature difference. Here, two parts of the walls of the tube, as the thermal walls, are kept at different temperatures, so the system has two areas connecting these different thermal walls as a simple network. The temperatures of the thermal walls are imposed by stochastic boundary conditions for particles to contact with these walls. In this circular system, we show that the temperature difference induces, not only energy currents, but also a steady circulating particle current, in the tube. This particle current is regarded as a “thermal convection” in the sense that it flows from the high-temperature region to the low-temperature one in one connecting area while it flows in the opposite direction of the temperature difference in the other connecting area. We also discuss transport properties of the energy currents via these two connecting areas, such as their oscillatory behaviors as functions of the positions of the thermal walls or the width of the tube.

Graphical abstract

Appendices

Appendix A: Transport and energy properties in high-density cases

In this appendix, we discuss particle currents, energy currents, and kinetic energies, especially in high-density cases, in the thermal network system introduced in this paper. In this appendix, we show results in the case of $\phi = 0$, so that the magnitude of the circulating particle current I (and its anomalous properties in high-density cases) would be enhanced rather than in the case of $\phi = \pi /4$ used mainly in the text of this paper.

Fig. 13

The particle currents I for $R_{1}=10^{-9}$ (red circles), $R_{1}=0.05$ (blue triangles), $R_{1}=0.1$ (green squares), $R_{1}=0.2$ (purple inverted triangles), and $R_{1}=0.3$ (light-blue diamonds), as functions of the particle density $\rho$ in the case of $k_{B}T_{2}=2$, $\varphi = \pi /4$, and $\phi = 0$

Our results in Fig. 3 show that the circulating particle current I by hard disks in a circular tube with a temperature difference decreases monotonically as the particle density decreases in a low-density region. Moreover, they also imply that the magnitude of the particle current I is reduced in a high-density region, probably because particles tend to be jammed inside the tube and are prevented to flow in such a region. (See the graph of I in $\rho > 10^{-2}$ in Fig. 3 as that showing this property.) However, the particle current I in a high-density region is not described by a monotonically decreasing function of the particle density $\rho$ in general. To discuss this point, we show Fig. 13 for the graphs of the particle currents I for $R_{1}=10^{-9}$ (red circles), $R_{1}=0.05$ (blue triangles), $R_{1}=0.1$ (green squares), $R_{1}=0.2$ (purple inverted triangles), and $R_{1}=0.3$ (light-blue diamonds), as functions of the particle density $\rho$ in the case of $k_{B}T_{2}=2$, $\varphi = \pi /4$, and $\phi = 0$. This figure suggests that in the density region of $\rho > 0.2$ the graphs of I have almost flat regions as functions of $\rho$ in the cases of $R_{1}=0.05$ and 0.3. It is also shown that the particle currents I are even increasing functions of $\rho$ partly in the cases of $R_{1}=10^{-9}$ around $\rho \approx 0.5$, and $R_{1}=0.1, 0.2$ around $\rho \approx 0.75$ in very-high-density cases.

Fig. 14

The particle currents I for $\rho =0.6$ (red circles), $\rho = 0.5$ (blue triangles), $\rho = 0.4$ (green squares), and $\rho = 0.3$ (purple inverted triangles), as functions of the radius $R_{1}$ in the case of $k_{B}T_{2}=2$, $\varphi = \pi /4$, and $\phi = 0$

To discuss another aspect of the circulating particle current I in high-density cases, we show Fig. 14 for the currents I for $\rho =0.6$ (red circles), $\rho = 0.5$ (blue triangles), $\rho = 0.4$ (green squares), and $\rho = 0.3$ (purple inverted triangles), as functions of the radius $R_{1}$ in the case of $k_{B}T_{2}=2$, $\varphi = \pi /4$, and $\phi = 0$. This figure shows oscillatory behaviors of the current I as functions of the radius $R_{1}$, whose oscillatory amplitudes become bigger in higher densities.

Fig. 15

The total energy currents $J_{A}+J_{B}$ for $R_{1}=10^{-9}$ (red circles), $R_{1}=0.05$ (blue triangles), $R_{1}=0.1$ (green squares), $R_{1}=0.2$ (purple inverted triangles), and $R_{1}=0.3$ (light-blue diamonds), as functions of the particle density $\rho$, as linear–log plots, in the case of $k_{B}T_{2}=2$, $\varphi = \pi /4$, and $\phi = 0$

Figure 15 is the graphs of the total energy currents $J_{A}+J_{B}$ from the thermal wall $\mathcal {W}_{2}$ to the thermal wall $\mathcal {W}_{1}$, for $R_{1}=10^{-9}$ (red circles), $R_{1}=0.05$ (blue triangles), $R_{1}=0.1$ (green squares), $R_{1}=0.2$ (purple inverted triangles), and $R_{1}=0.3$ (light-blue diamonds), as functions of the particle density $\rho$, as linear–log plots, in the case of $k_{B}T_{2}=2$, $\varphi = \pi /4$, and $\phi = 0$. It shows that the total energy currents $J_{A}+J_{B}$ are rapidly increasing functions of $\rho$, more rapid than their exponential increases, except in their extremely-high-density cases. The current $J_{A}+J_{B}$ increases as a function of $R_{1}$ in majority of cases, although it for $R_{1}=0.3$ is not larger than that for $R_{1}=0.2$ in high-density cases.

Fig. 16

The total energy currents $J_{A}+J_{B}$ for $\rho =0.6$ (red circles), $\rho = 0.5$ (blue triangles) $\rho = 0.4$ (green squares), and $\rho = 0.3$ (purple inverted triangles), as functions of the radius $R_{1}$ in the case of $k_{B}T_{2}=2$, $\varphi = \pi /4$, and $\phi = 0$

Figure 16 is the graphs of the total energy currents $J_{A}+J_{B}$ for $\rho =0.6$ (red circles), $\rho = 0.5$ (blue triangles), $\rho = 0.4$ (green squares), and $\rho = 0.3$ (purple inverted triangles), as functions of the radius $R_{1}$ in the case of $k_{B}T_{2}=2$, $\varphi = \pi /4$, and $\phi = 0$. It shows that in high-density cases the current $J_{A}+J_{B}$ shows oscillatory behaviors as functions of the radius $R_{1}$, and those oscillatory amplitudes becomes bigger in higher densities. This implies that the total heat current from a high-temperature wall to a low-temperature one could be manipulated by not only the particle density but also modifying the system shape with the inner radius $R_{1}$ in this thermal network system.

Fig. 17

The average kinetic energies $K_{tot}$ per disk for $R_{1}=10^{-9}$ (red circles), $R_{1}=0.05$ (blue triangles), $R_{1}=0.1$ (green squares), $R_{1}=0.2$ (purple inverted triangles), and $R_{1}=0.3$ (light-blue diamonds), as functions of the particle density $\rho$ in the case of $k_{B}T_{2}=2$, $\varphi = \pi /4$, and $\phi = 0$

In this paper, we have discussed mainly transport properties of a particle current and energy currents as nonequilibrium effects caused by a temperature difference. On the other hand, our model has many other quantities which are not described as currents but show important nonequilibrium effects. For such an example, we show Fig. 17 for the graphs of the time-average total kinetic energies $K_{tot}$ per disk for $R_{1}=10^{-9}$ (red circles), $R_{1}=0.05$ (blue triangles), $R_{1}=0.1$ (green squares), $R_{1}=0.2$ (purple inverted triangles), and $R_{1}=0.3$ (light-blue diamonds), as functions of the particle density $\rho$ in the case of $k_{B}T_{2}=2$, $\varphi = \pi /4$, and $\phi = 0$. This figure shows that the average total kinetic energy $K_{tot}$ per disk in our nonequilibrium model depends on the particle density $\rho$. Moreover, this kinetic energy also depends on system geometries such as the radius $R_{1}$, as also shown in Fig. 17. These properties of $K_{tot}$ are very contrastive to the ones in equilibrium states in which velocities of particles are distributed by the Maxwell distribution and the average kinetic energy is independent of the potential energy (so of the particle density $\rho$) and system geometries at a constant temperature. It may also be noted that the average total kinetic energy $K_{tot}$ per disk satisfies the inequality $1=k_{B}T_{1} \le K_{tot} \le k_{B}T_{2}=2$ in the data used for this figure.

Fig. 18

The average kinetic energies $K_{tot}$ per disk for $\rho =0.6$ (red circles), $\rho = 0.5$ (blue triangles) $\rho = 0.4$ (green squares), and $\rho = 0.3$ (purple inverted triangles), as functions of the radius $R_{1}$ in the case of $k_{B}T_{2}=2$, $\varphi = \pi /4$, and $\phi = 0$

Figure 18 is the graphs of the average kinetic energies $K_{tot}$ per disk for $\rho =0.6$ (red circles), $\rho = 0.5$ (blue triangles) $\rho = 0.4$ (green squares), and $\rho = 0.3$ (purple inverted triangles), as functions of the radius $R_{1}$ in the case of $k_{B}T_{2}=2$, $\varphi = \pi /4$, and $\phi = 0$. It also shows oscillatory behaviors of $K_{tot}$ with bigger oscillatory amplitudes for larger particle density, like in the particle currents I shown in Fig. 14 and the energy current $J_{A}+J_{B}$ shown in Fig. 16.

Fig. 19

The quantities 6I (red circles), $(J_{A}+J_{B})/34$ (blue triangles), $35K_{tot}-49$ (green squares) and $(R_{2}-R_{1})/(2r)$ (black filled circles) as functions of the radius $R_{1}$ in the case of $k_{B}T_{2}=2$, $\varphi = \pi /4$, $\phi = 0$, and $\rho =0.6$

As one of the important features of the particle current, the total energy current, and the kinetic energy in high-density cases, we discuss further their oscillatory behaviors as functions of the radius $R_{1}$. To discuss their properties and origins, we show Fig. 19 for the quantities 6I (red circles), $(J_{A}+J_{B})/34$ (blue triangles), $35K_{tot}-49$ (green squares) and $(R_{2}-R_{1})/(2r)$ (black filled circles) with the particle currents I, the total energy current $J_{A}+J_{B}$, the average kinetic energy $K_{tot}$, the width $R_{2}-R_{1}$ of the tube, and the radius r of disks, as functions of the radius $R_{1}$ in the case of $k_{B}T_{2}=2$, $\varphi = \pi /4$, $\phi = 0$, and $\rho =0.6$. This figure shows that the values of $R_{1}$ at the local maxima (minima) of $K_{tot}$ correspond to the one at the local minima (maxima) of I and $J_{A}+J_{B}$, except in the cases of the radius $R_{1}$ near $R_{2} = 0.5$. Furthermore, Fig. 19 also indicates that as the radius $R_{1}$ of the inner circle increases, the local maxima of I and $J_{A}+J_{B}$ (corresponding to the local minima of $K_{tot}$) appear just after the value of $(R_{2}-R_{1})/(2r)$ crosses an integer value. This result suggests that oscillatory behaviors of I, $J_{A}+J_{B}$, and $K_{tot}$ would be related to the number of disks passing simultaneously through a cross-section of the circular tube.

Appendix B: Local temperatures and particle-number density

The nonequilibrium thermodynamics describes thermodynamic transports based on local thermodynamic quantities, such as a local temperature, a particle density, a momentum density, and an energy density, near an equilibrium state [1,2,3]. From this point of view, in this appendix, we discuss briefly local profiles of temperature and particle-number density in the circular-tube model introduced in this paper.

Fig. 20

The kinetic temperatures ${\mathcal {T}}_{\theta }$ in the rotational direction for $k_{B}(T_{2}-T_{1})=1$ (red solid line), $k_{B}(T_{2}-T_{1})=0.4$ (blue dotted line), and $k_{B}(T_{2}-T_{1})=0$ (green broken line), and the kinetic temperatures ${\mathcal {T}}_{r}$ in the radial direction for $k_{B}(T_{2}-T_{1})=1$ (shin red solid line), $k_{B}(T_{2}-T_{1})=0.4$ (shin blue dotted line), and $k_{B}(T_{2}-T_{1})=0$ (shin green broken line), as functions of $\theta /(2\pi )$ in the case of $\rho = 0.01$, $R_{1}=0.4$, and $\varphi = \phi = \pi /4$. Here, the graphs of ${\mathcal {T}}_{\theta }$ and ${\mathcal {T}}_{r}$ in the equilibrium case of $k_{B}(T_{2}-T_{1})=0$ are almost indistinguishable. The black vertical lines $\theta /(2\pi ) = 0$, $\theta /(2\pi ) = 1/8$, $\theta /(2\pi ) = 1/4$, and $\theta /(2\pi ) = 3/8$ correspond to the ends of the thermal walls

Fig. 21

The particle-number densities $\sigma _{\theta }$ for $k_{B}(T_{2}-T_{1})=1$ (red solid line), $k_{B}(T_{2}-T_{1})=0.4$ (blue dotted line), and $k_{B}(T_{2}-T_{1})=0$ (green broken line) as functions of $\theta /(2\pi )$ in the case of $\rho = 0.01$, $R_{1}=0.4$, and $\varphi = \phi = \pi /4$. The black vertical lines $\theta /(2\pi ) = 0$, $\theta /(2\pi ) = 1/8$, $\theta /(2\pi ) = 1/4$, and $\theta /(2\pi ) = 3/8$ correspond to the ends of the thermal walls

Since we have discussed currents in the rotational direction in this model and spatial changes of local quantities in this direction would be important to discuss such currents, we introduce the small region $\mathcal {R}_{k}$ with the rotational angle $\theta \in [2(k-1)\pi /\mathcal {N}_{R}, 2k\pi /\mathcal {N}_{R})$ inside the tube ($k=1,2,\cdots ,\mathcal {N}_{R}$), and discuss position-dependences of these quantities with respect to the rotational direction by calculating these quantities in the regions $\mathcal {R}_{k}$, $k=1,2,\cdots ,\mathcal {N}_{R}$. Here, as the number $\mathcal {N}_{R}$ of the regions $\mathcal {R}_{k}$, $k=1,2,\cdots ,\mathcal {N}_{R}$, we use $\mathcal {N}_{R} = 2^{7}$ for the numerical results (shown in Figs. 20 and 21) in this paper.

As local nonequilibrium temperatures in the region $\mathcal {R}_{k}$, we consider the kinetic temperature ${\mathcal {T}}_{\theta }$ in the rotational direction and the kinetic temperature ${\mathcal {T}}_{r}$ in the radial direction. Here, we define the kinetic temperature ${\mathcal {T}}_{\theta }$ (${\mathcal {T}}_{r}$) so that the quantity $m k_{B}{\mathcal {T}}_{\theta }$ ($m k_{B}{\mathcal {T}}_{r}$) is given by the time-average of the variance of the rotating component (the radial component) of the momentum of the particles per disk in each region of $\mathcal {R}_{k}$, $k=1,2,\cdots ,\mathcal {N}_{R}$. (Here and hereafter, to specify particles in the region $\mathcal {R}_{k}$, each particle, namely disk, is regarded to be located at the center of the disk.) These kinetic temperatures coincide the equilibrium temperature in equilibrium states. In Fig. 20, we show the graphs of the kinetic temperatures ${\mathcal {T}}_{\theta }$ in the rotational direction for $k_{B}(T_{2}-T_{1})=1$ (red solid line), $k_{B}(T_{2}-T_{1})=0.4$ (blue dotted line), and $k_{B}(T_{2}-T_{1})=0$ (green broken line), and the kinetic temperatures ${\mathcal {T}}_{r}$ in the radial direction for $k_{B}(T_{2}-T_{1})=1$ (shin red solid line), $k_{B}(T_{2}-T_{1})=0.4$ (shin blue dotted line), and $k_{B}(T_{2}-T_{1})=0$ (shin green broken line), as functions of $\theta /(2\pi )$ in the case of $\rho = 0.01$, $R_{1}=0.4$, and $\varphi = \phi = \pi /4$ in our model. Here, the black vertical lines $\theta /(2\pi ) = 0$, $\theta /(2\pi ) = 1/8$, $\theta /(2\pi ) = 1/4$, and $\theta /(2\pi ) = 3/8$ correspond to the ends of the thermal walls. This figure shows that in the equilibrium case of $k_{B}(T_{2}-T_{1})=0$ the kinetic temperatures ${\mathcal {T}}_{\theta }$, ${\mathcal {T}}_{r}$, and the temperature $T_{1}=T_{2}$ on the thermal walls are equal with each other, as they should be. On the other hand, as shown in this figure, in the nonequilibrium cases of $k_{B}(T_{2}-T_{1})=0.4$ and 1, the gradients of the kinetic temperatures ${\mathcal {T}}_{\theta }$ and ${\mathcal {T}}_{r}$ appears as nonlinear functions of $\theta$ in each of the connecting areas $\mathcal {C}_{A}$ and $\mathcal {C}_{B}$. Furthermore, in these nonequilibrium cases, these kinetic temperatures ${\mathcal {T}}_{\theta }$ and ${\mathcal {T}}_{r}$ are different locally with each other, suggesting that the local equilibrium assumption is violated. This kind of direction dependence of the kinetic temperature appears in various nonequilibrium phenomena [31, 49].

As another example of local quantities, we consider the particle-number density $\sigma _{\theta }$, which is defined by the time-average of the number of particles divided by the square measure of $\mathcal {R}_{k}$ in the region $\mathcal {R}_{k}$ for $k=1,2,\cdots ,\mathcal {N}_{R}$. In Fig. 21, we show the graphs of the particle-number densities $\sigma _{\theta }$ for $k_{B}(T_{2}-T_{1})=1$ (red solid line), $k_{B}(T_{2}-T_{1})=0.4$ (blue dotted line), and $k_{B}(T_{2}-T_{1})=0$ (green broken line) as functions of $\theta /(2\pi )$ in the case of $\rho = 0.01$, $R_{1}=0.4$, and $\varphi = \phi = \pi /4$. Here, the black vertical lines $\theta /(2\pi ) = 0$, $\theta /(2\pi ) = 1/8$, $\theta /(2\pi ) = 1/4$, and $\theta /(2\pi ) = 3/8$ correspond to the ends of the thermal walls. In the equilibrium state of $k_{B}(T_{2}-T_{1})=0$, this particle-number density $\sigma _{\theta }$ is constant as a function of $\theta$. On the ther hand, in the nonequilibrium state of $k_{B}(T_{2}-T_{1})=0.4$ and 1, the spatial gradients of this density occur, and the particle-number density $\sigma _{\theta }$ appears as a nonlinear function of $\theta$ in each of the connecting areas $\mathcal {C}_{A}$ and $\mathcal {C}_{B}$. Roughly speaking, the particle-number density $\sigma _{\theta }$ is high in a region with a low kinetic temperature. On the other hand, the relation among the particle-number density $\sigma _{\theta }$, one of the local temperatures kinetic temperatures ${\mathcal {T}}_{\theta }$ and ${\mathcal {T}}_{r}$, and a local pressure acting on a part of the wall of the tube shows a deviation from van der Waal’s equation of state for two-dimensional imperfect gases in each region of $\mathcal {R}_{k}$, $k=1,2,\cdots ,\mathcal {N}_{R}$ in the nonequilibrium cases [44].

Now, we consider briefly whether the circulating particle current I in the circular-tube model introduced in this paper could be described by a linear combination of gradients of a local particle-number density and a local temperature discussed in Figs. 20 and 21, suggested by the linear nonequilibrium thermodynamics. It is obvious that the particle current I is not proportional to the gradient of the particle-number density $\sigma _{\theta }$, differently from the phenomena by Fick’s law only, because the gradient of the particle-number density $\sigma _{\theta }$ is not constant as a function of $\theta$ but the particle current I is independent of $\theta$ in this model. Similarly, the particle current I is not proportional to the gradient of the kinetic temperature ${\mathcal {T}}_{\theta }$, differently from the phenomena by the thermal diffusion only.

Furthermore, to introduce an effect not only of Fick’s law but also of the thermal diffusion, one might consider a linear combination ${\mathcal {I}} \equiv C_{\sigma } d \sigma _{\theta }/d\theta + C_{T} d {\mathcal {T}}_{\theta }/d\theta$ with constants $C_{\sigma }$ and $C_{T}$. (Note that a length of the tube is proportional approximately to the corresponding width of the angle $\theta$, and the gradients of $\sigma _{\theta }$ and ${\mathcal {T}}_{\theta }$ are approximately proportional to $d\sigma _{\theta }/d\theta$ and $d{\mathcal {T}}_{\theta }/d\theta$, respectively, in the rotational direction. Besides, the angle-derivative of ${\mathcal {T}}_{\theta }$ and $\sigma _{\theta }$ have to be introduced under the large number limit $\mathcal {N}_{R} \rightarrow \infty$ for the number $\mathcal {N}_{R}$ of the local regions $\mathcal {R}_{k}$, $k=1,2,\cdots ,\mathcal {N}_{R}$ where the quantities ${\mathcal {T}}_{\theta }$ and $\sigma _{\theta }$ are defined.) Similarly to the argument for Eq. (8), the integration of this quantity ${\mathcal {I}}$ over $[0, 2\pi )$ with respect to $\theta$ should be zero because of the periodic boundary conditions of $\sigma _{\theta }$ and ${\mathcal {T}}_{\theta }$: $\sigma _{\theta }|_{\theta = 0} = \sigma _{\theta }|_{\theta = 2\pi }$ and ${\mathcal {T}}_{\theta }|_{\theta = 0} = {\mathcal {T}}_{\theta }|_{\theta = 2\pi }$. On the other hand, in the case of $T_{1}\ne T_{2}$ the integral of the circulating particle current I over $[0, 2\pi )$ with respect to $\theta$ takes the non-zero value $2\pi I$ because I is constant as a function of $\theta$. Therefore, the non-zero circulating particle current I should not be equal to the quantity ${\mathcal {I}}$ represented as a linear combination of the gradients of the particle density $\sigma _{\theta }$ and the temperature ${\mathcal {T}}_{\theta }$.