Effects of weak magnetic field and finite chemical potential on the transport of charge and heat in hot QCD matter

Abstract

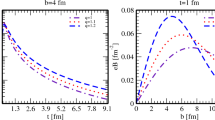
We have studied the effects of weak magnetic field and finite chemical potential on the transport of charge and heat in hot QCD matter by estimating their respective response functions, viz. the electrical conductivity ($\sigma _{\textrm{el}}$), the Hall conductivity ($\sigma _{\textrm{H}}$), the thermal conductivity ($\kappa _0$) and the Hall-type thermal conductivity ($\kappa _1$). The expressions of charge and heat transport coefficients are obtained by solving the relativistic Boltzmann transport equation in the relaxation time approximation at weak magnetic field and finite chemical potential. The interactions among partons are incorporated through their thermal masses. We have observed that $\sigma _{{\textrm{el}}}$ and $\kappa _0$ decrease and $\sigma _{{\textrm{H}}}$ and $\kappa _1$ increase with the magnetic field in the weak magnetic field regime. On the other hand, the presence of a finite chemical potential increases these transport coefficients. The effects of weak magnetic field and finite chemical potential on aforesaid transport coefficients are found to be more conspicuous at low temperatures, whereas at high temperatures, they have only a mild dependence on magnetic field and chemical potential. We have found that the presence of finite chemical potential further extends the lifetime of the magnetic field. Furthermore, we have explored the effects of weak magnetic field and finite chemical potential on the Knudsen number, the elliptic flow coefficient and the Wiedemann–Franz law.

Appendices

Appendices

Derivation of equation (31)

By substituting the following partial derivatives in eq. (30),

$$\begin{aligned} v_x\frac{\partial f_f}{\partial p_0}= & {} -\beta v_xf_f^0-qE\tau _f\beta f_f^0v_x^2\left( \frac{1}{\omega _f}+\beta \right) \nonumber \\{} & {} \nonumber -\beta f_f^0\Gamma _xv_x^2\left( \frac{1}{\omega _f}+\beta \right) \nonumber \\{} & {} -\beta f_f^0\Gamma _yv_xv_y\left( \frac{1}{\omega _f}+\beta \right) \nonumber \\{} & {} -\beta f_f^0\Gamma _zv_xv_z\left( \frac{1}{\omega _f}+\beta \right) , \end{aligned}$$

(A.59)

$$\begin{aligned} \nonumber v_x\frac{\partial f_f}{\partial p_y}= & {} -\beta v_xv_yf_f^0-qE\tau _f\beta f_f^0v_x^2v_y\left( \frac{1}{\omega _f}+\beta \right) \nonumber \\{} & {} \nonumber -\beta f_f^0\Gamma _xv_x^2v_y\left( \frac{1}{\omega _f}+\beta \right) \nonumber \\{} & {} -\beta f_f^0\Gamma _yv_xv_y^2\left( \frac{1}{\omega _f}+\beta \right) +\frac{v_x\Gamma _y\beta f_f^0}{\omega _f}\nonumber \\{} & {} -\beta f_f^0\Gamma _zv_xv_yv_z\left( \frac{1}{\omega _f}+\beta \right) , \end{aligned}$$

(A.60)

$$\begin{aligned} v_y\frac{\partial f_f}{\partial p_x}= & {} -\beta v_yv_xf_f^0-qE\tau _f\beta f_f^0v_yv_x^2\left( \frac{1}{\omega _f}+\beta \right) \nonumber \\{} & {} \nonumber +\frac{qE\tau _f\beta f_f^0v_y}{\omega _f} -\beta f_f^0\Gamma _xv_yv_x^2\left( \frac{1}{\omega _f}+\beta \right) \nonumber \\{} & {} +\frac{\Gamma _x\beta f_f^0v_y}{\omega _f} -\beta f_f^0\Gamma _yv_y^2v_x\left( \frac{1}{\omega _f}+\beta \right) \nonumber \\{} & {} -\beta f_f^0\Gamma _zv_yv_zv_x\left( \frac{1}{\omega _f}+\beta \right) , \end{aligned}$$

(A.61)

and then dropping higher order velocity terms, we get

$$\begin{aligned}{} & {} -qE\tau _fv_x+\left( \Gamma _xv_x+\Gamma _yv_y+\Gamma _zv_z\right) \nonumber \\{} & {} \quad -\frac{qB\tau _f}{\omega _f}\left( v_x\Gamma _y-v_y\Gamma _x\right) +\frac{\tau _f^2qBqEv_y}{\omega _f}=0.\end{aligned}$$

(A.62)

Comparing the coefficients of $v_z$ on both sides of Eq. (A.62), we get $\Gamma _z=0$. Then, we have

$$\begin{aligned}{} & {} -qEv_x+\frac{\Gamma _x}{\tau _f}v_x-\omega _c\Gamma _yv_x+\frac{\Gamma _y}{\tau _f}v_y+\omega _c\Gamma _xv_y\nonumber \\{} & {} \quad +\tau _f\omega _cqEv_y=0,\end{aligned}$$

(A.63)

where the cyclotron frequency, $\omega _c=\frac{qB}{\omega _f}$. Equating coefficients of $v_x$ and $v_y$ on both sides of Eq. (A.63), we get

$$\begin{aligned}{} & {} \frac{\Gamma _x}{\tau _f}-\omega _c\Gamma _y-qE=0, \end{aligned}$$

(A.64)

$$\begin{aligned}{} & {} \frac{\Gamma _y}{\tau _f}+\omega _c\Gamma _x+\tau _f\omega _cqE=0.\end{aligned}$$

(A.65)

After solving Eqs. (A.64) and (A.65), we obtain

$$\begin{aligned}{} & {} \Gamma _x=\frac{qE\tau _f\left( 1-\omega _c^2\tau _f^2\right) }{1+\omega _c^2\tau _f^2}, \end{aligned}$$

(A.66)

$$\begin{aligned}{} & {} \Gamma _y=-\frac{2qE\omega _c\tau _f^2}{1+\omega _c^2\tau _f^2}.\end{aligned}$$

(A.67)

Now, ansatz (29) can be written as

$$\begin{aligned} f_f{} & {} =f_f^0-qE\tau _f\frac{\partial f_f^0}{\partial p_x}-qE\tau _f\left( \frac{1-\omega _c^2\tau _f^2}{1+\omega _c^2\tau _f^2}\right) \frac{\partial f_f^0}{\partial p_x}\nonumber \\{} & {} \quad +2qE\left( \frac{\omega _c\tau _f^2}{1+\omega _c^2\tau _f^2}\right) \frac{\partial f_f^0}{\partial p_y}.\end{aligned}$$

(A.68)

By using $\frac{\partial f_f^0}{\partial p_x}=v_x\frac{\partial f_f^0}{\partial \omega _f}=-v_x\beta f_f^0\left( 1-f_f^0\right)$ and $\frac{\partial f_f^0}{\partial p_y}=v_y\frac{\partial f_f^0}{\partial \omega _f}=-v_y\beta f_f^0\left( 1-f_f^0\right)$, eq. (A.68) gets simplified into

$$\begin{aligned} \nonumber f_f= & {} f_f^0+qE\tau _fv_x\beta f_f^0\left( 1-f_f^0\right) \\{} & {} \nonumber +qE\tau _fv_x\beta \left( \frac{1-\omega _c^2\tau _f^2}{1+\omega _c^2\tau _f^2}\right) f_f^0\left( 1-f_f^0\right) \\{} & {} -2qEv_y\beta \left( \frac{\omega _c\tau _f^2}{1+\omega _c^2\tau _f^2}\right) f_f^0\left( 1-f_f^0\right) .\end{aligned}$$

(A.69)

This leads to the determination of $\delta f_f$ as

$$\begin{aligned} \delta f_f{} & {} =2qEv_x\beta \left( \frac{\tau _f}{1+\omega _c^2\tau _f^2}\right) f_f^0\left( 1-f_f^0\right) \nonumber \\{} & {} \quad -2qEv_y\beta \left( \frac{\omega _c\tau _f^2}{1+\omega _c^2\tau _f^2}\right) f_f^0\left( 1-f_f^0\right) .\end{aligned}$$

(A.70)

Derivation of Eq. (45)

Substituting the value of L (44) in eq. (43) and simplifying, we have

$$\begin{aligned}{} & {} \nonumber \frac{\left( \omega _f-h_f\right) }{T}v_x\left( \partial ^xT-\frac{T}{nh_f}\partial ^xP\right) +\frac{\Gamma _xv_x}{\tau _f}-\omega _c\Gamma _yv_x\\{} & {} \quad \nonumber -qEv_x+\frac{\left( \omega _f-h_f\right) }{T}v_y\left( \partial ^yT-\frac{T}{nh_f}\partial ^yP\right) +\frac{\Gamma _yv_y}{\tau _f}\\{} & {} \quad \nonumber +\omega _c\Gamma _xv_y+\tau _f\omega _cqEv_y +p_0\frac{DT}{T}-\frac{p^\mu p^\alpha }{p_0}\nabla _\mu u_\alpha \\{} & {} \quad +TD\left( \frac{\mu _f}{T}\right) =0. \end{aligned}$$

(B.71)

Equating the coefficients of $v_x$ and $v_y$ on both sides of the above equation, we obtain

$$\begin{aligned}{} & {} \frac{\left( \omega _f-h_f\right) }{T}\left( \partial ^xT-\frac{T}{nh_f}\partial ^xP\right) +\frac{\Gamma _x}{\tau _f}\nonumber \\{} & {} \quad -\omega _c\Gamma _y-qE=0, \end{aligned}$$

(B.72)

$$\begin{aligned}{} & {} \frac{\left( \omega _f-h_f\right) }{T}\left( \partial ^yT-\frac{T}{nh_f}\partial ^yP\right) +\frac{\Gamma _y}{\tau _f}\nonumber \\{} & {} \quad +\omega _c\Gamma _x+\tau _f\omega _cqE=0. \end{aligned}$$

(B.73)

By solving Eqs. (B.72) and (B.73), $\Gamma _x$ and $\Gamma _y$ are respectively determined as

$$\begin{aligned} \nonumber \Gamma _x= & {} \frac{qE\tau _f\left( 1-\omega _c^2\tau _f^2\right) }{1+\omega _c^2\tau _f^2}-\frac{\tau _f\left( \omega _f-h_f\right) }{T\left( 1+\omega _c^2\tau _f^2\right) }\left( \partial ^xT-\frac{T}{nh_f}\partial ^xP\right) \\ {}{} & {} -\frac{\omega _c\tau _f^2\left( \omega _f-h_f\right) }{T\left( 1+\omega _c^2\tau _f^2\right) }\left( \partial ^yT-\frac{T}{nh_f}\partial ^yP\right) , \end{aligned}$$

(B.74)

$$\begin{aligned} \nonumber \Gamma _y= & {} -\frac{2\omega _c\tau _f^2qE}{1+\omega _c^2\tau _f^2}-\frac{\tau _f\left( \omega _f-h_f\right) }{T\left( 1+\omega _c^2\tau _f^2\right) }\left( \partial ^yT-\frac{T}{nh_f}\partial ^yP\right) \\ {}{} & {} +\frac{\omega _c\tau _f^2\left( \omega _f-h_f\right) }{T\left( 1+\omega _c^2\tau _f^2\right) }\left( \partial ^xT-\frac{T}{nh_f}\partial ^xP\right) . \end{aligned}$$

(B.75)

Using the values of $\Gamma _x$ and $\Gamma _y$ in ansatz (29) and then simplifying, we get the infinitesimal change of the quark distribution function as

$$\begin{aligned} \nonumber \delta f_f= & {} \frac{2qE\tau _fv_x\beta f_f^0\left( 1-f_f^0\right) }{1+\omega _c^2\tau _f^2}-\frac{2qE\omega _c\tau _f^2v_y\beta f_f^0\left( 1-f_f^0\right) }{1+\omega _c^2\tau _f^2}\\{} & {} \nonumber -\beta ^2 f_f^0\left( 1-f_f^0\right) \frac{\tau _f(\omega _f-h_f)}{\left( 1+\omega _c^2\tau _f^2\right) } \\ {}{} & {} \nonumber \times \left[ v_x\left( \partial ^xT-\frac{T}{nh_f}\partial ^xP\right) +v_y\left( \partial ^yT-\frac{T}{nh_f}\partial ^yP\right) \right] \\{} & {} \nonumber -\beta ^2 f_f^0\left( 1-f_f^0\right) \\{} & {} \nonumber \times \frac{\omega _c\tau _f^2(\omega _f-h_f)}{\left( 1+\omega _c^2\tau _f^2\right) }\left[ v_x\left( \partial ^yT-\frac{T}{nh_f}\partial ^yP\right) \right. \\{} & {} \left. -v_y\left( \partial ^xT-\frac{T}{nh_f}\partial ^xP\right) \right] .\end{aligned}$$

(B.76)