The CHSH Bell Inequality: A Critical Look at Its Mathematics and Some Consequences for Physical Chemistry

Abstract

In the paper it is demonstrated that Bell’s theorem is an unprovable theorem. The unprovable characteristic has, on the chemical side, repercussions for e.g. spin chemistry and the related magneto-reception studies. We claim that the unprovability of this basic mathematics cannot be ignored by the physics and chemical research community. The demonstrated mathematical multivaluedness could be an overlooked aspect of nature.

Appendices

APPENDIX A

The claim that K_n goes to zero for large n was presented to the authors in a previous review process. The claim of the unknown referee was that the integral K_n had a closed form solution, as presented below. The reader can in this appendix verify the ”CHSH is true ”side of the claim. In the main text we have demonstrated K_n ≈ 1. Here it is rejected. We start again with

$$\begin{gathered} \hfill {{K}_{n}} = \frac{4}{{\pi {{n}^{2}}}}\int\limits_{x = 1/n}^{x = 1/4} {\frac{{dx}}{{{{x}^{4}} - 2\frac{{{{x}^{2}}}}{{{{n}^{2}}}} + \frac{{{{n}^{2}} + 1}}{{{{n}^{4}}}}}}} \\ \hfill = \frac{4}{\pi }\int\limits_{x = 1/n}^{x = 1/4} {\frac{{dx}}{{{{n}^{2}}{{x}^{4}} - 2{{x}^{2}} + 1 + \frac{1}{{{{n}^{2}}}}}}.} \\ \end{gathered} $$

(A.57)

The closed form is based on the subsequent function F_n

$${{F}_{n}}(x) = 2\frac{{n\sqrt 2 }}{{\pi \left( {{{n}^{2}} + \sqrt {1 + {{n}^{2}}} + 1} \right)}}\left( {{{G}_{n}}(x) + {{H}_{n}}(x)} \right),$$

(A.58)

with

$${{G}_{n}}(x) = n{\kern 1pt} \log \left( {\frac{{{{U}_{{n, + }}}(x) + \sqrt {1 + {{n}^{2}}} + 1}}{{{{U}_{{n, - }}}(x) + \sqrt {1 + {{n}^{2}}} + 1}}} \right),$$

(A.59)

with

$$\begin{gathered} {{U}_{{n, + }}}(x) = {{n}^{2}}\left( {{{x}^{2}}\left( {\sqrt {1 + {{n}^{2}}} + 1} \right) + 1} \right) \\ \pm \,\,n\sqrt 2 x{{\left( {\sqrt {1 + {{n}^{2}}} + 1} \right)}^{{(3/2)}}}, \\ \end{gathered} $$

together with

$$\begin{gathered} {{H}_{n}}(x) = 2\left( {\sqrt {1 + {{n}^{2}}} + 1} \right) \\ \times \,\,\left( {\arctan \left( {{{I}_{n}}(x)} \right) - \arctan \left( {{{J}_{n}}(x)} \right)} \right). \\ \end{gathered} $$

(A.60)

The I_n(x) and J_n(x) are defined by

$$\begin{gathered} {{I}_{n}}(x) = \frac{{\sqrt {\sqrt {1 + {{n}^{2}}} + 1} + nx\sqrt 2 }}{{\sqrt {\sqrt {1 + {{n}^{2}}} - 1} }}, \hfill \\ {{J}_{n}}(x) = \frac{{\sqrt {\sqrt {1 + {{n}^{2}}} + 1} - nx\sqrt 2 }}{{\sqrt {\sqrt {1 + {{n}^{2}}} - 1} }}. \hfill \\ \end{gathered} $$

(A.61)

This set of definitions (A.58)–(A.61) define the F_n(x) and we have

$${{K}_{n}} = {{F}_{n}}(1{\text{/}}4) - {{F}_{n}}(1{\text{/}}n).$$

(A.62)

The reader can check that indeed the K_n expressed in (A.62) approaches zero for increasing n. However, how to explain the contrast with K_n ≈ 1 such as given in the main text. We believe that this is the ultimate example of Gödel negation incompleteness in concrete mathematics. It is noted that the aforementioned reviewer presented the expression without the explicit proof that his is a single unique solution.

APPENDIX B

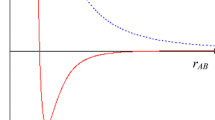
This result can be found in Fig. 1 above With the particular parameters in the code we get 1 ≥ K_n ≈ 0.9671.

program testAtAr

integer nmax, n, m,j,k

real*8 h,xx,funfArr,nlim,f2,ffHelp

real*8 pw

real*8 eps,beta,fact,y0,ystart,yfin

real*8 g1,g2,f,f0,resl, integral

parameter(nlim = 3.5e17)

parameter(nmax = 50)

parameter (m = 100)

parameter (h = 5.3e-6)

real*8 ffArray(m),xxVar(m)

c output for plot

open(1,

+file = 'res.txt'

+,status = 'unknown')

open(2,

+file = 'xres.txt'

+,status = 'unknown')

open(3,

+file = 'yres.txt'

+,status = 'unknown')

write(1,*)0.0

write(2,*)0.0

eps = 1/nlim

f0 = (nlim*nlim)*dsqrt(1.0+(eps*eps))

c determine the proper starting point given the integration

c interval h to 'catch' the singularity at a given n

beta = –(2.6)/(3.0)

y0 = 1.0/dsqrt(1.0+(eps*eps))

pw = –(2.0)/(3.0)

f2 = (2.0)**pw

y0 = y0*(f2*(nlim**beta)–(1/(nlim*nlim)))

ystart = y0–(9.0*h)

yfin = y0+(20.0*h)

c the yfin is there to not waste too much iterations

xx = ystart

j = 0

10 continue

if(xx.gt.0) then

j = j+1

xxVar(j) = xx

ffHelp = funfArr(xx,nlim)

g1 = ffHelp/dsqrt(1.0+(eps*eps))

f = f0*xx

g2 = f0/((f+1.0)**(1.5))

ffArray(j) = (g1*g2)/2.0

write(1,*)ffArray(j)

write(2,*)xxVar(j)

endif

xx = xx+h

if (xx.lt.yfin) go to 10

write(*,*) 'number of iterations = ',j

c integration

resl = integral(ffArray,h,j)

write(*,*)'integral = ',resl

write(3,*) resl

close(unit = 3)

close(unit = 2)

close(unit = 1)

stop

end

real*8 function integral(ffArray,h,n)

integer i,j,k,n,m

parameter (m = 100)

real*8 sum,h,ffArray(m)

sum = 0

do 10 j = 1,n

10 sum = sum+(ffArray(j)*h)

integral = sum

return

end

real*8 function funfArr(xx,nlim)

real*8 xx,nlim

real*8 pi,y,z

z = nlim*xx

pi = 4*atan(1.0)

y = (2/pi)*atan(z)

funfArr = y

return

end