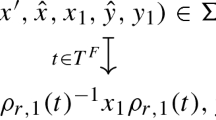Abstract
A convenient pure algebraic description of the coordinate groups of irreducible algebraic sets over a non-Abelian free 2-nilpotent group N of finite rank is given. Note that, in algebraic geometry over an arbitrary group N, it is natural to consider groups containing N as a subgroup (so-called N-groups) and homomorphisms of N-groups which are identical on N (N-homomorphisms). As a corollary, we describe all finitely generated groups H that are universally equivalent to N (with constants from N in the language). Additionally, we give a pure algebraic criterion determining when a finitely generated N-group H that is N-separated by N is, in fact, N-discriminated by N.
Similar content being viewed by others
Avoid common mistakes on your manuscript.
The basic concepts of algebraic geometry over groups were described in the works by Baumslag et al. [1], which we follow in the terminology and notation. More general, universal algebraic geometry applicable to arbitrary algebraic systems was initiated by Plotkin, Daniyarova, Myasnikov, and Remeslennikov and has been successfully developed for semigroups, noncommutative rings, semilattices, and graphs. At present, algebraic geometry over groups has become an important research tool in combinatorial, geometric, and model-theoretic group theory. The most thoroughly developed are algebraic-geometric methods for free groups, hyperbolic groups, and partially commutative groups, as well as for metabelian, free solvable, and rigid solvable groups. An open question of fundamental importance in this area is the construction of algebraic geometry over torsion-free nilpotent groups, in particular, over free nilpotent groups. In addition to direct interest in nilpotent groups, the importance of this issue lies in that the Fitting subgroup \(\Phi \) of a group \(G\) is often distinguished from \(G\) by a finite system of equations, so algebraic geometry over the nilpotent group \(\Phi \) is directly embedded in algebraic geometry over the initial possibly non-nilpotent group \(G\). In this paper, we describe the algebraic properties of coordinate groups of algebraic sets and their irreducible components over a group \(N\) (the Zariski topology over N is Noetherian, so every algebraic set is the finite union of its irreducible components). Due to these results, we can hope that other fundamental problems in algebraic geometry over \(N\) would be solved, for example, a reasonable description of solution sets of finite systems of equations over \(N\) would be obtained (despite the Diophantine problem over \(N\) is unsolvable).
Note that, in algebraic geometry over an arbitrary group \(N\), it is natural to consider groups containing \(N\) as a subgroup (so-called N-groups) and homomorphisms of N-groups that are identical on \(N\) \((N\)-homomorphisms). Our approach to the description of coordinate groups is based on some discrimination results, which are also of interest in themselves. Recall that an N-group \(H\) is \(N\)-separated (N-discriminated) by an N-group \(G\) if for any \(h \in H\), \(h \ne 1\) (for any nontrivial \({{h}_{1}},\; \ldots ,\;{{h}_{n}} \in H\)) there exists an N-homomorphism \(\phi :H \to G\) such that \(\phi (h) \ne 1\) (\(\phi ({{h}_{i}}) \ne 1\), \(i = 1,\; \ldots ,\;n\)). If N = 1, then we obtain the standard concepts of separation and discrimination, which appear in various areas of group theory: in the theory of group varieties, in combinatorial group theory (groups separated by a class of groups \(\mathcal{K}\)), in geometric group theory (as limits in Gromov–Hausdorff spaces), etc. In recent years, these concepts have played an important role in the theory of group models (for characterization of groups universally equivalent to a given one) and in algebraic geometry over groups.
In 1967 Baumslag proved that a group H is discriminated by a non-Abelian free group \(F\) if and only if it is separated by \(F\) and is commutative transitive, or a CT group (the commutation relation is transitive on the set of nontrivial elements of H, i.e., the centralizers of nontrivial elements of H are Abelian). Later, similar results were found to hold for many other groups (e.g., for torsion-free hyperbolic groups). However, non-Abelian nilpotent groups are never CT groups, since they always have a nontrivial center. Nevertheless, it turned out that the definition of a CT group can be slightly generalized so that the new definition works in classes of nilpotent groups as well. Namely, a group H is called a CT group of level k, \(k = 0,\;1,\; \ldots \), or a \({\text{C}}{{{\text{T}}}_{k}}\) group if the centralizer of any element that is not in \({{Z}_{k}}(H)\) is Abelian; here, \({{Z}_{k}}(H)\) is the \(k\)th term in the upper central series of H. Specifically, if k = 0, then \({{Z}_{0}}(H) = 1\), so \({\text{C}}{{{\text{T}}}_{0}}\) groups are exactly CT groups. Note that, for \({\text{C}}{{{\text{T}}}_{1}}\) groups, the centralizers of noncentral elements are Abelian. Clearly, \(N\) is a \({\text{C}}{{{\text{T}}}_{1}}\) group. The concept of a \({\text{C}}{{{\text{T}}}_{1}}\) group was introduced in [3].
Proposition 1. Let \(N\) be a non-Abelian free k-nilpotent group of finite rank. If an \(N\)-group \(H\) is \(N\)-discriminated by \(N\), then \(H\) is a \(C{{T}_{{k - 1}}}\) group.
In particular, if \(N\) is a 2-nilpotent group, then an N-group \(H\) that is N-discriminated by N is a \({\text{C}}{{{\text{T}}}_{1}}\) group. The following conjecture, if true, provides an analogue of Baumslag’s theorem for 2-nilpotent groups.
Conjecture 1. Let \(N\) be a non-Abelian free 2-nilpotent group of finite rank and H be a finitely generated N-group. Then H is N-discriminated by \(N\) if and only if H is a \({\text{C}}{{{\text{T}}}_{1}}\) group that is N-separated by N.
Now we describe our main results on algebraic geometry. For this purpose, we need some definitions.
Let \(G\) be a group from the variety \({{\mathcal{N}}_{2}}\) of nilpotent groups of step \( \leqslant 2\). The Cartesian power Gn = \(G \times \; \cdots \; \times G\) (n copies) is called the affine space over \(G\). Let \(X = \{ {{x}_{1}},\; \ldots ,\;{{x}_{n}}\} \) be a set of variables and \(G[X] = G{{ * }_{{{{\mathcal{N}}_{2}}}}}F(X)\) be the nilpotent product, where \(F(X)\) is a free nilpotent group in \({{\mathcal{N}}_{2}}\) with base X. A system of equations \(S\) (or \(S = 1\)) over \(G\) is an arbitrary subset of \(G[X]\). An element \(u \in S\) can be treated as a group word in variables \({{x}_{1}},\; \ldots ,\;{{x}_{n}}\) with coefficients from \(G\), \(u\, = \,u({{x}_{1}}, \ldots ,{{x}_{n}})\). An element p = (g1, ..., \({{g}_{n}})\, \in \,{{G}^{n}}\) is called a solution of the system S = 1 in \(G\) if \(u({{g}_{1}},\; \ldots ,\;{{g}_{n}}) = 1\) in \(G\) for every \(u \in S\). A subset \(V\) of the affine space \({{G}^{n}}\) is called a algebraic set over \(G\) if V is the set of all solutions of a system of equations \(S\) from \(G[X]\); in this case, we write \(V = {{V}_{G}}(S)\). Additionally, for \(V = {{V}_{G}}(S)\), we define Rad(V) = \(\{ u \in G[X]|u(p)\) = 1 \(\forall {\kern 1pt} p \in {{V}_{G}}(S)\} \). Obviously, \(Rad(V)\) is always a normal subgroup in \(G[X]\). The group \(\Gamma (V) = G[X]\,{\text{/}}Rad(V)\) is called a coordinate group of the algebraic set V. Note that \(\Gamma (V)\) is a G-group with respect to the natural embedding \(G \to \Gamma (V)\).
Using, as a prebasis of closed sets, all algebraic sets of \({{G}^{n}}\), we turn \({{G}^{n}}\) into a topological space (Zariski topology). An irreducible algebraic set in \({{G}^{n}}\) is defined in the standard manner. It is well known that, for any linear group \(G\), specifically, for every finitely generated nilpotent group, the Zariski topology on \({{G}^{n}}\) is Noetherian; therefore, each algebraic set in \({{G}^{n}}\) is a finite union of irreducible algebraic sets.
Theorem 1. Let \(N\) be a non-Abelian free nilpotent group of finite rank and \(H\) be a finitely generated N-group. Then the following conditions are equivalent:
(1) \(H\) is a coordinate group of an algebraic set in \({{N}^{n}}\) for some positive integer k.
(2) \(H\) is N-separated by N.
(3) \(H\) is an N-subgroup of a finite direct product \({{N}^{k}} = {{N}_{1}} \times \; \cdots \; \times {{N}_{k}}\) of groups \({{N}_{i}} \simeq N\) for some positive integer k in which N is diagonally embedded.
Finally, we can state the main result.
Theorem 2. Let N be a non-Abelian free 2-nilpotent group of finite rank and H be a coordinate group of an irreducible algebraic set from \({{N}^{n}}\). Then the following conditions are satisfied:
(1) H is \(N\)-discriminated by N.
(2) H is an N-subgroup of a finite direct product \({{N}^{k}} = {{N}_{1}} \times \; \cdots \; \times {{N}_{k}}\) of groups \({{N}_{i}} \simeq N\) for some positive integer \(k\) in which N is diagonally embedded; moreover, \(H\) is a \(C{{T}_{1}}\)-group.
(3) H is an \(N\)-subgroup of a finite direct product \({{N}^{k}} = {{N}_{1}} \times \; \cdots \; \times {{N}_{k}}\) of groups \({{N}_{i}} \simeq N\) for some positive integer \(k\) in which N is diagonally embedded; moreover, for any \(i = 1,\; \ldots ,\;k\), the intersection \(H \cap {{N}_{i}}\) is an Abelian normal subgroup in \(H\).
Conjecture 2. Conditions (1)–(3) for the groups N and H in Theorem 2 are equivalent.
Change history
27 December 2022
An Erratum to this paper has been published: https://doi.org/10.1134/S1064562422070018
REFERENCES
G. Baumslag, A. Miasnikov, and V. Remeslennikov, “Algebraic geometry over groups: I. Algebraic sets and ideal theory,” J. Algebra 219 (1), 16–79 (1999). https://doi.org/10.1006/jabr.1999.7881
G. Baumslag, “Residually free groups,” Proc. London Math. Soc. 17 (3), 402–418 (1967).
F. Levin and G. Rosenberger, “On power-commutative and commutation-transitive groups,” Proceedings of Groups, St. Andrews 1985, London Math. Soc. Lecture Note Ser. Vol. 121 (Cambridge Univ. Press, Cambridge, 1986), pp. 249–253.
Author information
Authors and Affiliations
Corresponding authors
Ethics declarations
The authors declare that they have no conflicts of interest.
Additional information
Translated by I. Ruzanova
The original online version of this article was revised: Due to a retrospective Open Access order.
Rights and permissions
Open Access. This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made. The images or other third party material in this article are included in the article's Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article's Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/.
About this article
Cite this article
Amaglobeli, M.G., Miasnikov, A.G. & Remeslennikov, V.N. Description of Coordinate Groups of Irreducible Algebraic Sets over Free 2-Nilpotent Groups. Dokl. Math. 105, 68–70 (2022). https://doi.org/10.1134/S1064562422020041
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1134/S1064562422020041