Abstract
We study within the Monte Carlo Glauber model the influence of collective quantum effects in the Pb nucleus on the azimuthal anisotropy coefficients \({{\epsilon }_{{2,3}}}\) in Pb + Pb collisions at the LHC energies. To account for the quantum effects, we modify the sampling of the nucleon positions by applying suitable filters that guarantee that the colliding nuclei have the mean squared quadrupole and octupole moments consistent with the ones extracted from the experimental quadrupole and octupole strength functions for the Pb nucleus with the help of the energy weighted sum rule. Our Monte Carlo Glauber model with the modified sampling of the nucleon positions leads to \({{\epsilon }_{2}}\){2}/\({{\epsilon }_{3}}\){2} ≈ 0.8 at centrality \( \lesssim \)1%, which allows to resolve the \({{{v}}_{2}}\)-to-\({{{v}}_{3}}\) puzzle.





Similar content being viewed by others
Notes
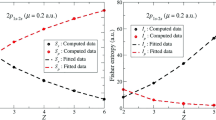
The reason for this property of \({{\epsilon }_{{2,3}}}\){2} is unclear. It may be connected with the fact that in the Glauber wounded nucleon model the variance of \({{\epsilon }_{n}}\) (as 〈\(Q_{{2,3}}^{2}\)〉) depends only on the two-nucleon correlators for the colliding nuclei. While 〈(\(Q_{{2,3}}^{2}\))2〉 depend on the four-nucleon correlators as well, which are not important for the variance of \({{\epsilon }_{n}}\) at all.
Note that our calculations show that the \(Q_{2}^{2}\)- and \(Q_{3}^{2}\)-filterings give almost zero effect on the higher harmonics \({{\epsilon }_{4}}\) and \({{\epsilon }_{5}}\), and hence we do not show them.
We ignore in this Appendix a very small effect of the c.m. nucleon correlations. However, in our numerical simulations they have been treated properly.
REFERENCES
T. Hirano, P. Huovinen, K. Murase, and Y. Nara, Prog. Part. Nucl. Phys. 70, 108 (2013).
R. Derradi de Souza, T. Koide, and T. Kodama, Prog. Part. Nucl. Phys. 86, 35 (2016).
P. Romatschke and U. Romatschke, arXiv: 1712.05815.
J.-Y. Ollitrault, Phys. Rev. D 46, 229 (1992).
F. G. Gardim, F. Grassi, M. Luzum, and J.-Y. Ollitrault, Phys. Rev. C 85, 024908 (2012).
H. Niemi, G. S. Denicol, H. Holopainen, and P. Huovinen, Phys. Rev. C 87, 054901 (2013).
M. Luzum and H. Petersen, J. Phys. G 41, 063102 (2014).
D. Teaney and L. Yan, Phys. Rev. C 83, 064904 (2011).
E. Retinskaya, M. Luzum, and J.-Y. Ollitrault, Nucl. Phys. A 926, 152 (2014).
M. L. Miller, K. Reygers, S. J. Sanders, and P. Steinberg, Ann. Rev. Nucl. Part. Sci. 57, 205 (2007).
M. Rybczynski, G. Stefanek, W. Broniowski, and P. Bozek, Comput. Phys. Commun. 185, 1759 (2014).
G. Policastro, D. T. Son, and A. O. Starinets, Phys. Rev. Lett. 87, 081601 (2001).
G. Policastro, D. T. Son, and A. O. Starinets, J. High Energy Phys. 09, 043 (2002).
J. S. Moreland, J. E. Bernhard, and S. A. Bass, Phys. Rev. C 92, 011901 (2015).
B. Schenke, P. Tribedy, and R. Venugopalan, Phys. Rev. Lett. 108, 252301 (2012).
F. Gelis, G. Giacalone, P. Guerrero-Rodrguez, C. Marquet, and J.-Y. Ollitrault, arXiv: 1907.10948.
S. Chatrchyan et al. (CMS Collab.), J. High Energy Phys. 1402, 088 (2014).
S. Acharya et al. (ALICE Collab.), J. High Energy Phys. 1807, 103 (2018).
C. Shen, Z. Qiu, and U. Heinz, Phys. Rev. C 92, 014901 (2015).
P. Carzon, S. Rao, M. Luzum, M. Sievert, and J. Noronha-Hostler, arXiv: 2007.00780.
D. Kharzeev and E. Levin, Phys. Lett. B 523, 79 (2001).
D. Kharzeev, E. Levin, and M. Nardi, Nucl. Phys. A 747, 609 (2005).
J.-B. Rose, J.-F. Paquet, G. S. Denicol, M. Luzum, B. Schenke, S. Jeon, and C. Gale, Nucl. Phys. A 931, 926 (2014).
P. Alba, V. Mantovani Sarti, J. Noronha, J. Noronha-Hostler, P. Parotto, I. Portillo Vazquez, and C. Ratti, Phys. Rev. C 98, 034909 (2018).
L. M. Robledo and G. F. Bertsch, Phys. Rev. C 84, 054302 (2011).
S. E. Agbemava, A. V. Afanasjev, and P. Ring, Phys. Rev. C 93, 044304 (2016).
A. Bohr and B. R. Mottelson, Nuclear Structure (W. A. Benjamin, New York, 1975), Vol. 2.
W. Greiner and J. A. Maruhn, Nuclear Models (Berlin, Springer, 1996).
S. Kamerdzhiev, J. Speth, and G. Tertychny, Phys. Rep. 393, 1 (2004).
X. Roca-Maza and N. Paar, Prog. Part. Nucl. Phys. 101, 96 (2018); arXiv: 1804.06256.
B. G. Zakharov, JETP Lett. 112, 393 (2020).
B. G. Zakharov, JETP Lett. 108, 723 (2018).
E. Lipparini and S. Stringari, Phys. Rep. 175, 103 (1989).
G. Giacalone, J. Noronha-Hostler, M. Luzum, and J.‑Y. Ollitrault, Phys. Rev. C 97, 034904 (2018).
J. Noronha-Hostler, Li Yan, F. G. Gardim, and J.‑Y. Ollitrault, Phys. Rev. C 93, 014909 (2016).
S. J. Das, G. Giacalone, P.-A. Monard, and J.-Y. Ollitrault, Phys. Rev. C 97, 014905 (2018).
W. Broniowski and W. Florkowski, Phys. Rev. C 65, 024905 (2002).
B. G. Zakharov, JETP Lett. 104, 6 (2016).
B. G. Zakharov, J. Exp. Theor. Phys. 124, 860 (2017).
B. G. Zakharov, Eur. Phys. J. C 78, 427 (2018).
D. Kharzeev and M. Nardi, Phys. Lett. B 507, 121 (2001).
B. Müller and K. Rajagopal, Eur. Phys. J. C 43, 15 (2005).
J. Speth and A. W. Thomas, Adv. Nucl. Phys. 24, 83 (1997).
W. Broniowski and M. Rybczynski, Phys. Rev. C 81, 064909 (2010).
B. G. Zakharov, JETP Lett. 105, 785 (2017).
G. Kh. Eyyubova, V. L. Korotkikh, A. M. Snigirev, and E. E. Zabrodin, J. Phys. G 48, 095101 (2021).
K. A. Bugaev, A. I. Ivanytskyi, V. V. Sagun, B. E. Grinyuk, D. O. Savchenko, G. M. Zinovjev, E. G. Nikonov, L. V. Bravina, E. E. Zabrodin, D. B. Blaschke, A. V. Taranenko, and L. Turko, Universe 5, 63 (2019); arXiv: 1810.00486.
M. I. Krivoruchenko, D. K. Nadyozhin, T. L. Rasinkova, Yu. A. Simonov, M. A. Trusov, and A. V. Yudin, Phys. At. Nucl. 74, 371 (2011).
V. I. Kukulin, Phys. At. Nucl. 74, 1567 (2011).
B. G. Zakharov and B. Z. Kopeliovich, Sov. J. Nucl. Phys. 42, 677 (1985).
J. Jia, arXiv: 2106.08768.
J. Schukraft, A. Timmins, and S. A. Voloshin, Phys. Lett. B 719, 394 (2013).
L. Adamczyk, J. K. Adkins, G. Agakishiev, et al. (STAR Collab.), Phys. Rev. Lett. 115, 222301 (2015).
A. Goldschmidt, Z. Qiu, C. Shen, and U. Heinz, Phys. Rev. C 92, 044903 (2015).
B. Alver, M. Baker, C. Loizides, and P. Steinberg, arXiv: 0805.4411.
H. de Vries, C. W. de Jager, and C. de Vries, At. Data Nucl. Data Tables 36, 495 (1987).
L. D. Landau and E. M. Lifshitz, Course of Theoretical Physics, Vol. 5: Statistical Physics, Part 1 (Pergamon, Oxford, 1980).
D. H. Youngblood, Y. W. Lui, H. L. Clark, B. John, Y. Tokimoto, and X. Chen, Phys. Rev. C 69, 034315 (2004).
M. N. Harakeh, B. van Heyst, K. van der Borg, and A. van der Woude, Nucl. Phys. A 327, 373 (1979).
Y. Fujita, T. Shimoda, H. Miyatake, N. Takahashi, and M. Fujiwara, Phys. Rev. C 45, 993 (1992).
T. Yamagata, S. Kishimoto, K. Yuasa, K. Iwamoto, B. Saeki, M. Tanaka, T. Fukuda, I. Miura, M. Inoue, and H. Ogata, Phys. Rev. C 23, 937 (1981);
Phys. Rev. C 23, 2798(E) (1981).
R. Pitthan, F. R. Buskirk, E. B. Dally, J. N. Dyer, and X. K. Maruyama, Phys. Rev. Lett. 33, 849 (1974);
Phys. Rev. Lett. 34, 848(E) (1975).
M. Sasao and Y. Torizuka, Phys. Rev. C 15, 217 (1977).
T. A. Carey, W. D. Cornelius, N. J. Digiacomo, J. M. Moss, G. S. Adams, et al., Phys. Rev. Lett. 45, 239 (1980).
B. F. Davis, U. Garg, W. Reviol, M. N. Harakeh, A. Bacher, et al., Phys. Rev. Lett. 79, 609 (1997).
J. P. Blaizot, Phys. Rep. 64, 171 (1980).
D. Patel, U. Garg, M. Itoh, H. Akimune, G. P. A. Berg, et al., Phys. Lett. B 735, 387 (2014).
ACKNOWLEDGMENTS
I am grateful to S.P. Kamerdzhiev for helpful discussions on physics of the giant resonances and our method of calculation of the squared L-multipole moments. This work was partly supported by RFBR grant 18-02-40069mega.
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
The authors declare that they have no conflicts of interest.
Appendices
APPENDIX
Calculation of the Mean Squared Multipole Moments of the 208Pb Nucleus
For completeness, in this Appendix we briefly review the method of [31] for calculation of the mean squared multipole moments of the 208Pb nucleus with the help of the EWSR [27, 33], and give the ratios between the mean squared multipole moments obtained using the ordinary MC WS sampling of the nucleon positions and those calculated using the EWSR.
We assume that in the ground state the 208Pb nucleus is spherical. We write the nuclear density in the WS form
with RA = 6.62 fm, and d = 0.546 fm [55, 56]. We define the quadrupole and octupole moments in terms of the spherical harmonics, YLm, with L = 2 and 3. The needed isosinglet L-multipole operator reads (see, e.g. [27, 28, 30])
with ni = ri/|ri|. The mean squared L-multipole moment, 〈\(Q_{L}^{2}\)〉, of a nucleus in the ground state can be defined quantum mechanically as
Classical calculation of 〈\(Q_{L}^{2}\)〉 for the uncorrelated WS nuclear density givesFootnote 3
Of course, this formula becomes invalid if one includes the effect of the short range hard core NN correlations. But their effect is not very strong (see below). To perform quantum calculation of 〈\(Q_{L}^{2}\)〉 of the 208Pb nucleus we use the EWSR (for a review, see [33]) for strength function, S(ω) of the operator FL. It is defined as
where ωn = En – E0 and En are the nucleus state energies. In terms of moments of the strength function, given by
we can write 〈0|\(F_{L}^{ + }\)FL|0〉 = m0. It is convenient to rewrite it as
where
is the so called the centroid energy Ec, which can be viewed as the typical excitation energy for the operator FL acting on the ground state. The representation (A.7) is more convenient than the one via m0, because the experimental errors in the normalization of the strength function are not important for the ratio m1/m0, and the moment m1 can be exactly calculated with the help of the EWSR, which for L ≥ 2 [27, 30, 33] gives
where mN is the nucleon mass. Thus, we have
Comparing (A.10) with (A.4), we see that the ratio between the mean squared multipole moments for the ordinary MC sampling of the nucleon positions and that for quantum calculation with the help of the EWSR reads
We calculate the centroid energy using the Breit-Wigner parametrization of the strength function. Since the strength function is proportional to the imaginary part of the polarisability (susceptibility) α (which, as usual, should satisfy the relation α(–ω*) = α*(ω) [57]) for the operator FL, for each resonance a double Breit-Wigner parametrization with the poles at ±ωR – iΓR/2 (with the same residues) should be used (see Eq. (20) of [32]). For N resonances this gives
with fi the fraction of the ith resonance contribution to the EWSR.
For the isoscalar F2 operator, the EWSR for the 208Pb nucleus is practically exhausted by the isoscalar giant quadrupole resonance with ω ≈ 10.89 MeV and Γ ≈ 3 MeV [58]. Formula (A.12) with these parameters gives Ec ≈ 11.9 MeV, then from (A.11) one can obtain r2 ≈ 2.25. Thus, we see that probabilistic treatment of the 208Pb nucleus with the WS nuclear density overestimates the 3D quadrupole fluctuations. It is clear that this can lead to incorrect predictions for the 2D fluctuations of the initial QGP fireball in AA collisions as well. As in [31], our strategy to cure this problem is to modify the MC sampling of the nucleon positions by applying a suitable filter that generates the nuclear configurations with the mean squared quadrupole moment consistent with the EWSR.
To calculate r3 we need the strength function for F3. For the 208Pb nucleus, the function S(ω) for the operator F3 is distributed in a broad range of ω. There are several very narrow peaks in the low-energy region ω \( \lesssim \) 7 MeV [59–61], in which the low lying 3– state with ω ≈ 2.615 MeV exhausts ~20–25% of the EWSR [59–61] and several more states in the region 4.7 MeV \( \lesssim \) ω \( \lesssim \) 7 MeV (so called the low-energy octupole resonance (LEOR) region) that exhaust about 8–13% of the EWSR [59, 60]. In the high-energy region there is a broad resonance at ω ~ 16–20 MeV with Γ ~ 5–8 MeV [58, 61–65]. The measured EWSR fraction of the high-energy octupole resonance (HEOR) varies from ~20–50% [63, 64] to ~60– 90% [58, 61, 62, 65]. Using the data from [60], that give 21% for the EWSR fraction of the 2.615 MeV 3– state, and 8.3% for the EWSR fraction of the LEOR region, together with parameters of the HEOR from [58] (ω ≈ 19.6 ± 0.5 MeV, ω ≈ 7.4 ± 0.6 MeV with the EWSR fraction 70 ± 14%) we obtain r3 ≈ 0.84. However, if we take 25% for the EWSR fraction of the 2.615 MeV state as obtained in [61], and the parameters of the HEOR obtained in [63] (ω = 16 MeV, Γ = 6 MeV), then we obtain r3 ≈ 0.7. Thus, we see that the experimental data on the octupole strength function of the 208Pb nucleus support that r3 \( \lesssim \) 1. But due to the experimental uncertainties for the octupole strength function, we have uncertainties in the value of r3 about 15–20%. In the present analysis we perform calculations for two values r3 = 0.84 and 0.7.
The above values of the coefficients r2 and r3 correspond to the MC sampling of the nucleon positions with the uncorrelated WS nuclear density. Calculations using the WS distribution with restrictions on the minimum nucleon–nucleon distances, to mimic the NN hard core, give somewhat di.erent values of r2,3. However, the effect of the NN hard core on r2,3 is relatively small: we obtained the reduction of r2 by the factor 0.78 (0.926), and the reduction of r3 by the factor 0.81 (0.928) for the core radius rc = 0.9 (0.6) fm.
In the present analysis we modify the MC sampling of the nucleon positions only with the filters for the isoscalar L = 2 and 3 moments, which correspond to the nuclear shape fluctuations. We do not use a filter for the L = 0 mode, that corresponds to the pure radial fluctuations. The radial fluctuations may be characterized by the squared moment for the monopole isoscalar operator F0 = \(\sum\nolimits_{i = 1}^A {(r_{i}^{2}} \) – 〈r2〉). The EWSR for this operator gives m1 = 2〈r2〉/mN [66]. Using this formula, for the uncorrelated WS nuclear density, we obtain for the analogue of (A.11) in the case of the L = 0 mode
For the isoscalar L = 0 mode the EWSR is practically exhausted by the isoscalar giant monopole resonance with ω ≈ 13.6–13.9 MeV and Γ ≈ 3 MeV [58, 67]. These parameters give Ec ≈ 15 MeV, and calculation using (A.13) for the WS distribution (A.1) gives r0 ~ 1.6. This means that for the MC sampling of the nuclear configurations with the uncorrelated WS nuclear density the magnitude of the pure radial fluctuations is somewhat overpredicted as compared to that extracted from the experimental monopole strength function. However, we have found that adding the filtering for the L = 0 mode, that decreases the mean squared L = 0 moment to its EWSR value, practically does not affect the azimuthal coefficients \({{\epsilon }_{{2,3}}}\). Therefore we do not use filtering for the L = 0 fluctuations.
Rights and permissions
About this article
Cite this article
Zakharov, B.G. Influence of Collective Nuclear Vibrations on Initial State Eccentricities in Pb + Pb Collisions. J. Exp. Theor. Phys. 134, 669–681 (2022). https://doi.org/10.1134/S1063776122050077
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1134/S1063776122050077