Effect of the Orbit Eccentricity on the Flow in the Envelope of a Hot Jupiter

Abstract

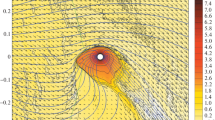
A three-dimensional numerical gas–dynamic model is presented. The aim of the model is to study the structure of the flow in the envelopes of hot Jupiters with elliptical orbits. To increase the speed and improve the accuracy of the simulation, the calculation is carried out in a noninertial frame of reference that is moving along with the planet in an elliptical orbit. The proper rotation of the coordinate frame is set in such a way as to maintain a constant direction to the star. This way it is possible to simplify the equations used in the simulation and to perform calculations on nonuniform grids, avoiding accuracy losses caused by the motion of the planet along the grid. A calculation is conducted for the flow structure in the extended envelope of a hot Jupiter moving in an orbit with an eccentricity of $e = 0.2$. It is shown that the orbit eccentricity leads to periodic variations in the flow of gas lost by the planet’s atmosphere after the planet passes the periastron. The average mass loss rate is roughly the same as the quantity obtained in the model with a circular orbit for the same size of the semimajor axis.

Appendix

To obtain equations that describe the additional forces in a noninertial frame of reference that is moving along with the planet in an elliptical orbit, we first write down these forces in the frame of reference of a stationary observer. For the sake of simplicity, we consider only two-dimensional coordinates in a plane coinciding with the plane of the orbit, and we place the star’s center of mass at the origin of the coordinates. In a polar coordinate frame, the planet’s center of mass is determined by the position angle

$$\phi = {{\phi }_{0}} + \Omega t + \frac{{{{{\dot {\Omega }}}^{2}}t}}{2}$$

and the radius

$$r = {{r}_{0}} + \dot {r}t + \frac{{\ddot {r}{{t}^{2}}}}{2}{\kern 1pt} ,$$

where ${{\phi }_{0}}$ and ${{r}_{0}}$ are certain initial values of the position angle and the radius corresponding to the planet’s position at time $t = 0$; $\Omega$ and $\dot {r}$ are the angular and radial velocity of the planet; $\dot {\Omega }$ and $\ddot {r}$ are the corresponding accelerations of the planet at the same point in time.

The local Cartesian coordinate frame bound to the planet is specified by two unit vectors, one of which (the ${\mathbf{x}}{\kern 1pt} '$ axis) is collinear to the radius vector ${\mathbf{r}}$ and the other (the ${\mathbf{y}}{\kern 1pt} '$ axis) is perpendicular to it and codirected with the velocity vector of the planet’s orbital motion:

$$\begin{gathered} {\mathbf{x}}{\kern 1pt} ' = \left( {\cos (\phi ),\sin (\phi )} \right), \\ {\mathbf{y}}{\kern 1pt} ' = \left( { - \sin (\phi ),\cos (\phi )} \right). \\ \end{gathered} $$

Let us assume that in this coordinate frame, a particle has a velocity vector ${\mathbf{v}} = ({{{v}}_{x}},{{{v}}_{y}})$. Then its motion in the laboratory coordinate frame (near time $t = 0$) is described by the equations

$$\begin{gathered} x = r\cos (\phi ) + {{{v}}_{x}}t\cos (\phi ) - {{{v}}_{y}}t\sin (\phi ), \\ y = r\sin (\phi ) + {{{v}}_{x}}t\cos (\phi ) + {{{v}}_{y}}t\sin (\phi ). \\ \end{gathered} $$

Differentiating these equations with respect to $t$ and assuming that $t = 0$, we obtain instantaneous accelerations in the laboratory coordinate frame:

$$\begin{gathered} {{a}_{x}} = \cos ({{\phi }_{0}})\left( {\ddot {r} - {{r}_{0}}{{\Omega }^{2}} - 2{{{v}}_{y}}\Omega } \right) \\ - \;\sin ({{\phi }_{0}})\left( {2\dot {r}\Omega + {{r}_{0}}\dot {\Omega } + 2{{{v}}_{x}}\Omega } \right), \\ {{a}_{y}} = \sin ({{\phi }_{0}})\left( {\ddot {r} - {{r}_{0}}{{\Omega }^{2}} - 2{{{v}}_{y}}\Omega } \right) \\ + \;\cos ({{\phi }_{0}})\left( {2\dot {r}\Omega + {{r}_{0}}\dot {\Omega } + 2{{{v}}_{x}}\Omega } \right). \\ \end{gathered} $$

Making a transition into the local coordinate frame, we obtain

$$\begin{gathered} {{a}_{{x{\kern 1pt} '}}} = {{a}_{x}}\cos (\phi ) + {{a}_{y}}\sin (\phi ) = \ddot {r} - {{r}_{0}}{{\Omega }^{2}} - 2{{{v}}_{y}}\Omega , \\ {{a}_{{y{\kern 1pt} '}}} = - {{a}_{y}}\sin (\phi ) + {{a}_{y}}\cos (\phi ) = 2\dot {r}\Omega - {{r}_{0}}\dot {\Omega } + 2{{{v}}_{x}}\Omega . \\ \end{gathered} $$

Here, the third term in both equations corresponds to the Coriolis force; the first term in the equation for ${{a}_{{x{\kern 1pt} '}}}$ is the radial acceleration of the coordinate frame; the second term in ${{a}_{x}}$ is the centrifugal force. The angular acceleration of the coordinate frame is present in the second term of the equation for ${{a}_{{y{\kern 1pt} '}}}$. The first term in the equation for ${{a}_{{y{\kern 1pt} '}}}$ is an analogue of the Coriolis force associated with the radial acceleration of the rotating coordinate frame. For orbital parameters characteristic of hot Jupiters, the main contribution (~97%) comes from the centrifugal force, if the Coriolis force is not taken into account.