Abstract
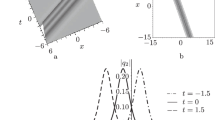
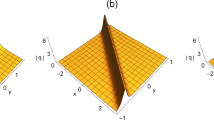
A remarkable method for investigating solutions of nonlinear soliton equation is the \(\bar\partial\)-dressing method. Although there are other methods that can also be used for that aim, the \(\bar\partial\)-dressing method is the most transparent and leads directly to the final results. The \((2+1)\)-dimensional Sawada–Kotera equation is studied by analyzing the eigenfunction and the Green’s function of its Lax representation as well as by the inverse spectral transformation, yielding a new \(\bar\partial\) problem. The solution is constructed based on solving the \(\bar\partial\)-problem by choosing a proper spectral transformation. Furthermore, once the time evolution of the spectral data is determined, we are able to completely obtain a formal solution of the Sawada–Kotera equation.
Similar content being viewed by others
References
M. J. Ablowitz and P. A. Clarkson, Solitons, Nonlinear Evolution Equations and Inverse Scattering (London Mathematical Society Lecture Note Series, Vol. 149), Cambridge Univ. Press, Cambridge (1991).
R. Hirota, “A new form of Bäcklund transformations and its relation to the inverse scattering problem,” Progr. Theor. Phys., 52, 1498–1512 (1974).
J. Weiss, M. Tabor, and G. Carnevale, “The Painlevé property for partial differential equations,” J. Math. Phys., 24, 522–526 (1983).
R. Hirota, “Exact solution of the Korteweg–de Vries equation for multiple collisions of solitons,” Phys. Rev. Lett., 27, 1192–1994 (1971).
R. Hirota, The Direct Method in Soliton Theory (Cambridge Tracts in Mathematics, Vol. 155, A. Nagai, J. Nimmo, and C. Gilson, eds.), Cambridge Univ. Press, Cambridge (2004).
R. Hirota, “Exact envelope-soliton solutions of a nonlinear wave equation,” J. Math. Phys., 14, 805–809 (1973).
R. Hirota and J. Satsuma, “Nonlinear evolution equations generated from the Bäcklund transformation for the Toda lattice,” Progr. Theor. Phys., 57, 797–807 (1977).
C. Rogers and W. F. Shadwick, Bäklund Transformations and Their Applications (Mathematics in Science and Engineering, Vol. 161), Academic Press, New York (1982).
A. M. Bruckner and J. B. Bruckner, “Darboux transformations,” Trans. Amer. Math. Soc., 128, 103–111 (1967).
V. E. Zakharov and S. V. Manakov, “Construction of higher-dimensional nonlinear integrable systems and of their solutions,” Funct. Anal. Appl., 19, 89–101 (1985).
M. J. Ablowitz, D. Bar Jaacov, and A. S. Fokas, “On the inverse scattering transform for the Kadomtsev–Petviashvili equation,” Stud. Appl. Math., 69, 135–143 (1983).
V. G. Dubrovsky, “The application of the \(\bar\partial\)-dressing method to some integrable \((2+1)\)-dimensional nonlinear equations,” J. Phys. A: Math. Gen., 29, 3617–3630 (1996).
B. G. Konopelchenko, Introduction to Multidimensional Integrable Equations. The Inverse Spectral Transform in \(2+1\) Dimensions, Springer Science, Business Media, New York (2013).
J. Zhu and X. Geng, “A hierarchy of coupled evolution equations with self-consistent sources and the dressing method,” J. Phys. A: Math. Theor., 46, 035204, 18 pp. (2012).
P. A. Deift and X. Zhou, “A steepest descent method for oscillatory Riemann–Hilbert problems. Asymptotics for the MKdV equation,” Ann. Math., 137, 295–368 (1993).
V. E. Zakharov, “Commutating operators and nonlocal \(\bar\partial\)-problem,” in: Plasma Theory and Nonlinear and Turbulent Processes in Physics, Vol. 1 (Kiev, USSR, 13–25 April, 1987, N. S. Erokhin, V. E. Zakharov, A. G. Sitenko, V. M. Chernousenko, and V. G. Bar’yakhtar, eds.), Naukova Dumka, Kiev (1988), pp. 152–154.
B. G. Konopelchenko, Solitons in MultiDimensions. Inverse Spectral Transform, World Sci., Singapore (1993).
E. V. Doktorov and S. B. Leble, A Dressing Method in Mathematical Physics (Mathematical Physics Studies, Vol. 28), Springer, Dordrecht (2007).
A. S. Fokas and V. E. Zakharov, “The dressing method and nonlocal Riemann–Hilbert problems,” J. Nonlinear Sci., 2, 109–134 (1992).
B. G. Konopelchenko and B. T. Matkarimov, “Inverse spectral transform for nonlinear evolution equation generating the Davey–Stewartson and Ishimory equations,” Stud. Appl. Math., 82, 319–359 (1990).
V. Dubrovsky and A. Topovsky, “Multi-lump solutions of KP equation with integrable boundary via \(\bar\partial\)-dressing method,” Phys. D, 414, 132740, 11 pp. (2020).
J. Zhu and Y. Kuang, “CUSP solitons to the long-short waves equation and the \(\bar\partial\)-dressing method,” Rep. Math. Phys., 75, 199–211 (2015).
Y. Kuang and J. Zhu, “The higher-order soliton solutions for the coupled Sasa–Satsuma system via the \(\bar\partial\)-dressing method,” Appl. Math. Lett., 66, 47–53 (2017).
B. G. Konopelchenko and V. G. Dubrovsky, “Some new integrable nonlinear evolution equations in \(2+1\) dimensions,” Phys. Lett. A, 102, 15–17 (1984).
A. P. Fordy and J. Gibbons, “Factorization of operators I. Miura transformations,” J. Math. Phys., 21, 2508–2510 (1980).
J. M. Dye and A. Parker, “On bidirectional fifth-order nonlinear evolution equations, Lax pairs, and directionally dependent solitary waves,” J. Math. Phys., 42, 2567–2589 (2001).
Funding
This work was supported by the National Natural Science Foundation of China (grant No. 11971475) and the Postgraduate Research & Practice Innovation Program of Jiangsu Province (grant No. KYCX21_2134).
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
The authors declare no conflicts of interest.
Additional information
Translated from Teoreticheskaya i Matematicheskaya Fizika, 2021, Vol. 209, pp. 465–474 https://doi.org/10.4213/tmf10096.
Rights and permissions
About this article
Cite this article
Chai, X., Zhang, Y. & Zhao, S. Application of the \(\bar\partial\)-dressing method to a \((2+1)\)-dimensional equation. Theor Math Phys 209, 1717–1725 (2021). https://doi.org/10.1134/S0040577921120059
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1134/S0040577921120059