INTRODUCTION

Material objects (in particular, charged particles, dipoles such as atoms and molecules, etc.) cannot travel at a speed equal to or greater than the speed of light \(c\) in a vacuum [1]. However, many different objects, long known in optics, such as light spots, effective charges, polarization, current pulses, etc., can move at an arbitrary velocity that is even faster than the velocity of light [2–7]. These include, for example, a light from a rapidly rotating projector on a remote screen [2], or a spotlight, which is the area of intersection of a short light pulse with a flat screen [2–4]. Such objects can be sources of coherent electromagnetic radiation, e.g., Vavilov–Cherenkov radiation [2–7], as well as ultrashort electromagnetic pulses [8, 9].

In recent years, significant progress has been made in the generation of extremely short pulses of electromagnetic radiation [10–15]. The limit for shortening the pulse duration when fixing the spectral range (the carrier frequency of the field oscillations is not subject to change) is to obtain half-cycle unipolar pulses containing only one half-wave of the electric field. Such pulses make it possible to effectively control the parameters of a medium on ultrafast time scales that are inaccessible when using multicycle optical pulses [16].

Recently, several different methods have been proposed that make it possible to obtain half-cycle pulses in both the terahertz (THz) and optical ranges. In particular, half-cycle pulses were obtained as precursors during multiphoton ionization in electro-optical crystals [17–19], as a result of cascade processes in plasma [20], when a metal target was excited by high-power femtosecond pulses [21–25], and during the propagation of an ultrashort pulse in nonequilibrium plasma [26–28]. In addition, in a number of works, soliton solutions were theoretically found in the form of half-cycle unipolar pulses in various nonlinear resonant media [29–34].

This paper demonstrates that by using a pair of extremely short half-cycle pulses in a resonant medium, it is possible to create an unusual object: a solitary polarization pulse that moves at the speed of light; later, the radiation of such an object is considered. Both cases of a polarization pulse of constant amplitude and when it’s changing are discussed.

As far as we know, such an object has not been considered before since the dipole moment implies the presence of charges and masses associated with it, which does not allow for travelling at the speed of light. However, an object similar to the hypothetical one mentioned above can be created in a resonant medium. This is the so-called stopped polarization pulse (SPP) [35, 36] (see also [37, 38]), which occurs when a resonant medium is excited by a pair of pulses with a duration shorter than the period of the resonant transition of the medium \({{T}_{{12}}}\). If the interval between pulses is equal to half the period of the resonant transition of the medium \({{T}_{{12}}}{\text{/}}2\), then the first pulse causes resonant oscillations of polarization in the medium, and the second pulse stops them. As a result, the time dependence of the polarization has the form of a half-wave. This SPP is essentially an artificial dipole with finite dimensions. We also note that the movement of such a dipole is not accompanied by the movement of masses in the direction of its propagation.

We explore analytically and numerically the unusual properties of the radiation produced by such a solitary polarization pulse of constant or varying amplitude. The numerical solution is carried out for the case when a solitary pulse of stopped polarization is induced by a pair of half-cycle pulses in a layer of a two-level or multilevel resonant medium. It is shown that, in the case of a spatially inhomogeneous layer of the medium, an SPP can emit a pair of unipolar rectangular pulses.

EXAMPLE OF AN ANALYTICAL SOLUTION OF A ONE-DIMENSIONAL WAVE EQUATION FOR A SOLITARY POLARIZATION PULSE

The evolution of the electric field is described by a wave equation containing the polarization of the medium on the right side. In the one-dimensional case, it looks like

$$\frac{{{{\partial }^{2}}E(z,t)}}{{\partial {{z}^{2}}}} - \frac{1}{{{{c}^{2}}}}\frac{{{{\partial }^{2}}E(z,t)}}{{\partial {{t}^{2}}}} = \frac{{4\pi }}{{{{c}^{2}}}}\frac{{{{\partial }^{2}}P(z,t)}}{{\partial {{t}^{2}}}},$$
(1)

where P is the polarization of the medium, E is the electric field strength, and c is the speed of light in vacuum.

Let us assume there is a given polarization pulse, which is localized in a certain region, is equal to zero outside this region and whose field strength P has a smooth first derivative when moving from a region where the polarization is zero to a region where it is nonzero. Let the polarization pulse propagate with a constant arbitrary speed V and its shape, satisfying the indicated conditions, has the form

$$P(z,t) = {{\Pi }_{0}}\left( {1 - \cos {{\omega }_{0}}\left( {t - \frac{z}{V}} \right)} \right),$$
$$\frac{z}{V} \leqslant t \leqslant \frac{z}{V} + {{T}_{0}},\quad {{T}_{0}} = \frac{{2\pi }}{{{{\omega }_{0}}}}.$$
(2)

In this case, we need to find a solution to the wave Eq. (1), where expression (2) is on the right side. The corresponding solution is easy to find in the form

$$E(z,t) = {{E}_{0}}\cos {{\omega }_{0}}\left( {t - \frac{z}{V}} \right),\quad \frac{z}{V} \leqslant t \leqslant \frac{z}{V} + {{T}_{0}},$$
(3)

where the following expression is obtained for the amplitude:

$${{E}_{0}} = {{\Pi }_{0}}\frac{{4\pi {{V}^{2}}}}{{{{V}^{2}} - {{c}^{2}}}}.$$
(4)

Taking into account the solution of the homogeneous wave Eq. (1) without the right side, the general solution of the inhomogeneous equation in the presence of a polarization pulse (2), taking into account the solution (3), (4), can be represented as:

$$E(z,t) = {{\Pi }_{0}}\frac{{4\pi {{V}^{2}}}}{{{{V}^{2}} - {{c}^{2}}}}$$
$$ \times \;\left[ {\cos {{\omega }_{0}}\left( {t - \frac{z}{V}} \right) - \cos {{\omega }_{0}}\left( {t - \frac{z}{c}} \right)} \right] + {{f}_{1}}\left( {t - \frac{z}{c}} \right)$$
(5)
$$ + \;{{f}_{2}}\left( {t + \frac{z}{c}} \right),\quad \frac{z}{V} \leqslant t \leqslant \frac{z}{V} + {{T}_{0}},$$

with arbitrary functions \({{f}_{1}}\) and \({{f}_{2}}\). Now, let us consider the case of solitary polarization pulse propagating at the speed of light; i.e., \(V = c\). Then the first term in (5) contains an uncertainty, and expression (5) is reduced to:

$$E(z,t) = \frac{{2\pi }}{c}{{\Pi }_{0}}{{\omega }_{0}}z\sin {{\omega }_{0}}\left( {t - \frac{z}{c}} \right),\quad \frac{z}{c} \leqslant t \leqslant \frac{z}{c} + {{T}_{0}}.$$
(6)

Equation (6) describes the linear growth of an electric field inside a solitary polarization pulse as it propagates in the medium layer; outside the region of existence of polarization, there is no electric field.

Note that in the analytical description above, the polarization is assumed to be given. Therefore, we neglect the self-action of this polarization pulse. This means that the field generated by this polarization is considered weak and incapable of causing significant changes in the polarization that generated it. In the next section, these effects will be taken into account in the numerical calculation.

EXAMPLE OF THE PRACTICAL IMPLEMENTATION OF A SOLITARY POLARIZATION PULSE AND THE FEATURES OF ITS RADIATION IN A HOMOGENEOUS AND INHOMOGENEOUS RESONANT MEDIUM

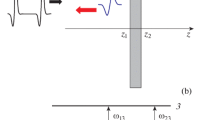
The situation in which it is possible to practically implement a solitary polarization pulse with a constant or increasing amplitude and show the features of its radiation in a homogeneous or inhomogeneous medium is presented schematically in Fig. 1.

Fig. 1.
figure 1

(Color online) Two excitatory unipolar pulses with a delay between them equal to half the period of the medium’s resonant transition (\({{T}_{{12}}}{\text{/}}2\)) are incident perpendicularly to an extended layer of a two-level medium (colored rectangle). The particle concentration distribution inside the layer \(N(z)\) has a rectangular or trapezoidal shape, the latter of which is shown schematically.

Full size image

A pair of half-cycle unipolar pulses propagating along the z axis moves from vacuum normal to an extended two-level medium (from left to right in Fig. 1). The volume concentration of particles in the medium \(N(z)\) varies along the layer and has a rectangular or trapezoidal profile (the latter is shown schematically in Fig. 1). The time delay between the exciting pulses is equal to half the period of the resonant transition of the medium \({{T}_{{12}}}{\text{/}}2 = \pi {\text{/}}{{\omega }_{{12}}}\), where \({{\omega }_{{12}}}\) is the medium’s transition frequency. We take the following expression for the electric field of incident pulses:

$$E(t) = {{E}_{0}}{{e}^{{ - {{{(t - z/c)}}^{2}}/\tau _{p}^{2}}}} + {{E}_{0}}{{e}^{{ - {{{(t - z/c - {{T}_{{12}}}/2)}}^{2}}/\tau _{p}^{2}}}}$$
(7)

with a pulse amplitude \({{E}_{0}}\) and duration \({{\tau }_{p}}\). These two excitatory unipolar pulses (7) will create an SPP, which, in the case of a sufficiently low concentration, moves along with them in the medium at a speed nearly equal to the speed of light.

MODEL’S EQUATIONS

To describe the response of a two-level medium to the external pulses’ field, we used the standard equations for the density matrix of a two-level resonant medium [39]:

$$\begin{gathered} \frac{{\partial {{\rho }_{{12}}}(z,t)}}{{\partial t}} = - \frac{{{{\rho }_{{12}}}(z,t)}}{{{{T}_{2}}}} \\ + \;i{{\omega }_{{12}}}{{\rho }_{{12}}}(z,t) - \frac{i}{\hbar }{{d}_{{12}}}E(z,t)n(z,t), \\ \end{gathered} $$
(8)
$$\begin{gathered} \frac{{\partial n(z,t)}}{{\partial t}} = - \frac{{n(z,t) - {{n}_{0}}(z)}}{{{{T}_{1}}}} \\ + \;\frac{4}{\hbar }{{d}_{{12}}}E(z,t){\text{Im}}{\kern 1pt} {{\rho }_{{12}}}(z,t), \\ \end{gathered} $$
(9)
$$P(z,t) = 2{{N}_{0}}{{d}_{{12}}}{\text{Re}}{\kern 1pt} {{\rho }_{{12}}},$$
(10)

where \({{\rho }_{{12}}}\) is the off-diagonal element of the density matrix of a two-level medium, \(n = {{\rho }_{{11}}} - {{\rho }_{{22}}}\) is the population difference (inversion) of a two-level medium, \({{d}_{{12}}}\) is the dipole moment of the transition, \({{n}_{0}}\) is the equilibrium difference between the populations of two levels in the absence of an electric field (for an absorbing medium), \({{T}_{1}}\) is relaxation time of the upper level of the medium, \({{T}_{2}}\) is the relaxation time of the medium polarization, and \(\hbar \) is the reduced Planck constant.

The equations for the medium’s response must be supplemented with a one-dimensional wave equation for the evolution of the electric field (1). A joint numerical solution of Eqs. (1) and (8)–(10) was carried out; the parameters used (see Table 1) correspond to the transition from the ground to the lower excited state in the hydrogen atom [40]. We note that the considered one-dimensional model could be implemented in coaxial waveguides, in which there is no cutoff frequency and the propagation of unipolar pulses is possible without loss of unipolarity [41].

Table 1. Parameters of the resonant two-level medium and excitation pulses used in calculations
Full size table

The result of numerical calculation of the SPP field in the case of a homogeneous medium (\(N(z) = {\text{const}}\)) presented in Fig. 2. Note that in Fig. 2 the field of excitation pulses (7) was subtracted from the total electric field, so that the result corresponds only to the radiation of the medium layer. As seen from Fig. 2, the field emitted by the medium remains localized inside the SPP and increases linearly as the SPP propagates through the layer.

Fig. 2.
figure 2

(Color online) Evolution of spatial distribution of the electric field emitted by the medium over time for the case of a homogeneous layer of the medium; the formation of a one-cycle field pulse is clearly seen. It is localized inside the SPP, and the amplitude increases linearly as the pulse propagates through the medium.

Full size image

In contrast to the situation as it is considered analytically, the polarization pulse has the characteristic form of a half-wave, shown in Fig. 3. In the analytical example, the duration of the polarization pulse had an arbitrary value. If, to compare the results, we assume that the frequency in expression (2) corresponds to the frequency of the transition of the medium, then the duration of the SPP is two times less than in the analytical problem.

Fig. 3.
figure 3

(Color online) Evolution of the spatial distribution of the polarization of the medium over time for the case of a homogeneous layer of the medium; the formation of an SPP half-wave with a constant amplitude is clearly seen.

Full size image

As in the analytical calculation, a radiation pulse arises, moving along with the SPP. Here, the source of radiation is an artificial dipole: a pulse of stopped polarization, which moves in the medium at the speed of light and is located between exciting unipolar pulses (7). Such an artificial dipole, as follows from the solution, radiates only at the moment of its formation and disappearance, occurring at the medium boundaries [35]. While moving through the medium, it does not radiate outward. If it would, for example, then the speed of radiation propagation would be greater than the speed of light. Inside the SPP, as stated above, there is a field in the form of a one-cycle pulse, and its amplitude grows in direct proportion to the distance traveled in the medium. We also note that such a short-term polarization (current) pulse moving at the speed of light could be created by moving a femtosecond laser pulse through a tube filled with gas, placed in a constant external electric field [42]. In this case, the radiation would be in the form of a short subcycle THz pulse.

Therefore, we find that the results of the analytical and numerical analyses of the radiation properties produced by solitary polarization pulses are consistent for a homogeneous medium. These properties include the localization of the field within the limits of the polarization pulse, the proportionality of the pulse shape to the time derivative of the polarization shape, and a linear increase in radiation during the movement of such a formation through space.

Let us now discuss the formation of a solitary polarization pulse in a spatially inhomogeneous layer of a resonant medium (see also [38]). Consider the trapezoidal dependence of the concentration on the coordinate, as shown in Fig. 1, namely, in the form

$$N(z) = {{N}_{0}}\frac{{3z}}{L},\quad 0 \leqslant z \leqslant 1\;\mu {\text{m}},$$
$$N(z) = {{N}_{0}},\quad 1 \leqslant z \leqslant 2\;\mu {\text{m}},$$
$$N(z) = {{N}_{0}}\frac{{3(L - z)}}{L},\quad 2 \leqslant z \leqslant 3\;\mu {\text{m}}.$$

The results of numerical simulation for when the SPP is created and moves in an inhomogeneous medium with such dependence of the concentration on the coordinate are shown in Fig. 4. This figure clearly depicts the formation of an SPP in the form of a half-wave, whose amplitude initially increases linearly, then remains constant, and finally decreases linearly to zero, following the concentration profile of resonant atoms shown in Fig. 1.

Fig. 4.
figure 4

(Color online) Evolution of the spatial distribution of the medium polarization over time in the inhomogeneous medium layer with a trapezoidal profile; the formation of an SPP half-wave with a variable amplitude clearly seen.

Full size image

The calculation results of the time dependence of the of the electric field strength emitted by the medium in the direction opposite to the propagation of the exciting pulses (7) are shown in Fig. 5. Seen clearly as the SPP creates a pair of rectangular unipolar pulses of opposite polarity in a backward radiation. The first square wave has a negative polarity; the second has a positive polarity. The distance between pulses is determined by the length of the medium area with a constant concentration of particles. The duration of rectangular pulses, in turn, is determined by the length of the medium sections, with the concentration varying linearly.

Fig. 5.
figure 5

(Color online) Time dependence of the field emitted by a layer of a two-level medium; it takes the form of a pair of rectangular unipolar pulses of opposite polarity.

Full size image

MULTILEVEL MEDIUM

The medium response in Fig. 5 was obtained using a two-level medium approximation. However, due to the short, subcycle duration of the excitation pulses, higher energy levels can significantly affect the interaction of the pulses with the resonant medium, thus making the two-level approximation inapplicable. To clarify the role of other energy levels, let us consider the excitation of a layer of a multilevel medium by pulses (7). In particular, we will take the 5 lower levels of the hydrogen atom, which correspond to the values of the main quantum number from 1 to 5. The interaction of the five-level medium with the field of exciting pulses is described using equations for the amplitudes of bound states, which have the form [39]:

$$\psi ({\mathbf{r}},t) = \sum\limits_{n = 1}^5 {{a}_{n}}(t){{\psi }_{n}}({\mathbf{r}}){{e}^{{ - \frac{{i{{E}_{n}}t}}{\hbar }}}},$$
$${{\dot {a}}_{n}}(t) = \frac{i}{\hbar }\sum\limits_{n = 1}^5 {{d}_{{nm}}}{{a}_{m}}(t)E(t){{e}^{{i{{\omega }_{{nm}}}t}}},$$
(11)
$${{\omega }_{{nl}}} = \frac{{{{E}_{n}} - {{E}_{l}}}}{\hbar },$$

where \({{a}_{n}}(t)\) are the amplitudes of the atom wave function expansion in terms of its wave eigenfunctions \({{\psi }_{n}}({\mathbf{r}})\), \({{\omega }_{{nm}}}\) are transition frequencies between levels n and m, and \({{d}_{{nm}}}\) are the corresponding dipole moments. The medium polarization is determined by using the solution of Eqs. (11) in the form

$$P(z,t) = {{N}_{0}}\sum\limits_{n = 1}^5 {{d}_{{nm}}}{{a}_{n}}(t)a_{m}^{*}(t){{e}^{{i{{\omega }_{{nm}}}t}}} + {\text{c}}{\text{.c}}.,$$
(12)

where \({{N}_{0}}\) is the volume concentration of atoms. The parameters of the multilevel resonant medium used in the calculations are presented in Table 2. Other parameters (for the exciting pulses, thickness of the medium layer, and volume concentration of atoms) were the same as in Table 1.

Table 2. Parameters of a multilevel resonant medium
Full size table

The evolution of the SPP in a multilevel medium layer with a trapezoidal dependence of the concentration on the coordinate is shown in Fig. 6. As can be seen, this case is, overall, similar to the case of a two-level medium in Fig. 4, but, due to the contribution of higher energy levels, more intense residual oscillations of the medium polarization appear after the SPP passage.

Fig. 6.
figure 6

(Color online) Time evolution of the polarization spatial distribution in an inhomogeneous layer of a multilevel medium with a trapezoidal profile of resonant atom concentration.

Full size image

Figure 7 shows the results of calculating the time dependence of the electric field strength emitted by the layer of the same multilevel medium. As in Fig. 5, we observe a pair of rectangular unipolar pulses of different polarity. By comparing Figs. 5 and 7, we see that accounting for a greater number of energy levels in the medium basically has no effect on the shape and duration of the received pulses. The main differences are reduced to the appearance of additional oscillations due to the contribution of higher levels to the polarization of the medium (12), which distort the shape of the generated pulses (see also [38]).

Fig. 7.
figure 7

(Color online) Time dependence of the field emitted by a layer of a 5-level medium; pair of rectangular unipolar pulses of opposite polarity clearly seen.

Full size image

ANALYTICAL DESCRIPTION OF SPP RADIATION IN AN INHOMOGENEOUS MEDIUM

The situation considered above can be described analytically for the case of a solitary polarization pulse that grows as it propagates through space. An analytical solution of the one-dimensional wave Eq. (1) is known in the form [43]:

$${{E}_{{{\text{rad}}}}}(z,t) = - \frac{{2\pi }}{c}\int\limits_{{{z}_{1}}}^{{{z}_{2}}} \frac{{\partial P}}{{\partial t}}\left( {z{\kern 1pt} ',t - \frac{{{\text{|}}z - z{\kern 1pt} '{\text{|}}}}{c}} \right)dz{\kern 1pt} ',$$
(13)

with the spatial integration carried out over the entire thickness of the layer. According to this expression, the field radiated by the medium particles in the one-dimensional geometry is proportional to the first time derivative of the medium polarization; and the derivative of the polarization half-wave can be quite accurately described as one period of the harmonic function. Thus, for simplicity, we will assume that each infinitely thin layer of atoms of thickness \(\delta z\) inside the extended layer in Fig. 1 emits a single-cycle harmonic pulse in the form

$$e(z,t) = {{A}_{0}}\sin {{\omega }_{{12}}}\left( {t - \frac{z}{c}} \right)\delta z,\quad \frac{z}{c} \leqslant t \leqslant \frac{z}{c} + {{T}_{{12}}},$$

we will assume coefficient \({{A}_{0}}\) to be constant for the entire layer, which is acceptable for a sufficiently low concentration of resonant centers. If radiation (13) is detected or observed in location to the left of the layer (i.e., the field is observed in reflection) at a distance D from the left boundary of the layer, then at the observation point the radiation has the form

$$\tilde {e}(z,z{\kern 1pt} ' = - D,t) = {{A}_{0}}\sin {{\omega }_{{12}}}\left( {t - \frac{{z + \;{\text{|}}z - z{\kern 1pt} '{\text{|}}}}{c}} \right)\delta z$$
$$ = {{A}_{0}}\sin {{\omega }_{{12}}}\left( {t - \frac{{2z + D}}{c}} \right)\delta z,$$
$$\frac{{2z + D}}{c} \leqslant t \leqslant \frac{{2z + D}}{c} + {{T}_{{12}}}.$$

Radiation from the entire layer with a spatial particle concentration profile \(N(z)\) is given by the following expression:

$$E(z{\kern 1pt} ' = - D,t) = \int\limits_0^L N(z)\tilde {e}(z,z{\kern 1pt} ' = - D,t).$$
(14)

Let us consider the case when the concentration of particles increases linearly, i.e., corresponding to the profile in Fig. 1. Then the integral (14) takes the form

$$E(z{\kern 1pt} ' = - D,t) = \int\limits_0^L \kappa z\tilde {e}(z,z{\kern 1pt} ' = - D,t).$$
(15)

In the time interval

$${{T}_{{12}}} + \frac{D}{c} \leqslant t \leqslant \frac{{2L + D}}{c},$$

when both exciting pulses are inside the medium layer, integral (15) is reduced to the form

$$E(z{\kern 1pt} ' = - D,t)$$
$$ = \int\limits_{(ct - c{{T}_{{12}}} - D)/2}^{(ct - D)/2} {{A}_{0}}\kappa z\sin {{\omega }_{{12}}}\left( {t - \frac{{2z + D}}{c}} \right)dz$$
(16)
$$ = \frac{{\pi {{A}_{0}}\kappa {{c}^{2}}}}{{2\omega _{{12}}^{2}}},$$

e.g., the electric field does not depend on time, which corresponds to a rectangular unipolar pulse, similar to that shown in Fig. 5. In the case of a trapezoidal concentration profile, in addition to the area with a linear increase in concentration in the left part of the layer, there is a similar area in the right with a linear concentration decrease. Therefore, integral (14) adopts a shape that is essentially identical to integral (16), with a difference only in the sign of the resulting field. And, in the area where the concentration is constant, there is no backward radiation. Thus, when a layer of a medium with a trapezoidal profile of the resonant atoms concentration is excited, the emitted field is analytically shown to be a pair of unipolar rectangular pulses of equal amplitude but opposite polarity, with a time delay between them that is directly proportional to the thickness of the central part of the layer with a constant concentration, corresponding to the results of numerical simulation for the SPP.

CONCLUSIONS

In this work, the radiation of a hypothetical object: a solitary polarization pulse moving through space at the speed of light, as well as the production of a similar object in the form of a stopped polarization pulse, are studied analytically and numerically in both two-level and multilevel resonant medium models.

It is shown that the radiation generated by the polarization pulse accumulates within its localization. If the amplitude of the polarization pulse changes during the motion, then backward radiation occurs. If there is a linear rise (decrease) in the amplitude of the polarization pulse, the field strength of the backward radiation takes the shape of a rectangular unipolar pulse whose duration is equal to the time during which the change in the amplitude of the polarization pulse happens. It should be noted that the pulses obtained by us correspond to the optical range, while earlier unipolar pulses with rectangular waveform were produced in the radio and THz frequency ranges only.

The development in photonics of ultrashort and unipolar pulses [10–16], which enables them to be produced, makes the consideration of such objects as a solitary polarization pulse relevant. Previously, such possibilities of ultrafast control of polarization and dipole moments in quantum systems seemed practically inaccessible and, as far as we know, were not considered by other authors.