INTRODUCTION

An important new data on the cross sections for the open charm and beauty production in deep inelastic electron–proton scattering (DIS) have appeared [1] by combining the results of research from the H1 and ZEUS collaborations at HERA. Measurements have shown that the production of heavy flavor Q in DIS occurs predominantly due to the photon–gluon fusion process \(\gamma g \to Q\bar {Q}\) and therefore depend strongly on gluon density in the proton and mass \({{m}_{Q}}\)Footnote 1 of produced heavy quark (\(Q = c\), b and t). Theoretical studies usually serve to confirm that available HERA data can be described by perturbative heavy flavor generation in QCD (see, for example, review [2] and references therein). Further investigations are planned at future lepton–hadron and hadron–hadron colliders [3–5], such as EIC, LHeC, FCC-eh and FCC-hh (see also [6–11] for reviews), where the measurements can be performed with much increased precision and extended to much smaller x and high \({{Q}^{2}}\) values.

In our previous consideration [12, 13] we have analyzed latest experimental data [1] taken by the H1 and ZEUS Collaborations at HERA. In particular, we have studied the heavy quark contributions to the proton structure function (SF) \({{F}_{2}}(x,{{Q}^{2}})\) and reduced charm and beauty cross sections \(\sigma _{{{\text{red}}}}^{{c\bar {c}}}(x,{{Q}^{2}})\) and \(\sigma _{{{\text{red}}}}^{{b\overline b }}(x,{{Q}^{2}})\) measured mostly at small values of the Bjorken variable x in a wide region of \({{Q}^{2}}\) [14–17]. An additional important result of the evaluations [13] is that the compact analytical expressions for these DIS coefficient functions have been presented up to next-to-next-to-leading order (NNLO) accuracy. These expressions were used very recently to investigate the top quark production in the FCC-he kinematical regime [18, 19]. Here we continue our study [12, 13]. Using the previously derived expressions for DIS coefficient functions, we study the ratio \({{R}^{Q}}(x,{{Q}^{2}})\) = \(F_{L}^{Q}(x,{{Q}^{2}}){\text{/}}F_{2}^{Q}(x,{{Q}^{2}})\) in the first three orders of perturbation theory. We demonstrate an approximate \(x\)‑independence of this ratio for non-large \({{Q}^{2}}\) values, namely, \({{Q}^{2}} \leqslant 8{-} 10m_{Q}^{2}\). Moreover, we show a very slow ratio’s dependence on the choice of used gluon density.

BASIC FORMULAS

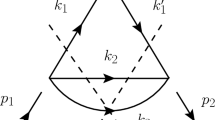
It is well known that the differential cross section \({{\sigma }^{{Q\bar {Q}}}}\) of heavy flavor production in DIS can be presented in the simple form

$$\begin{gathered} \frac{{{{d}^{2}}{{\sigma }^{{Q\bar {Q}}}}}}{{dxdy}} \\ = \frac{{2\pi {{\alpha }^{2}}}}{{x{{Q}^{4}}}}\left[ {\left( {1 - y + \frac{{{{y}^{2}}}}{2}} \right)F_{2}^{Q}(x,{{Q}^{2}}) - \frac{{{{y}^{2}}}}{2}F_{L}^{Q}(x,{{Q}^{2}})} \right], \\ \end{gathered} $$
(1)

where x and y are the usual Bjorken scaling variables.

Suggesting that the SFs \(F_{k}^{Q}(x,{{Q}^{2}})\) (\(k = 2,L\)) are driven at small x by gluonsFootnote 2 (see [2]), it is easily to obtain the following results (see, for example, [20–22]):

$$F_{k}^{Q}(x,{{Q}^{2}}) = {{C}_{{k,g}}}(x,{{Q}^{2}},m_{Q}^{2}) \otimes {{f}_{g}}(x,{{Q}^{2}}),$$
(2)

where the symbol \( \otimes \) marks the Mellin convolution:

$$\begin{gathered} {{C}_{{k,g}}}(x,{{Q}^{2}},{{a}_{Q}}) \otimes {{f}_{g}}(x,{{Q}^{2}}) \\ \equiv \int\limits_x^b {\frac{{dy}}{y}} {{C}_{{k,g}}}(y,{{Q}^{2}},{{a}_{Q}}){{f}_{g}}(x{\text{/}}y,{{Q}^{2}}), \\ \end{gathered} $$
(3)

with

$$b = \frac{1}{{1 + 4{{a}_{Q}}}}, \quad {{a}_{Q}} = \frac{{m_{Q}^{2}}}{{{{Q}^{2}}}}.$$
(4)

So, the ratio \({{R}^{Q}}(x,{{Q}^{2}})\) can be presented as

$$\begin{gathered} {{R}^{Q}}(x,{{Q}^{2}}) = \frac{{F_{L}^{Q}(x,{{Q}^{2}})}}{{F_{2}^{Q}(x,{{Q}^{2}})}} \\ = \frac{{{{C}_{{L,g}}}(x,{{Q}^{2}},{{a}_{Q}}) \otimes {{f}_{g}}(x,{{Q}^{2}})}}{{{{C}_{{2,g}}}(x,{{Q}^{2}},{{a}_{Q}}) \otimes {{f}_{g}}(x,{{Q}^{2}})}}, \\ \end{gathered} $$
(5)

where \({{f}_{g}}(x,{{Q}^{2}})\) is the gluon density in a proton.Footnote 3 In fact, the ratio \({{R}^{Q}}(x,{{Q}^{2}})\) depends slowly on non-perturbative input \({{f}_{g}}(x,{{Q}^{2}})\), which contribute to the both numerator and denominator of the ratio \({{R}^{Q}}(x,{{Q}^{2}})\).

As we already noted in Introduction, we study the ratio \({{R}^{Q}}(x,{{Q}^{2}})\) at small values of the Bjorken variable x. Using the results [23–28], below we give formulas for the high energy asymptotics of collinear coefficient functions of the heavy quark production process in the first three orders of the perturbation theory.

Wilson Coefficients

Taking the Wilson coefficient in the \(m\) order of perturbation theory, we have

$${{C}_{{k,g}}}(x) = e_{Q}^{2}{{a}_{s}}({{\mu }^{2}})\bar {C}_{{k,g}}^{{(m)}}(x),$$
$$\bar {C}_{{k,g}}^{{(m)}}(x) = B_{{k,g}}^{{(0)}}(x,{{a}_{Q}})$$
(6)
$$ + \;\sum\limits_{l = 1}^m a_{s}^{l}{\kern 1pt} B_{{k,g}}^{{(l)}}(x,{{a}_{Q}},\mu )\quad (m = 0,1,2),$$

where \({{e}_{Q}}\) is the charge of heavy quark Q and \({{a}_{s}}({{\mu }^{2}}) = {{\alpha }_{s}}({{\mu }^{2}}){\text{/}}(4\pi )\) is a strong coupling constant. Moreover, μ is the renormalization scale, which is usually taken in the form [2, 6–11]

$${{\mu }^{2}} = \mu _{Q}^{2} \equiv {{Q}^{2}} + 4m_{Q}^{2}.$$
(7)

1. The leading order (LO) Wilson coefficients have the form

$$B_{{2,g}}^{{(0)}}(x,a) = - 2x\beta [(1 - 4x(2 - a)(1 - x))$$
$$ - \;(1 - 2x(1 - 2a) + 2{{x}^{2}}(1 - 6a - 4{{a}^{2}}))L(\beta )],$$
(8)
$$B_{{L,g}}^{{(0)}}(x,a) = 8{{x}^{2}}\beta [(1 - x) - 2xaL(\beta )],$$

where

$${{\beta }^{2}} = 1 - \frac{{4ax}}{{(1 - x)}},\quad L(\beta ) = \frac{1}{\beta }\ln \frac{{1 + \beta }}{{1 - \beta }}.$$
(9)

So, for the ratio \(R_{{{\text{LO}}}}^{Q}(x,{{Q}^{2}})\) we have

$$R_{{{\text{LO}}}}^{Q}(x,{{Q}^{2}}) = \frac{{B_{{L,g}}^{{(0)}}(x,{{a}_{Q}}) \otimes {{f}_{g}}(x,{{Q}^{2}})}}{{B_{{2,g}}^{{(0)}}(x,{{a}_{Q}}) \otimes {{f}_{g}}(x,{{Q}^{2}})}}.$$
(10)

2. The next-to-leading order (NLO) coefficient functions \(B_{{k,g}}^{{(1)}}(x,a,\mu )\) of photon–gluon fusion subprocess are rather lengthy and not published in print; they are only available as computer codes [29, 30]. Following [20–22], it is sufficient to work in the high energy regime, defined by \(x \ll 1\), where they assume the compact formFootnote 4 [23–28]:

$$B_{{k,g}}^{{(1)}}(x,a,\mu )$$
$$ = \beta [R_{{k,g}}^{{(1)}}(1,a) + 4{{C}_{A}}B_{{k,g}}^{{(0)}}(1,a)L] + O(x),$$
(11)
$$L = \ln \frac{{4a{{Q}^{2}}}}{{{{\mu }^{2}}}},$$

with

$$R_{{2,g}}^{{(1)}}(1,a) = \frac{8}{9}{{C}_{A}}[5 + (13 - 10a)J(a) + 6(1 - a)I(a)],$$
$$R_{{L,g}}^{{(1)}}(1,a) = - \frac{{16}}{9}{{C}_{A}}b$$
(12)
$$ \times \;[1 - 12a - (3 + 4a(1 - 6a))J(a) + 12a(1 + 3a)I(a)]$$

and

$$\begin{gathered} B_{{2,g}}^{{(0)}}(1,a) = \frac{2}{3}[1 + 2(1 - a)J(a)], \\ B_{{L,g}}^{{(0)}}(1,a) = \frac{4}{3}{\kern 1pt} b{\kern 1pt} [1 + 6a - 4a(1 + 3a)J(a)], \\ \end{gathered} $$
(13)

whereFootnote 5

$$J(a) = - \sqrt b \ln t,$$
$$\begin{gathered} I(a) \\ = - \sqrt b \left[ {\zeta (2) + \frac{1}{2}{{{\ln }}^{2}}t - \ln (ab)\ln t + 2{\text{L}}{{{\text{i}}}_{2}}( - t)} \right], \\ \end{gathered} $$
(14)
$$t = \frac{{1 - \sqrt b }}{{1 + \sqrt b }},$$

with

$${\text{L}}{{{\text{i}}}_{2}}(x) = - \int\limits_0^1 {\frac{{dy}}{y}} \ln (1 - xy),$$
(15)

being the dilogarithmic function. We would like to note that \(B_{{k,g}}^{{(0)}}(1,a)\) are the first moments of the LO Wilson coefficients \(B_{{k,g}}^{{(0)}}(x,a)\) (see (8)):

$$B_{{k,g}}^{{(0)}}(n,a) = \int\limits_0^b {dx} {{x}^{{n - 2}}}B_{{k,g}}^{{(0)}}(x,a).$$
(16)

So, at NLO we have

$$R_{{{\text{NLO}}}}^{Q}(x,{{Q}^{2}}) = \frac{{\bar {C}_{{L,g}}^{{(1)}}(x,{{a}_{Q}}) \otimes {{f}_{g}}(x,{{Q}^{2}})}}{{\bar {C}_{{2,g}}^{{(1)}}(x,{{a}_{Q}}) \otimes {{f}_{g}}(x,{{Q}^{2}})}}{\kern 1pt} .$$
(17)

3. In the high energy regime the coefficient \(B_{{k,g}}^{{(2)}}(x,a,\mu )\) has the compact form

$$\begin{gathered} B_{{k,g}}^{{(2)}}(x,a,\mu ) = \beta \ln (1{\text{/}}x)[R_{{k,g}}^{{(2)}}(1,a) \\ + \;4{{C}_{A}}R_{{k,g}}^{{(1)}}(1,a){{L}_{\mu }} + 8C_{A}^{2}B_{{k,g}}^{{(0)}}(1,a)L_{\mu }^{2}] + O({{x}^{0}}), \\ \end{gathered} $$
(18)

with

$$R_{{2,g}}^{{(2)}}(1,a) = \frac{{32}}{{27}}C_{A}^{2}[46 + (71 - 92a)J(a)$$
$$\begin{gathered} + \;3(13 - 10a)I(a) - 9(1 - a)K(a)], \\ R_{{L,g}}^{{(1)}}(1,a) = \frac{{64}}{{27}}C_{A}^{2}b\{ 34 + 240a - [3 + 136a + 480{{a}^{2}}] \\ \end{gathered} $$
(19)
$$ \times \;J(a) + 3[3 + 4a(1 - 6a)]I(a) + 18a(1 + 3a)K(a)\} ,$$

where \(J(a)\) and \(I(a)\) are defined by (14), respectively, and

$$K(a) = - \sqrt b $$
$$\begin{gathered} \times \;[4(\zeta (3) + {\text{L}}{{{\text{i}}}_{3}}( - t) - {\text{L}}{{{\text{i}}}_{2}}( - t)\ln t - 2{{S}_{{1,2}}}( - t)) \\ + \;2\ln (ab)(\zeta (2) + 2{\text{L}}{{{\text{i}}}_{2}}( - t)) \\ \end{gathered} $$
(20)
$$ - \;\frac{1}{3}\mathop {\ln }\nolimits^3 t - {{\ln }^{2}}(ab)\ln t + \ln (ab){{\ln }^{2}}t],$$

where t is given in (14) and

$$\begin{gathered} {\text{L}}{{{\text{i}}}_{3}}(x) = \int\limits_0^1 {\frac{{dy}}{y}} \ln (y)\ln (1 - xy), \\ {{S}_{{1,2}}}(x) = \frac{1}{2}\int\limits_0^1 {\frac{{dy}}{y}} {{\ln }^{2}}(1 - xy), \\ \end{gathered} $$
(21)

are the trilogarithmic function \({\text{L}}{{{\text{i}}}_{3}}(x)\) and Nilsen polylogarithm \({{S}_{{1,2}}}(x)\) (see [31]). The results for \(K(a)\) in the form of harmonic polylogarithms [32] can be found in [28].

So, at NNLO we have

$$R_{{{\text{NNLO}}}}^{Q}(x,{{Q}^{2}}) = \frac{{\bar {C}_{{L,g}}^{{(2)}}(x,{{a}_{Q}}) \otimes {{f}_{g}}(x,{{Q}^{2}})}}{{\bar {C}_{{2,g}}^{{(2)}}(x,{{a}_{Q}}) \otimes {{f}_{g}}(x,{{Q}^{2}})}}.$$
(22)

Gluon Density in a Proton

In the previous paper [13], the gluon density \({{f}_{g}}(x,{{Q}^{2}})\) coming from the generalized double asymptotic scaling (gDAS) approach [33–35] has been applied. The usage of the gluon density leads to a good description of experimental data for the SFs \({{F}_{2}}(x,{{Q}^{2}})\) and \(F_{2}^{c}(x,{{Q}^{2}})\) (see, for example, [35] and [20–22], respectively). Moreover, the parton densities of the generalized DAS approach have been used successfully [12, 13, 36] to generate the TMD parton densities (see the recent paper [37]), applied in the \({{k}_{T}}\)-factorization approach [38–43], which is very useful in partially to study \(F_{2}^{Q}(x,{{Q}^{2}})\) and the corresponding (reduced) charm and beauty cross sections \(\sigma _{{{\text{red}}}}^{{c\bar {c}}}\) and \(\sigma _{{{\text{red}}}}^{{b\overline b }}\).

Here, with a purpose to implement more information from experimental data, additionally we will use the gluon density, corresponding to H1 parametrization [44] (see also [45–47]) for the SF \({{F}_{2}}(x,{{Q}^{2}})\):

$$\begin{gathered} F_{2}^{{\text{H}}}(x,{{Q}^{2}}) = {{C}_{{\text{H}}}}{\kern 1pt} {{x}^{{ - {{\lambda }_{{\text{H}}}}({{Q}^{2}})}}}, \\ {{\lambda }_{{\text{H}}}}({{Q}^{2}}) = {{a}_{{\text{H}}}}{\kern 1pt} \ln \left( {\frac{{{{Q}^{2}}}}{{\Lambda _{{\text{H}}}^{2}}}} \right), \\ \end{gathered} $$
(23)

with the following parameters [44]:

$${{C}_{{\text{H}}}} = 0.18,\quad {{a}_{{\text{H}}}} = 0.048,\quad {{\Lambda }_{{\text{H}}}} = 292{\kern 1pt} \;{\text{Me}}{{{\text{V}}}^{2}}.$$
(24)

Assuming the basic contribution of \({{f}_{g}}(x,{{Q}^{2}})\) to the SF \({{F}_{2}}(x,{{Q}^{2}})\) at small x values, we can suggest it in the form

$$f_{g}^{{\text{H}}}(x,{{Q}^{2}}) = C_{{\text{H}}}^{g}{\kern 1pt} {{x}^{{ - {{\lambda }_{{\text{H}}}}({{Q}^{2}})}}},$$
(25)

with some unknown \(C_{{\text{H}}}^{g}\), which value is not so important for the present investigations.

RESUME

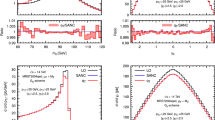
The predictions are shown in Figs. 1–3. Our calculations show a flat (x-independent) behavior of \({{R}^{Q}}(x,{{Q}^{2}})\) for small values of x (\(x \leqslant {{10}^{{ - 2}}}\)) and at not very large \({{Q}^{2}}\)-values (\({{Q}^{2}} \leqslant 8{-} 10m_{Q}^{2}\)).Footnote 6 For larger values of \({{Q}^{2}}\), some dependence on x appears, especially in the NNLO case, which has strongly singular coefficient functions \( \sim {\kern 1pt} 1{\text{/}}{{(n - 1)}^{2}}\), where n is the Mellin moment (see Eq. (16)), and leads to the strengthening \( \sim {\kern 1pt} \ln x\) in the NNLO contributions at low x (see Appendix B in [13]). In the case of c and b quarks, such flat behavior is in agreement with one observed earlier [13, 52] within the framework of the \({{k}_{T}}\)-factorization approach [38–43]. In the case of t quark, this flat behavior was recently discussed in [19].Footnote 7 Very large NNLO contribution to \({{R}^{t}}(x,{{Q}^{2}})\) for \({{Q}^{2}} \ll 4m_{t}^{2}\) indicates a strong need for resummation for small x (see, for example, [23–27, 53–58] and discussions therein), but that is beyond the scope of this short paper.

Fig. 1.
figure 1

(Color online) Ratio \({{R}^{c}}(x,{{Q}^{2}})\) of the c-quark versus x at different \({{Q}^{2}}\) values. The purple (1), azure (3) and yellow (5) lines correspond to the results obtained with AKL gluon density function [36] at LO, NLO and NNLO, respectively. The green (2), orange (4) and blue (6) lines correspond to the results obtained with H1 parametrization from Eq. (25) at LO, NLO and NNLO, respectively.

Full size image
Fig. 2.
figure 2

(Color online) Ratio \({{R}^{b}}(x,{{Q}^{2}})\) of the b-quark versus x at different \({{Q}^{2}}\) values. Notation of lines is the same as in Fig. 1.

Full size image
Fig. 3.
figure 3

(Color online) Ratio \({{R}^{t}}(x,{{Q}^{2}})\) of the t-quark versus x at different \({{Q}^{2}}\) values. Notation of lines is the same as in Fig. 1.

Full size image

As one can see in Figs. 1–3, the obtained results for \({{R}^{Q}}(x,{{Q}^{2}})\) are very similar for both gluon densities even though they have very different low x asymptotics. So, this shows a very slow dependence of the ratio \({{R}^{Q}}(x,{{Q}^{2}})\) on used gluon density. It is interesting that such flat behavior observed for \({{R}^{Q}}(x,{{Q}^{2}})\) at the first three orders of perturbation theory strongly contradicts the behavior of the ratio \(R = {{F}_{L}}{\text{/}}({{F}_{2}} - {{F}_{L}})\), which becomes strongly x-dependent in higher orders of perturbation theory (see, e.g., [2]). An attempt to resum the large terms in the perturbation theory coefficients leads [59, 60] to large values of the argument of the strong coupling constant.

We hope that the observed approximate x-independence of \({{R}^{Q}}(x,{{Q}^{2}})\) at low x will be useful in further phenomenological studies of the (reduced) cross sections \(\sigma _{{{\text{red}}}}^{{Q\overline Q }}\) and SFs \(F_{2}^{Q}(x,{{Q}^{2}})\) at future lepton–hadron and hadron–hadron colliders, such as EIC, LHeC, FCC-eh and FCC-hh.