Light Quark Masses in the Theory with the Dynamical Breaking of Chiral Symmetry

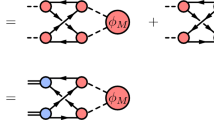
Mass formulas and decay constants of pseudoscalar π and K mesons have been obtained in the theory with four-quark interactions. To calculate the quark determinant, a Volterra series, which makes it possible to take into account the inequality of the constituent quark masses ${{M}_{u}} \ne {{M}_{d}} \ne {{M}_{s}}$ in the expansion of the effective action in the powers of 1/M², has been used for the first time. It is additionally assumed that the masses of light quarks are ${{m}_{i}} \sim \mathcal{O}(1{\text{/}}{{N}_{{\text{c}}}})$. It has been shown that the theory not only reproduces known inequalities for ratios of the masses of light quarks but also allows one to calculate some parameters characterizing the degree of breaking of chiral symmetry.

1 INTRODUCTION

If the masses of light u, d, and s quarks are neglected, quantum chromodynamics (QCD) at the classical level has the chiral U(3)_L × U(3)_R symmetry, which is spontaneously broken at low energies to the SU(3)_f × U(1)_V subgroup. This leads to the appearance of a nonet of massless Goldstone bosons (Goldstone theorem) in the spectrum. Nonzero light quark masses ${{m}_{u}} \ne {{m}_{d}} \ne {{m}_{s}}$ explicitly break the flavor SU(3)_f symmetry. As a result, Goldstone particles acquire nonzero masses.

The current algebra technique makes it possible to relate masses of pseudoscalar mesons, which appear in the Gell-Mann–Oaks–Renner relation, to the ratio of the light quark masses:

$$\frac{{{{m}_{u}}}}{{{{m}_{d}}}} = \frac{{\bar {\mu }_{{{{K}^{ + }}}}^{2} - \bar {\mu }_{{{{K}^{0}}}}^{2} + \bar {\mu }_{{{{\pi }^{ + }}}}^{2}}}{{\bar {\mu }_{{{{K}^{0}}}}^{2} - \bar {\mu }_{{{{K}^{ + }}}}^{2} + \bar {\mu }_{{{{\pi }^{ + }}}}^{2}}} \equiv {{R}_{x}},$$

(1)

$$\frac{{{{m}_{s}}}}{{{{m}_{d}}}} = \frac{{\bar {\mu }_{{{{K}^{ + }}}}^{2} + \bar {\mu }_{{{{K}^{0}}}}^{2} - \bar {\mu }_{{{{\pi }^{ + }}}}^{2}}}{{\bar {\mu }_{{{{K}^{0}}}}^{2} - \bar {\mu }_{{{{K}^{ + }}}}^{2} + \bar {\mu }_{{{{\pi }^{ + }}}}^{2}}} \equiv {{R}_{y}}.$$

(2)

Here, $\bar {\mu }_{{{{\pi }^{ + }}}}^{2} = {{B}_{0}}({{m}_{u}} + {{m}_{d}})$, $\bar {\mu }_{{{{K}^{ + }}}}^{2} = {{B}_{0}}({{m}_{u}} + {{m}_{s}})$, and $\bar {\mu }_{{{{K}^{0}}}}^{2} = {{B}_{0}}({{m}_{d}} + {{m}_{s}})$ are the masses of the mesons in the absence of the electromagnetic interaction with ${{B}_{0}} = {\text{|}}{{\langle \bar {q}q\rangle }_{0}}{\text{|/}}{{F}^{2}}$ determined by the quark condensate, where F ≃ 90 MeV.

The inclusion of the electromagnetic interaction increases the masses of charged states:

$$\mu _{{{{\pi }^{ + }}}}^{2} = \bar {\mu }_{{{{\pi }^{ + }}}}^{2} + \Delta _{{{\text{el}}}}^{2},\quad \mu _{{{{\pi }^{0}}}}^{2} = \bar {\mu }_{{{{\pi }^{ + }}}}^{2} = \bar {\mu }_{{{{\pi }^{0}}}}^{2},$$

(3)

$$\mu _{{{{K}^{ + }}}}^{2} = \bar {\mu }_{{{{K}^{ + }}}}^{2} + \tilde {\Delta }_{{el}}^{2},\quad \mu _{{{{K}^{0}}}}^{2} = \bar {\mu }_{{{{K}^{0}}}}^{2}.$$

(4)

The difference between the masses of the charged and neutral pions is due primarily to the electromagnetic interaction. The contribution of the strong interaction is proportional to ${{({{m}_{d}} - {{m}_{u}})}^{2}}$ and is thereby negligibly small.

Using the Dashen theorem [1]

$$\Delta _{{{\text{el}}}}^{{\text{2}}} = \tilde {\Delta }_{{{\text{el}}}}^{{\text{2}}},$$

(5)

which is a strict result of the current algebra, one arrives at the well-known result obtained by Weinberg [2]

$$\frac{{{{m}_{u}}}}{{{{m}_{d}}}} = \frac{{2\mu _{{{{\pi }^{0}}}}^{2} - \mu _{{{{\pi }^{ + }}}}^{2} + \mu _{{{{K}^{ + }}}}^{2} - \mu _{{{{K}^{0}}}}^{2}}}{{\mu _{{{{K}^{0}}}}^{2} - \mu _{{{{K}^{ + }}}}^{2} + \mu _{{{{\pi }^{ + }}}}^{2}}} = 0.56,$$

(6)

$$\frac{{{{m}_{s}}}}{{{{m}_{d}}}} = \frac{{\mu _{{{{K}^{ + }}}}^{2} + \mu _{{{{K}^{0}}}}^{2} - \mu _{{{{\pi }^{ + }}}}^{2}}}{{\mu _{{{{K}^{0}}}}^{2} - \mu _{{{{K}^{ + }}}}^{2} + \mu _{{{{\pi }^{ + }}}}^{2}}} = 20.18.$$

(7)

Leutwyler [3] developed an effective theory involving expansion simultaneously in the mass of light quarks, momenta, and 1/N_c, where N_c is the number of color degrees of freedom. This allowed the calculation of the first correction to the Weinberg result; as a result, Eqs. (6) and (7) are transformed to the inequalities

$$\frac{{{{m}_{u}}}}{{{{m}_{d}}}} > \frac{{2m_{{{{\pi }^{0}}}}^{2} - m_{{{{\pi }^{ + }}}}^{2} + m_{{{{K}^{ + }}}}^{2} - m_{{{{K}^{0}}}}^{2}}}{{m_{{{{K}^{0}}}}^{2} - m_{{{{K}^{ + }}}}^{2} + m_{{{{\pi }^{ + }}}}^{2}}} \equiv {{R}_{{x{\text{D}}}}},$$

(8)

$$\frac{{{{m}_{s}}}}{{{{m}_{d}}}} < \frac{{m_{{{{K}^{ + }}}}^{2} + m_{{{{K}^{0}}}}^{2} - m_{{{{\pi }^{ + }}}}^{2}}}{{m_{{{{K}^{0}}}}^{2} - m_{{{{K}^{ + }}}}^{2} + m_{{{{\pi }^{ + }}}}^{2}}} \equiv {{R}_{{y{\text{D}}}}},$$

(9)

where ${{m}_{{{{\pi }^{0}}}}},{{m}_{{{{\pi }^{ + }}}}},{{m}_{{{{K}^{0}}}}},{\text{ and }}{{m}_{{{{K}^{ + }}}}}$ are the physical masses of the pseudoscalar mesons including both the mentioned first correction and the electromagnetic contribution from virtual photons ~e² (here and below, the subscript D marks expressions that are derived with the Dashen theorem).

The aim of this work is the study of the mechanism of breaking of chiral symmetry in QCD at low energies. The specificity of this mechanism is that it involves two processes: explicit breaking of chiral symmetry through nonzero light quark masses and spontaneous symmetry breaking imposed on this picture. The latter process forms a gap in the spectrum of fermions, i.e., leads to the appearance of heavy constituent quarks. As known, four-quark interactions of the Nambu–Jona-Lasinio type [4, 5] can be responsible for this process. The bosonization of such theory is related to the calculation of the determinant of the Dirac operator with the diagonal mass matrix with nonequal elements that are much larger than the light quark masses. A mathematical formalism [6–8] based on the Fock–Schwinger proper time method [9–11] and expansion in the Volterra series has been recently proposed for such cases. A Taylor series was previously used to this end.

It is noteworthy that the calculation of the masses of pseudoscalar mesons is an excellent test to verify the efficiency of a new asymptotic expansion because it is known that the result of such expansion significantly differs from the result obtained by means of the Taylor series. This concerns both masses formulas and other low-energy characteristics of pseudoscalar mesons. Below, it is shown that the formalism proposed here reproduces both the Weinberg formulas Eqs. (6) and (7) and Leutwyler inequalities (8) and (9). Moreover, it allows one to calculate a number of physical quantities characterizing the explicit breaking of chiral symmetry, which is important for test of the predictive capability of the theory.

2 MODEL

To describe the mechanism of spontaneous breaking of chiral symmetry and to obtain the effective meson action at low energies, researchers widely use the Lagrangian with four-quark interactions [12–15]

$$\mathcal{L} = \bar {q}(i{{\gamma }^{\mu }}{{\partial }_{\mu }} - m)q + {{\mathcal{L}}_{{{\text{int}}}}}.$$

(10)

Here, ${{\gamma }^{\mu }}$ are the Dirac matrices, q are the quark fields, and m = diag(m_u, m_d, m_s) is the diagonal matrix consisting of the current masses of u, d, and s quarks. The Lagrangian density describing four-quark interaction has the form ${{\mathcal{L}}_{{{\text{int}}}}} = {{\mathcal{L}}^{{(0)}}} + {{\mathcal{L}}^{{(1)}}}$, where the first and second terms are chirally symmetric combinations with spin-0 and spin-1, respectively:

$${{\mathcal{L}}^{{(0)}}} = \frac{{{{G}_{S}}}}{2}\left[ {{{{(\bar {q}{{\lambda }_{a}}q)}}^{2}} + {{{(\bar {q}i{{\gamma }_{5}}{{\lambda }_{a}}q)}}^{2}}} \right],$$

(11)

$${{\mathcal{L}}^{{(1)}}} = - \frac{{{{G}_{V}}}}{2}\left[ {{{{(\bar {q}{{\gamma }^{\mu }}{{\lambda }_{a}}q)}}^{2}} + {{{(\bar {q}{{\gamma }^{\mu }}{{\gamma }_{5}}{{\lambda }_{a}}q)}}^{2}}} \right],$$

(12)

where ${{\lambda }_{0}} = \sqrt {2{\text{/}}3}$ and ${{\lambda }_{i}}$ are the Gell-Mann matrices. Each of the two terms in ${{\mathcal{L}}_{{{\text{int}}}}}$ is invariant under the transformation of the U(3)_L × U(3)_R group.

The method of the functional integral allows the equivalent representation of the Lagrangian density (10) in the form

$$\begin{gathered} \mathcal{L}{\kern 1pt} ' = \bar {Q}(i{{\gamma }^{\mu }}{{d}_{\mu }} - M + \sigma )Q + \frac{1}{{4{{G}_{V}}}}{\kern 1pt} {\text{tr}}{\kern 1pt} (V_{\mu }^{2} + A_{\mu }^{2}) \\ - \;\frac{1}{{4{{G}_{S}}}}{\kern 1pt} {\text{tr}}{\kern 1pt} \left( {{{\sigma }^{2}} - \{ \sigma ,M\} + (\sigma - M)\Sigma } \right), \\ \end{gathered} $$

(13)

where the functional freedom of choice of dynamic variables was used in favor of the nonlinear realization of chiral symmetry. Vector, axial vector, scalar, and pseudoscalar fields are described by Hermitian matrices ${{V}_{\mu }} = V_{\mu }^{a}{{\lambda }_{a}}$, ${{A}_{\mu }} = A_{\mu }^{a}{{\lambda }_{a}}$, $\sigma = {{\sigma }_{a}}{{\lambda }_{a}}$, $\phi = {{\phi }_{a}}{{\lambda }_{a}}$,

$$Q = (\xi {{P}_{{\text{R}}}} + {{\xi }^{\dag }}{{P}_{{\text{L}}}})q,$$

(14)

$${{d}_{\mu }} = {{\partial }_{\mu }} - i\left[ {\xi _{\mu }^{{( + )}} + {{V}_{\mu }} + {{\gamma }_{5}}\left( {\xi _{\mu }^{{( - )}} + {{A}_{\mu }}} \right)} \right],$$

(15)

$$\xi _{\mu }^{{( \pm )}} = \frac{i}{2}\left( {\xi {{\partial }_{\mu }}{{\xi }^{\dag }} \pm {{\xi }^{\dag }}{{\partial }_{\mu }}\xi } \right),$$

(16)

$$\Sigma = \xi m\xi + {{\xi }^{\dag }}m{{\xi }^{\dag }},\quad \xi = \exp \left( {\frac{i}{2}{\kern 1pt} \phi } \right).$$

(17)

The projection operators have the form P_R = (1 + γ₅)/2 and P_L = (1 – γ₅)/2. The pseudoscalar field ϕ is dimensionless and acquires the necessary dimension of mass later at the passage to field functions of physical states. The matrix M = diag(M_u, M_d, M_s) is the diagonal matrix consisting of the masses of constituent quarks Q. These masses appear through the dynamical breaking of symmetry and are controlled by the gap equation.

To obtain the effective meson Lagrangian, it is necessary to integrate the corresponding generating functional over the quark fields Q. Since quark masses are different, it is necessary to take into account difference effects accumulated in t-dependent coefficients of the expansion of the effective action in the power series of the proper time t. This property distinguishes the Volterra series from the Taylor series. In the limit of equal masses of the quarks, these coefficients are transformed to an exponential, which is completely factorized; as a result, the Volterra series is transformed to the Taylor series. For the above reasons, the Volterra series [8] is used at this step, which is distinguished from the standard consideration of the Nambu–Jona-Lasinio model.

In particular, the expansion leads to the self-consistency condition (condition of the absence of terms linear in σ in the effective Lagrangian) known as the gap equation:

$${{M}_{i}}\left( {1 - \frac{{{{N}_{c}}{{G}_{S}}}}{{2{{\pi }^{2}}}}{{J}_{0}}({{M}_{i}})} \right) = {{m}_{i}}\quad (i = u,d,s),$$

(18)

where

$${{J}_{0}}({{M}_{i}}) = {{\Lambda }^{2}} - M_{i}^{2}\ln \left( {1 + \frac{{{{\Lambda }^{2}}}}{{M_{i}^{2}}}} \right).$$

(19)

The cutoff parameter Λ characterizes the scale at which the effective theory is studied. In this case, this scale is the scale of hadron masses, which is taken to be 4πF ≈ 1.1 GeV.

In the tight binding regime

$${{G}_{S}}{{\Lambda }^{2}} > \frac{{2{{\pi }^{2}}}}{{{{N}_{{\text{c}}}}}} = 6.58,$$

(20)

each of three equations (18) has a nontrivial solution, which leads to the appearance of the gap in the spectrum of fermions and, as a result, to the dynamical breaking of chiral symmetry. According to QCD, the spontaneous breaking of chiral symmetry and confinement occur in the limit of large N_c [16, 17]; consequently, it follows from Eq. (20) that ${{G}_{S}} \sim \mathcal{O}(1{\text{/}}{{N}_{{\text{c}}}})$. It is obvious that $\Lambda \sim \mathcal{O}(1)$.

In the theory with broken chiral symmetry, pseudoscalar states are mixed with axial vector states. To remove this mixing, it is necessary to redefine the axial vector fields as [18]

$${{A}_{\mu }} = A_{\mu }^{'} - {{\kappa }_{A}} \circ \xi _{\mu }^{{( - )}},$$

(21)

where ${{\kappa }_{A}}$ is a matrix and $\circ$ stands for the Hadamard product of matrices [19], which is defined by the term-by-term product of the corresponding matrix elements

$${{(A \circ B)}_{{ij}}} = {{A}_{{ij}}}{{B}_{{ij}}}$$

(22)

without summation over repeating indices. Unlike the standard product, this product is commutative, but it is still associative and distributive.

Mixing is absent if the matrix elements of the matrix ${{\kappa }_{A}}$ have the form

$$({{\kappa }_{A}})_{{ij}}^{{ - 1}} = 1 + \frac{{8{{\pi }^{2}}}}{{{{N}_{c}}{{G}_{V}}\left[ {2({{M}_{i}} + {{M}_{j}}{{)}^{2}}{{J}_{{ij}}} + {{{(\Delta {{J}_{0}})}}_{{ij}}}} \right]}}.$$

(23)

The explicit form of the integrals ${{J}_{{ij}}} = {{J}_{1}}({{M}_{i}},{{M}_{j}})$ and ${{(\Delta {{J}_{0}})}_{{ij}}}$ is presented in Appendix D in [8]. In particular, ${{G}_{V}} \sim \mathcal{O}(1{\text{/}}{{N}_{c}})$ according to this formula.

Let us obtain expressions for the masses of the pseudoscalar mesons. Only masses of the ${{K}^{ \pm }}$, ${{K}^{0}},$ and ${{\bar {K}}^{0}}$ kaons and ${{\pi }^{ \pm }}$ charged pions are of interest. The calculated spectrum of neutral states ${{\pi }^{0}},\eta ,\eta {\kern 1pt} '$ will be presented elsewhere because it is not required in this work.

The kinetic part of the Lagrangian of free meson fields has the standard form in terms of the redefined variables

$$\begin{gathered} {{\pi }^{ \pm }} = f_{{ud}}^{{ - 1}}\pi _{{{\kern 1pt} \;{\text{ph}}{\kern 1pt} }}^{ \pm },\quad {{K}^{ \pm }} = f_{{us}}^{{ - 1}}K_{{{\kern 1pt} \;{\text{ph}}{\kern 1pt} }}^{ \pm }, \\ {{K}^{0}} = f_{{ds}}^{{ - 1}}K_{{{\kern 1pt} \;{\text{ph}}{\kern 1pt} }}^{0},\quad {{{\bar {K}}}^{0}} = f_{{ds}}^{{ - 1}}\bar {K}_{{{\kern 1pt} \;{\text{ph}}{\kern 1pt} }}^{0}, \\ \end{gathered} $$

(24)

where the subscript ph marks physical states, which have the dimension of mass as easily seen, and the constants ${{f}_{{ij}}} \sim \mathcal{O}(\sqrt {{{N}_{{\text{c}}}}} )$ have the form

$${{f}_{{ij}}} = \sqrt {\frac{{{{{({{\kappa }_{A}})}}_{{ij}}}}}{{4{{G}_{V}}}}} .$$

(25)

The mass formulas of pseudoscalars follow from the last term in Eq. (13), where it is necessary to pass to physical fields:

$$\bar {M}_{{{{\pi }^{ \pm }}}}^{2} = \frac{1}{{4{{G}_{S}}f_{{ud}}^{2}}}({{M}_{u}} + {{M}_{d}})({{m}_{u}} + {{m}_{d}}),$$

(26)

$$\bar {M}_{{{{K}^{ \pm }}}}^{2} = \frac{1}{{4{{G}_{S}}f_{{us}}^{2}}}({{M}_{u}} + {{M}_{s}})({{m}_{u}} + {{m}_{s}}),$$

(27)

$$\bar {M}_{{{{K}^{0}}}}^{2} = \frac{1}{{4{{G}_{S}}f_{{ds}}^{2}}}({{M}_{d}} + {{M}_{s}})({{m}_{d}} + {{m}_{s}}).$$

(28)

Here, the overline means that these expressions were obtained without electromagnetic corrections, as above. It should be emphasized that Eqs. (25)–(28) differ from similar expressions obtained in [14, 15] and in other available works where the model with four-quark interactions is used. However, the results completely coincide in the limit of exact SU(3)_f symmetry.

3 1/N _c EXPANSION

The effective theory under consideration contains six dimensional parameters Λ, ${{G}_{S}}$, ${{G}_{V}}$, and ${{m}_{i}}$. The first parameter determines the characteristic energy scale and the remaining parameters should be small compared to it. The natural QCD-induced measure can be their 1/N_c behavior. Accepting this, we suppose that ${{m}_{i}} \sim \mathcal{O}(1{\text{/}}{{N}_{{\text{c}}}})$. A similar assumption is used in 1/N_c chiral perturbation theory [3, 20]. This assumption means that Eqs. (25)–(28) contain contributions of different orders in 1/N_c; each of these contributions, beginning with a certain step of chiral expansion, should be corrected by the corresponding contributions from loop meson diagrams.

Further, we consider relations (18) between the masses of constituent and current quarks. As in [21], the solution is sought in the form of the expansion in the light quark masses

$${{M}_{i}}({{m}_{i}}) = {{M}_{0}} + M{\kern 1pt} '(0){\kern 1pt} {{m}_{i}} + \mathcal{O}(m_{i}^{2}).$$

(29)

It is obvious that the masses of all constituent quarks in the chiral limit ${{m}_{i}} = 0$ are equal to the same value M₀, which can be determined from the equation

$$1 - \frac{{{{N}_{{\text{c}}}}{{G}_{S}}}}{{2{{\pi }^{2}}}}{{J}_{0}}({{M}_{0}}) = 0.$$

(30)

The coefficients in the Taylor expansion (29) can be determined by differentiating Eq. (18) under the assumption that m_i are independent variables. At the first step,

$$M{\kern 1pt} '(0) = \frac{{{{\pi }^{2}}}}{{{{N}_{c}}{{G}_{S}}M_{0}^{2}J_{1}^{0}}} = \frac{{{{G}_{V}}}}{{{{G}_{S}}}}\left( {\kappa _{{A0}}^{{ - 1}} - 1} \right) \equiv a.$$

(31)

Here and below, the index 0 means that this function of the quark masses ${{m}_{i}}$ is calculated in the limit ${{m}_{i}} \to 0$. In particular, $J_{1}^{0} = {{J}_{1}}({{M}_{0}})$ and $\kappa _{{A0}}^{{ - 1}} = \mathop {\lim }\nolimits_{{{m}_{i}},{{m}_{j}} \to 0} ({{\kappa }_{A}})_{{ij}}^{{ - 1}}$. Since

$${{J}_{1}}(x) = \ln \left( {1 + \frac{{{{\Lambda }^{2}}}}{{{{x}^{2}}}}} \right) - \frac{{{{\Lambda }^{2}}}}{{{{\Lambda }^{2}} + {{x}^{2}}}}$$

(32)

is a monotonically decreasing positive definite function of x in the region x > 0, a > 0 according to Eq. (31).

Substituting Eq. (29) into Eqs. (26)–(28) and retaining the leading order in the current quark masses, we obtain the known result of the current algebra for masses of the pseudoscalar mesons, where the coefficient B₀ appearing below Eq. (2) is given by the expression

$${{B}_{0}} = - \frac{{{{{\langle \bar {q}q\rangle }}_{0}}}}{{{{F}^{2}}}} = \frac{{2{{G}_{V}}{{M}_{0}}}}{{{{G}_{S}}{{\kappa }_{{A0}}}}} = \frac{{{{M}_{0}}}}{{2{{G}_{S}}{{F}^{2}}}}.$$

(33)

Since the coefficient B₀ depends only on the parameters of the model, the mass formulas can provide information not only on ratios (6) and (7) but also on the absolute values of quark masses.

To calculate the first correction to the result of current algebra, we take the next term in the expansion of Eqs. (26)–(28) in light quark masses:

$$\bar {m}_{{{{\pi }^{ + }}}}^{2} = \bar {\mu }_{{{{\pi }^{ + }}}}^{2}\left( {1 + \frac{{{{m}_{u}} + {{m}_{d}}}}{{2{{M}_{0}}}}{\kern 1pt} {{\delta }_{M}}} \right),$$

(34)

$$\bar {m}_{{{{K}^{ + }}}}^{2} = \bar {\mu }_{{{{K}^{ + }}}}^{2}\left( {1 + \frac{{{{m}_{u}} + {{m}_{s}}}}{{2{{M}_{0}}}}{\kern 1pt} {{\delta }_{M}}} \right),$$

(35)

$$\bar {m}_{{{{K}^{0}}}}^{2} = \bar {\mu }_{{{{K}^{0}}}}^{2}\left( {1 + \frac{{{{m}_{d}} + {{m}_{s}}}}{{2{{M}_{0}}}}{\kern 1pt} {{\delta }_{M}}} \right).$$

(36)

Here,

$${{\delta }_{M}} = a\left\{ {1 - 2(1 - {{\kappa }_{{A0}}})\left[ {1 - \frac{{{{\Lambda }^{4}}}}{{J_{1}^{0}{{{({{\Lambda }^{2}} + M_{0}^{2})}}^{2}}}}} \right]} \right\}.$$

(37)

Representing Eqs. (1) and (2) in terms of mass formulas (34)–(36), we obtain

$$\begin{gathered} \frac{{\bar {m}_{{{{K}^{ + }}}}^{2} - \bar {m}_{{{{K}^{0}}}}^{2} + \bar {m}_{{{{\pi }^{ + }}}}^{2}}}{{\bar {m}_{{{{K}^{0}}}}^{2} - \bar {m}_{{{{K}^{ + }}}}^{2} + \bar {m}_{{{{\pi }^{ + }}}}^{2}}} \\ = \frac{{{{m}_{u}}}}{{{{m}_{d}}}}\left[ {1 - \frac{{{{\delta }_{M}}}}{{2{{M}_{0}}}}{\kern 1pt} {{m}_{s}}\left( {\frac{{{{m}_{d}}}}{{{{m}_{u}}}} - \frac{{{{m}_{u}}}}{{{{m}_{d}}}}} \right)} \right], \\ \end{gathered} $$

(38)

$$\begin{gathered} \frac{{\bar {m}_{{{{K}^{ + }}}}^{2} + \bar {m}_{{{{K}^{0}}}}^{2} - \bar {m}_{{{{\pi }^{ + }}}}^{2}}}{{\bar {m}_{{{{K}^{0}}}}^{2} - \bar {m}_{{{{K}^{ + }}}}^{2} + \bar {m}_{{{{\pi }^{ + }}}}^{2}}} \\ = \frac{{{{m}_{s}}}}{{{{m}_{d}}}}\left[ {1 + \frac{{{{\delta }_{M}}}}{{2{{M}_{0}}}}{\kern 1pt} {{m}_{u}}\left( {\frac{{{{m}_{s}}}}{{{{m}_{d}}}} - \frac{{{{m}_{d}}}}{{{{m}_{s}}}}} \right)} \right]. \\ \end{gathered} $$

(39)

According to these formulas, inequalities (8) and (9) are valid at δ_M > 0 and after the inclusion of electromagnetic corrections. If δ_M < 0, the reverse inequalities are valid. The sign of δ_M coincides with the sign of the expression in the braces in Eq. (37), which is a monotonically increasing function of the variable M₀ (at M₀ ≥ 0) and becomes strictly positive beginning with a certain value M_0min. At the point M_0min, δ_M = 0.

If M₀ = M_0min, the first correction vanishes and the Weinberg relations are satisfied. At Λ = 1.1 GeV, the five parameters ${{G}_{S}}$, ${{G}_{V}}$, and ${{m}_{i}}$ can be fixed by the condition δ_M = 0, the phenomenological values of the masses ${{\mu }_{{{{\pi }^{ + }}}}}$, ${{\mu }_{{{{K}^{0}}}}}$, ${{\mu }_{{{{K}^{ + }}}}}$, and the weak decay constant of the pion F = 91.5 MeV. As a result, we obtain M₀ = M_0min = 244 MeV, G_S = 6.4 GeV^–2, and G_V = 3.6 GeV^–2. The magnitude of the quark condensate is $- \langle \bar {q}q\rangle _{0}^{{1/3}}{\kern 1pt}$ = 267 MeV. The light quark masses are m_u = 2.87 MeV, m_d = 5.14 MeV, and m_s = 103.7 MeV (the Dashen theorem was used). In this case, the parameter characterizing the relative magnitude of breaking of isotopic symmetry compared to the breaking of SU(3)_f symmetry is

$$\begin{gathered} R = \frac{{{{m}_{s}} - \hat {m}}}{{{{m}_{d}} - {{m}_{u}}}} = 44.0,\quad {\text{where}} \\ \hat {m} = ({{m}_{u}} + {{m}_{d}}){\text{/}}2. \\ \end{gathered} $$

(40)

If the first correction is nonzero, M₀ increases, leading to an increase in the quark condensate. Consequently, the light quark masses decrease. Therefore, the above estimates for the masses m_u, m_d, and m_s should be considered as upper bounds appearing in the model under consideration.

Let us return to the analysis of mass formulas (34)–(36) at δ_M ≠ 0. A number of combinations where the explicit dependence on δ_M is absent can be composed from these formulas. Thus, one can analyze the properties of the theory without exact information on the parameter δ_M, which is absorbed in the physical masses of the mesons.

Leutwyler considered only one of the possibilities, that is, a second-order curve in the variables x = m_u/m_d and y = m_s/m_d. We arrive at this curve using the relations

$${{R}_{1}} = \frac{{\bar {m}_{{{{K}^{ + }}}}^{2}}}{{\bar {m}_{{{{\pi }^{ + }}}}^{2}}} = \frac{{{{m}_{u}} + {{m}_{s}}}}{{{{m}_{u}} + {{m}_{d}}}}\left[ {1 + \frac{{{{m}_{s}} - {{m}_{d}}}}{{2{{M}_{0}}}}{\kern 1pt} {{\delta }_{M}}} \right],$$

(41)

$$\frac{{\bar {m}_{{{{K}^{0}}}}^{2} - \bar {m}_{{{{K}^{ + }}}}^{2}}}{{\bar {m}_{{{{K}^{0}}}}^{2} - \bar {m}_{{{{\pi }^{ + }}}}^{2}}} = \frac{{{{m}_{d}} - {{m}_{u}}}}{{{{m}_{s}} - {{m}_{u}}}}\left[ {1 + \frac{{{{m}_{s}} - {{m}_{d}}}}{{2{{M}_{0}}}}{\kern 1pt} {{\delta }_{M}}} \right],$$

(42)

from which it follows that

$${{Q}^{2}} \equiv \left( {\frac{{\bar {m}_{{{{K}^{ + }}}}^{2}}}{{\bar {m}_{{{{\pi }^{ + }}}}^{2}}}} \right)\frac{{\bar {m}_{{{{K}^{0}}}}^{2} - \bar {m}_{{{{\pi }^{ + }}}}^{2}}}{{\bar {m}_{{{{K}^{0}}}}^{2} - \bar {m}_{{{{K}^{ + }}}}^{2}}} = \frac{{m_{s}^{2} - m_{u}^{2}}}{{m_{d}^{2} - m_{u}^{2}}}.$$

(43)

The first part of this expression depends only on the ratios x and y of the light quark masses. The locus of these (x, y) points is the ellipsis

$${{y}^{2}} - {{x}^{2}}(1 - {{Q}^{2}}) = {{Q}^{2}}.$$

(44)

Taking into account electromagnetic corrections, according to the Dashen theorem, we obtain

$${{Q}^{2}} \to Q_{{\text{D}}}^{{\text{2}}} = \frac{{(m_{{{{K}^{0}}}}^{2} - m_{{{{\pi }^{0}}}}^{2})(m_{{{{K}^{ + }}}}^{2} - m_{{{{\pi }^{ + }}}}^{2} + m_{{{{\pi }^{0}}}}^{2})}}{{m_{{{{\pi }^{0}}}}^{2}(m_{{{{K}^{0}}}}^{2} - m_{{{{K}^{ + }}}}^{2} + m_{{{{\pi }^{ + }}}}^{2} - m_{{{{\pi }^{0}}}}^{2})}},$$

(45)

which gives Q_D = 24.3 for physical values of the masses.

The direct substitution into Eq. (44) immediately shows that the point (x, y) = (R_xD, R_yD) belongs to an ellipse with the semimajor axis $Q \to {{Q}_{{\text{D}}}}$. Below, for the sake of brevity, this point is called the Weinberg point, where δ_M = 0 and, consequently, the Weinberg relations (6) and (7) are satisfied.

The comparison of these results with similar formulas from [3] shows that the parameter Δ_M used in [3] in this case has the form

$${{\Delta }_{M}} = \frac{{{{m}_{s}} - {{m}_{d}}}}{{2{{M}_{0}}}}{\kern 1pt} {{\delta }_{M}}.$$

(46)

To exclude the dependence on δ_M, other combinations of meson masses can be considered. Two examples that make it possible to establish bounds for the maximum deviations from the second-order curve are discussed below.

The first example is related to the ratios R₁ and

$$\begin{gathered} {{R}_{z}} = \frac{{{{R}_{y}}}}{{{{R}_{x}}}} = \frac{{\bar {m}_{{{{K}^{ + }}}}^{2} + \bar {m}_{{{{K}^{0}}}}^{2} - \bar {m}_{{{{\pi }^{ + }}}}^{2}}}{{\bar {m}_{{{{K}^{ + }}}}^{2} - \bar {m}_{{{{K}^{0}}}}^{2} + \bar {m}_{{{{\pi }^{ + }}}}^{2}}} \\ = \frac{y}{x}\left[ {1 + {{m}_{d}}\left( {\frac{y}{x} - \frac{x}{y}} \right)\frac{{{{\delta }_{M}}}}{{2{{M}_{0}}}}} \right]. \\ \end{gathered} $$

(47)

Excluding δ_M, we arrive at the elliptic curve

$$x(y - 1)(x{{R}_{z}} - y) = (y - x)(x + 1){{R}_{1}} + {{x}^{2}} - {{y}^{2}},$$

(48)

which has two connected components one of which passes through the Weinberg point and determines the lower bound in Fig. 1. Here, as the dashed ellipsis, the physical values of the meson are used, where electromagnetic corrections are taken into account according to the Dashen theorem; i.e.,

$${{R}_{1}} \to {{R}_{{1{\text{D}}}}} = 1 + \frac{{m_{{{{K}^{ + }}}}^{2} - m_{{{{\pi }^{ + }}}}^{2}}}{{m_{{{{\pi }^{0}}}}^{2}}},$$

(49)

$${{R}_{z}} \to {{R}_{{z{\text{D}}}}} = \frac{{m_{{{{K}^{ + }}}}^{2} + m_{{{{K}^{0}}}}^{2} - m_{{{{\pi }^{ + }}}}^{2}}}{{2m_{{{{\pi }^{0}}}}^{2} + m_{{{{K}^{ + }}}}^{2} - m_{{{{K}^{0}}}}^{2} - m_{{{{\pi }^{ + }}}}^{2}}}.$$

(50)

Fig. 1.

(Color online) (Dashed line) Ellipse specified by Eq. (44) and curves given by (lower solid line) Eq. (48) and (upper solid line) Eq. (51) obtained with the Dashen theorem. The red circle is the Weinberg point belonging to all three lines.

The second example is based on the choice of the ratios R_z and R_x. It leads to the fifth order curve

$$xy(1 - {{x}^{2}})(x{{R}_{z}} - y) = ({{y}^{2}} - {{x}^{2}})(x - {{R}_{x}}).$$

(51)

It has three connected components. Figure 1 shows the component passing through the Weinberg point. It is also obtained with the Dashen theorem: R_z → R_zD and R_x → R_xD, and it lies primarily above the ellipsis specified by Eq. (44). The other possible curves lie inside the boundaries given by these lines. The common property of these curves is that all of them pass through the Weinberg point.

The existence of numerous curves generated by mass formulas (34)–(36) does not affect the satisfaction of the Leutwyler inequalities, but significantly expands the region of values of the variables x and y. This in turn imposes additional constrains on the results obtained with any individual curve, particularly at sufficiently large deviations from the Weinberg point.

For illustration, we calculate the ratios x and y from Eqs. (34)–(36) and show that the resulting (x, y) point does not belong to the ellipsis. To this end, we fix the parameters Λ = 1.1 GeV, G_S = 6.6 GeV^–2, and G_V = 6.8 GeV^–2 so that δ_M ≠ 0. At these parameters, M₀ = 274 MeV, Δ_M = 0.092, x = 0.62, y = 19.10. The (x = 0.62, y = 19.10) point lies under the (x = 0.62, y = 19.17) point of the ellipsis. Since the parameter Δ_M is small, the deviation is small, but the deviation increases with the parameter Δ_M. For completeness, the values obtained for the light quark masses, weak decay constants of the mesons, and the parameter R are m_u = 2.8 MeV, m_d = 4.5 MeV, and m_s = 86.1 MeV, f_π = 93.2 MeV, ${{f}_{{{{K}^{ + }}}}}{\text{/}}{{f}_{\pi }} = 1.21$, and R = 47.6.

As an alternative situation, we consider the widely discussed problem of СР-parity conservation in QCD [22]. This phenomenon could be explained within the theory with the massless u quark. According to Eqs. (34) and (35) at m_u = 0 and taking into account the Dashen theorem,

$${{\Delta }_{M}} = \frac{{{{R}_{{1{\text{D}}}}}}}{y} - 1.$$

(52)

For the ellipsis y = Q_D, we obtain Δ_M = –0.45. Leutwyler established that this result corresponds to overly large corrections and is beyond the allowed lower bound Δ_M > –0.07 dictated by the $\eta {\kern 1pt} - {\kern 1pt} \eta {\kern 1pt} '$ level splitting. This indicates that the value m_u = 0 is highly improbable.

In the case of the curve specified by Eq. (48), Eq. (52) gives the different estimate Δ_M = 0, which seemingly does not exclude the variant with m_u = 0. However, the condition Δ_M = 0 means the absence of the first correction. In this case, the Weinberg formula (6) is valid; the left-hand side of Eq. (6) is now zero and the right-hand side of Eq. (6) is 0.56, as follows from the meson spectrum. Such a strong discrepancy with experimental data shifts the lower bound to the region Δ_M > 0. Leutwyler obtained a similar inequality from the in-depth analysis of the spectrum of neutral states ${{\pi }^{0}},\eta ,\eta {\kern 1pt} '$ with the inclusion of effects caused by the breaking of $U{{(1)}_{A}}$ symmetry and the Zweig rule. It is seen that the inequality Δ_M > 0 is a direct consequence of Eqs. (34)–(36).

The parameter Δ_M characterizes the degree of breaking of SU(3)_f symmetry. It cannot be calculated within 1/N_c chiral perturbation theory. The estimate 0 < Δ_M ≤ 0.13 can be obtained via additional reasonable considerations [23]. As shown above, the model under consideration gives the value Δ_M = 0.092, which belongs to this interval.

The Dashen theorem is valid only in the leading approximation of the chiral expansion. Since Δ_M ≠ 0, it is necessary to generally take into account the possible deviation from Eq. (5), i.e., to accept that $\tilde {\Delta }_{{{\text{el}}}}^{2} \ne \Delta _{{{\text{el}}}}^{2} = m_{{{{\pi }^{ + }}}}^{2} - m_{{{{\pi }^{0}}}}^{2}$. As a result, mass formulas (34)–(36) acquire an additional dependence on the parameter ${{\tilde {\Delta }}_{{{\text{el}}}}}$, which varies in the range that can be determined from the observed $\eta \to {{\pi }^{ + }}{{\pi }^{ - }}{{\pi }^{0}}$ decay width [3]. The ratio Q_D is in the range Q_D = 22.7 ± 0.8, which corresponds to ${{\tilde {\Delta }}_{{{\text{el}}}}} = (44.8 \pm 4.5){\kern 1pt} {\kern 1pt}$ MeV. For comparison, Δ_el = 35.5 MeV.

Calculations with allowance for violation of the Dashen theorem give ${{\Delta }_{M}} = 0.092 \pm 0.002$, R = 41.6 ± 2.9 (cf., e.g., R = 41 ± 4 from [24]), and

$${{m}_{u}} = \left( {2.65 \pm 0.07} \right){\text{ MeV}},$$

$${{m}_{d}} = \left( {4.63 \pm 0.07} \right){\text{ MeV}},$$

$${{m}_{s}} = \left( {85.94 \pm 0.07} \right){\text{ MeV}},$$

(53)

$${{m}_{u}}{\text{/}}{{m}_{d}} = 0.57 \pm 0.03,$$

$${{m}_{s}}{\text{/}}{{m}_{d}} = 18.55 \pm 0.29.$$

Consequently, the masses of constituent quarks are M_u = 283 MeV, M_d = 290 MeV, and M_s = 575 MeV, which hardly vary under the variation of quark masses in the range allowed by the data on the $\eta \to {{\pi }^{ + }}{{\pi }^{ - }}{{\pi }^{0}}$ decay width.

4 CONCLUSIONS

The first results of the study of the mechanism of breaking of chiral symmetry at low energies within the model with four-quark interactions have been presented. In contrast to numerous studies based on the Nambu–Jona-Lasinio model, an alternative approach based on two new ideas has been proposed. The first idea is the use of the Volterra series, which is successively applied to analyze nonlinear systems, instead of the standard Taylor expansion in proper time. This step leads to the effective action that includes a much larger number of terms than that in the standard consideration of the Nambu–Jona-Lasinio model, but the number of main parameters of the model remains the same. The second idea is the hypothesis of the 1/N_c behavior of light quark masses: ${{m}_{i}} \sim \mathcal{O}(1{\text{/}}{{N}_{{\text{c}}}})$. This hypothesis allows one to reduce the calculation of the spectrum of $\pi$ and $K$ mesons to determination of the light quark masses from the experimental masses of pseudoscalar mesons.

It has been shown that the result of the current algebra supplemented by the first 1/N_c correction successfully reproduces the masses of the ${{\pi }^{ \pm }}$, ${{K}^{ \pm }}$, ${{K}^{0}}$, and ${{\bar {K}}^{0}}$ mesons; decay constants ${{f}_{\pi }}$ and ${{f}_{K}}$; ratios m_u/m_d and m_s/m_d; and the parameters Δ_M and R characterizing the breaking of isotopic and SU(3)_f symmetries, respectively. The absolute values obtained for the light quark masses and quark condensate are less successful. They are in complete agreement with the respective values of the running quark masses and condensate obtained in the $\overline {MS}$ scheme at a scale of 2 GeV (see, e.g., [25]). However, our estimates correspond to an energy scale of 1 GeV. Such a discrepancy can indicate that it is necessary to consider the next 1/N_c correction, which, as known, includes chiral logarithms. This problem should be studied separately.