The asset allocation of defined benefit pension plans: the role of sponsor contributions

Abstract

What percentage of its assets should a defined benefit pension plan invest into stocks as its funding ratio varies? We show that the answer to this question depends on the institutional setting and in particular on the extent to which the sponsoring company contributes to the fund as the funding ratio varies. We consider two settings: in one setting, the sponsoring company contributes to its pension fund only if the funding ratio is below the target level (as is the case, for example, in the US); in the other setting, the sponsoring company always contributes to its pension fund (as is the case, for example, in Switzerland). We show that these two institutional frameworks lead to two different dynamics, conditional distributions of the funding ratios, and relationships between the current funding ratio and investment into stocks. For settings like the US, that relation is non-monotonic while for settings like in Switzerland, it is monotonically decreasing. Previous empirical findings point towards a similar pattern.

Introduction

How can one design policies so that pension funds can actually deliver the promised and hoped-for amounts of pensions? Recently, as the population ages, life-expectancy in developed countries increases and bond yields remain low, the deficit problem of pension funds has become increasingly vexing. This is particularly true of defined benefit (DB) pension funds. The company for which an employee works—the sponsoring company—establishes a pension fund and contributes to it. The benefit an employee receives upon retirement is linked to the employee’s salary and is set upfront, i.e., defined. By contrast, in a defined contribution (DC) scheme, a sponsoring company contributes a certain percentage of the employee’s salary, so that how much the employee gets when she retires depends on the market return of the investment strategy.

DB plans are more expensive and more risky for sponsoring companies than DC plans.¹ Consequently, DC plans have been replacing the DB plans in recent years (Economist 2018b). However, DB plans are still important and popular in many countries. For example, according to the OECD (2018), in Switzerland 89% of pension assets were in DB pension funds (and 11% in private pension plans); in Canada, 60% of assets were in DB plans (and 4% in DC and 36% in private plans); in the US, more than 32% were in DB plans (and 26% in DC plans and 42% in private plans). DB schemes are widely used in the public sector (Mohan and Zhang 2014). As history shows, the deficit of DB pension funds may cause cities to declare bankruptcy [e.g., Detroit in 2013, see Economist (2018a)].

In this paper, we investigate the optimal asset allocation of DB pension plans.² Specifically, what allocation of stocks and bonds should a pension fund choose? How should this allocation vary as the plan’s funding situation changes?³ We build a simple model in which the sponsoring company invests the pension fund’s wealth and the welfare of the sponsoring company depends on the funding ratio (assets/ liabilities) of the pension fund.⁴ Our primary interest is in the impact of the institutional rules that govern sponsoring company contributions.

We consider two distinct institutional settings. In the first one, the sponsoring company contributes to its pension fund if the funding ratio is less than the target level set by institutions. In the second one, the sponsoring company always contributes. The US is an instance of the first institutional context; Switzerland is an example of the second one.⁵

We find that these rules have a critical effect on the dynamics of the funding ratio and its conditional distribution. Using dynamic optimization to retrieve the optimal investment strategy⁶, we show that the dynamics of the funding ratio and its conditional distribution shape the relationship between the current funding ratio and investment into stocks.

In particular, in the US case, the relationship between the current funding ratio and investment into stocks is non-monotonic (first negative, then positive) and has a hump shape. The sponsoring company switches to a more risky investment strategy if its funding ratio is not much higher than the target level (1 in the US example), in order to keep the funding ratio above 1 in the future.

Prior empirical work on defined benefit pension funds has established results that are broadly consistent with our analysis. If we look at financially distressed pension funds, Table 3 in An et al. (2013) shows that the higher the funding ratio (i.e., the lower the “Shumway bankruptcy”) of pension funds is, the less risky an investment strategy they choose. Similarly, Andonov et al. (2017) find that the higher funding ratio of US public pension funds (which are essentially underfunded as they note in their footnote 4) is, the less risky the asset allocation becomes. On the other hand, the risk-management hypothesis tells us that if the funding ratio increases, then the asset allocation becomes riskier. Findings of An et al. (2013) in Table 4 confirm this hypothesis for the overall pool of pension funds. These empirical results together imply a non-monotonic relationship between the funding ratio and risky investment. Our theoretical model can explain these empirical findings for the US case.

Our results for the US case are broadly in line with findings of Siegmann (2007), though they differ both in the source of the effects and the detailed outcomes. In his model, the pension fund maximizes expected wealth given an expected shortfall penalty (due to loss aversion) and a budget constraint. The optimal relationship between the funding ratio and investment into stocks then exhibits a V-shape. By contrast, in our paper, it is the institutional setting, namely, the contribution pattern of the sponsoring company, that is responsible for the non-monotonic relationship between the funding ratio and investment into stocks. It is interesting that even without the behavioral assumption of loss aversion, such a pattern emerges.

In the Swiss case, the relationship between the current funding ratio and investment into stocks is monotonic. The higher the current funding ratio is, the less the sponsoring company invests into stocks. Overall, the results show that the institutional framework matters greatly for the optimal asset allocation.

Our findings complement prior theoretical work on defined-benefit pension funds. As already mentioned, our focus is on the effect of the institutional setting on the optimal investment strategy for the pension fund. Our analysis includes underfunded pension funds and we do not assume that the sponsoring company could directly boost a pension fund’s status to fully or overfunded. However, there is a well established strand of literature that examines the optimal investment for defined-benefit pension funds that are either fully or overfunded.

If we apply the generalized logarithmic model of Rubinstein (1976) to our setting, the sponsoring company should invest the amount equal to the subsistence level of the pension fund (which is liabilities of the pension fund) into cash and the discretionary wealth (which is assets of the pension fund minus liabilities of the pension fund) into risky assets, assuming the risk-free rate being zero for simplicity. This implies, in a sense, a two-fund separation. Wilcox (2000, 2003) shows that the sponsoring company should invest the discretionary wealth times the optimal leverage into risky securities. Finally, diBartolomeo (2015) argues that the sponsoring company should invest into conventional bonds, stocks and in a call option on the debt of the sponsoring company so that the funding ratio is above one with probability almost one.

The paper is organized as follows. In “Model” section , we develop a model of optimal asset allocation that accommodates two institutional settings: the sponsoring company does not always contribute and the sponsoring company always contributes. In “Results” section , we provide the results obtained from the model and the intuition driving the results. “Conclusion” section  concludes.

Model

Basic model ingredients

Funding ratio

The key object in our paper that characterizes the financial situation of the pension fund and that affects the investment decision of the sponsoring company is the funding ratio. Let $A_t$ and $L_t$ be assets and liabilities of the pension fund at time t. The funding ratio $X_t$ is defined as

$$\begin{aligned} X_t : = \frac{A_t}{L_t}. \end{aligned}$$

(1)

Intertemporal change of assets and liabilities

At time t, the assets are first $A_t$. The sponsoring company pays $CF_t$ through the pension fund to retirees and contributes $CON_t$ to the pension fund. Then, the sponsoring company invests the adjusted assets $\tilde{A}_t : = A_t - CF_t + CON_t$ into the stocks and bonds. The asset dynamics thus becomes

$$\begin{aligned} A_{t+1} = R_{t+1}\left( A_t - CF_t + CON_t \right) , \end{aligned}$$

(2)

where $R_{t+1}$ is the gross return from time t to time $t+1$.

At time t, the liabilities are first $L_t$. Next, the liabilities decrease by the amount paid to retirees $CF_t$ and increase by the service cost earned by pension $SC_t$ plan members. Here, we define the service cost as “the present value of the pension benefits earned by employees” during the period (Coronado and Sharpe 2003). The adjusted liabilities $\tilde{L}_t: = L_t - CF_t + SC_t$ grow at the rate $(1+g)$ from time t to time $t+1$. The liabilities dynamics thus becomes

$$\begin{aligned} L_{t+1} = (1+g) \left( L_t - CF_t + SC_t \right) \end{aligned}$$

(3)

Model Assumptions

Define the benchmark level $\eta >0$ as the funding ratio level such that if the funding ratio of the pension fund exceeds this funding ratio level $\eta$, then the sponsoring company does not have to contribute to the pension fund.

Assumption 1

If the funding ratio $X_t$ of the pension fund is less than a benchmark level $\eta$ (usually $\eta = 1$), then the sponsoring company contributes the service cost in a given year: $SC_t = CON_t$.

Assumption 2

If the sponsoring company contributes (which will be the key institutional characteristic of interest in what follows, see “Case 1: sponsoring company does not always contribute” and “Case 2: sponsoring company always contributes” sections ), then the cashflow paid to retirees $CF_t$ is equal to the contribution payment $CON_t$ of the sponsoring company: $CF_t = CON_t$.

Assumption 2 simplifies calculations. In reality, the relation depends on the expected salary growth rate, the discount rate, and the change in the number of plan participants. If the number of plan participants grows rapidly, $CON_t$ tends to be bigger. If in reality $CON_t = SC_t > CF_t$, the adjusted assets $\tilde{A}_t$ and the adjusted liabilities $\tilde{L_t}$ are understated by the same amount. Therefore, the overall bias is reduced. The same mitigating effect works in the other direction, too. Therefore, we believe that this simplification has only a very limited effect on the predictive power of the model.

Assumption 3

The ratio of the cashflow paid to retirees $CF_t$ to liabilities $L_t$ is constant:

$$\begin{aligned} \frac{CF_t}{L_t} = : CL >0. \end{aligned}$$

(4)

Assumption 4

The asset return $R_{t+1}$ follows a log-normal distribution:

$$\begin{aligned} R_{t+1} = \exp {\left( \mu _{t} - \frac{\sigma _t^2}{2} + \sigma _t \xi \right) }, \end{aligned}$$

(5)

where $\mu _t$ and $\sigma _t$ depend on the fraction of pension fund assets invested into stocks $\theta _t \in [0,1]$ from time t till time $t+1$ and on the fraction of pension fund assets invested into bonds $1 - \theta _t$. The random variable $\xi$ has a standard normal distribution: $\xi {\mathop {=}\limits ^{d}}{N}(0,1)$.

Let ${\mathbb {E}}[R_{t+1}^S]$ and ${\mathbb {E}}[R_{t+1}^B]$ be the expected stock and bond returns. Likewise, let ${\mathbb {V}}{\mathbb {A}}{\mathbb {R}}[R_{t+1}^S]$ and ${\mathbb {V}}{\mathbb {A}}{\mathbb {R}}[R_{t+1}^B]$ be the variances of stock and bond returns. Let $\rho ^{SB}$ stand for the correlation between the stock return and the bond return. Then parameters $\mu _t$ and $\sigma _t$ in equation (5) are given by

$$\begin{aligned} \begin{aligned} \mu _t&= \log {\mathbb {E}}_t[R_{t+1}] ,\\ \sigma _t^2&= \log \left( \frac{{\mathbb {V}}{\mathbb {A}}{\mathbb {R}}_t[R_{t+1}]}{{\mathbb {E}}_t^2[R_{t+1}]} + 1 \right) , \end{aligned} \end{aligned}$$

(6)

where

$$\begin{aligned} \begin{aligned} {\mathbb {E}}_t[R_{t+1}]&= \theta _t {\mathbb {E}}[R_{t+1}^S]+ (1 - \theta _t) {\mathbb {E}} [R_{t+1}^B], \\ {\mathbb {V}}{\mathbb {A}}{\mathbb {R}}_t[R_{t+1}]&= \theta ^2_t {\mathbb {V}}{\mathbb {A}}{\mathbb {R}}[R_{t+1}^S] + (1 - \theta _t)^2 {\mathbb {V}}{\mathbb {A}}{\mathbb {R}}[R_{t+1}^B] \\&\quad + \,2 \theta _t (1 - \theta _t) \rho ^{SB}\sqrt{{\mathbb {V}}{\mathbb {A}}{\mathbb {R}}[R_{t+1}^S] {\mathbb {V}}{\mathbb {A}}{\mathbb {R}}[R_{t+1}^B]}. \end{aligned} \end{aligned}$$

(7)

Dynamic optimization

At time t with $0 \le t \le T-1$, the sponsoring company solves the following maximization problem:⁷

$$\begin{aligned} \max _{\{\theta _{s-1}\}^{T}_{s=t+1}} {\mathbb {E}}_t \left[ \sum _{s=t+1}^{T}\beta ^{s-t}u(X_s)\right] \end{aligned}$$

(8)

where $u: {\mathbb {R}} \rightarrow {\mathbb {R}}$ is a time-separable utility function (which we specify in “Contribution rules” section ), $X_s$ is the funding ratio at time s, $\beta$ is the time discount factor, $\theta _{s-1}$ is a share of investment into stock at time $s-1$ with $t+1 \le s \le T$.

Contribution rules

Depending on formal and informal institutions which prevail in society, the sponsoring company may not always contribute to the pension fund or may always contribute. In the subsections below we look at these two cases.

Case 1: sponsoring company does not always contribute

In this case, the sponsoring company contributes to the pension fund if the funding ratio $X_{t}$ is less than the benchmark level $\eta \in (0, \infty )$; otherwise, it does not contribute. In particular, under Assumption 2:

$$\begin{aligned} CON_t = {\left\{ \begin{array}{ll} CF_t, &{} {\text {if}}\, X_t < \eta \\ 0, &{} \text {otherwise.} \end{array}\right. } \end{aligned}$$

(9)

Assumptions 2 and 3 imply that the contribution-to-liability ratio q is given by

$$\begin{aligned} q : = \frac{CON_t}{L_t} = {\left\{ \begin{array}{ll} CL, &{}{\text {if}}\, X_t<\eta , \\ 0, &{}\text {otherwise,} \end{array}\right. } \end{aligned}$$

(10)

where CL is the payout ratio $\frac{CF_t}{L_t}$ from Eq. 4.

Proposition 1

The funding ratio dynamics for the case where the sponsoring company does not always contribute to the pension fund is

$$\begin{aligned} X_{t+1} = {\left\{ \begin{array}{ll} R_{t+1} \frac{X_{t} }{(1+g)} &{} {\text {if}}\, X_{t}<\eta \\ R_{t+1} \frac{(X_{t} - CL)}{(1+g)} &{} {\text {otherwise,}} \end{array}\right. } \end{aligned}$$

(11)

where $R_{t+1}$ is the return from time t to $t+1$, CL is the payout ratio $\tfrac{CF_t}{L_t}$ from Eq. (4), and g is the liability growth rate.

Proof

Under Assumption 2, the asset dynamics is given by

$$\begin{aligned} A_{t+1} = {\left\{ \begin{array}{ll} R_{t+1} A_{t}, &{} {\text {if}}\, X_t < \eta \\ R_{t+1} (A_{t} - CF_t), &{} {\text {otherwise.}} \end{array}\right. } \end{aligned}$$

(12)

Under Assumptions 1 and 2, the liability dynamics is

$$\begin{aligned} L_{t+1} = L_{t} (1 + g). \end{aligned}$$

(13)

Using Assumptions  2 and 3, the funding ratio dynamics is

$$\begin{aligned} X_{t+1} = \frac{A_{t+1}}{L_{t+1}} = {\left\{ \begin{array}{ll} \frac{R_{t+1} A_t}{L_t (1+g)} &{} {\text {if}}\, X_t < \eta , \\ \frac{R_{t+1} (A_t - CF_t)}{L_t (1+g)} &{} {\text {otherwise.}} \end{array}\right. } \end{aligned}$$

(14)

$\square$

Since we assume that $R_{t+1}$ has a lognormal distribution (see Eq. 5), the funding ratio $X_{t+1}$ given information at time t (in particular, given $X_t = x_t$) has a lognormal distribution: $X_{t+1} \vert X_t {\mathop {=}\limits ^{d}}\log {\mathcal {N}}$. The mean ${\mathbb {E}}_t[\log X_{t+1}]$ of the corresponding conditional normal distribution is

$$\begin{aligned} {\mathbb {E}}_{t}[\log X_{t+1}] = {\left\{ \begin{array}{ll} F_t + \log \left( \frac{x_t}{x_t - CL}\right) , &{} {\text {if}}\, x_t < 1 \\ F_t, &{} \text {otherwise}, \end{array}\right. } \end{aligned}$$

(15)

where $F_t : = \mu _t - \tfrac{\sigma _t^2}{2} + \log (x_t - CL) - \log (1+g)$ and $x_t$ stands for the realization of $X_t$. The variance ${\mathbb {V}}{\mathbb {A}}{\mathbb {R}}_t[\log X_{t+1}]$ of the corresponding conditional normal distribution is $\sigma _t^2$.

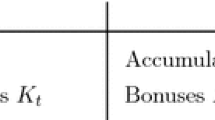
The utility function for the sponsoring company depends on the funding ratio $x_t$ and is given by

$$\begin{aligned} u(x_t) = {\left\{ \begin{array}{ll} x_t &{} {\text {if}}\, x_t < \eta , \\ \eta + Bonus \times CL &{} \text {otherwise,}\\ \end{array}\right. } \end{aligned}$$

(16)

where $Bonus \in (0, \infty )$. If the current funding ratio $x_t$ is less than the benchmark $\eta$, the sponsoring company contributes to the pension fund and prefers a better funded to a worse funded fund. The utility function reflects this. In the other case where $x_t \ge \eta$, the sponsoring company does not contribute to the pension fund, cannot retrieve surpluses easily and is, therefore, indifferent between $x_t = \eta$ and $x_t > \eta$. Moreover, since the sponsoring company does not contribute when $x_t \ge \eta$, the normalized saving of the sponsoring company is $CL : = \frac{CON_t}{L_t} = \frac{CF_t}{L_t}$. The utility reward per unit of normalized savings (or simply benefits from saved contributions) is Bonus. The utility premium of the sponsoring company is thus $Bonus \times CL$. In Appendix A, we replace the linearity assumption when $x_t<\eta$ with various alternatives, allowing, in particular for risk-aversion, risk-shifting tendencies, or disutility from bankruptcy.

Case 2: sponsoring company always contributes

If the sponsoring company always contributes then the funding ratio dynamics is given by

$$\begin{aligned} X_{t+1} = R_{t+1} \frac{X_t}{(1+g)}. \end{aligned}$$

(17)

Given information at time t, the funding ratio $X_{t+1}$ has a lognormal distribution: $X_{t+1} \vert X_t {\mathop {=}\limits ^{d}} \log {\mathcal {N}}$. The mean ${\mathbb {E}}_t[\log X_{t+1}]$ of the corresponding conditional normal distribution is

$$\begin{aligned} {\mathbb {E}}_t[ \log X_{t+1}] = F_t + \log \left( \frac{x_t}{x_t - CL}\right) \end{aligned}$$

(18)

where $F_t : = \mu _t - \tfrac{\sigma _t^2}{2} + \log (x_t - CL) - \log (1+g)$ and $x_t$ stands for the realization of $X_t$. The variance ${\mathbb {V}}{\mathbb {A}}{\mathbb {R}}_t[\log X_{t+1}]$ of the corresponding conditional normal distribution is $\sigma _t^2$. The utility function of the sponsoring company is

$$\begin{aligned} u(x_t) = {\left\{ \begin{array}{ll} x_t &{} {\text {if}}\, x_t < \eta , \\ \eta &{} {\text {otherwise}}. \end{array}\right. } \end{aligned}$$

(19)

The explanation of the utility function is the same as in the Case 1, except for the utility premium $Bonus \times CL$. The latter does not show up here because the sponsoring company always contributes, even if $x_t > \eta$.

Results

This section provides results for both institutional settings. An example for Case 1 is the US institutional setting where sponsoring companies stop contributing once the funding ratio is above 1: $\eta ^{US}=1$ [see Boon et al. (2018) for funding requirements]. An example for Case 2 is the Swiss institutional framework where sponsoring companies continue contributing even when the funding ratio is above 1.15: $\eta ^{CH}=1.15$.⁸

In Table 1, we list the parameters that we assume to illustrate the workings of the model (and we study the impact of changes in these parameters in what follows). To have a clear comparison, we set the market data parameters equal for the US and Swiss frameworks. We also set the time discount rate and the rate at which liabilities grow equal for both institutional frameworks. Benefits from saved contributions Bonus and the contribution as a percentage of liabilities CL are applicable only to the US framework because, unlike in the Swiss setting, in the US setting, sponsoring companies do not contribute to pension funds upon achieving the funding ratio of $\eta ^{US}=1.0$.

Table 1 Parameters for the main case of our numerical simulations

Results for case 1: US institutional framework

In this subsection, we look at the case where the sponsoring company does not contribute to the pension fund once the current funding ratio is above 1. Figure 1 shows that the optimal investment into stocks is a non-monotonic function of the current funding ratio for different lengths of investment horizon, different expected returns, and different risk-free rates. To see the intuition behind a hump-shape in Fig. 1, consider the incentives of the sponsoring company.

If the current funding ratio is below 1, we observe first a non-increasing and then a decreasing relationship between the stock investment and the current funding ratio. The lower the current funding ratio is, the riskier an investment strategy the sponsoring company chooses, so that it will achieve the funding ratio equal to 1 faster and hence will stop contributing to the pension fund sooner.

If the current funding ratio is between 1 and 1.3, then the sponsoring company does not contribute to the pension fund, but the pension fund still pays out to retirees. As a result, the mean of the conditional distribution of the future funding ratio decreases (compared to the situation where the sponsoring company continues contributing even if the funding ratio surpasses the benchmark of 1). The decrease is proportional to the contribution-to-liability ratio CL. Now, in order to keep the funding ratio above 1, the sponsoring company offsets this decrease in the mean of the conditional distribution for the future funding ratio by investing more into stocks. The relationship between the current funding ratio and the investment into stocks becomes increasing.

If the current funding ratio is above 1.3, we observe a decreasing relationship between the current funding ratio and investment into stocks. In this situation, the higher the current funding ratio is, the less risky the investment strategy the sponsoring company needs to adopt in order to keep the future funding ratio above 1.

Critically, note how the institutional framework determines the dynamics of the funding ratio [see Eq. (11)] and the conditional distribution [see Eq. (15)], in particular, the mean of the conditional distribution. If the current funding ratio is less than 1, the mean of the corresponding conditional normal distribution is $F_t + \log \left( \frac{x_t}{x_t - CL}\right)$ whereas if the current funding ratio is above 1, the mean of the corresponding conditional normal distribution is $F_t$. This difference in means of the conditional distribution causes a non-monotonic relationship (hump-shape) between the current funding ratio and investment into stocks. To verify this statement, if we set the current contribution to current liabilities ratio CL close to zero (see Fig. 2), then the hump-shape disappears. By contrast, we show in Fig. 2 that if we set the Bonus parameter to zero, then the hump shape remains. Likewise, in Appendix A, we show that if we change the utility function (allowing for risk-aversion, risk-shifting tendencies, or disutility from bankruptcy), the hump-shape persists.

Results for Case 2: Swiss Institutional Framework

In this subsection, we consider optimal asset allocation when the sponsoring company always contributes. Figure 3 shows a monotonic relationship between the current funding ratio and investment into stocks: it is first non-increasing and then decreasing. On the interval [0, 1.15], the lower the current funding ratio is, the more the sponsoring company invests into stocks in order to achieve the benchmark level of 1.15. If the current funding ratio is higher than the target level of 1.15, then the sponsoring company invests 20% of the pension fund’s wealth into stocks and 80% into bonds.

Since the sponsoring company always contributes, the funding ratio dynamics does not depend on whether the funding ratio is above or below the target level of 1.15 (see equation (17)). This means that the mean of the conditional distribution does not decrease if the funding ratio moves beyond 1.15. Therefore, there is no need for a sponsoring company to choose a more risky investment strategy to comply with the funding regulation.

Fig. 1

Optimal asset allocations for the U.S. regulatory framework for varying planning horizon (top), expected stock market return (middle), and risk-free rate (bottom). The general features of the optimal curve, in particular its hump-shape, are stable for a large parameter range

Fig. 2

Optimal asset allocations for the U.S. regulatory framework for varying benefits from saved contribution Bonus (top) and varying levels of contribution to liabilities CL. As Bonus increases, the hump shape remains. As CL decreases, the hump shape disappears

Fig. 3

Optimal asset allocations for the Swiss regulatory framework for varying planning horizon (top), expected stock market return (middle), and risk-free rate (bottom). The optimal proportion of stocks is monotonically decreasing as the funding ratio increases

Policy implications

This analysis gives rise to a simple but important policy implication. It may be optimal to treat underfunded and overfunded pension funds differently when it comes to regulation of their equity risk allocation. If the regulator wants to limit the investment strategy risk of underfunded funds, a cap on the weight of risky assets in the pension fund’s portfolio is optimal. By contrast, if the regulator wants to limit the investment strategy risk of overfunded funds, it should require that the sponsoring company keep on contributing to the pension fund.

Robustness

The main model is analyzed under the assumption of linearity of the company’s utility function in the underfunded region. However, Appendix A shows that, for Case 1 (US institutional framework), we obtain the non-monotonic relationship between the funding ratio and the asset allocation into the stocks with concave or convex utility functions, and with and without a safety net.⁹

Conclusion

The institutional framework for defined benefit pension plans affects the conditional distribution of the funding ratio which, in turn, determines the optimal investment into stocks. To illustrate the workings of our model, we consider two institutional settings. In the first one, the sponsoring company contributes if the funding ratio is below the target level set by institutions. In the second one, the sponsoring company always contributes. For each institutional context, we provide an example. The US regulation serves as an instance where the sponsoring company contributes to its pension fund if the funding ratio is below the target level. Here, the relationship between the current funding ratio and the investment into stocks is non-monotonic which is in line with empirical observations for the US. The Swiss regulation is used as an instance where the sponsoring company always contributes. Here, the relationship is monotonic. The higher the current funding ratio of the pension fund is, the less of the pension fund’s wealth the sponsoring company invests into stocks. The overall insight is that the institutional context has to be taken into account when assessing proposals for the asset allocation of pension funds.

Appendix A: Robustness check: functional form of a utility function

Up to now we have assumed that the utility of the company in the underfunded regime, i.e., when $x_t < \eta ^{US} = 1$, depends linearly on the funding ratio $x_t$. In reality, however, deviations from linearity might be natural.

In particular, we consider three sources of such non-linearity:

• Risk-averse preferences: A sponsoring company may have risk-averse preferences, leading to a concave utility function.

• “Safety net”: If a fund is severely underfunded, external funds might be added (e.g., by the government or an insurance) to save the fund. This “safety net” effectively limits potential amounts of underfunding and thus the disutility of such states. The resulting utility function is bounded from below and hence convex.

• Bankruptcy risk: A company might go bankrupt. Since a bankrupt company cannot experience disutility from the funding ratio anymore, the bankruptcy risk leads to a reduction of the low-funding-ratio effect, which we model with a convex utility function in the underfunded regime.¹⁰

In this subsection our goal is to show that, in the case where the sponsoring company does not always contribute to the pension fund, the non-monotonic relationship between the funding ratio and the allocation into stocks persists, even if we account for the risk-aversion, safety-net or bankruptcy risk.

We therefore study three plausible models: one is a classical model for constant relative risk-aversion (Model 1), one describes the “safety net” (Model 2) and one the effect of the bankruptcy probability (Model 3), see Table 2. In all three models, we adjust only the utility function for underfunded states.

• In Model 1, we replace the linear utility in the underfunded region by a constant relative risk aversion (CRRA) utility

  $$\begin{aligned} u(x_{t})= {\left\{ \begin{array}{ll} x_{t}^{1-\gamma } &{} {\text {for}}\, \gamma <1 \\ 2-x_{t}^{1-\gamma } &{} {\text {for}}\, \gamma >1 \end{array}\right. }, \end{aligned}$$

  (20)

  where $\gamma \ge 0$ is the standard risk aversion. The precise form of the utility is chosen such that $u(1)=1$ and $u(x_{t})$ is concave for $x_{t}<1$. In the limit $\gamma =0$, this corresponds to the standard model.

• In Model 2, we cut off low funding ratios by defining for $x_{t}<1$

  $$\begin{aligned} u(x_t)=\max (LB, x_t), \end{aligned}$$

  (21)

  where $LB \in [0,1]$ is the cut-off level, i.e., the funding ratio where the safety net is activated. In the limit $LB=0$, this corresponds to the standard model.

• In Model 3, the amount of the disutility in the underfunded states continuously declines as the bankruptcy risk increases resulting in

  $$\begin{aligned} u(x_t)=b(x_t-1)^2+x_t, \end{aligned}$$

  (22)

  where $b \in [0,1/2]$ denotes the strength of this effect and $b=0$ corresponds to the standard model.

Table 2 Models for non-linear utility functions in the underfunded regime

Figures 4, 5 and 6 show numerical solutions of the three models. The upper panels of Figs. 4, 5 and 6 show the utility functions while the lower panels show the optimal asset allocation, which are qualitatively similar to the linear case. To be more precise, the lower panels of Figs. 4, 5 and 6 confirm our conjecture about the “hump shape” of the optimal asset allocation (non-monotonic relationship) for the example of Case 1 where the sponsoring company does not always contribute (US). An explanation for these robust results is the following. When the funding ratio is higher than $\eta ^{US}=1$ and the sponsoring company does not contribute, the conditional distribution of the next period’s funding ratio shifts to the left and the “hump shape” arises. The precise shape of the utility in the underfunded regime does not influence the “hump shape”¹¹.

Fig. 4

Utility and resulting optimal asset allocations for the U.S. regulatory framework in Model 1 (company risk averse when fund underfunded). The amount of risky assets in the optimal allocation for underfunded states decreases with increasing risk aversion, but the overall pattern is robust even for high levels of risk aversion

Fig. 5

Utility and resulting optimal asset allocations for the U.S. regulatory framework in Model 2 (safety net below a certain funding ratio LB). The amount of risky assets in the optimal allocation increases in the underfunded regime, but the overall pattern is unaffected

Fig. 6

Utility and resulting optimal asset allocations for the U.S. regulatory framework in Model 3 (continuously increasing risk at lower funding ratio). The optimal asset allocations are very similar to the linear case studied in the previous subsections